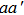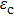† Corresponding author. E-mail:
A key issue in metallic uranium and its related actinide compounds is the character of the f electrons, whether it is localized or itinerant. Here we grew well ordered uranium films on a W(110) substrate. The surface topography was investigated by scanning tunneling microscopy. The Fermi surface and band structure of the grown films were studied by angle-resolved photoemission spectroscopy. Large spectral weight can be observed around the Fermi level, which mainly comes from the f states. Additionally, we provided direct evidence that the f bands hybridize with the conduction bands in the uranium ordered films, which is different from previously reported mechanism of the direct f–f interaction. We propose that the above two mechanisms both exist in this system by manifesting themselves in different momentum spaces. Our results give a comprehensive study of the ordered uranium films and may throw new light on the study of the 5f-electron character and physical properties of metallic uranium and other related actinide materials.
Uranium (U), being the heaviest natural element, exhibits rich physical properties:[1,2] three allotropes, anisotropic thermal expansion, a series of three low-temperature charge density wave (CDW) structural phase changes in the normal state, and a superconducting transition below 2 K.[3] It is also the only element in the periodic table with the CDW transition at ambient pressure.[4] In the normal state, the first CDW transition occurs at 43 K (


Metallic U provides a unique platform to understand the role of f electrons in the complex behavior of the actinides. Moreover, the production of epitaxial films has led to the discovery of a variety of new electronic, magnetic, and structural phenomena. The interaction of the deposited material with the substrate can lead to properties which may differ dramatically from those of the bulk. For U, it is reported that a hexagonal hcp-U phase can be obtained by deposition of thin U metal films onto the W(110) substrate,[10,11] and theoretical calculations predicted that the unusual hcp-U phase has an electronic instability, leading to a possible CDW or magnetic ordering.[12] It is also reported that different orientations of the α-orthorhombic phase can be obtained by depositing U onto a variety of buffer/seed layers on sapphire.[13,14]
A key point to understand the above exotic properties is to understand the electronic structure and f-electron character of U, whether it is localized or itinerant, which depends on the external conditions.[15] The electronic structure of α-U has been studied both experimentally and theoretically in the previous studies.[12,16,17] The Fermi surface of the α-U single crystals at ambient pressure from 0.02 K to 10 K with magnetic fields up to 35 T has been studied by torque magnetometry, and a rich set of orbits for α-U at low temperature have been observed.[16] Theoretical study of α-U/W(110) thin films has been performed by density functional theory (DFT) calculations, and it is proposed that the total density of states is dominated by the 5f states in the vicinity of the Fermi level.[12] Many-body electronic structure of metallic α-U has also been studied using a quasiparticle self-consistent GW method.[17]
Angle-resolved photoemission spectroscopy (ARPES) is a powerful tool to study the electronic states in solid materials.[18–23] Earlier pioneer ARPES measurements have been carried out on the high quality U single crystals,[24] and they found a well-ordered orthorhombic crystallographic structure representative of the bulk material.[25] The valence band structure of the α-U single crystal has been studied by ultraviolet photoemission spectroscopy and x-ray photoemission spectroscopy.[24] Later on, the band structure has been obtained by further ARPES measurements performed at 173 K.[25] For the ordered overlayers of U metal on a W(110) substrate, bandlike properties of the U 5f states were observed, which was proposed to arise from direct f–f interaction,[10] and scanning tunneling microscopy/spectroscopy (STM/STS) results further showed that the density of states close to the Fermi level is dominated by the 5f states.[11] Previous ARPES and STM studies have shed light on the electronic structure of U. However, the Fermi surface topology of either α-U single crystals or ordered U films has never been revealed by ARPES. Moreover, previous ARPES experiments carried out on the ordered films on W(110) were measured with 50–98 eV photons, with the energy resolution of about 100 meV. Since the energy scale of the heavy quasiparticles is relatively small in f-electron compounds, ARPES measurements with higher energy resolution are necessary to resolve the fine structures of the f states.
In the present study, well-ordered U epitaxial films were grown on a W(110) surface, and the surface topographies of the films were studied by STM. The Fermi surface topology and band structure of the grown films were investigated by ARPES. Comparing with previous ARPES results, we observed some fine structures of the ordered films with better energy resolution. More importantly, our results reveal direct evidence for the hybridization of the f states and conduction electrons, which is different from the previously proposed mechanism of the f–f interaction. We proposed that both the f–f interaction and f–c hybridizaiton exist in this system and manifest themselves in different momentum spaces.
Sample preparations and film growth were performed in several ultra high vacuum (UHV) chambers. These chambers are connected using a radical distribution chamber with a base pressure of 5×10−10 mbar. Ordered U films were prepared by in situ deposition onto the prepared W(110) substrate. After a long time outgassing of the U metal source, U metal was evaporated from a tungsten crucible, which was heated to about 2000 K during evaporation. The evaporation rate (3 Å/min) and the thickness of the deposited films were calibrated by the quartz oscillator. The base pressure was better than 5×10−10 mbar during evaporation. The directly deposited U film was a nonordered overlayer. After annealing at 800 K for 5 min, the ordered U films could be obtained. The samples were transferred immediately to STM and ARPES chambers under UHV conditions.
STM experiments were performed in an ultrahigh vacuum, low temperature STM apparatus with a base pressure of 5×10−11 mbar. All the measurements were performed at 78 K with an electro-chemically etched tungsten tip. All topographic images were recorded in the constant current mode. ARPES measurements were performed with a UVLS discharge lamp (21.2 eV, He-Iα light). All data were collected with a Scienta R4000 electron analyzer. The overall energy resolution was 15 meV or better, and the typical angular resolution was 0.2°. A freshly evaporated gold sample was used to determine the Fermi level.
Structurally ordered U films were grown at room temperature onto a W(110) substrate. About 70 Å U was deposited by evaporation from a tungsten crucible. A sharp hexagonal low energy electron diffraction (LEED) pattern was observed, which is consistent with the previously reported results of U films on W(110),[10] and is not compatible with any of the known bulk phases of U metal at ambient pressure (orthorhombic α, tetragonal β, and bcc γ phases). The LEED pattern suggests that this growing and annealing procedure leads to the formation of a close-packed hcp structure with an interactomic U–U distance of 3.15 ± 0.1 Å, which is in line with the results of Molodtsov et al.[10] and Bautista et al.[11] This suggests that the substrates, thickness of the films, growing and annealing conditions are especially important to obtain different phases. This is not surprising, since the two-dimensional morphology of thin films and interactions with the substrate can lead to properties which differ dramatically from those of the bulk. For thinner U films deposited on W(110), it has come to the agreement that they are in a hexagonal phase. However, if a buffer layer was added between tungsten and the U films, the orthorhombic structure of α-U can be obtained. Especially, by changing the buffer layer, the orientational relationship can also be changed.[13]
Figures 
Figure
 | Fig. 2. (a) Valence band structure of the ordered U films on W(110) taken at 20 K. (b) Angle-intergrated EDCs of panel (a). |
Besides the two sharp features, three humps can also be observed, located at the BEs of 1.3 eV, 1.5 eV, and 2.3 eV, respectively. From the band structure in Fig.
Figure
To concentrate on the fine structure near the Fermi level, Figure
 | Fig. 4. (a) Photoemission intensity plot along cut1 in Fig. |
Figure
In the previous ARPES studies of the ordered U films, the dispersion of the U 5f states was also observed, and it was proposed to be caused by direct f–f interaction, rather than by hybridization between f bands and conduction bands, which is different from the 4f-derived bands in Ce systems.[10] In our results, we have observed two kinds of dispersive bands at different momentum spaces. For the hybridization behavior in Fig.
To summarize, we have grown well-ordered U metallic films on a W(110) surface, and the topography of the grown films has been studied by STM. Further APRES results present both the Fermi surface topology and electronic structure of the ordered films. The 5f spectral weight can be observed near the Fermi level, indicating the bandlike feature of the f electrons in the ordered U films. More importantly, We have observed two kinds of hybridization behaviors in different momentum spaces, which indicates that both the f–f interaction and f–c hybridization exist and contribute in the hybridization process. Our results may shed light on the understanding of the 5f-electron character and physical properties in this and other actinide compounds.
| [1] | |
| [2] | |
| [3] | |
| [4] | |
| [5] | |
| [6] | |
| [7] | |
| [8] | |
| [9] | |
| [10] | |
| [11] | |
| [12] | |
| [13] | |
| [14] | |
| [15] | |
| [16] | |
| [17] | |
| [18] | |
| [19] | |
| [20] | |
| [21] | |
| [22] | |
| [23] | |
| [24] | |
| [25] | |
| [26] | |
| [27] | |
| [28] | |
| [29] | |
| [30] |