† Corresponding author. E-mail:
Project supported by the Key Research Program of Frontier Sciences of Chinese Academy of Sciences (Grant No. Y7Y1472Y61), the National Natural Science Foundation of China (Grant Nos. 11205123, 11574329, 11774358, 11747601, and 11675017), the Joint NSFC-ISF Research Program (Grant No. 51561145002), the CAS Biophysics Interdisciplinary Innovation Team Project (Grant No. 2060299), the CAS Strategic Priority Research Program (Grant No. XDA17010504), and the Fundamental Research Funds for the Central Universities (Grant No. 2017EYT24).
We proposed a modified ratchet model including power-stroke and elastic coupling to study the efficiency of collective non-processive motors such as myosin II in muscle. Our theoretical results are in good agreement with the experimental data. Our study not only reveals that the maximum efficiency depends on elasticity and is independent of transition rates but also indicates that the parameters fitted to fast muscle are different from those fitted to a slow one. The latter may imply that the structure of the fast muscle is different from that of the slow one. The main reason that our model succeeds is that velocity in this model is an independent variable.
The efficiencies of processive motors such as FoF1-ATPase and kinesin have been investigated.[1–6] The efficiency of FoF1-ATPase approaches nearly 100% due to the elastic rotor γ subunit,[2] while that of kinesin-1 also reaches at 70% because neck-linker will extent elastically during docking.[5] However, it is very difficult for non-processive motors such as myosin II to be studied to determine their efficiency at single molecule level due to low duty ratio. Fortunately, most non-processive motors work collectively; for example, the contraction of muscle results from the cooperation of large amount of myosin II motors.[7–14] The elementary unit of muscle is sarcomere, which is composed of regular arrays of thick filament and thin filament.[8,15–17] Each thick filament is self-assembled with a large number of myosin II motors. These motors “walk along” the thin filaments, which leads to relative sliding between thick filament and thin one. The contraction of muscle results from this sliding.
Some researchers have theoretically studied the efficiency of muscle based on kinetic models,[12,18–20] and their results are consistent with experiments.[21–25] Jülicher et al. also studied the efficiency of collective motors based on a simplest ratchet model.[26] In the ratchet model, the motors are simplified as Brownian particles which diffuse among periodic potentials and transit between the potentials.[26–31] In the kinetic models, the motors transit directly between different states with different rates and the detailed diffusion is not considered. The ratchet model, however, involved more physical information.
In this paper, motivated by experimental results,[21] we add an elastic element, which corresponds the long coiled coil stalk[32,33] with stiffness about 1.5 pN/nm∼ 3 pN/nm,[11,33–35] and a power-stroke, which corresponds the cross-bridge so that localized the transition of particle between states, into the ratchet model to study the efficiency of collective myosin II motors.
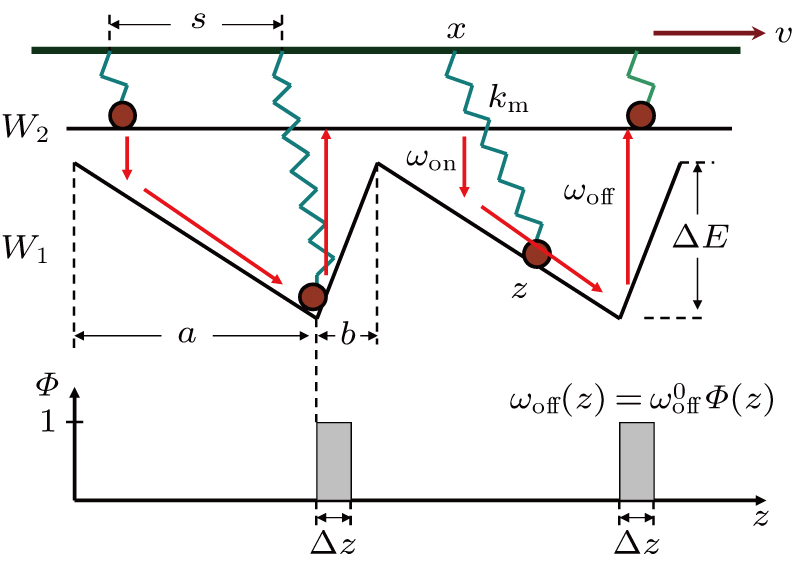
We first recall the ratchet model under consideration of motor elasticity.[36] In this model, motors are elastically coupled to a backbone with periodical spacing s by a springs (with a stiffness of km) as shown as Fig. 
At the molecular level, muscle contraction is the sliding between a thick filament and a thin one, which is cyclically driven by myosin cross-bridges (which consists mainly of myosin head, lever arm and elastic stalk) between the two filaments. The energy outputted by a single cross-bridge during a cycle of attachment is coupled to the hydrolysis of one molecule of ATP. The ‘mechanical’ power-stroke of the attached cross-bridge can reach 5 nm∼ 10 nm in each cycle, as shown in Fig.
According to Fig., we propose a modified model (Model II) as shown in Fig. 

Furthermore, we simplify the potential W1 into a piecewise one. There is a platform and a bottom in each period of W1, the platform corresponds to the weak bound pre-power-stroke state of myosin and is denoted by “A”, while the bottom corresponds to post-power-stroke state and is denoted by “B”. Therefore, the transition from “A” to “B” is the power-stroke process. After a power-stroke, the motor can detach from the actin filament and lever arm reprimes by a recovery power-stroke, the next cycle then begins. This idea is consistent with the cross-bridge model of muscle.[7,9]
We define the probability density pi(x,z,t) for a motor with its tail at x and head at z in the state i (i = 1,2), which obey the following coupled Fokker–Planck equations[36]
The average external force applied on one motor is given by[36]
The efficiency of collective motors that are elastically coupled is an important property at steady state. For comparison with experimental results,[21] it can be defined as
The numerical results of Eq. (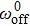


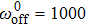
 | Fig. 3. (color online) (a) The efficiency of collective motor {vs} velocity for different motor stiffness at 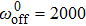   |
Duty ratio of collective motors can be defined as

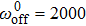
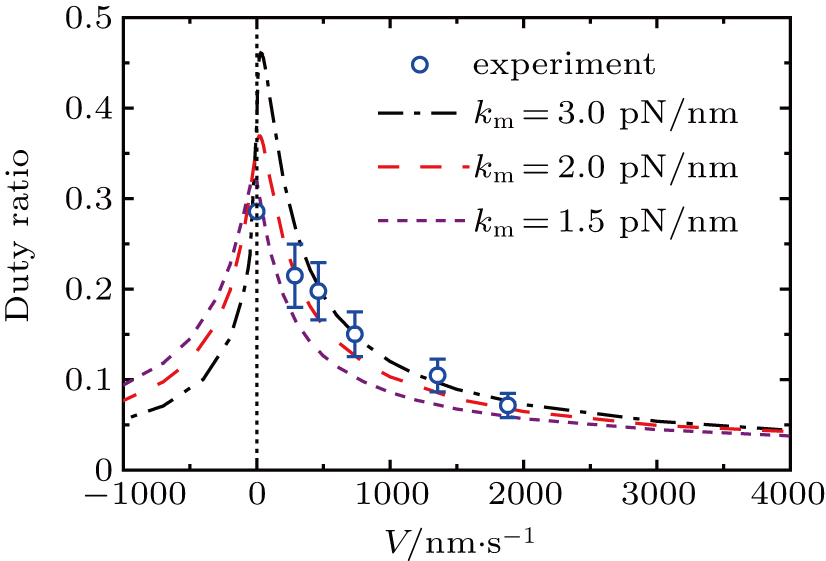 | Fig. 4. (color online) Duty ratio of collective motors versus velocity for different motor stiffness at  |
We have lumped the ratchet model, power-stroke and elastic coupling together into Model II. The relation between efficiency and velocity, as well as the relation between duty ratio and velocity has been investigated. The experimental data has been successfully explained. The reasonable parameters such as km and 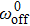
The mean velocity is the main variable that has to be calculated in study of collective motors. For example, the mean field method can be engaged to study efficiency of the rigid coupling collective motors if the number of motors (N) is large enough and s is incommensurate with l,[27,31] otherwise, N-coupling-equations have to be numerically simulated if N is limited.[45,46] However, the mean velocity here is independent variable. In Model II, the cooperation among motors has been involved in Fext. This may be a convenient method to estimate the efficiency. Theoretical results revealed that it is the elasticity, local transition and reflection boundary that improve the efficiency of collective elastic coupling motors. It must be pointed that the final results does not depend on the value of l; i.e., it is flexible (for example, l = 8 nm for microtubule and l = 36 nm for actin filament) because of 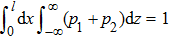
| [1] | |
| [2] | |
| [3] | |
| [4] | |
| [5] | |
| [6] | |
| [7] | |
| [8] | |
| [9] | |
| [10] | |
| [11] | |
| [12] | |
| [13] | |
| [14] | |
| [15] | |
| [16] | |
| [17] | |
| [18] | |
| [19] | |
| [20] | |
| [21] | |
| [22] | |
| [23] | |
| [24] | |
| [25] | |
| [26] | |
| [27] | |
| [28] | |
| [29] | |
| [30] | |
| [31] | |
| [32] | |
| [33] | |
| [34] | |
| [35] | |
| [36] | |
| [37] | |
| [38] | |
| [39] | |
| [40] | |
| [41] | |
| [42] | |
| [43] | |
| [44] | |
| [45] | |
| [46] |