Anomalous Hall effect (AHE) has continually received a certain amount of attention in both fundamental research and device application for decades.^{[1– 5]} The Hall resistivity in a magnetic thin film consists of two terms: one represents the ordinary Hall effect and the other represents the AHE, which is much larger than the former. The current understanding of AHE's mechanism in a clean ferromagnetic material is focused on three proposed mechanisms:^[4] the intrinsic mechanism, ^{[5, 6]} extrinsic skew-scattering (SS), ^[7] and side-jump (SJ).^[8] The intrinsic AHE was first interpreted to stem from spin– orbit coupling in the crystal band structure, ^[6] but now has been reinterpreted in terms of the Berry curvature of the occupied Bloch states.^[5]

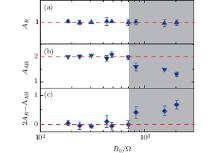
In general, a scaling relation between the anomalous Hall resistivity (ρ _AH) and longitudinal resistivity (ρ _xx)

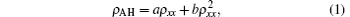
is approximately valid, and it is used to investigate the origin of AHE.^[9] Here, the first term represents the contribution of SS and the second term represents the contribution of SJ. However, the reduced dimensionality can induce quantum correction on AHE, ^{[10– 14]} particularly in the presence of disorder. The resistivity shows an obvious minimum at low temperature due to the weak localization (WL) effect.^{[13, 14]} Thus, the above simple scaling relation between ρ _AH and ρ _xx is not valid in the whole temperature region. So far, there has been no systematic study of the AHE mechanism in highly disordered thin film.

Here we have investigated the anomalous Hall effect in CoFeB thin film with thickness down to 1.7  nm. The reason we chose CoFeB is that it is the most promising candidate to serve as the magnetic sensing layer in spintronic devices.^{[15– 17]} However, CoFeB thin film deposited using a standard sputtering process is always amorphous and shows a resistivity minimum at low temperature.^[18] Thus the effect of quantum correction should be considered when the device works at low temperature. In this paper, we report an obvious weak localization correction to anomalous Hall conductance (AHC) when the film is thinner than 3  nm. We also find that both the weak localization to AHC and the mechanism of the anomalous Hall effect in CoFeB are related to its thickness.

Amorphous Co₄₀Fe₄₀B₂₀ film samples (1.7  nm– 10.5  nm) were deposited on thermally oxidized silicon wafer by DC magnetron sputtering with a base pressure of 5× 10^{− 6}  Pa. The sputtering details can be seen elsewhere.^[17] All the CoFeB films were capped with a 3-nm-thick MgO to prevent oxidation. High purity MgO (99.99%) and Co₄₀Fe₄₀B₂₀ (99.9%, Functional Materials International, Japan) were used as the target materials. The layer thickness was identified by an x-ray reflectometer (XRR, Brucker D8) and confirmed by XRR obtained at 1  W 1  A, Beijing Synchrotron Radiation Facility (BSRF). Hall-bar patterned films were used for the resistivity and Hall measurements from 5  K to 300  K with a Quantum Design® physical property measurement system (PPMS). To exclude the ordinary Hall effect, we define anomalous Hall resistivity as ρ _AH, which is obtained as the zero field extrapolation of the high field.

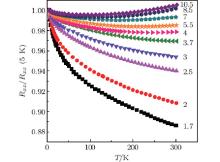
Figure  1 shows the temperature dependence of the normalized sheet resistance R_xx/R_xx (5  K) for the CoFeB films down to 1.7  nm. Similar to the traditional amorphous metallic alloys, ^[19] the temperature dependence of R_xx for the CoFeB thin film shows an obvious resistance minimum at low temperature, which is accompanied by a change from a negative temperature coefficient of resistivity (TCR) to a positive TCR. Figure  2 shows the representative temperature dependence of R_xx/R_xx (5  K), which can be further fitted by using a single logarithmic form, ln(T/T₀), in the temperature ranging from 5  K to 50  K. This indicates the WL effect in the amorphous CoFeB thin films at low temperature. A similar characteristic has already been found in these highly disordered thin films.^{[11, 14]}

Fig.  1.

	Figure Option ViewDownloadNew Window
	Fig.  1. Temperature dependence of the normalized Rxx for CoFeB films.

Fig.  2.

	Figure Option ViewDownloadNew Window
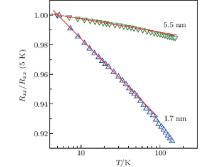	Fig.  2. Temperature dependencies of Rxx/Rxx (5  K) for CoFeB of 1.7  nm (up triangles) and 5.5  nm (down triangles). The solid lines are fitted by using a single logarithmic form, ln(T/T0).

In order to further study the WL effect in amorphous CoFeB thin films, we define the normalized relative change following the work in Refs.  [10] and [11], Δ ^N(Q_ij) = (1/L₀₀R₀)(δ Q_ij/Q_ij), with respect to our reference temperature T₀ = 5  K < T, where δ Q_ij = Q_ij(T)− Q_ij(T₀) and Q_ij refers to either resistance R_xx, R_xy (R_AH) or conductivity σ _xx, σ _xy (σ _AH). Here, we defined σ _xx≅ 1/ρ _xx and 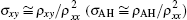since ρ _xy, ρ _AH ≪ ρ _xx in our case. Thus,

Due to δ R_xx ≪ R and R_AH ≪ R_xx, the anomalous Hall conductance σ _AH obeys

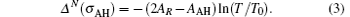
Figure  3 shows the representative temperature dependencies of Δ ^N(σ _AH), together with Δ ^N(R_xx) and Δ ^N(R_AH) for comparison. Apparently, both Δ ^N(R_xx) and Δ ^N(R_AH) obey the dependence of − ln(T/T₀). However, Δ ^N(σ _AH) shows different temperature dependences, i.e. Δ ^N(σ _AH) ≈ 0 for R₀ = 308  Ω (t = 5.5  nm), but increases with temperature for R₀ = 2.0  kΩ (t = 1.7  nm). For the former film, the relation of 2A_R ≈ A_AH is valid. Here R₀ represents the sheet resistence at 5  K. Similar relations of 2A_R = A_AH and Δ ^N(σ _AH) = 0 have been reported for the Fe granular film with R₀ = 140  Ω .^[11] For the latter film, the temperature dependencies of Δ ^N(R_xx) and Δ ^N(R_AH) nearly overlap each other, indicative of A_R ≈ A_AH ≈ 1, an overlap quite similar to that in the previous granular Fe film with high disorder (R₀ = 2.7  kΩ ).^[11]

Fig.  3.

	Figure Option ViewDownloadNew Window
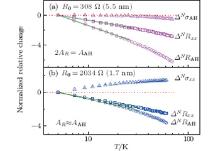	Fig.  3. Normalized relative change Δ NRxx (blue open triangles), Δ NRAH (red open squares), and Δ ^Nσ AH (green open circles) for R0 = 308  Ω (a) and 2034  Ω (b) respectively. The dashed lines are guides for the eye. The solid lines are results of fitting by using Eq.  (2).

Figure  4 shows the three fitting parameters, A_R, A_AH, and 2A_R − A_AH, for CoFeB thin films with R₀ varying from 184  Ω to 2.0  kΩ . A_R is always equal to unity whereas the R dependencies of A_AH and 2A_R − A_AH are separated as two parts. In the range of R₀ ≤ 700  Ω (t ≥ 3  nm), the WL correction to AHC is negligible. For thin CoFeB films (t < 3  nm), the prefactor of Δ ^Nσ _AH, 2A_R − A_AH is nonzero, which indicates the WL correction to AHC. The thickness-dependence of the WL correction behavior suggests that the AHE mechanism is thickness-dependent. Indeed, a theory based on a granular model of AHC predicts that the SS and SJ mechanisms have different roles in the localization corrections.^{[11, 20– 22]} In this theory, ^[11] the WL correction to AHC is described by

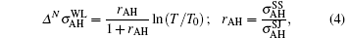
where r_AH is the ratio of two different mechanisms contributing to AHE, namely, the side jump and skew scattering . As seen from Eq.  (4), vanishes for the SJ mechanism (r_AH≪ 1), but is nonzero due to the SS (r_AH≫ 1).

Fig.  4.

	Figure Option ViewDownloadNew Window
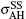
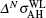
	Fig.  4. Dependence of the coefficients AR (a) and AAH (b) as defined in Eq.  (2), and of 2AR − AAH (c) on the sheet resistance R0. The dashed lines are guides for the eye. The grey range indicates WL corrections to AHC.

As we mentioned above, the AHE mechanisms can be generally determined by the simple relation between ρ _AH and ρ _xx. If the sample has a high Curie temperature, such as Fe, the AHE mechanisms can be directly investigated from the relationship between ρ _AH and ρ _xx.^[9] Otherwise, a temperature-dependent factor f(T) should be considered.^[4] Thus, the determination of the AHE mechanism is strongly related to the accuracy of magnetization measurement of the samples. As for the CoFeB thin films, f(T) is almost equal to 1 at low temperature.^[23] We can neglect the effect of the temperature-dependent factor if we only focus on the temperature region of the WL effect, which is normally below 40  K in our case. In other words, equation  (1) is valid when T < 40  K. Because ρ _AH ≪ ρ _xx equation  (1) is also rewritten as

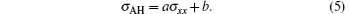
The first term comes from the SS mechanism and the second from the SJ mechanism.

Figure  5 shows the disorder factor R₀ dependence of r_AH. The representative σ _xx dependencies of σ _AH at low temperature are plotted in the inset. The solid lines are the results of fitting by using Eq.  (5). It is readily apparent that the relationship between σ _AH and σ _xx is thickness-dependent. For the very thin film, for example t = 2  nm, both the SS and SJ mechanisms contribute to the AHC. On the other hand, it is almost scattering-independent for the thick film. Thus, the solid squares in Fig.  4 are the ratio of . The open circles are directly calculated from the temperature dependence of Δ ^Nσ _AH. It is clear that the thickness dependence of WL correction behavior in CoFeB thin films can be well described by the granular model of WL correction to AHC.^{[11, 20– 22]} This is to say that the SJ mechanism dominates the AHE when t ≥ 3  nm (R₀ ≤ 700  Ω ). Meanwhile, the WL correction to AHC vanishes. As for very thin CoFeB films (t < 3  nm), it is found that A_AH < 2. 2A_R − A_AH increases from 0 to 0.68, i.e. the WL corrections to AHC appear. This is consistent with the fact that the two mechanisms, SJ and SS, give comparable contributions due to the onset of granularity in the high disorder regime.

Fig.  5.

	Figure Option ViewDownloadNew Window
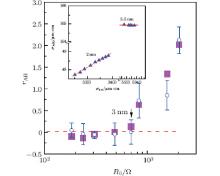	Fig.  5. The disorder factor R dependence of rAH. The solid squares are the ratio of 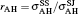, and they are fitted by using Eq.  (5). The open circles are calculated from the temperature dependence of Δ Nσ AH. The inset shows the representative σ xx dependencies of σ AH at low temperature for t = 2  nm and 5.5  nm.

In summary, an obvious weak localization correction to anomalous Hall conductance in very thin CoFeB film is reported. We find that in CoFeB films, both the weak localization to AHC and the mechanism of the anomalous Hall effect are related to the film thickness. When the film is thicker than 3  nm, the side jump mechanism dominates and the weak localization to AHC vanishes. Otherwise, both side jump and skew scattering mechanisms contribute to the anomalous Hall effect and the weak localization correction to AHC is observed for very thin CoFeB films.