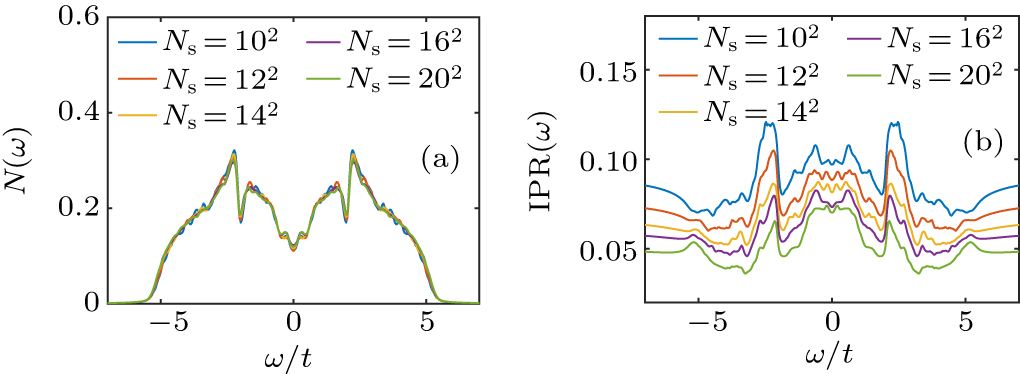
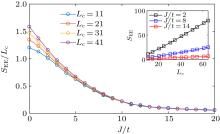
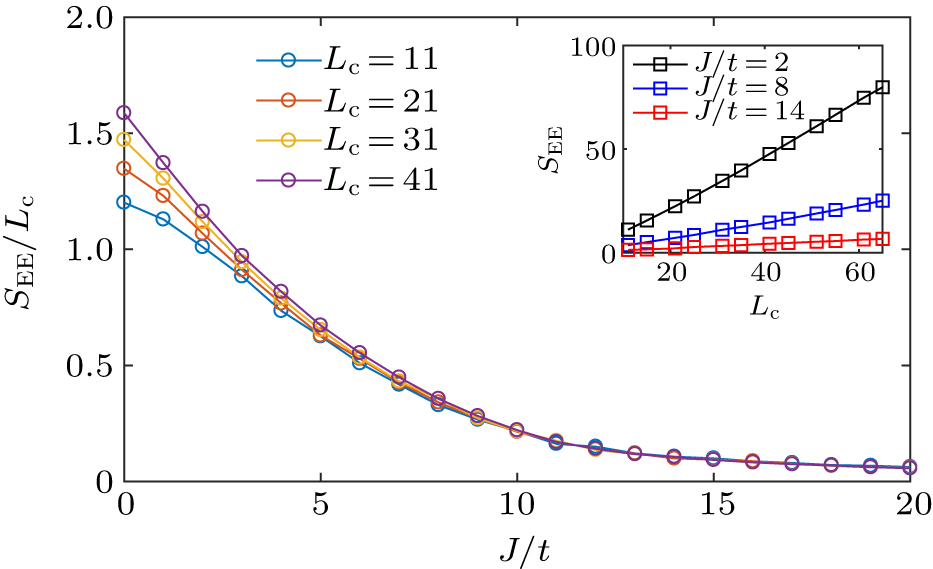
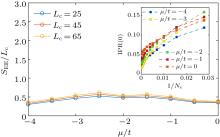
中国物理B ›› 2020, Vol. 29 ›› Issue (10): 107301-.doi: 10.1088/1674-1056/ab99b0
Wei-Wei Yang(杨薇薇)1, Lan Zhang(张欄)1, Xue-Ming Guo(郭雪明)1, Yin Zhong(钟寅)1,†( )
)
Hidden Anderson localization in disorder-free Ising–Kondo lattice
Wei-Wei Yang(杨薇薇), Lan Zhang(张欄), Xue-Ming Guo(郭雪明), and Yin Zhong(钟寅)†
- 1
School of Physical Science and Technology & Key Laboratory for Magnetism and Magnetic Materials of the MoE, Lanzhou University , Lanzhou 730000,China