† Corresponding author. E-mail:
Project supported by the National Natural Science Foundation of China (Grant No. 51971178), the Astronautics Supporting Technology Foundation of China (Grant No. 2019-HT-XG), the Natural Science Foundation of Shaanxi Province, China (Grant No. 2019JM-344), the Russian Science Foundation (Grant No. 20-62-46003), and the Fundamental Research Funds for the Central Universities, China (Grant Nos. 3102019ghxm007 and 3102017JC01003).
A relationship between thermal effects and relaxation of the high-frequency shear modulus upon heat treatment of bulk Zr48(Cu5/6Ag1/6)44Al8 metallic glass is found. This relationship is attributed to the relaxation of a interstitial-type defect system frozen-in from the melt upon glass production. Calorimetric data show that thermal effects occurring on heating include heat release below the glass transition temperature, heat absorption above it and heat release caused by crystallization. The equation derived within the Interstitialcy theory can be used to calculate the shear modulus relaxation using the calorimetric data. The obtained results are used to trace the defect concentration as functions of temperature and thermal prehistory.
Elastic moduli, which essentially reflect interatomic forces, constitute key physical parameters of metallic glass. In particular, an important but still unresolved problem is why the shear modulus G and Young’s modulus E of metallic glasses are reduced by about 20% to 40% as compared with the maternal crystalline state.[1] It is believed that this phenomenon is related to the structural heterogeneity or internal defects of metallic glasses.[2] In fact, the microstructural heterogeneity of metallic glass has been extensively investigated in recent years.[3–5] It should be emphasized that the shear modulus is tightly related to the structural origin of plastic deformation and glass transition in metallic glasses.[6–8] In recent years, many investigations were focused on correlations among the shear modulus, glass forming ability, glass transition and mechanical properties of metallic glasses.[2,9–11]
On the other hand, the shear modulus is an important physical parameter of the Interstitialcy theory (IT) proposed by Granato.[10,12] The theory is based on an experimental observation that interstitial defects produced by soft neutron irradiation at T = 4 K result in a sharp decrease of the shear modulus of single crystalline Cu.[13] The extrapolation of these experimental data shows that the shear modulus should drop to nearly zero if the concentration of interstitial defects can reach 2% to 3%. Meanwhile, it is known that a vanishing shear modulus is a signature of liquids.[13] Although interstitial defects in crystalline materials can be understood as two atoms trying to occupy the same lattice position, these defects do not have such a clear geometric structure in amorphous materials. However, it is still considered that the interstitial defects in glass retain their basic characteristics in the crystalline state, such as high sensitivity to the external shear stress, specific low-frequency vibration modes and high vibrational entropy.[14,15] These defects remain identifiable structural units in the melt becoming frozen in solid glass upon melt quenching. Diverse relaxation phenomena occurring upon structural relaxation and crystallization in metallic glasses can be quantitatively attributed to thermoactivated changes of the defect concentration.[16,17] It is important, therefore, to trace how this quantity changes due to different heat treatment procedures.
Within the framework of the Interstitialcy theory, the shear modulus of a metallic glass is determined by the shear modulus μ of maternal crystal (i.e., the one used for glass production) and the defect concentration c,[12]



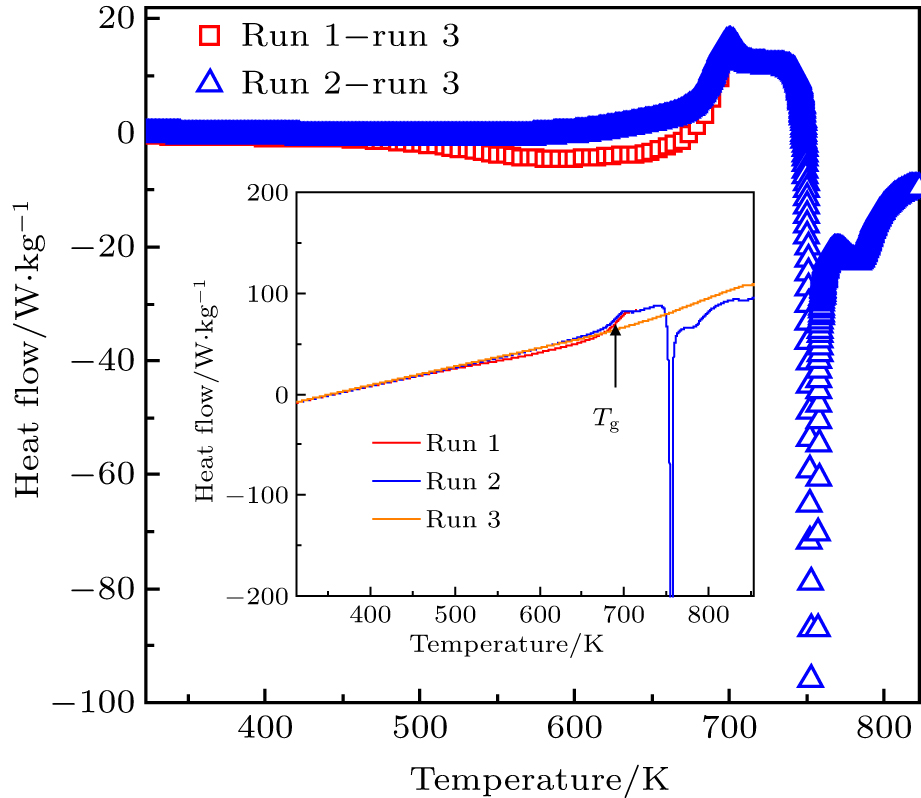
In the present study, model glassy Zr48(Cu5/6Ag1/6)44Al8 (at.%) prepared by melt suction technique was selected for the investigation. The glassy nature of samples was confirmed by x-ray diffraction (XRD, Rigaku D/max 2500 X). Thermal properties were determined by differential scanning calorimetry (Hitachi DSC 7020) carried out in flowing high purity N2 at a heating rate of 3 K/min.
Electromagnetic acoustic transformation (EMAT) method was used for the high-frequency f (≈ 600–700 kHz) shear modulus measurements of 5 mm× 5 mm× 2 mm samples. In this method, transverse vibration of a sample is excited as a result of the Lorentz interaction of external magnetic field and surface current of a metallic sample.[22] The relative error in frequency measurements was estimated to be about 100 ppm near the glass transition temperature Tg and by an order of magnitude smaller well below Tg.[19] The shear modulus was calculated by 
Integrating Eq. (

Figure
Figure
Figure
The agreement between the experimental and calculated shear modulus data shown in Fig.

Figure
In the relaxed state, the right-hand side of Eq. (
Equation (

In this work, we show that temperature dependence of the shear modulus of Zr48(Cu5/6Ag1/6)44Al8 metallic glass can be quantitatively predicted by using calorimetric data. This fact provides the evidence that the origin of structural relaxation can be related to a thermoactivated change of the concentration of interstitial-type defects assumed to be responsible for the relationship between the shear modulus and heat effects. Upon heating the initial glass, the defect concentration first decreases due to structural relaxation below Tg, which is accompanied by the heat release. This effect disappears in the relaxed state obtained by heating into the supercooled liquid state. Upon approaching Tg, the defect concentration start to rapidly increase both in the initial and relaxed state leading to the heat absorption. Heat release is determined by the release of the total formation enthalpy of the defects disappeared upon structural relaxation below Tg. Heat absorption near and above Tg corresponds to the absorption of the total formation enthalpy of defects generated at these temperatures. All these physical processes are reflected in the changes of the shear modulus of glass. The latter constitutes a major thermodynamic parameter of metallic glass structure.
| [1] | |
| [2] | |
| [3] | |
| [4] | |
| [5] | |
| [6] | |
| [7] | |
| [8] | |
| [9] | |
| [10] | |
| [11] | |
| [12] | |
| [13] | |
| [14] | |
| [15] | |
| [16] | |
| [17] | |
| [18] | |
| [19] | |
| [20] | |
| [21] | |
| [22] | |
| [23] | |
| [24] | |
| [25] |