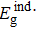† Corresponding author. E-mail:
Project supported by the Deanship of Scientific Research, Taif University, Kingdom of Saudi Arabia (Grant No. 1-440-6136).
In this study, CdS/ZnO (2:3 mol%) thin films are successfully deposited on quartz substrates by using the sputtering technique. Good images on the structural and optical characteristic features of CdS/ZnO thin films before and after annealing are obtained. The CdS/ZnO thin films are annealed respectively at temperatures of 373 K, 473 K, and 573 K and the structural features are examined by XRD, ATR-FTIR, and FESEM. The optical properties of CdS/ZnO thin films such as refractive indices, absorption coefficients, optical band gap energy values, Urbach energy values, lattice dielectric constants, and high frequency dielectric constants are determined from spectrophotometer data recorded over the spectral range of 300 nm–2500 nm. Dispersion parameters are investigated by using a single-oscillator model. Photoluminescence spectra of CdS/ZnO thin films show an overall decrease in their intensity peaks after annealing. The third-order nonlinear optical parameter, and nonlinear refractive index are also estimated.
In recent years, the binary nanocomposites materials have received much attention of scientists due to their distinguished performances in microelectronic, optoelectronic devices, photocatalysts, and optics. Binary nanocomposites such as cadmium sulphide–zinc oxide (CdS/ZnO) shows good stable transport properties and has many interesting applications in optoelectronic devices.[1–7] Coupling of CdS/ZnO nanostructures has been continuously attractive and produced innovative prospects for numerous modern optoelectronic devices. Thin films consisting of (CdS/ZnO) nanocomposites have been widely explored due to their applications in the area of optoelectronic, photo-catalysis and optics.[8–12]
The CdS possesses a narrow direct optical band gap (∼ 2.4 eV) and is extensively used as a visible light photocatalyst. The CdS is the most appropriate visible sensitizer for zinc oxide (ZnO) because its crystal lattice constant is similar to that of ZnO, having the optical band-gap energy in the visible light region creating a heterojunction with zinc oxide, which enables a very fast interband electronic charge transfer between these compounds. The electronic charge injection from ZnO to CdS produces an efficient electronic charge separation due to a decrease in the exciton recombination; one of the excitons is bounded to the ZnO and the other to the coupled CdS.[13,14] The CdS is suitable to constructing the core-shell heterostructures with ZnO, including one-dimensional core–shell heterostructure, which are the most promising heterostructures for photo-catalytic and solar energy applications.[15–21] In the CdS/ZnO nanostructures, ZnO is a wide optical band gap in the range of ∼ 3.34 eV. It is responsible for a charge separation that suppresses the recombination process.[22] The CdS/ZnO nanostructures also have better physicochemical characteristics than the constituents. For example, the conductivity of CdS/ZnO nanostructure is better than that of pure ZnO nanorod.[23,24]
Structural and optical characteristic features of nanostructured CdS/ZnO thin films have been previously investigated by many researchers.[25–28] They reported that the optical properties and the optical band gaps of the nanostructured CdS/ZnO thin films can be controlled by the content ratio.
In the present study, we focus our attention on the influence of the annealing temperature on microstructural (FTIR, XRD, EDX and FESEM), optical, and photoluminescence properties of binary nanocomposites materials (CdS/ZnO) thin films.
The CdS/ZnO thin films were deposited on quartz substrates by using the UNIVEX 350 sputtering coating technique. The deposition parameters of CdS/ZnO thin films are listed in Table
| Table 1. Experimental parameters of sputtering-deposited CdS/ZnO thin films. . |
The chemical bonds of the CdS/ZnO thin films were estimated using attenuated total reflection Fourier transform infrared (ATR-FTIR) spectrometer (Bruker, Germany) with using reflection mode. The attenuated total reflection Fourier transform infrared (ATR-FTIR) spectra of the CdS/ZnO thin films were obtained in the spectral range from 4000 cm−1 to 450 cm−1. FESEM analysis of transparent CdS/ZnO thin films was done using SEM Model Quanta 250 field emission gun, FEI Company, Netherlands. The crystalline structures of CdS/ZnO thin films were examined by x-ray diffraction (XRD) using a D8 Advance (Bruker, USA) x-ray diffractometer with Cu Kα (λ = 1.54056 Å) operated at 40 kV and 40 mA. The scanning angular range was from 7° to 90° in 2θ.
The optical data (transmittance and reflectance) were taken by using a V-670 JASCO spectrophotometer that covers wavelengths of 300 nm–2500 nm. The V-670 JASCO spectrophotometer was connected with a constant angle specular reflection accessory (5°). The optical quantities n (real part of the refractive index) and k (extinction coefficient) of the CdS/ZnO thin films are calculated using the same methods as that reported in Refs. [29–32].
As reported in Ref. [33], the experimental errors in measuring the film thickness and the T (or R) parameter were estimated at ±2% and ± 1%, respectively; whereas, the errors in measuring n and k were less than ±4%. A JASCO (FP-8200A) specrofluorometer with λexcitation = 300 nm was used to investigate the emission spectra of the as-deposited and thermally annealed CdS/ZnO thin films.
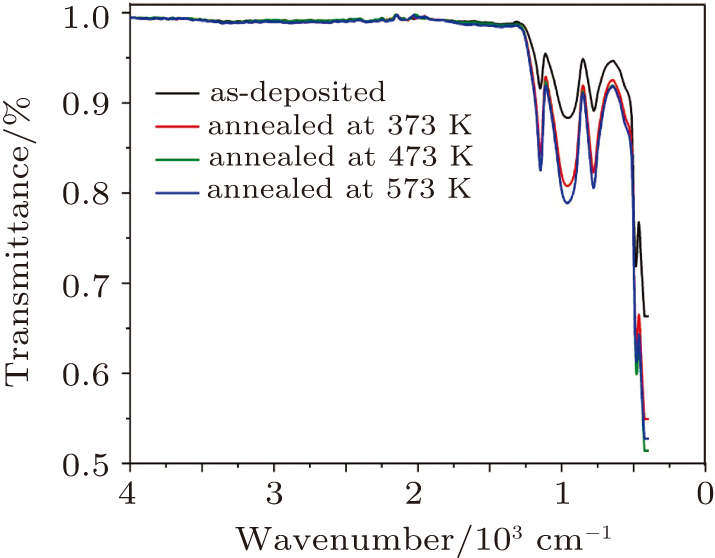
The FT-IR spectra of CdS-ZnO for the as-deposited and thermally annealed CdS/ZnO thin films are shown in Fig.
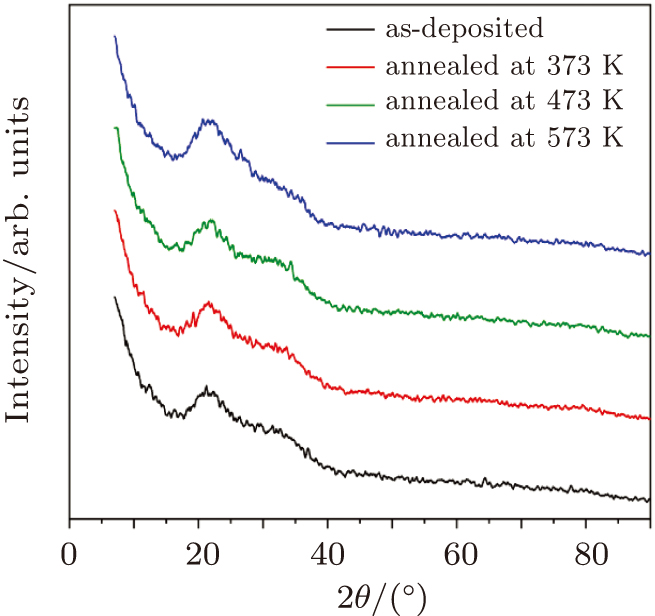
Figure
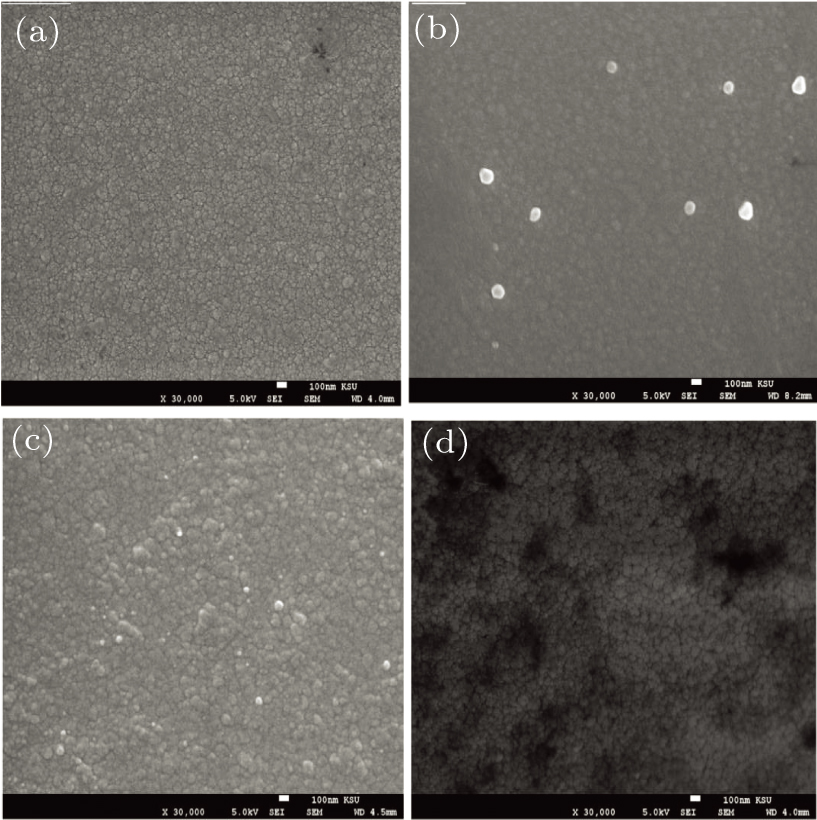
Figure
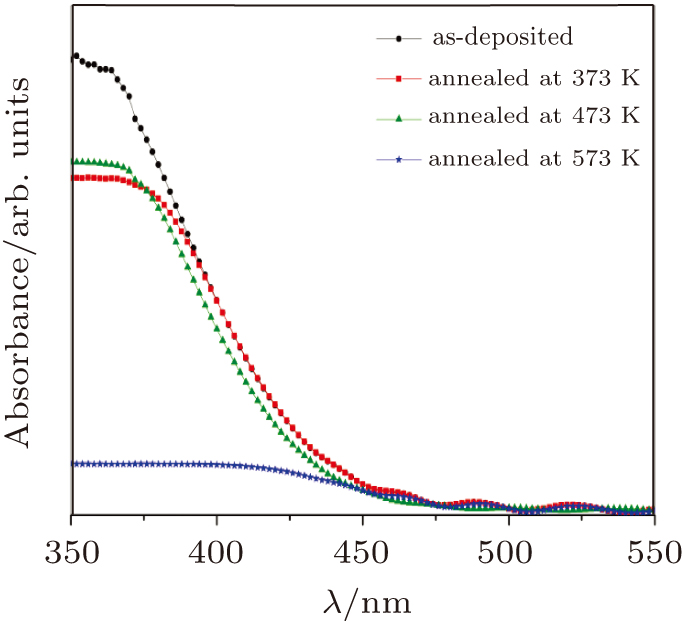
The absorbance spectra of the as-deposited and thermally annealed CdS/ZnO thin films are presented in Fig.
Figure
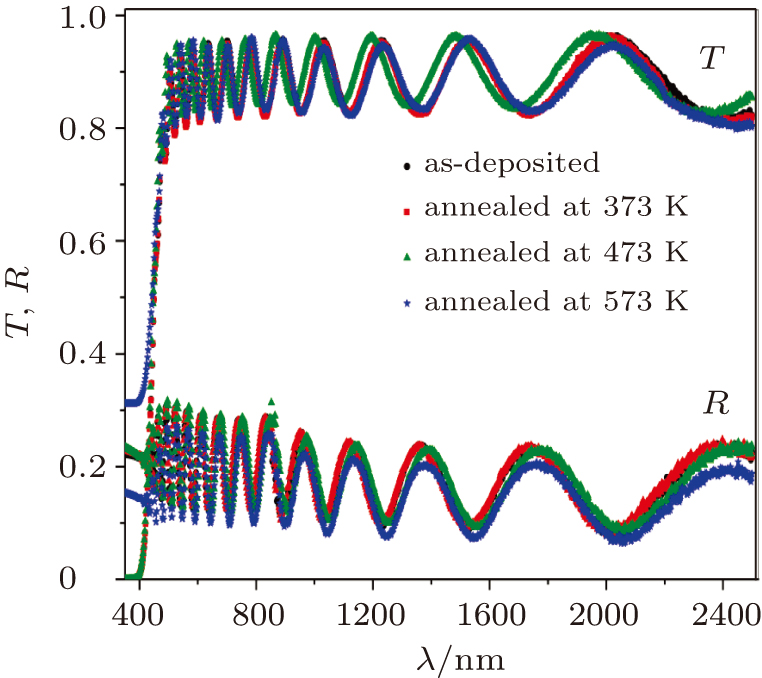 | Fig. 5. Transmittance and reflectance spectra of asdeposited and thermally annealed CdS/ZnO thin films. |
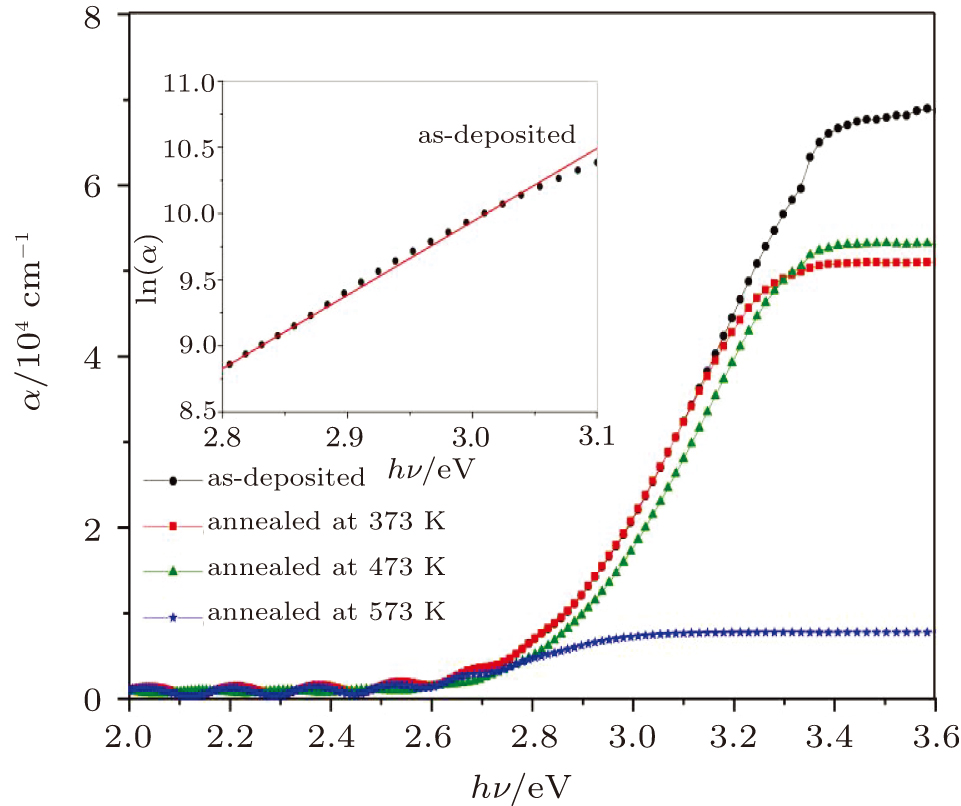
The variations of absorption coefficient α with wavelength (where α = 4πk/λ) for the as-deposited and thermally annealed CdS/ZnO thin films are shown in Fig.
In the lower energy region, where the absorption coefficients vary exponentially with hν, the exponential dependence of the absorption at the band edge is due to the perturbation of the density of the states (DOS). The spectral dependence of the absorption edge follows the Urbach energy (Eu), which describes the width of the absorption tail as given in the following expression:[38]
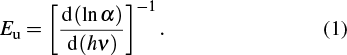
| Table 2. Optical parameters of transparent CdS/ZnO thin films. . |
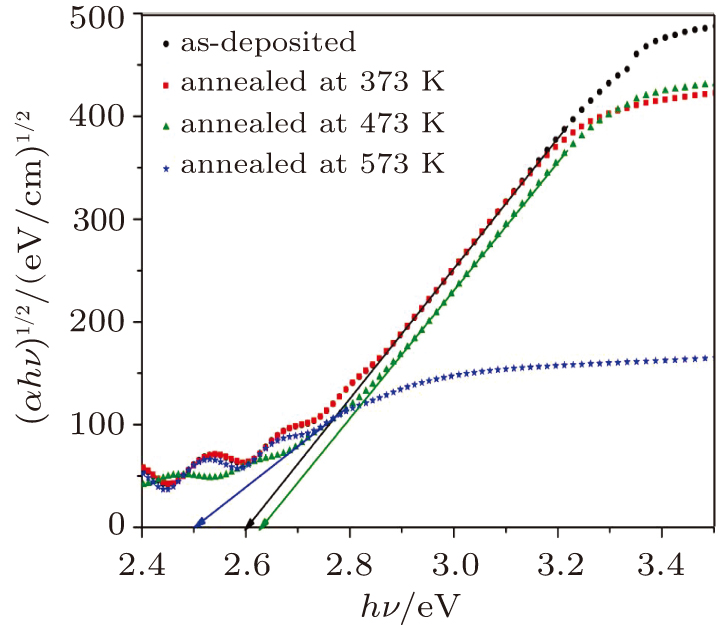
The optical band gap energy values of the as-deposited and thermally annealed CdS/ZnO thin films and the type of optical electronic transitions are assessed from the examination of the absorption coefficient, α, versus hν nearby the fundamental absorption edge by using the allowed indirect transitions expression:[39]
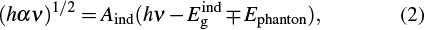
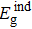
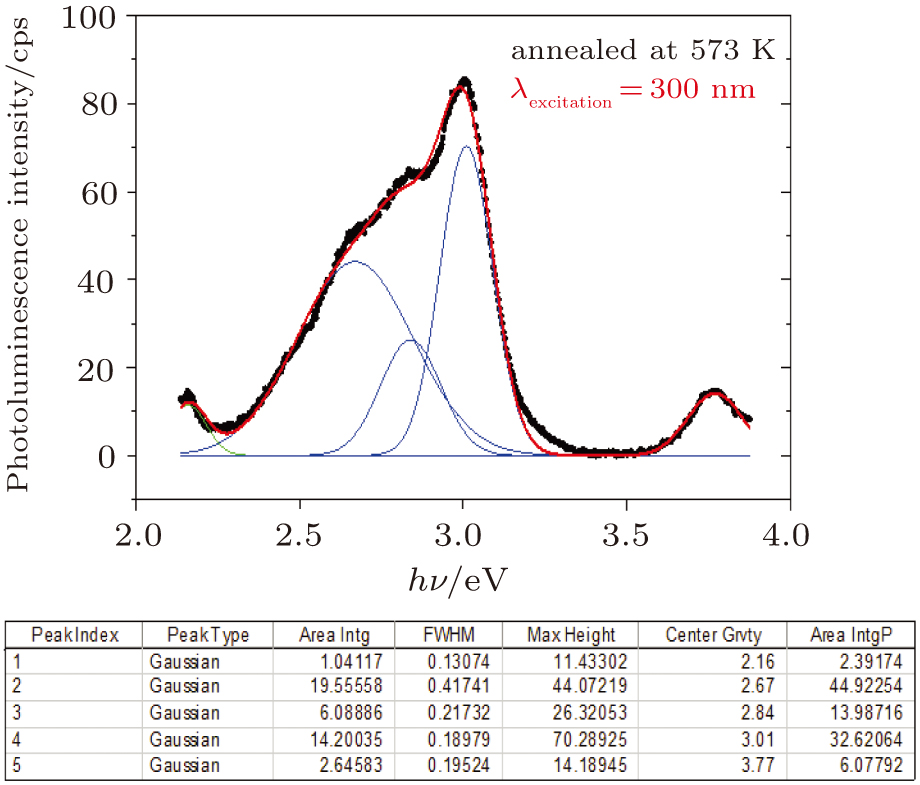
Photoluminescence emission is a key mechanism in the study of light emission properties of optoelectronic semiconductor devices, optical communication and lasers.[43] Photoluminescence emission at room temperature is performed for the as-deposited and thermally annealed CdS/ZnO thin films with λexcitation = 300 nm. The photoluminescence emission spectra are shown in Fig.
 | Fig. 8. Room-temperature PL spectra of as-deposited and thermally annealed CdS/ZnO thin films excited at 300 nm. |
Figure
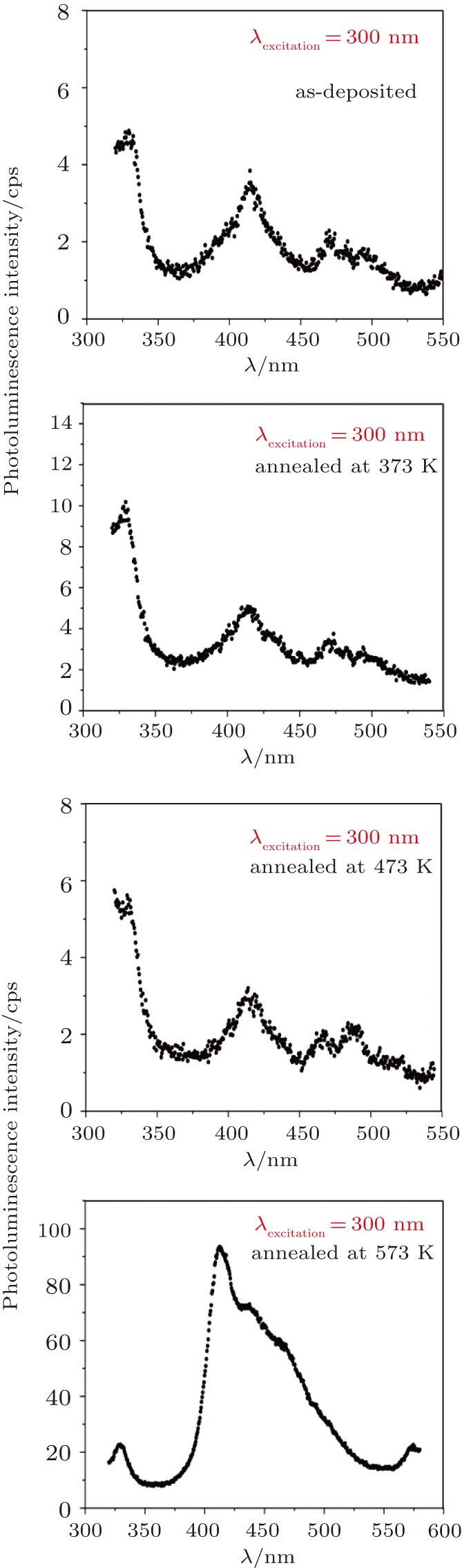
 | Fig. 10. Spectral dependence of mean real part of refractive index, n, of as-deposited and thermally annealed CdS/ZnO thin films. |
At the low optical frequencies, the single oscillator parameters of the as-deposited and thermally annealed CdS/ZnO thin films can be estimated from the following equation[45,46]
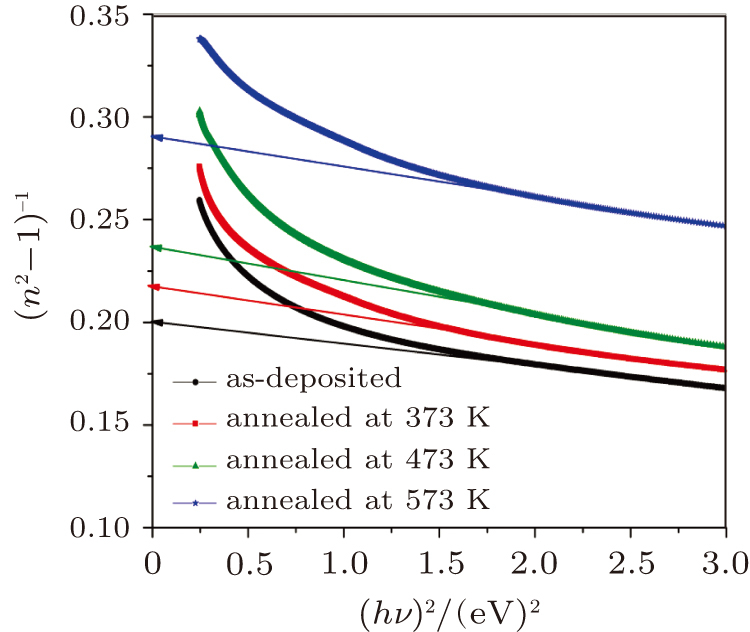 | Fig. 11. Plots of (n2 − 1)−1 versus (hν)2 for as-deposited and thermally annealed of CdS/ZnO thin films. |
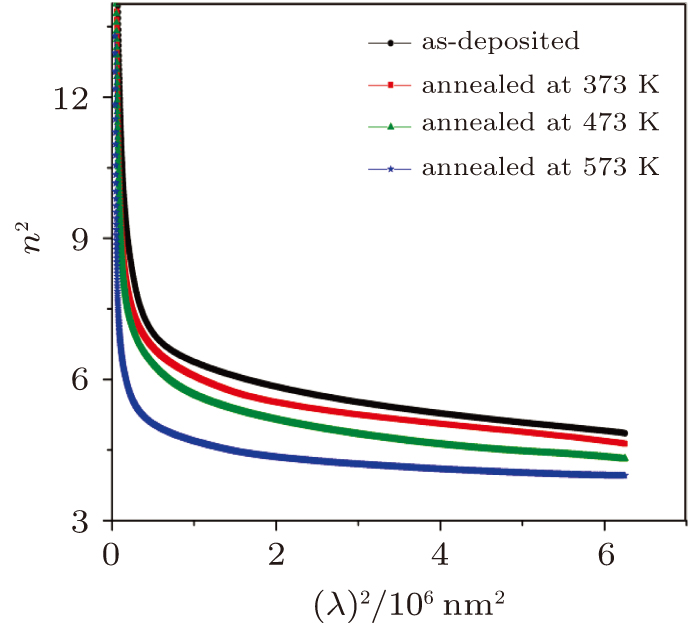
Also, at the low optical frequencies, the relationship between squared refractive index (n2) and squared wavelength (λ2) is given by the following equation[47]
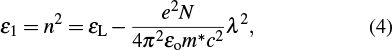
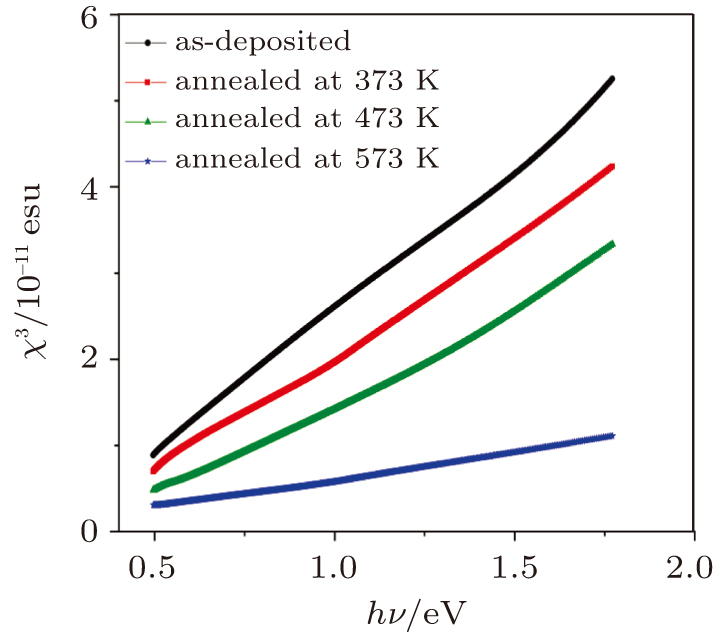
In a system showing a negligible absorption, the third-order nonlinear susceptibility for the as-deposited and thermally annealed CdS/ZnO thin films can be calculated from the following relation[48–51]
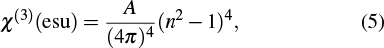
 | Fig. 13. Spectral behaviors of third-order nonlinear susceptibility, χ(3) for as-deposited and thermally annealed of CdS/ZnO thin films. |
Furthermore, the nonlinear refractive index (n2) can be determined from the relation[51]
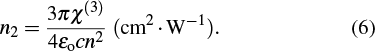
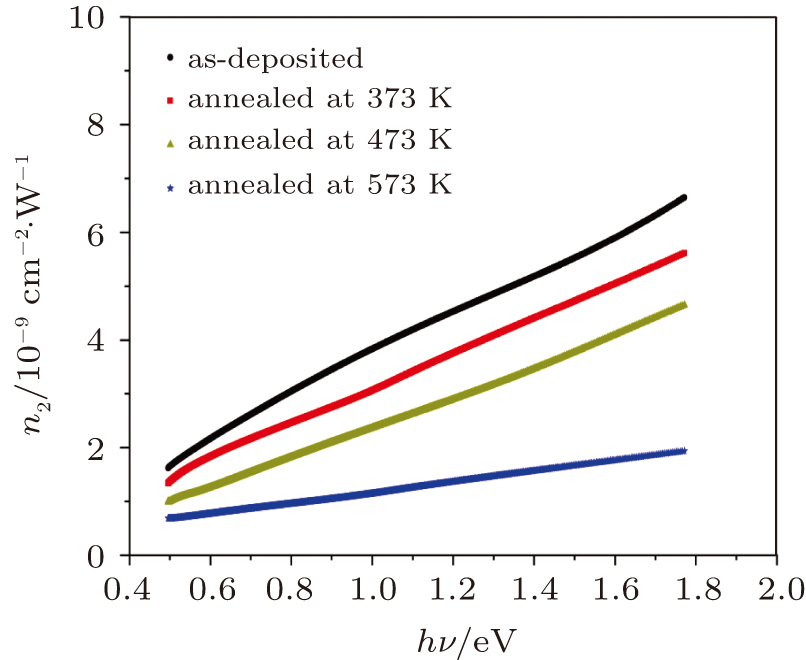 | Fig. 14. Spectral behaviors of nonlinear refractive index n2 for as-deposited and thermally annealed of CdS/ZnO thin films. |
From the above experimental results, it may be concluded that the influences of the annealing temperature on the microstructural (FTIR, XRD, and FESEM), optical and photoluminescence properties of binary nanocomposite materials (CdS/ZnO) thin films can be attributed to physical rather than chemical changes, for this can be explained on the basis of the difference in chemical bonding structure after annealing and the reaction between the atoms of both cadmium and zinc metal. Thus, the nature of chemical bonding among certain constituent atoms should play a significant role in the onset of non-metallic physical properties of quasicrystals bearing transition-metal elements. On the other hand, the self-similar symmetry of the underlying structure gives rise to the presence of an extended chemical bonding network due to a hierarchical nesting of clusters. Also, the dislocation density and the compressive stress induced in the as-deposited CdS/ZnO thin films decrease with annealing temperature increasing.
The influences of annealing temperature on the morphological, structural, and optical characteristics of RF sputtered CdS/ZnO thin films are analyzed. The FTIR spectra are verified by the existence of the band centered at 485 cm−1 assigned to Zn–O zinc oxide bond, two bands at 960 cm−1 and 775 cm−1 assigned to the stretching frequency of Cd–S bond and the band at 1150 cm−1 assigned to the S–O stretching vibration of the CdS–ZnO thin films. The XRD analyses of the as-deposited and thermally annealed CdS/ZnO thin films reveal that the films are all amorphous in nature. The FESEM images reveal that the thin films possess uniform distribution of identical nanoparticles in the range of (120 ± 9) nm. Photoluminescence emission spectra of CdS/ZnO thin films show overall decrease in their peak intensities after annealing. The emission peaks can be attributed to the intrinsic defects such as sulfur vacancies, surface states, and oxygen vacancies. The optical band gaps of CdS/ZnO thin films all show a distinct blue shift with temperature increasing up to 473 K, which can be related to the band bending effect. The slight red shift in the band gap at 573 K can be attributed to the band gap narrowing effect through many-body effects. The microstructural and optical properties of the as-deposited and thermally annealed CdS/ZnO thin films are studied to identify and verify the characteristics of CdS/ZnO thin films for optoelectronic applications. Additionally, the estimated third-order nonlinear susceptibility and nonlinear refractive index are observed to be reduced as the annealing temperature increases.
| [1] | |
| [2] | |
| [3] | |
| [4] | |
| [5] | |
| [6] | |
| [7] | |
| [8] | |
| [9] | |
| [10] | |
| [11] | |
| [12] | |
| [13] | |
| [14] | |
| [15] | |
| [16] | |
| [17] | |
| [18] | |
| [19] | |
| [20] | |
| [21] | |
| [22] | |
| [23] | |
| [24] | |
| [25] | |
| [26] | |
| [27] | |
| [28] | |
| [29] | |
| [30] | |
| [31] | |
| [32] | |
| [33] | |
| [34] | |
| [35] | |
| [36] | |
| [37] | |
| [38] | |
| [39] | |
| [40] | |
| [41] | |
| [42] | |
| [43] | |
| [44] | |
| [45] | |
| [46] | |
| [47] | |
| [48] | |
| [49] | |
| [50] | |
| [51] |