† Corresponding author. E-mail:
Project supported by the Joint Fund of the National Natural Science Foundation of China and the China Academy of Engineering Physics (NSAF) (Grant No. U1530134), the Foundation of National Key Laboratory of Shock Wave and Detonation Physics, China (Grant No. 6142A0306010817), and the Chinese Academy of Sciences (Grant Nos. KJCX2-SW-N20 and KJCX2-SW-N03).
The structural phase transitions of bismuth under rapid compression has been investigated in a dynamic diamond anvil cell using time-resolved synchrotron x-ray diffraction. As the pressure increases, the transformations from phase I, to phase II, to phase III, and then to phase V have been observed under different compression rates at 300 K. Compared with static compression results, no new phase transition sequence appears under rapid compression at compression rate from 0.20 GPa/s to 183.8 GPa/s. However, during the process across the transition from phase III to phase V, the volume fraction of product phase as a function of pressure can be well fitted by a compression-rate-dependent sigmoidal curve. The resulting parameters indicate that the activation energy related to this phase transition, as well as the onset transition pressure, shows a compression-rate-dependent performance. A strong dependence of over-pressurization on compression rate occurs under rapid compression. A formula for over-pressure has been proposed, which can be used to quantify the over-pressurization.
Bismuth (Bi) has been widely studied experimentally and theoretically under high pressure due to its complex phase diagram and its abundant pressure-induced polymorphic phases.[1–20] Previous studies have indicated that bismuth undergoes three pressure-induced structural phase transitions in the pressure range below 10 GPa at room temperature. Under ambient conditions, bismuth is stable in phase I (Bi-I) having a rhombohedral A7-type crystal structure with space group 
Up to now, the pressure-induced structure transformation in bismuth has mainly been studied using static compression in diamond anvil cell (DAC) or shock wave loading. However, the kinetics of a pressure-induced phase transition, e.g., transition pressure and characteristic time, should be related to the pressure loading rate. Recently, the development of dynamic diamond anvil cells (dDAC) with time-resolved synchrotron x-ray diffraction make structural measurement possible under rapid compression with timescales in the order of a millisecond.[22]
In this paper, we present a study of structural phase transitions in bismuth under rapid compression at room temperature using the fast loading DAC technique with time-resolved synchrotron x-ray diffraction, which has been recently developed at the Beijing Synchrotron Radiation Facility (BSRF). The experimental results show that Bi-I, Bi-II, Bi-III, and Bi-V appear one by one as the pressure increases from ambient pressure to ∼ 12 GPa. There is no change in the phase transition path of bismuth under rapid compression at different compression rates (0.20–183.8 GPa/s) compared with the static high pressure experiments. In the phase transition process from Bi-III to Bi-V, the increasing volume fraction of Bi-V versus pressure generally gives a sigmoidal curve which can be well fitted by a compression-rate-dependent function. The fitting results show that, as the compression rate increases, the activation energy associated with this transformation decreases while the onset transition pressure increases. Moreover, a strong compression rate dependence of over-pressurization occurs under rapid compression, and a formula of over-pressure is defined to quantify over-pressurization.
A symmetric diamond anvil cell equipped with a pair of diamond anvils with a culet diameter of 250 μm was used for the rapid compression experiments. A rhenium (Re) gasket was used with the sample chamber diameter of ∼ 100 μm and pre-indented thickness of ∼ 40 μm. Bismuth sample powder with 99.5% purity (Alfa Aesar) was pressed into a thin slice, and then loaded into the sample chamber. Sodium chloride (NaCl, from Alfa Aesar) was added as the pressure medium to obtain quasi-hydrostatic pressure conditions, as well as the internal pressure marker. After sample loading, the DAC was inserted into a dDAC which was driven by a piezoelectric actuator.
Time-resolved synchrotron x-ray diffraction experiments were carried out using the angle-dispersive diffraction method with a monochromatic x-ray beam wavelength of 0.6199 Å at the 4W2 high pressure station at BSRF. The incident x-ray beam was focused in the horizontal and vertical directions to a 25 × 10 μm2 (full width at half maximum (FWHM)) spot on the sample using Kirkpatrick–Baez mirrors.[21] The two-dimensional diffraction pattern was collected using a PILATUS2 2M area detector with an exposure period of 100 ms and an exposure time of 90 ms for each image.
A function generator and a digital oscilloscope were used for synchronization, triggering, and monitoring the timing between the x-ray detector and the dDAC rapid compression device. We used trapezoid waveforms to trigger the piezoelectric actuator to modulate the rapid compressions in the dDAC, and used pulse waveform to trigger the detector to collect the diffraction patterns. By adjusting the timing of the trigger waveforms of the dDAC and detector, the pressure compression and the x-ray diffraction image collection proceeded simultaneously. The Fit2D program[23] was used to integrate the diffraction images to obtain intensity versus 2-theta plots. Then the diffraction data were analyzed to index the diffraction peaks by using the Jade (MDI Jade 6) program. The sample pressure was determined from the NaCl (200) diffraction peak in each diffraction image by using the equation of state (EOS) previously reported.[24] The compression rate of the experiment was estimated by the rising slope of the determined pressure versus time.
In the case of rapid compression, the volume fraction of product phase during the phase transformation process as a function of pressure can be described by Eq. (
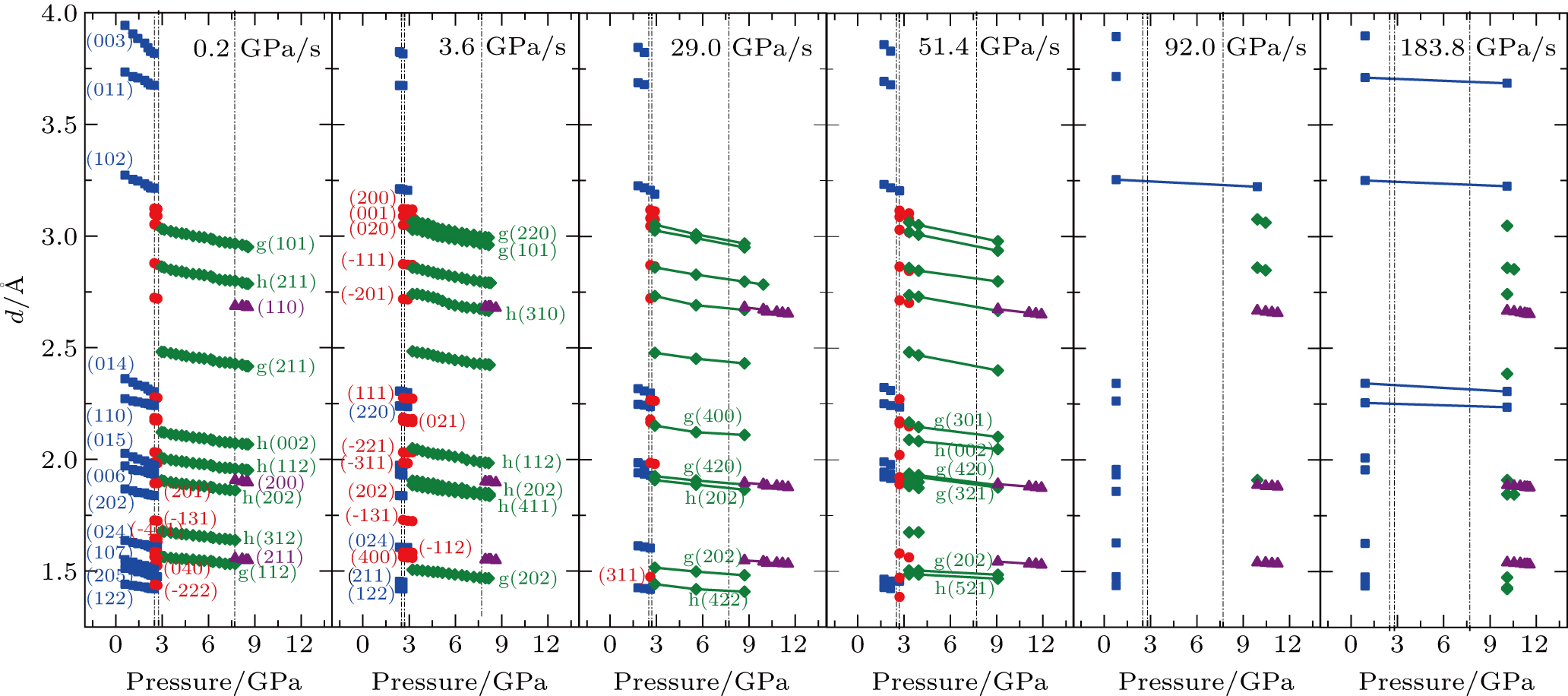
Figure
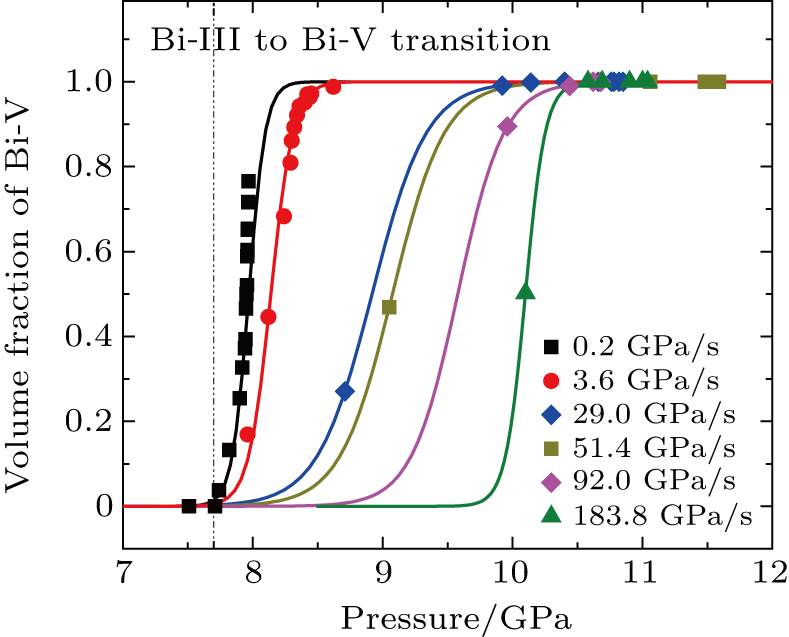
The pressure-induced structural phase transition process is studied by tracking the spectral changes in the intensities of the diffraction peaks of the parent phase and the product phase as the pressure increases. Focusing on the phase transition from Bi-III to Bi-V, we obtain the volume fraction of the Bi-V as a function of pressure at each compression rate, as shown in Fig.
According to Eq. (
The fitting results of the onset phase transition pressure P0 at different compression rates are also plotted in Fig.
The transformation rate equation, well-known as the JMAK rate equation, is often used to describe kinetic processes of phase transitions induced by temperature under heating at given heating rates.[27–29] Similarly, the differentiation of Eq. (
In summary, using a dynamic-DAC and time-resolved synchrotron x-ray diffraction, the structural evolution of bismuth induced by rapid compression has been probed with 100 ms time resolution at room temperature. The structural evolutions of bismuth induced by rapid compressions in the rate range from 0.2 GPa/s to 183.3 GPa/s have been observed. The phase transition sequence is the same as that in static high pressure experiments. In the process from Bi-III to Bi-V, the volume fraction of Bi-V versus pressure can be well fitted by a sigmoidal curve. The fitting results show that the activation energy associated with this transformation depends on the compression rate. A strong dependence of over-pressurization on compression rate has been found under rapid compression. In order to quantify the over-pressurization, a formula for over-pressure has been proposed.
| [1] | |
| [2] | |
| [3] | |
| [4] | |
| [5] | |
| [6] | |
| [7] | |
| [8] | |
| [9] | |
| [10] | |
| [11] | |
| [12] | |
| [13] | |
| [14] | |
| [15] | |
| [16] | |
| [17] | |
| [18] | |
| [19] | |
| [20] | |
| [21] | |
| [22] | |
| [23] | |
| [24] | |
| [25] | |
| [26] | |
| [27] | |
| [28] | |
| [29] |