† Corresponding author. E-mail:
The Zintl compound Mg3Sb2 has been recently identified as promising thermoelectric material owing to its high thermoelectric performance and cost-effective, nontoxicity and environment friendly characteristics. However, the intrinsically p-type Mg3Sb2 shows low figure of merit (zT=0.23 at 723 K) for its poor electrical conductivity. In this study, a series of Mg3−xLixSb2 bulk materials have been prepared by high-energy ball milling and spark plasma sintering (SPS) process. Electrical transport measurements on these materials revealed significant improvement on the power factor with respect to the undoped sample, which can be essentially attributed to the increased carrier concentration, leading to a maximum zT of 0.59 at 723 K with the optimum doping level x = 0.01. Additionally, the engineering zT and energy conversion efficiency are calculated to be 0.235 and 4.89%, respectively. To our best knowledge, those are the highest values of all reported p-type Mg3Sb2-based compounds with single element doping.
To recover the massive global-existing waste heat dissipated from industrial or civilian sources to useful energies, thermoelectric (TE) has been one of the most desirable solutions owing to their capability to convert energy between heat and electricity reversibly and directly.[1–4] The performance of TE materials is primarily governed by its dimensionless figure of merit (zT), which is defined as follows: 

The “phonon–glass, electron–crystal” (PGEC) concept introduced by Slack[11] has been an effective way to partially decouple those electrical and thermal parameters for optimizing thermoelectric properties. According to this concept, ideal TE materials prone to have independent functional modules to modulate electron and phonon transport separately. Through optimization on these modules, materials with electron transport characteristics of crystal and phonon transport characteristics similar to glass can be obtained. Among notable families of TE materials such as lead telluride,[12] skutterudites,[13] half-heuslers,[6] MgAgSb,[14] and so on, Zintl phase compounds are found to meet such criterions. It consists of anions (post-transition elements from group 13–15) and cations (alkali metal or alkaline earth metal elements) which exhibits large electronegativity difference.[15,16] During the formation of ionic bonds, the cations donate electrons to the anions while the latter would further build covalent bonds among themselves to satisfy valence balance, thus leading to formation of complicated lattice structure. The stable covalent-bond lattice contributes to the characteristic of “electron crystal”, which would dominate the electrical conductivity of the material. In addition, anions in the weak ionic bonding area would form locally disordered structure, offering it the characteristics of the “phonon glass”.
Over the past decade, significant progresses have been made on Zintl phase thermoelectric materials, a large number of high performance Zintl phase bulk materials such as Yb14MnSb11,[17] BaGa2Sb2,[18] CaxYb1−xZn2Sb2,[19] Ba24InxGe100−x,[20] and NaGaSn2[21] have been reported. However, they are restricted in the practical applications for their toxic or expensive constituent elements. Recently, a cheap and nontoxic Zintl phase compound, Mg3Sb2, has greatly attracted the attentions. The Bi and Te co-doped n-type Mg3Sb2 bulk material was prepared with good reproducibility using ball milling and SPS method, in which zT value achieved 1.5 at 723 K.[22] Thus indicates the Mg3Sb2-based bulk material might be promising thermoelectric materials with potential application in the middle temperature ranges.[23–27] However, the practical application is severely limited by the poor thermoelectric performance of p-type Mg3Sb2 materials, which is the direct motivation of this study.
For undoped Mg3Sb2, it shows a persistent p-type characteristic since the thermally activated vacancies at Mg-sites with negative charges. However, its low carrier concentration results in a poor electrical conductivity. A variety of approaches have been used to solve this problem, such as doping Pb,[28] Bi[29] at the Sb site, or doping Na,[30] Ag,[31] Mn,[32] Zn[33] at the site of Mg. Among them, Na doping seems to be the most effective way to increase the carrier concentration, but this also increased the lattice thermal conductivity of the material. Other dopants could reduce the thermal conductivity, while the improvement on electrical transport is less satisfactory.
In this work, the alkali metal lithium is used to dope at cationic site in Mg3Sb2 to tune the carrier concentration. Experimental results reveal that the doping operation effectively improved the electrical transport performance of the Li-doped samples. Meanwhile, a slightly decrease of the lattice thermal conductivity is observed, which should be attributed to the increased point defects scattering on phonon. As a result, improved TE properties have been achieved in a broad range of temperatures. The peak zT of Mg2.99Li0.01Sb2 bulk material reached 0.59 at 723 K, increased by 158% compared with the undoped sample. Moreover, the calculated engineering zT and efficiency are 0.235 and 4.89%, respectively, indicating that Li-doped Mg3Sb2 compound could be a good p-type thermoelectric material.
The Mg3−xLixSb2 (x = 0, 0.005, 0.01, 0.02) bulk samples were prepared by ball milling and spark plasma sintering (SPS). In a typical method, magnesium (Mg, Alfa Aesar, 99.8%, turnings), lithium (Li, Sigma Aldrich, 99.9%, ribbon), and antimony (Sb, Alfa Aesar, 99.999%, pieces) were weighed according to the stoichiometry in an Ar-filled glove box. Then the raw materials were loaded into a stainless steel jar and ball milled for 10 h by using a SPEX Sample Prep 8000 Mixer/Mill. Finally, the obtained powder was put into a graphite die (diameter, 12.7 mm) and treated by SPS process at 1023 K for 10 min under a pressure of 50 MPa. The density of those as-pressed samples was measured by using Archimedes method to be in the range of 96% to 98% of the theoretical density.
The x-ray diffraction (XRD) patterns of as-prepared samples were collected by a diffractometer (PANalytical X’Pert Pro) operated at 40 kV and 40 mA with Cu Kα radiation (λ =1.542 Å). The measurement was carried out in the 2θ range of 20°–80° in air. The microstructures of samples were characterized by a scanning electron microscopy (SEM, XL30S-FFG) on the samples’ cross sections. More details were obtained on a transmission electron microscopy (TEM, JEM-2100 Plus).
The temperature dependence of electrical resistivity and Seebeck coefficient were measured on a commercial system (LSR-3, Linseis) in the atmosphere of high purity helium. The temperature dependence of total thermal conductivity was defined as 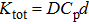
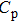

As shown in Fig. 
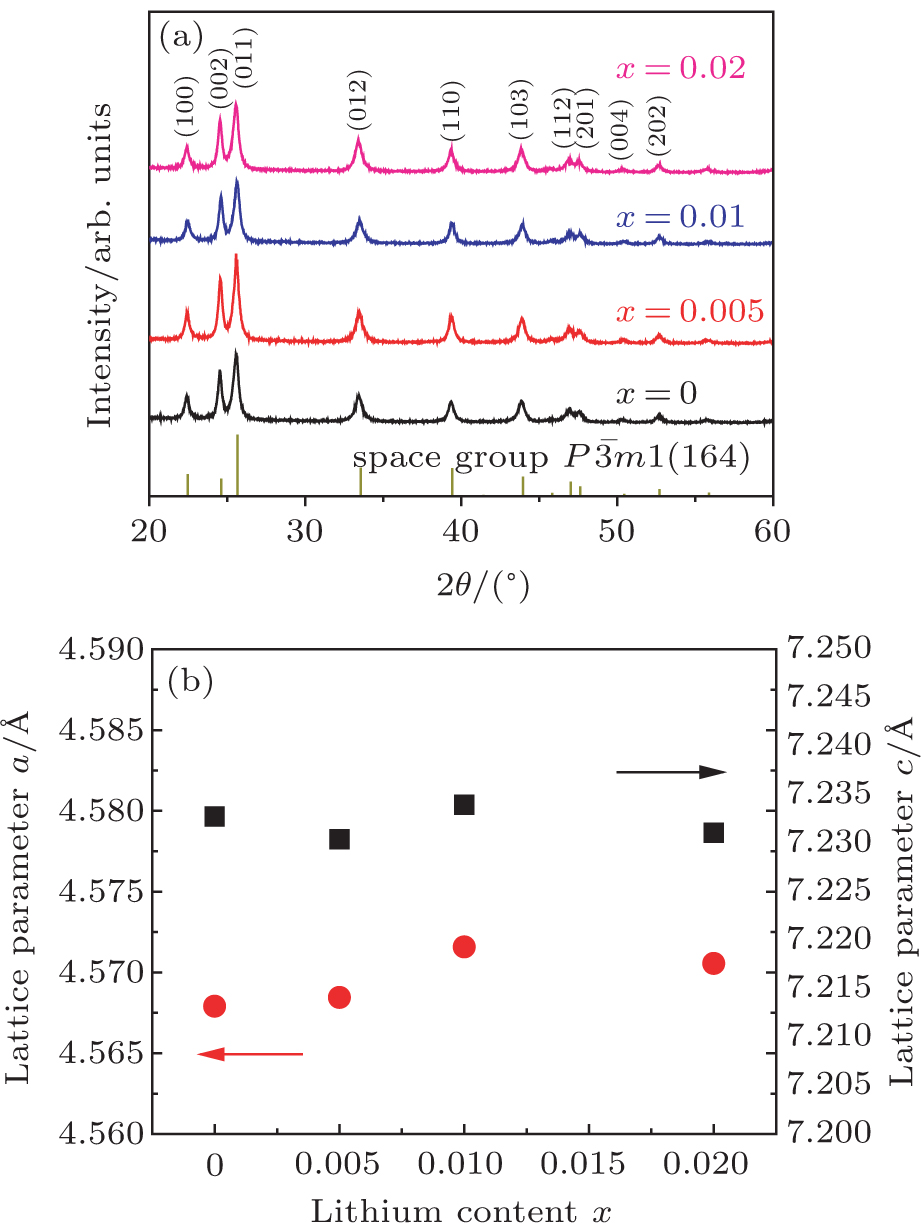 | Fig. 1. (color online) (a) XRD patterns and (b) lithium doping dependence of lattice parameters for series of Li-doped Mg3−xLixSb2 (x = 0, 0.005, 0.01, 0.02) compounds. |
Figure
The temperature dependence of electrical resistivity and Seebeck coefficient are shown in Fig. 




In the approximation of energy independent scattering, the Seebeck coefficient can be described using a single parabolic band (SPB) model:




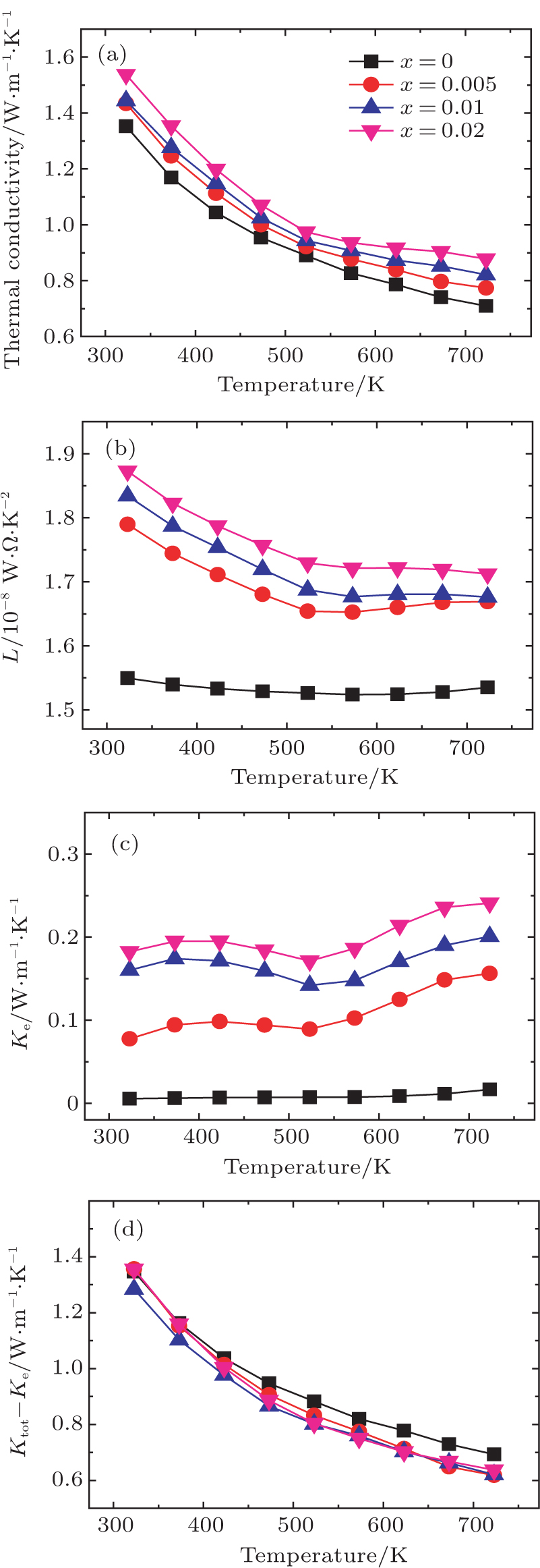
Figure 


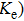






The obtained L and carrier thermal conductivity 
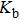



The calculated Figure of merit (zT) is shown in Fig. 
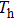


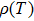










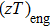
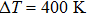
 | Fig. 6. (color online) Calculated thermoelectric performance of Mg3−xLixSb2 (x = 0, 0.005, 0.01, 0.02) compounds: (a) zT; (b) engineering zT; and (c) energy conversion efficiency. |
To further evaluate the impact of different dopants (such as Li, Na, Ag, Pb, Bi) on the TE performance of Mg3Sb2, figure 



In summary, a series of Li-doped p-type Mg3−xLixSb2 (x = 0, 0.005, 0.01, 0.02) bulk material has been prepared by combination of ball milling and SPS process. The thermoelectric performance was greatly improved in comparison to that of undoped Mg3Sb2 sample. For the optimized Mg2.99Li0.01Sb2 sample, the peak zT and maximum 
| [1] | |
| [2] | |
| [3] | |
| [4] | |
| [5] | |
| [6] | |
| [7] | |
| [8] | |
| [9] | |
| [10] | |
| [11] | |
| [12] | |
| [13] | |
| [14] | |
| [15] | |
| [16] | |
| [17] | |
| [18] | |
| [19] | |
| [20] | |
| [21] | |
| [22] | |
| [23] | |
| [24] | |
| [25] | |
| [26] | |
| [27] | |
| [28] | |
| [29] | |
| [30] | |
| [31] | |
| [32] | |
| [33] | |
| [34] | |
| [35] | |
| [36] | |
| [37] | |
| [38] |