† Corresponding author. E-mail:
Project supported by the National Basic Research Program of China (Grant No. 2014CB643900), the Open Fund of IPOC (BUPT), the Open Program of State Key Laboratory of Functional Materials for Informatics, the National Natural Science Foundation for Theoretical Physics Special Fund “Cooperation Program” (Grant No. 11547039), and Shaanxi Provincial Institute of Scientific Research Plan Projects, China (Grant No. SLGKYQD2-05).
First-principles method is used to simulate the stable structure and optical properties of a 96-atom fused silica. The preferable structure of NBOHC-E′ (non-bridging oxygen hole center (NBOHC) and E′ center) pair defect is predicted to be located at 2.4 Å for the Si–O bond length. The quasi-particle G0W0 calculations are performed and an accurate band gap is obtained in order to calculate the optical absorption properties. With the stretching of the Si1–O1 bond, an obvious redshift can be observed in the absorption spectrum. In the case of NBOHC-E′ pair, the p-orbital DOS of Si1 atom will shift to the conduction band. Two obvious absorption peaks can be observed in the absorption spectrum. The calculation reproduced the peak positions of the well-known optical absorption bands.
Properties of fused silica have attracted attention in the past decade due to its potential technological applications, including microelectronics and optical transmission media. Basically, it is crucial to stabilize specific defects by controlling the thermochemical process if we are to better understand their structural and electronic properties. Whether they are generated by manufacturing, irradiation, or mechanical deformation, the defects capable of trapping charge in silica are responsible for degrading device performance, leading to threshold voltage shifts in metal-oxide-semiconductor transistors, actuation voltage changes in mechanical systems, and even attenuation in high power laser devices.[1,2] Two main paramagnetic defects, i.e., the non-bridging oxygen hole center (NBOHC) and E′ center where E′ refers to a three coordinate Si atom,[3] can be observed at room temperature in irradiated fused silica, which have been considered to dominate the light absorptions. As is well known, the NBOHC is created by breaking the Si–O bond and forming the NBOHC-E′ pair. Therefore, the NBOHC represents the oxygen dangling bond or non-bridging oxygen hole center with a structure of ≡Si–O·, where (≡) refers to bonds with three oxygen atoms and (·) denotes an unpaired electron.[4,5]
Extensive experimental and theoretical studies[6–16] have been devoted to point defects in silica due to their relevance in the optical transmission properties of SiO2-based electronic devices. Bakos et al.[7] reviewed the experiments that are used to support the identification of the NBOHC defect responsible for the optical absorption (OA) at 4.8 eV and photoluminescence (PL) bands at 1.9 eV. In Ref. [8], three OA peaks of NBOHC in SiO2 glass induced by low-temperature F2 laser-irradiation are evident: the most intense peak at 4.8 eV, a medium intensity peak at ∼ 6.8 eV, and a low-intensity peak at ∼ 2 eV. Benoit et al.[9] and Cannas et al.[10] presented the OA bands extended from visible (2.0 eV), to ultraviolet (UV) (4.8 eV) and deep ultraviolet (DUV) (6.8 eV) regions. In the theoretical aspect, Raghavachari et al.[11] studied the OA of a series of point defects in SiO2 cluster models by the time-dependent density functional response theory (TD-DFT). Suzuki et al.[12] calculated the electronic structures and the natures of optical transitions in oxygen dangling bond in silica glass. Saeta and Greene[13] discussed the kinetics of photoinduced NBOHC-E′ defect formation in high-purity silicas by femtosecond transient absorption spectroscopy in the visible and UV regions. For the typical feature of NBOHC-E′ pair defect, Giordano et al.[17] reported that the terminal Si–O bond in an NBO· defect of hydroxylated silica surface is close to 1.69 Å. Although several studies have focused on optical transitions mode and the OA properties, the prediction of the preferable structure with representative defects in fused silica remains a significant computational challenge.
In this paper, a series of ab initio calculations are carried out to reveal the preferable structure, electronic and optical properties of an NBOHC-E′ pair in fused silica. A typical model with the NBOHC-E′ pair is generated by using molecular dynamics (MD) simulation. The structural optimization is realized by using the generalized gradient approximation (GGA) with the framework of first-principles DFT.[18–20] The electronic and optical properties are investigated by using the Bethe–Salpeter equation (BSE) based on the quasi-particle G0W0 method[21] in order to improve the accuracy of the calculation. The rest of this paper is organized as follows. The computational methods and models are described in Section 2. Our results and discussion are presented in Section 3. Finally, some conclusions are drawn from the present study in Section 4.
A 2×2×2 supercell containing 96 atoms is built and optimized with a force tolerance of 0.1 eV/Å. The size of supercell (∼ 10 Å) is large enough to neglect the interaction with its periodic images. A Large-scale Atomic/Molecular Massively Parallel Simulator (LAMMPS) based on the classical MD method[22] is used to construct the fused silica model, where a three-stage heating-cooling procedure is performed. At first, the 96-atom supercell system is heated for 50 ps under periodic boundary conditions (PBCs) with an initial temperature of 5000 K to achieve the condition of equilibrium; then, it is quenched to 500 K by using 9 chained quenching-equilibrating steps; finally, the system is cooled to 300 K and equilibrated for 50 ps. Subsequently, equilibration MD simulations are performed in time steps of 0.5 fs under the isothermal-isobaric (NPT) ensemble. The external pressure is set to be the air pressure under ambient conditions. The system temperature is controlled by using the Langevin thermostat. The simulated density for the supercell is found to be 2.2 g/cm3, which agrees well with the experimental result (2.2 g/cm3).[23] Figure
First-principles simulations are performed in the framework of DFT implemented in Vienna ab initio simulation package (VASP).[24,25] The GGA exchange-correlation functional in the Perdew–Burke–Ernzerhof (PBE) form[26] is used to describe the electron correlation. The electron wave function is expanded in plane waves with a cutoff energy of 450 eV, which is tested to give converged results. The Brillouin-zone is sampled in the k space within the Monkhorst–Pack scheme by 2×2×2 mesh points and a small Gaussian broadening σ = 0.1 eV is used. Geometry minimizations are used in the single-point calculations to validate the geometry of the NBOHC-E′ pair. The optimization will be completed when the following conditions are satisfied (the maximum force on each atom achieves 0.01 eV/Å, and the maximum energy change between two ionic steps converges at 10− 5 eV). The electronic structure and optical properties of the NBOHC-E′ pair are obtained by solving the BSE based on the quasi-particle G0W0 calculations.
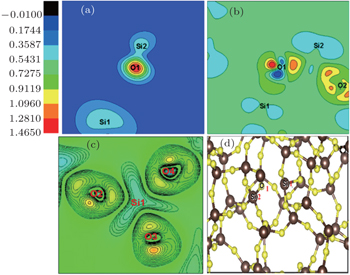
A typical NBOHC-E′ pair defect is generally created by breaking the Si–O–Si bond, which is caused by a bound electron-pair. The O1 is “knocked” out of the Si1–O1 network, and then trapped by the nearby atoms and forms a new ring. Therefore, a key problem is to predict the preferable Si1–O1 distance in order to represent the actual NBOHC-E′ defect. In Fig.
Figure
To verify the feasibility of our prediction for the preferable NBOHC-E′ structure, two more models are constructed and the calculated preferable structures are shown in Fig.
GGA-PBE functional in DFT is used to calculate the band structure of the fused silica model in Fig.
 | Fig. 4. Total DOSs of perfect supercell (red dashed line) and fused silica with NBOHC-E′ defect (black solid line). |
To further depict the contribution of band structure, the partial densities of states (PDOSs) are introduced to explain the origins of these impurity states. The corresponding PDOSs of the Si atoms and the adjacent O atoms are plotted in Fig.
In order to understand the forming mechanism of the defect states, the partial charge density for the defect states are shown in Fig.
Based on the G0W0 calculations, optical properties of both defect and perfect structures are predicted by solving the BSE in the Tamm–Dankoff approximation. The OA coefficient is directly related to the imaginary part of the dielectric function.
Figure
In this work, the classical MD method is used to obtain a stable structure and optical properties for the 96-atom supercell of fused silica. The formation of the NBOHC-E′ center is found by stretching the Si1–O1 bond based on first-principles methods and the preferable NBOHC-E′ defect is found to have a Si1–O1 bond length of about 2.4 Å. The electronic and optical properties are studied by using the G0W0/BSE method. Compared with the perfect model, two obvious OA peaks of the NBOHC-E′ pair are found and show a redshift with the increase of Si1–O1 distance. Our results are of significance for comprehensively analyzing the inherent mechanism of the NBOHC-E′ defect which could be induced by high-power laser. This work can provide technique support for improving laser induced damage performance of fused silica, which is of significance for enhancing the working life of a laser transmission device.
| 1 | |
| 2 | |
| 3 | |
| 4 | |
| 5 | |
| 6 | |
| 7 | |
| 8 | |
| 9 | |
| 10 | |
| 11 | |
| 12 | |
| 13 | |
| 14 | |
| 15 | |
| 16 | |
| 17 | |
| 18 | |
| 19 | |
| 20 | |
| 21 | |
| 22 | |
| 23 | |
| 24 | |
| 25 | |
| 26 | |
| 27 | |
| 28 | |
| 29 | |
| 30 | |
| 31 | |
| 32 | |
| 33 | |
| 34 |