† Corresponding author. E-mail:
Project supported by the National Natural Science Foundation of China (Grant No. 11074050).
We theoretically and experimentally study the optimal duty cycle and pumping rate for square-wave amplitude-modulated Bell–Bloom magnetometers. The theoretical and the experimental results are in good agreement for duty cycles and corresponding pumping rates ranging over 2 orders of magnitude. Our study gives the maximum field response as a function of duty cycle and pumping rate. Especially, for a fixed duty cycle, the maximum field response is obtained when the time averaged pumping rate, which is the product of pumping rate and duty cycle, is equal to the transverse relaxation rate in the dark. By using a combination of small duty cycle and large pumping rate, one can increase the maximum field response by up to a factor of 2 or π/2, relative to that of the sinusoidal modulation or the 50% duty cycle square-wave modulation respectively. We further show that the same pumping condition is also practically optimal for the sensitivity due to the fact that the signal at resonance is insensitive to the fluctuations of pumping rate and duty cycle.
The principle of Bell–Bloom magnetometer (BBM) is based on the discovery of Bell and Bloom in 1961, that the precession of atomic ground state polarization at Larmor frequency can be induced by synchronous amplitude-modulation (AM) of the optical pumping light with either circular[1] or linear[2] polarization. This technology was soon used in magnetometry and various versions of the BBM have been developed,[3–7] including frequency-[8,9] and polarization-modulation.[10–12]
The precession of the atomic polarization can be measured by transmission of circular polarized light as well as nonlinear magneto-optical rotation (paramagnetic Faraday rotation) of linearly polarized light.[13] Very high signal-to-noise ratio can be achieved by phase-sensitive detection referenced to the 1st or higher harmonics of the modulation frequency. It is often practical and convenient to pump and probe with a single beam. However, two-beam scheme has the advantage of allowing the separate study and optimization of the pumping and probing processes.[14] Pustelny et al. studied AM BBM using a single linearly polarized beam for different light intensities, duty cycles and modulation wave forms.[15] Schultze et al. compared the characteristics and performance of the M–x magnetometer with those of the AM BBM for different duty cycles and modulation depths.[16] Grujic and Weis modeled and partially verified the magnetic resonance signals occurring in amplitude-, frequency-, and polarization-modulated light for the single-beam scheme.[17] In those studies the pumping rate was much smaller than the transverse relaxation rate in the dark Γ2 and power broadening was neglected. Compared with sinusoidal AM whose pumping-rate effect was well studied,[18] square-wave AM has an extra control parameter, the duty cycle, allowing further tweaking the performance of the magnetometer. In this article we theoretically and experimentally analyze the effects of the pumping rate and the modulation duty cycle on the field response and the noise of the system. While most previous studies used pumping rates smaller than or comparable to Γ2 and duty cycles on the order of several tens of percent, our study covers a range of duty cycles and corresponding pumping rates over 2 orders of magnitude. We find that, using a small duty cycle while keeping the time averaged pumping rate close to Γ2, we can increase the field response of magnetometer at resonance by up to a factor of π/2 comparing to the common case of 50% duty cycle. The sensitivity is also optimized in the same condition since the fluctuation of pumping parameters has virtually no effect on the field response at resonance.
We assume that a vapor cell containing alkali atoms with gyromagnetic ratio γ is placed in a static magnetic field B0 along the z direction corresponding to a Larmor frequency ω0 = γB0. The atoms are optically pumped by a circularly polarized beam in the x direction whose intensity is modulated by a square wave with 100% modulation depth. The atomic spin polarization is detected by a linearly polarized probe beam along the y axis using paramagnetic Faraday rotation (PFR) method, the signal of which is due primarily to the magnetic dipole moment (MDM) of the atomic ensemble along the beam direction.[19,20] We also assume that ω0 is much smaller than the linewidths of the pump and the probe light. If we define
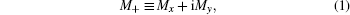
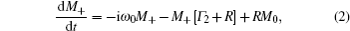
The square-wave amplitude-modulation of the pump beam is assumed to be symmetrical about the time zero without loss of generality, so that the Fourier series expansion of R(t) contains only cosine terms as shown below.
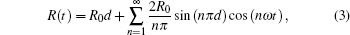
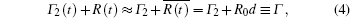
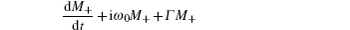
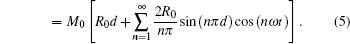
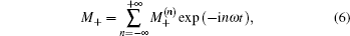
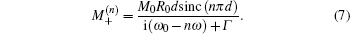
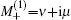
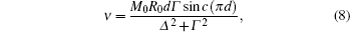
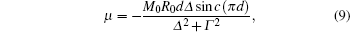
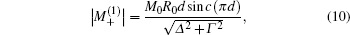
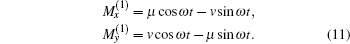
The precession of the transverse MDM is detected by paramagnetic Faraday rotation. When a linearly polarized nearresonant probe beam propagates through an atomic vapor with a finite MDM along the beam direction, its polarization plane is rotated due to the circular birefringence of the vapor.[19] If the detuning of the probe from the optical transition resonance is much larger than the linewidth of the transition, in the case of y-propagating probe, the rotation angle is proportional to My, and quadrature component of its 1st harmonics ϕ is proportional to μ,[22] i.e.,
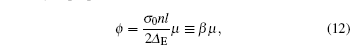
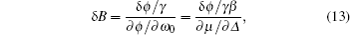
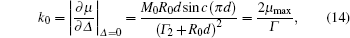
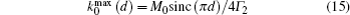
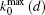
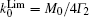
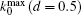
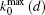
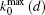
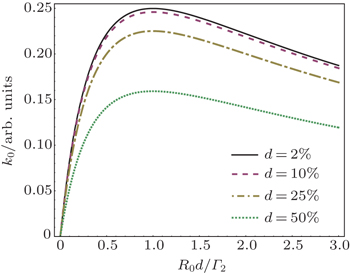 | Fig. 1. Dependences of the resonant response k0 on average pumping rate R0d for various duty cycles. The solid, dash, dash-dot, and dot lines are for d = 2%, 10%, 25%, and 50%, respectively. |
For a fixed R0, the maximum value of k0 achievable by varying d, which we denote by 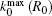
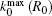
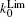
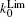
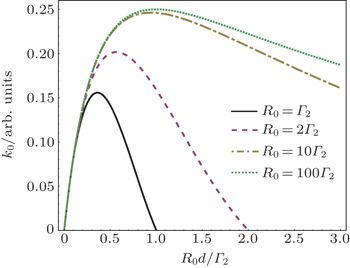 | Fig. 2. Dependences of the resonant response k0 on average pumping rate R0d for various pumping rates. The solid, dash, dash-dot and dot lines are for R0 = Γ2, 2Γ2, 10Γ2, and 100Γ2, respectively. |
It is interesting to compare the result of square-wave AM with that of sinusoidal AM where R(t) = R0+R0 cos (ωt). One can find that for sinusoidal AM, the maximum field response is obtained at R0 = Γ2 and is equal to M0/8Γ2, which is only half of the 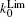
After having studied the effect of pumping on the field response, we turn to its effect on the noise. The noise δϕ is generally contributed by the photon shot noise of the probe beam, the spin projection noise of the atoms, light shift back-action noise and other technical noises.[19] Here we only consider the possible noise related to the pumping parameters. Since β is independent of the pumping parameters, we have
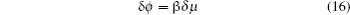
To study the noise of μ due to fluctuation of pumping rate, we differentiate Eq. (
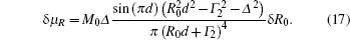
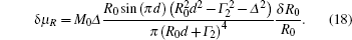
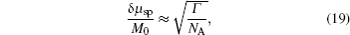
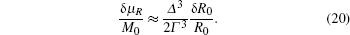
We will show that under our experimental conditions the above noise is already smaller than the spin projection noise δμsp.
Similarly, we can derive the noise of μ due to fluctuation of the duty cycle. Keep in mind that, in practice, δd is usually independent of d. Assuming Δ ≪ Γ, we have
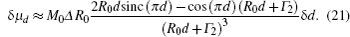
One can see that δμd is also insensitive to the fluctuation of d at resonance. In the case of Δ ≠ 0, δμd is also proportional to 1/R0 for large R0. However, when R0d = Γ2 = Γ/2 and d ≪ 1, we have
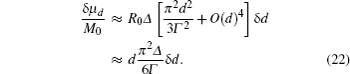
We see that δμd can be tuned down by d until it becomes negligible compared with other noises.
We conclude that because the dispersive signal μ is insensitive to the fluctuation of pumping parameters near resonance, pumping induced noises are usually smaller than other fundamental noises in practice. Thus the optimal pumping condition for the sensitivity of AM BBM is R0d = Γ2 and d → 0, where the resonant field response |∂μ/∂Δ|Δ = 0 is maximized.
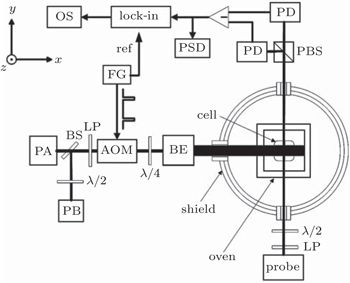
To verify our theory we conducted the following experiment. The schematic design of the experimental setup is shown in Fig.
Figure
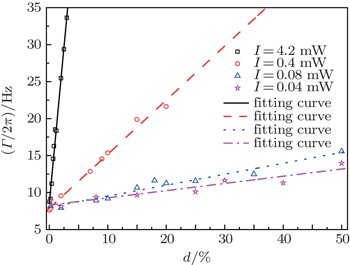
The dependences of the linewidth Γ on duty cycle for various pumping rates are measured and plotted in Fig.
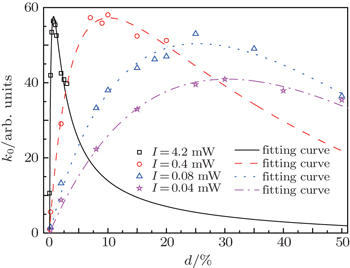
Figure 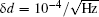
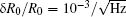
The fitted pumping rate and the optimal duty cycle obtained from the fittings are listed in Table
| Table 1. Fitting parameters of the experimental data. IP is the total pump intensity. RΓ and Γ2 are respectively the R0 and the Γ2 obtained by fitting the data in Fig. |
Large average pumping rate also causes a deviation of the experimental signal line shape from the pure dispersive Lorentzian profile given by Eq. (
We theoretically study the dependences of the line shape of a square-wave amplitude-modulated Bell–Bloom magnetometer on the intensity and duty cycle of the pump light. We show that the maximum field response of the magnetometer can be achieved at the resonance center of the dispersive parametric-Faraday-rotation signal together on the condition that the duty cycle is small and the average pumping rate is approximately equal to the transverse relaxation rate in the dark Γ2. The theoretical results are in agreement with experimental results for pumping rates ranging over two orders of magnitude above Γ2. We also show that the signal at resonance is insensitive to the fluctuations of pumping rate and duty cycle. Thus it is the same condition for both the maximum field response and the optimal sensitivity. The line shape of experimental signal starts to deviate from the prediction of the theory when the average pumping rate exceeds Γ2. A full density matrix calculation, which includes the hyperfine pumping and the dynamics of higher rank polarization moments is expected to give a more accurate description of this phenomenon.
| 1 | |
| 2 | |
| 3 | |
| 4 | |
| 5 | |
| 6 | |
| 7 | |
| 8 | |
| 9 | |
| 10 | |
| 11 | |
| 12 | |
| 13 | |
| 14 | |
| 15 | |
| 16 | |
| 17 | |
| 18 | |
| 19 | |
| 20 | |
| 21 | |
| 22 | |
| 23 | |
| 24 | |
| 25 | |
| 26 | |
| 27 |