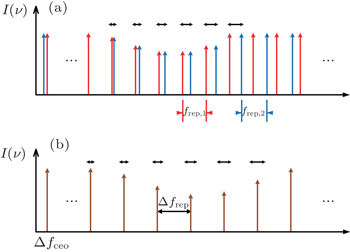
An optical frequency comb,^[1] providing hundreds of thousands of even spaced and mutual coherent longitudinal modes which are traced to frequency standard, pushes laser metrology forward by a tremendous step including molecular spectroscopy.^[2] Based on multi-heterodyne detection that is similar to conventional Fourier transform spectrometry,^[3] a dual-comb spectrometer employs two comb lasers with slightly different repetition rates to mimic scanning sampling. In this scenario, detuned pulse trains act as asynchronous optical sampling probes for each other.^[4,5] Thus, a dual-comb system can also be applied to monitor instantaneous phenomenon^[6] or remote, fast sensing.^[7] Because of the unique measurement principle, it has many advantages, such as high-speed acquisition, high resolution, and high-frequency accuracy.

To a remarkable extent, the three ‘High’ advantages are confined by comb laser performance. In one comb, there are two freedoms, i.e., repetition rate (f_rep) and carrier-envelope offset frequency (f_ceo). The stabilities of f_rep and f_ceo directly affect the performance of the measurement system. In order to record non-distorted interferograms by a dual-comb spectrometer, one method is to use highly stabilized laser combs via phase-locking both freedoms to optical frequency standards.^[8,9] Owing to the precise control of laser combs, the radio frequency (RF) beats generated by pairs of nearby comb modes are fixed. Alternatively, a raw distorted interferogram of free-running laser combs can be corrected by mixing adaptive signals which contain phase error information on real-time compensation hardware^[10] or by a posteriori correction algorithm.^[11,12] No frequency standards in these systems cause a little trouble in mapping spectral features along the horizontal axis. However, these studies show the solution of laser fluctuation, but they do not include how the spectrum degrades since laser performance deteriorates.

In this paper, the frequency stabilities of f_rep and f_ceo are evaluated. According to the principle, the repetition rate fluctuation is the main reason that causes spectral frequency misalignment compared with carrier-envelope offset frequency variation. By establishing a dual-comb system traced to a Rubidium clock, we measure the P branch in the 2v₃ rotational–vibrational band of hydrogen cyanide (H¹³C¹⁴N). The measured spectra show absorption peak jitter that is in agreement with unstable pulse interference simulation. The signal-to-noise ratio of the absorption spectrum is effectively improved by averaging modified spectra in the frequency domain. Finally, discussion and further improvement are presented.

A well-stabilized mode-locked laser provides a train of ultrafast pulses at a constant repetition rate and with equal carrier-envelope phase shift in the time domain. In the optical domain, the comb structure of laser modes extends hundreds of nanometers in the optical domain. The output field can be described as

Fig. 1.

	Figure Option ViewDownloadNew Window
	Fig. 1. Principle of dual-comb spectrometry. A two-frequency comb generates an RF beat comb according to multi-heterodyne detection. The double-sided arrow denotes laser mode fluctuation. As n goes up, the fluctuation becomes more and more considerable. (a) Optical domain, (b) RF domain.

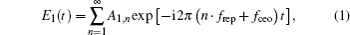
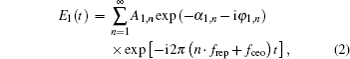
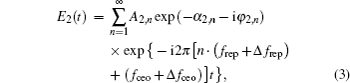
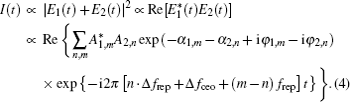
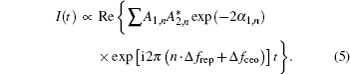
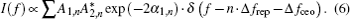
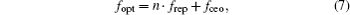
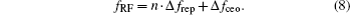
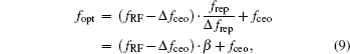
Equation (9) demonstrates that the absorption peak fluctuation, including the zooming and shifting effect originating from f_rep and f_ceo variation, would happen if laser modes could not keep fixed during measurement. Between these two unstable parameters, the former is more critical than the latter, because a small offset in Δf_rep makes a large change in β, further resulting in spectral frequency misalignment. What is more, since the stabilization strategy of f_rep directly locks to the RF frequency standard, the phase noise of the comb mode in the optical region is n² times larger than that of f_rep. Considerable phase noise of the mode in the optical region reduces the signal-to-noise ratio of the interferometric signal. Given this, some methods mentioned in the previous section come into use.

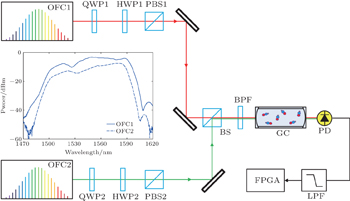
The schematic of the experimental setup is illustrated in Fig. 2. Two Er-doped fiber optical frequency combs (M-Comb, Menlo Systems) locked at f_rep ∼ 250 MHz provide pulse trains with an average power of ∼ 20 mW and a duration of ∼ 80 fs. The 20-dB output bandwidths stretch for 100 nm around 1.55 μm just as indicted in the inset in Fig. 2. The carrier-envelope offset frequencies are self-referenced with f–2f interferometers.^[13] All phase lock loops are referred to as an Rb clock (Symmetricom, 8040C). With the help of quarter wave plates, half wave plates and polarized beam splitters, the polarization and power of each beam are fine-adjusted to improve modulation depth and avoid detector saturation. A region of interest, i.e., the 2v₃-P rotational–vibrational band of H¹³C¹⁴N, is selected by an optical band-pass filter (Thorlabs, FB1550-12). The combined beam, outgoing from a 200 Torr (1 Torr = 1.33322×10² Pa), 7cm-long H¹³C¹⁴N gas cell, is aligned to an InGaAs photodetector with a bandwidth of 125 MHz (New Focus, 1811-FS). A high-speed data acquisition system based on a field programmable gate array (National Instruments) is synchronized to one of the laser combs.

Fig. 2.

Figure Option ViewDownloadNew Window

Fig. 2. Dual-comb spectrometer. The inset shows the output spectra of the frequency combs. Colorful lines represent optical beams, while black lines are electric cables. OFC: optical frequency comb; QWP: quarter waveplate; HWP: half waveplate; PBS: polarized beam splitter; BS: non-polarized beam splitter; BPF: optical band-pass filter; GC: gas cell; PD: photodetector; LPF: electronic low-pass filter.

Fig. 3.

	Figure Option ViewDownloadNew Window
	Fig. 3. Stabilities of locked fceo and frep. (a) Locked fceo with a gate time of 1 s. (b) The Allan deviation of locked fceo as a function of average time. (c) The frequency noise of locked frep derived from the power spectral density of phase noise.

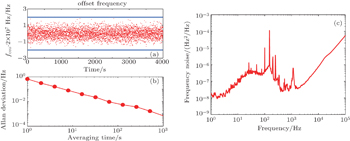
The stabilities of f_ceo and f_rep are evaluated first. Achieved by a sequence of amplification, nonlinear spectrum boardening and second harmonic generation, the linewidth of f_ceo is on the order of several hundred kHz. It is better to evaluate this parameter via Allan deviation. We use a Π-type frequency counter with no dead time at a gate time of 1 s to record locked f_ceo for 4000 s. The result is shown in Fig. 3(a). A glitch exceeding the threshold is kicked out when calculating the Allan deviation. From Fig. 3(b), 1-s average time Allan deviation of locked f_ceo is ∼ 0.66 Hz. The Allan deviation comes down as the average time increases. The power spectral density of the phase noise is suitable to locked f_rep. Figure 3(c) shows the frequency noise of locked f_rep with Fourier frequency from 1 Hz to 100 kHz. The integrated RMS residual frequency modulation d f_rep is 1.4 Hz.

To obtain the interferometric signal, laser combs are locked at different repetition rates, f_rep,1 = 250.000 MHz and f_rep,2 = 250.002 MHz. Thus, the difference Δf_rep is 2 kHz, and interferograms are repeatedly generated with a time interval of 0.5 ms. 25 interferograms are recorded within 12.5 ms. Figure 4(a) shows one of the interferograms. The dynamic range is optimized to 825. Free-induction decay (FID)^[6] can be seen on both sides of the centerburst as both laser beams interact with the gas sample. According to the above parameters, we simulate dual-comb interferometric signals. Figure 4(b) shows one of 25 simulated interferograms. The FID signal is also clear around the centerburst. The simulation only takes laser frequency fluctuation into consideration.

Fig. 4.

	Figure Option ViewDownloadNew Window
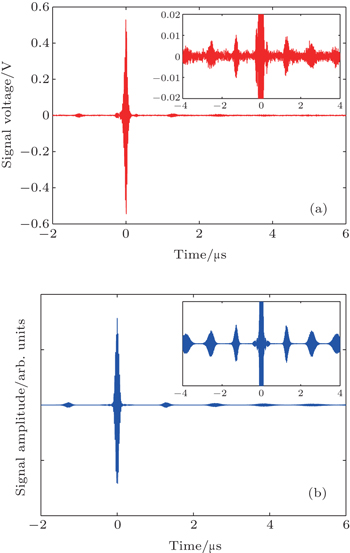	Fig. 4. (a) Interferometric signal of asynchronous dual laser combs. Inset: partial magnified free-induction decay (FID) signal. (b) Simulated interferometric signal.

Figure 5(a) shows six measured spectra. The sidelobes around the absorption peaks are suppressed due to apodization with a 12-μs triangular window. In order to average in the next step, all the interferograms are phase corrected. As laser frequency fluctuates during measurement, the absorption peak presents frequency jitter relative to the reference peak in the database, and the amplitude of the absorption peak also varies randomly.

The nominal conversion factor β is 1.25× 10⁵. As indicated in the previous analysis, a small fluctuation in f_rep leads to a considerable change in β, which further causes significant misalignment in up-conversion to the optical domain. With the parameters of the experimental setup, the RF comb is located around 46 MHz. Therefore, the RMS spectral frequency jitter is estimated from

Fig. 5.

	Figure Option ViewDownloadNew Window
	Fig. 5. Six spectra obtained from measurement (a) and simulation (c) are offset for viewing clarity. Panels (b) and (d) show the partially expanded curves in panels (a) and (c), respectively. The dashed line denotes the absorption peak at 6446.16 cm−1 from GEISA database.

Fig. 6.

	Figure Option ViewDownloadNew Window
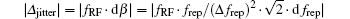
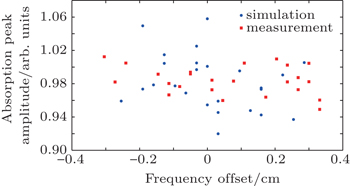	Fig. 6. Amplitude and frequency offset distributions of the absorption peak near 6446.16 cm−1 in the measured and simulated results.

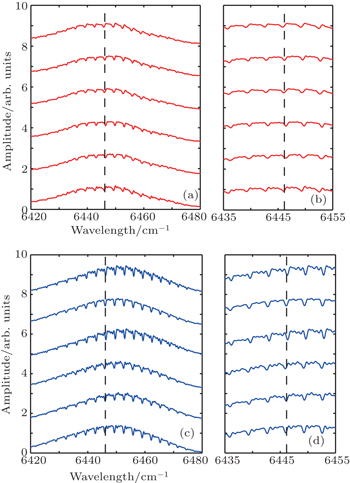
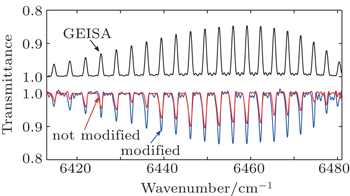
Focusing on a narrow region of interest, the frequency jitter of each absorption peak in one spectrum could be approximately treated as a constant. Therefore, the measured spectra are dynamically modified one by one with respect to the absorption peak at 6446.16 cm⁻¹. In Fig. 7, we can compare the averaged spectra with and without frequency modification, and the simulated spectrum based on the GEISA database. The signal-to-noise ratio degrades by directly averaging unmodified spectra, while it is effectively improved in the averaged spectrum with modification. The spectral resolution is 0.8 cm⁻¹.

Fig. 7.

	Figure Option ViewDownloadNew Window
	Fig. 7. Comparison between the averaged spetrum with and without modification and the simulation based on GEISA.

In this study, we demonstrate that the repetition rate fluctuation has a significant effect on spectral frequency misalignment by considering the inevitable phenomenon of laser fluctuation. By measuring the frequency noise of the repetition rate, the RMS residual frequency modulation of locked f_rep is 1.4 Hz in our system. According to the experimental setup, we simulate absorption peak jitter which is proven experimentally. By modifying the spectra with respect to the reference peak and averaging in the frequency domain, the signal-to-noise ratio is effectively improved. The averaged spectrum agrees well with the simulation result. However, this kind of modification is limited to the case of a low-resolution spectrometer. When it comes to high-resolution, more attention should be paid to the phase noises of both laser combs.

Further, the relative linewidth between laser combs is the most important parameter that seriously restricts the application of interferometric spectrometry. Next, a narrow linewidth cw laser is expected. By utilizing a phase-lock loop with a large control bandwidth, the phase noise of the comb mode that is offset-locked to the cw laser could be suppressed.^[15,16] It will benefit the coherent averaging in the time domain.