† Corresponding author. E-mail:
‡ Corresponding author. E-mail:
Project supported by the Doctoral Research Start-up Funding of Northeast Dianli University, China (Grant No. BSJXM-201332), the National Natural Science Foundation of China (Grant Nos. 11547114, 11534004, 11474129, 11274141, 11447192, and 11304116), and the Graduate Innovation Fund of Jilin University, China (Grant No. 2015091).
The excitation process of electrons from the ground state to the first excited state via the resonant laser pulse is investigated by the Bohmian mechanics method. It is found that the Bohmian particles far away from the nucleus are easier to be excited and are excited firstly, while the Bohmian particles in the ground state is subject to a strong quantum force at a certain moment, being excited to the first excited state instantaneously. A detailed analysis for one of the trajectories is made, and finally we present the space and energy distribution of 2000 Bohmian particles at several typical instants and analyze their dynamical process at these moments.
The excitation process has been studied since the foundation of quantum mechanics, and perturbation theory and numerical solution of Schrödinger equation are the two main theoretical methods to study the resonant excitation process.[1–3] The two methods are both based on the quantum theory of the Copenhagen School which describes electronic state via the concept of probability.[4–6] Copenhagen school abandons the concept of trajectory, and as a result, the excitation process of electrons from the ground state to the first excited one can only be described through the concept of probability rather than that of trajectory.
Recently, Bohmian mechanics method has attracted increasing attention,[7–10] for this method has obvious advantages in describing the interaction between the intense laser pulses and atoms as well as molecules,[11–19] through which a more intuitive understanding of the strong field physics can be obtained via the detailed analysis of the “classical” mechanical quantities, including trajectory, force, and energy.[20–31] An excited state is a quantum state whose energy is higher than the ground state of atom and molecule or cluster. Irradiated by the laser pulse resonant with the ground and first excited states of the atom, the electron in the ground state can be excited to the first excited state thoroughly as the pulse area is appropriate.[32–34] When the electron is subject to a laser pulse with long period and low intensity, and its frequency is the resonance frequency between the ground state and first excited state, electron can be excited to first excited state completely. The traditional quantum mechanics can only present the temporal evolution of the probability of each state of electron. In our work, utilizing the Bohmian mechanics method, we can describe the electronic excitation process from the ground state to the first excited one starting from the particulate nature of electron.
To study excitation process simply, we select one-dimensional model of single electron. Under the dipole approximation in the length gauge, the time-dependent Schrödinger equation (TDSE) that describes the interaction between strong laser pulse and atom is given by (atomic units are used throughout, unless otherwise stated)
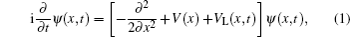
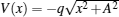
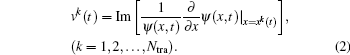

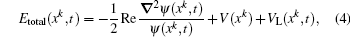

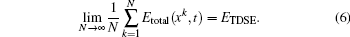
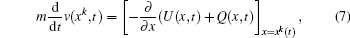
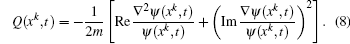
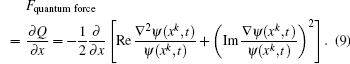
We choose a laser field whose peak amplitude, central frequency, and duration are 0.002 a.u., 0.2693 a.u., and 160 optical cycles, and this laser field can fully stimulate the excitation of electron from ground state to first excited state, as shown in Fig.
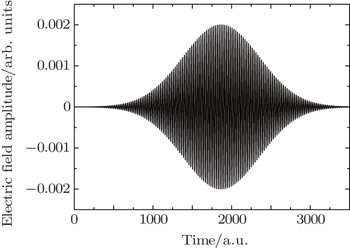 | Fig. 1. Time evolution of laser field whose peak amplitude, central frequency, and duration are 0.002 a.u., 0.2693 a.u., and 160 optical cycles, respectively. |
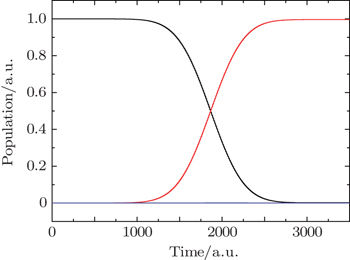 | Fig. 2. Time evolution of the population of ground state (black curve), first excited state (red curve), and other excited states (blue curve) under the action of the laser field shown in Fig. |
To illustrate that the energy calculated by the Bohmian mechanics method is still consistent with that obtained by numerically solving the TDSE, we present in Fig.
 | Fig. 3. Temporal evolution of the statistical average of the total energy of 2000 Bohmian particles (red curve) and that of average energy of electron calculated from the TDSE (black curve). |
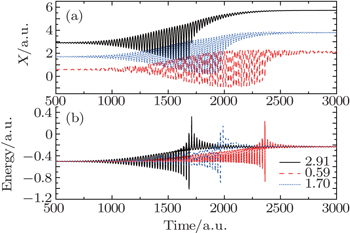
In order to better study the excitation process, we also present the evolution of the electronic wave-packet dynamics, and the corresponding Bohmian trajectories, as shown in Fig.
We choose three typical Bohmian particles whose initial positions are 2.91, 1.70, and 0.59, respectively. It is obvious that the Bohmian particle far away from the nucleus (solid black curve) is easier to be excited, and its excitation time is around 1688.93; in contrast, the Bohmian particle near the nucleus is excited after a moment (dashed red curve), and its excitation time is around 2363.23. Therefore, we can say that the Bohmian particles far away from the nucleus are easier to be excited, and are excited firstly.
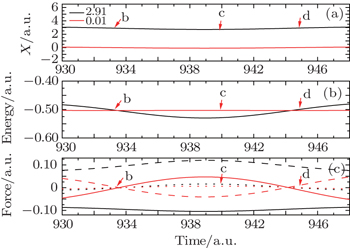
We choose one of the Bohmian particles to analyze its excitation process, as shown in Fig.
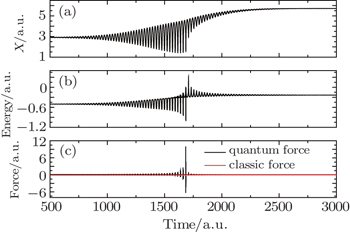 | Fig. 6. Excitation process of one Bohmian particle whose initial position is 2.9. (a) Trajectory, (b) energy, and (c) force. |
We select 11 typical instants from Fig.
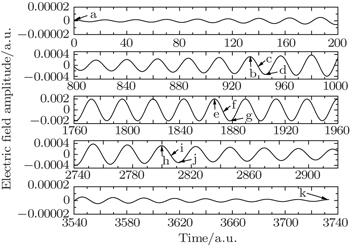 | Fig. 7. Amplified electric field shown in Fig. |
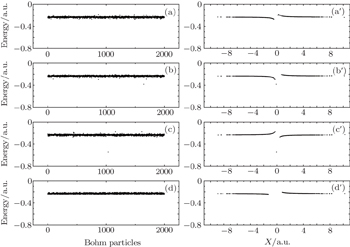
From Fig.
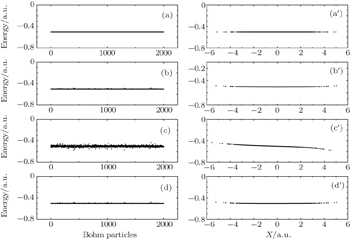 | Fig. 8. Relationship between energies and spatial positions of 2000 Bohmian particles. Panels (a), (b), (c), (d) and (a′), (b′), (c′), (d′) correspond to the four instants a, b, c, d shown in Fig. |
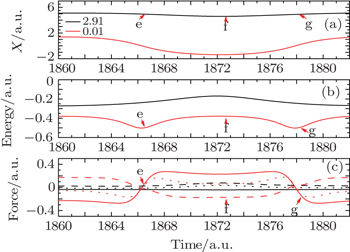
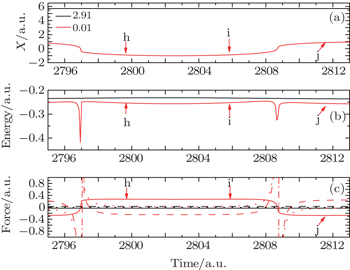
Here, we select two typical particles to explain the above phenomena: one is from the center of nuclear region, and the other one is from the edge of nuclear region whose initial positions are 2.91 and 0.01, respectively. In Fig.
As the amplitude of the electric field reaches the peak value 0.002 a.u., i.e., around the moment 1/2T, three typical instants, i.e., e, f, and g are chosen. These three instants correspond to three different electric field strengths, as shown in Fig.
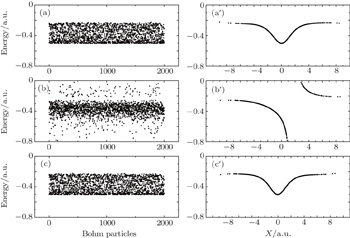 | Fig. 10. (a), (b), (c) and (a′), (b′), (c′) correspond to the three instants e, f, g shown in Fig. |
We still utilize the two Bohminan particles to analyze the phenomena shown in Fig.
Around the moment 3/4T, we select three typical instants, i.e., h, i, and j, as shown in Fig.
Figure
In this paper, we utilize the Bohmian mechanics method to describe the electronic excitation process from the angle of the trajectory. It is found that the excitation process is not a slowly changing process, but an abruptly changing one, which can be attributed to the fact that the Bohmian particle is subject to a strong quantum force at several specific moments. We also give the energy and position of Bohmian particles at several typical instants and make a detailed analysis for the dynamical process so as to make it intuitive to understand the excitation process.
| 1 | |
| 2 | |
| 3 | |
| 4 | |
| 5 | |
| 6 | |
| 7 | |
| 8 | |
| 9 | |
| 10 | |
| 11 | |
| 12 | |
| 13 | |
| 14 | |
| 15 | |
| 16 | |
| 17 | |
| 18 | |
| 19 | |
| 20 | |
| 21 | |
| 22 | |
| 23 | |
| 24 | |
| 25 | |
| 26 | |
| 27 | |
| 28 | |
| 29 | |
| 30 | |
| 31 | |
| 32 | |
| 33 | |
| 34 |