Narrow-linewidth lasers have important applications in optical atomic clocks, ^{[1– 7]} high-resolution laser spectroscopy, ^{[7, 8]} tests of fundamental physics, ^[9] and gravitational wave detection.^[10] To meet these applications, lasers with a linewidth of 1 hertz or below have been constructed.^{[7, 8, 11– 17]} Most of those lasers are frequency-stabilized to the resonance of ultra-stable, high-finesse Fabry– Perot (FP) optical cavities by using the Pound– Drever– Hall (PDH) technique.^[18] Different optical cavities are constructed according to different applications since lasers at different wavelengths are locked to their own optical cavities. In this letter, we introduce a technique to construct narrow-linewidth lasers by coherence transfer via a referenced optical frequency comb (OFC). An OFC is precisely phase-locked to an optical reference such as a cavity-stabilized narrow-linewidth laser at an available wavelength covered by the OFC. The OFC can faithfully transfer the coherence from the reference laser to any laser at wavelength within the spectrum of the comb. For applications that use multiple narrow-linewidth ultra-stable lasers, by using this technique, only one reference cavity is needed. Moreover, the frequency of those comb-stabilized lasers can conveniently be coarsely tuned by phase-locking to different comb lines and be finely tuned with phase-lock-loops (PLL).

In many applications of optical frequency comparisons between optical frequency standards at different places, OFC is often used as a “ flywheel” to transfer the frequency stability of a laser to another laser in IR for transfer through optical fiber.^[19] However, coherence transfer via OFC is also important for optical frequency synthesis^{[20, [21} and low-phase noise microwave generation.^{[20– 24]} In Ref.  [17], coherence is transferred from a cryogenic-cavity-stabilized laser at 1.5  μ m to a clock laser at 698  nm with a fiber-based laser frequency comb. In the present letter, coherence is transferred from a cavity-stabilized 1064-nm laser to 578-nm laser for probing the clock transition of ytterbium (Yb).^[25] We evaluated the linewidth of the coherence transferred laser as well as its frequency stability by comparing against a second cavity-stabilized laser at 578  nm.

In our previous work, ^[26] we constructed an OFC based on a Ti:sapphire femtosecond (fs) laser, which was referenced to a subhertz-linewidth 1064-nm laser.^[27] The absolute linewidth of the comb lines was measured to be 0.6– 1.2  Hz from 1064  nm to 532  nm; we call it a narrow-linewidth OFC. In the present work, a laser at 578  nm (f_L, slave laser) was phase-locked to the optically referenced OFC. By comparing against a second cavity-stabilized laser at 578  nm (f_T, test laser), each laser has been characterized with an absolute linewidth of 1.1  Hz and a fractional frequency instability of ∼ 1.3 × 10^{− 15} at 1  s averaging time.

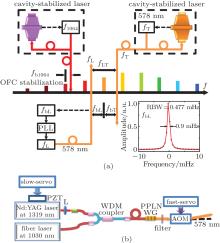
Figure  1(a) depicts the experimental setup and measurement diagram for the narrow-linewidth lasers. Both laser sources for f_L and f_T at 578  nm are constructed in a similar way, as shown in Fig.  1(b). The yellow laser light at 578  nm is generated from summing of a 1319-nm Nd:YAG laser and a 1030-nm fiber laser in a periodically poled lithium niobate (PPLN) waveguide. With 40  mW 1319-nm light and 30  mW 1030  nm laser light, 8  mW 578-nm light is generated. The frequency of the 578-nm laser can be adjusted via a piezoelectric transducer (PZT) attached to the laser crystal of the 1319-nm laser (slow-servo) and an acousto-optic modulator (AOM) located at the output of the PPLN waveguide (fast-servo).

Fig.  1.

Figure Option ViewDownloadNew Window

Fig.  1. (a) Experimental setup and measurement diagram for the narrow-linewidth lasers. The inset graph at the right corner is the spectrum of fbL observed on a fast Fourier transform (FFT) spectrum analyzer with the resolution bandwidth (RBW) of 0.477 mHz when fL was phase-locked to the narrow-linewidth comb. (b) The generation of 578-nm lasers. f1064 is the optical frequency of the reference laser at 1064  nm for OFC stabilization. fL and fT are the optical frequencies of the slave laser at 578  nm and the test laser at 578  nm, respectively. fb1064 is the beat note between the reference laser and a comb tooth at 1064  nm. fbL is the beat note between fL and the nearest comb tooth. fbT is the beat note between fT and the nearest comb tooth. fLT is the beat note between fL and fT. FNC: fiber noise cancellation; PLL: phase-lock-loop, PZT: piezoelectric transducer; PPLN-WG: periodically poled lithium niobate waveguide; AOM: acousto-optic modulator.

The OFC is based on a Ti:sapphire fs mode-locked laser with a repetition rate (f_r) of 800 MHz. A collinear self-referencing technique^[28] is employed to detect the carrier-envelope offset frequency (f_ceo), and the signal to noise ratio (SNR) of f_ceo is larger than 50 dB with the resolution bandwidth (RBW) of 300  kHz. The signal of f_ceo is phase-locked to a radio-frequency (RF) reference by controlling the pumping power of the fs laser via an AOM. To make each comb tooth gain high phase coherence, we phase-locked the OFC to a cavity-stabilized 1064-nm Nd:YAG laser (f₁₀₆₄, reference laser), which has the most probable linewidth of 0.6  Hz and a frequency instability of 1.2 × 10^{− 15} between 1  s and 40  s.^[27] The beat signal f_b1064 between the reference laser and a comb tooth at 1064  nm is used to control the repetition rate of the comb via a PLL. To increase the SNR of f_b1064, we coupled both the reference laser light and the mode-locked laser light into a piece of photonics crystal fiber (PCF) to generate sidebands beside the reference laser due to cross-phase-modulation (XPM).^[29] In this way, a number of comb teeth contribute to the beat signal instead of a single comb tooth. The SNR of f_b1064 can be higher than 40 dB (RBW: 300  kHz). Then f_b1064 is fixed by adjusting the cavity length of the fs laser via PZTs attached to the laser cavity mirrors. Each comb tooth inherits phase coherence and frequency stability of the subhertz-linewidth 1064  nm laser. By comparing against other independent narrow-linewidth lasers, we measured the absolute linewidth of the optically referenced comb teeth to be 0.6– 1.2  Hz over an octave spectrum.^[26]

The slave laser light f_L is delivered to the platform where the OFC is located via a piece of 10-meter-long polarization-maintaining (PM) fiber employing fiber noise cancellation (FNC) system^[30] to cancel the random noise induced by fiber length fluctuation. (More details are introduced in the following paragraph.) The slave laser light f_L beats with the nearest comb tooth on a fast-speed photo-detector (PD), as shown in Fig.  1(a). The beat note, f_bL, is sent to a PLL for phase-locking f_L to the OFC. The SNR of f_bL is more than 30 dB (RBW: 300  kHz), which is large enough for PLL. In order to verify that the laser has sufficient capability to track the reference laser, we measured the linewidth of f_bL on a fast Fourier transform (FFT) spectrum analyzer (in-loop), as shown in the right corner of Fig.  1(a). This measurement was limited by the RBW of the acquisition instrument.

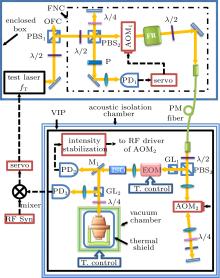
We also made out-of-loop measurements with a test laser f_T, which is frequency-stabilized to the resonance of an ultra-stable FP optical cavity by the PDH technique, as shown in Fig.  2. The FP cavity is 7.75 cm long and is made of ultra-low-expansion (ULE) glass. It is mounted vertically to suppress the sensitivity to vibration.^[31] The cavity finesse was measured to be ∼ 6.7 × 10⁵, corresponding to a cavity linewidth of ∼ 3  kHz. The cavity as well as the experimental setup for the PDH technique is on a passive vibration isolation platform (VIP) for vibration reduction. The VIP resides in a custom-made acoustic isolation chamber with an acoustic attenuation of more than 20 dB. The laser light is transferred to the VIP via a piece of PM optical fiber employing FNC.

The schematic diagram for FNC is also shown in Fig.  2. The environmental perturbation induced random phase noise through fiber is measured by comparing the light reflected from the far end surface of the PM fiber with the light from a short reference arm, and is cancelled by tuning the RF driving frequency of AOM₁. A Faraday rotator (FR) and PBS₂ are used to steer the reflected light from the far end of the fiber onto PD₁.

The laser source and the experimental setup for FNC are enclosed in a plexiglass box to reduce the disturbance of air flow. On the VIP, the output of the PM fiber passes AOM₂ twice and is phase-modulated in an electro-optic modulator (EOM). The leaking light from a partially reflecting mirror (M₁) is detected on PD₂ for stabilizing the intensity of cavity input light by controlling the RF driving power of AOM₂. The cavity reflected light is detected on PD₃ for demodulating the frequency discrimination signal (also called the PDH signal), which is used to lock the laser frequency to the cavity resonance.

Fig.  2.

Figure Option ViewDownloadNew Window

Fig.  2. Experimental setup of frequency stabilization for the test laser fT. The cavity as well as its supporting posts is located in a gold-coated copper cylinder thermal shield, all of which is enclosed in a vacuum chamber (∼ 1 × 10− 7  torr) with temperature stabilization. OFC: optical frequency comb; PBS: polarization beam splitter; λ /4: quarter-wave plate; λ /2: half-wave plate; FR: Faraday rotator; P: polarizer; GL: Glan-laser polarizer; AOM: acousto-optic modulator; EOM: electro-optic modulator; ISO: optical isolator; PD: photo-detector; FNC: fiber noise cancellation; PM: polarization-maintaining; T. control: temperature control; RF Syn: radio-frequency synthesizer; VIP: vibration isolation platform.

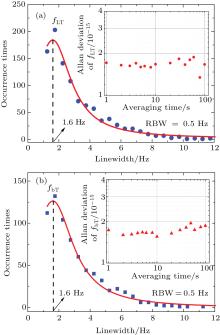
We measured the absolute linewidth and the fractional instability of the comb-stabilized slave laser f_L by comparing against the cavity-stabilized laser f_T, as shown in Fig.  1(a). The beat note f_LT between f_L and f_T, without any compensation of laser frequency drift, was mixed down to nearly 5  kHz to be analyzed on an FFT spectrum analyzer with the RBW of 0.5  Hz. One thousand groups of spectra were recorded automatically by a computer. Figure  3(a) shows the linewidth distribution (dots) of f_LT and its Lorentzian fit (solid curve). The most probable linewidth of f_LT is ∼ 1.6  Hz. Thereby, the absolute linewidth of each laser at 578  nm is 1.1  Hz, assuming that each laser contributes to the linewidth measurements equally. Meanwhile, the frequency of f_LT was automatically recorded by a digital counter. The Allan deviation of f_LT is 1.7 × 10^{− 15} at 1  s averaging time. As shown by the dots in the inset of Fig.  3(a), when a linear drift of 0.05  Hz/s was removed by fitting the data. Assuming f_L and f_T have the same frequency noise, the fractional frequency instability of each laser is calculated to be 1.3 × 10^{− 15} at 1 s averaging time, which approaches the cavity thermal noise of f_T.^{[32, 33]} According to Ref.  [26], the linewidth of the comb tooth at 578  nm is estimated to be 1.1  Hz and the fractional frequency instability is 1.3 × 10^{− 15} at 1 s averaging time, which agrees with the measured performance of this comb-stabilized slave laser.

Fig.  3.

Figure Option ViewDownloadNew Window

Fig.  3. Out-of-loop measurements of fLT and fbT. (a) Linewidth distribution of 1000 groups of the fLT (dots) and its Lorentzian fit (solid curve) when the RBW of the FFT spectrum analyzer is 0.5  Hz. The inset shows the Allan deviation of fLT (dots). (b) Linewidth distribution of 700 groups of the fbT (squares) and its Lorentzian fit (solid curve) when the RBW of the FFT spectrum analyzer is 0.5  Hz. The inset shows Allan deviation of fbT (triangles).

The same measurements of f_bT, the beat note between f_T and the nearby tooth of the narrow linewidth OFC, were made in order to evaluate the tracking ability of f_L and its limitation. The most probable linewidth of f_bT is ∼ 1.6  Hz, as shown in Fig.  3(b) and Allan deviation of f_bT is 1.7 × 10^{− 15} at 1  s averaging time, as shown in the inset of Fig.  3(b). These results, which are almost the same as those of f_LT, prove that f_L is tightly phase-locked to the nearest tooth of the OFC and its linewidth and frequency instability is limited by that of the narrow-linewidth OFC, not the phase lock loop at this point. Further improvements on the reference laser, especially to reduce the thermal noise of its reference FP cavity, ^{[7, 8, 16]} will help to reduce the linewidth of the slave laser. With the generation of low thermal-noise-limited lasers, a new comb coherence transfer technique has been developed so that the spectral purity can be transferred between optical wavelengths at the 10^{− 18} level at 1 s averaging time.^[34]

In summary, a 1-Hz-linewidth 578-nm laser was realized by phase locking to a narrow-linewidth OFC referenced to a sub-hertz 1064-nm laser. To characterize this laser, we compared it with a cavity-stabilized narrow-linewidth laser at 578  nm. Measurements show the absolute linewidth of each 578-nm clock laser is 1.1  Hz, and the frequency stability is about 1.3 × 10^{− 15} at 1  s average time.

The narrow-linewidth OFC can transfer not only the excellent frequency stability but also the phase coherence from 1064  nm to 578  nm. For applications of optical atomic clocks, multiple lasers (both cooling and clock lasers) can be simultaneously frequency-stabilized to the narrow-linewidth OFC. Moreover, clock lasers which are referenced to cold neutral atoms or a single ion can be used as the reference laser for an OFC. Then the high performance of the clock lasers could be easily transferred to lasers at ∼ 1550  nm for optical frequency dissemination and telecommunication by this technique. In many applications, high coherence as well as precise and wide-frequency-tunable lasers are preferred. They are called optical frequency synthesizers, similar to RF synthesizer but in the optical region. When replacing the slave laser with a cw laser (whose frequency can be continuously and precisely tuned), an optical frequency synthesizer will be developed. The output of the optical frequency synthesizer will have low phase noise, high coherence, and high frequency stability, depending on the reference laser.