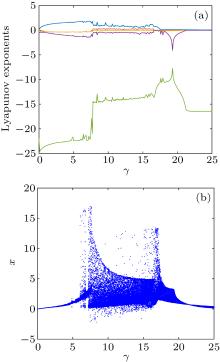
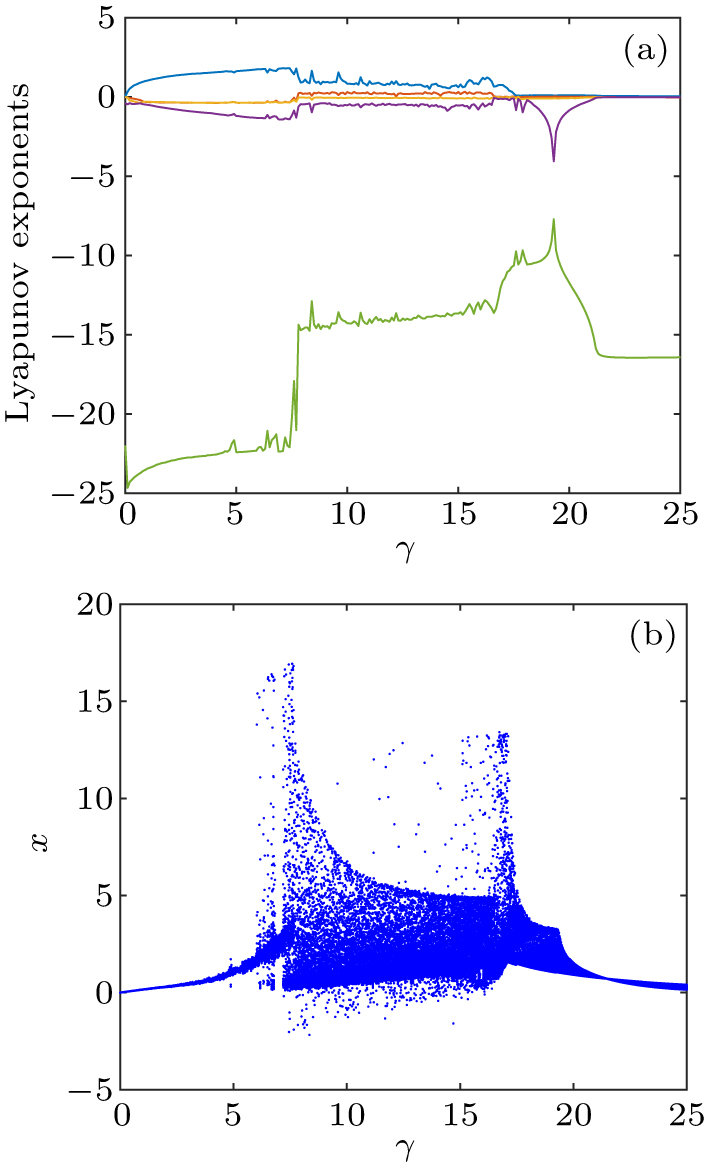
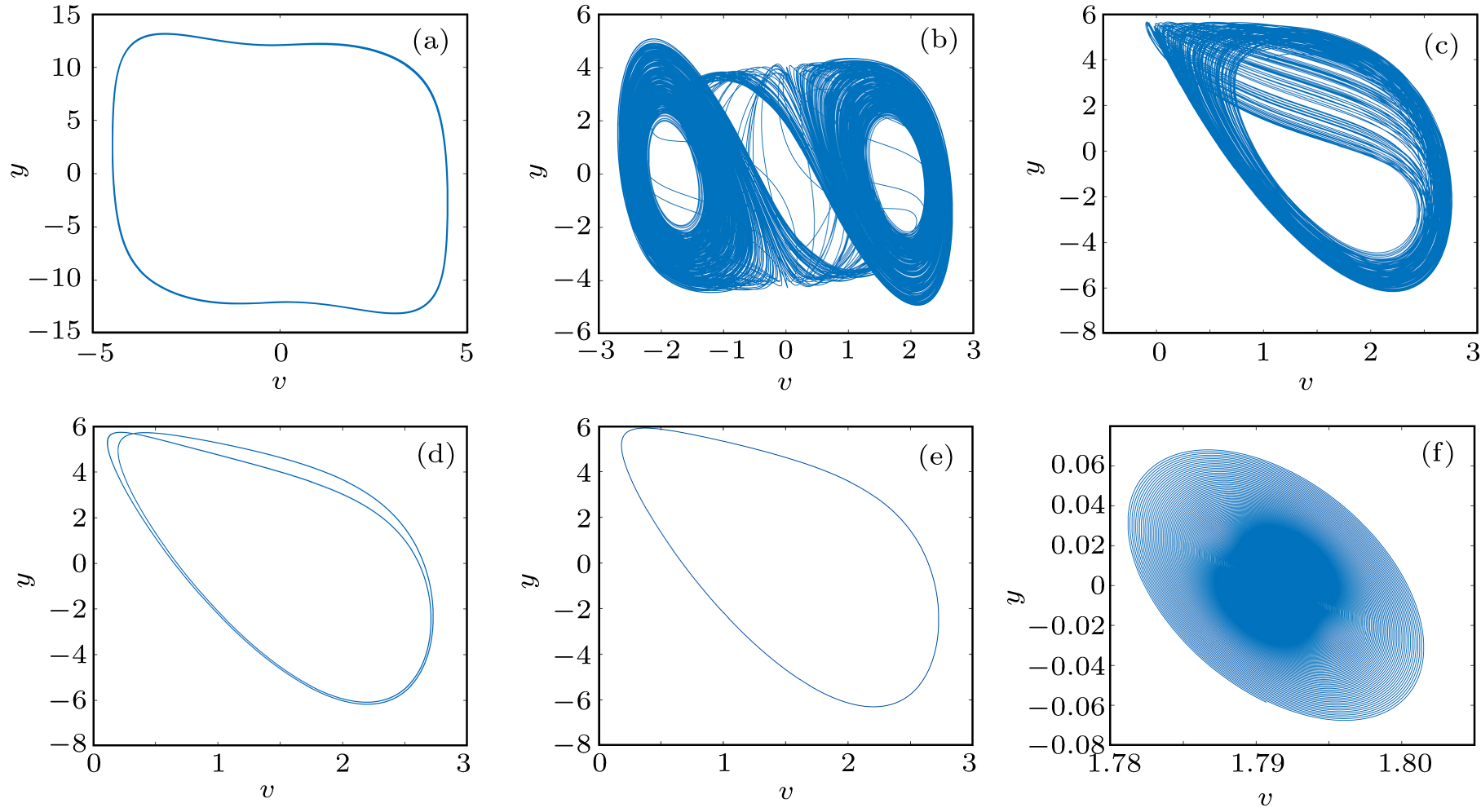
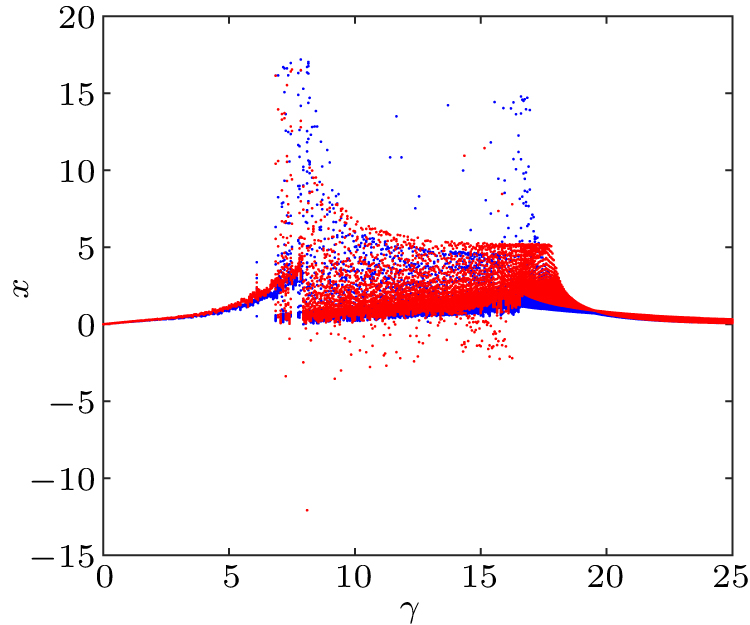
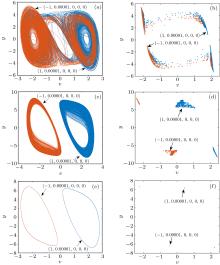
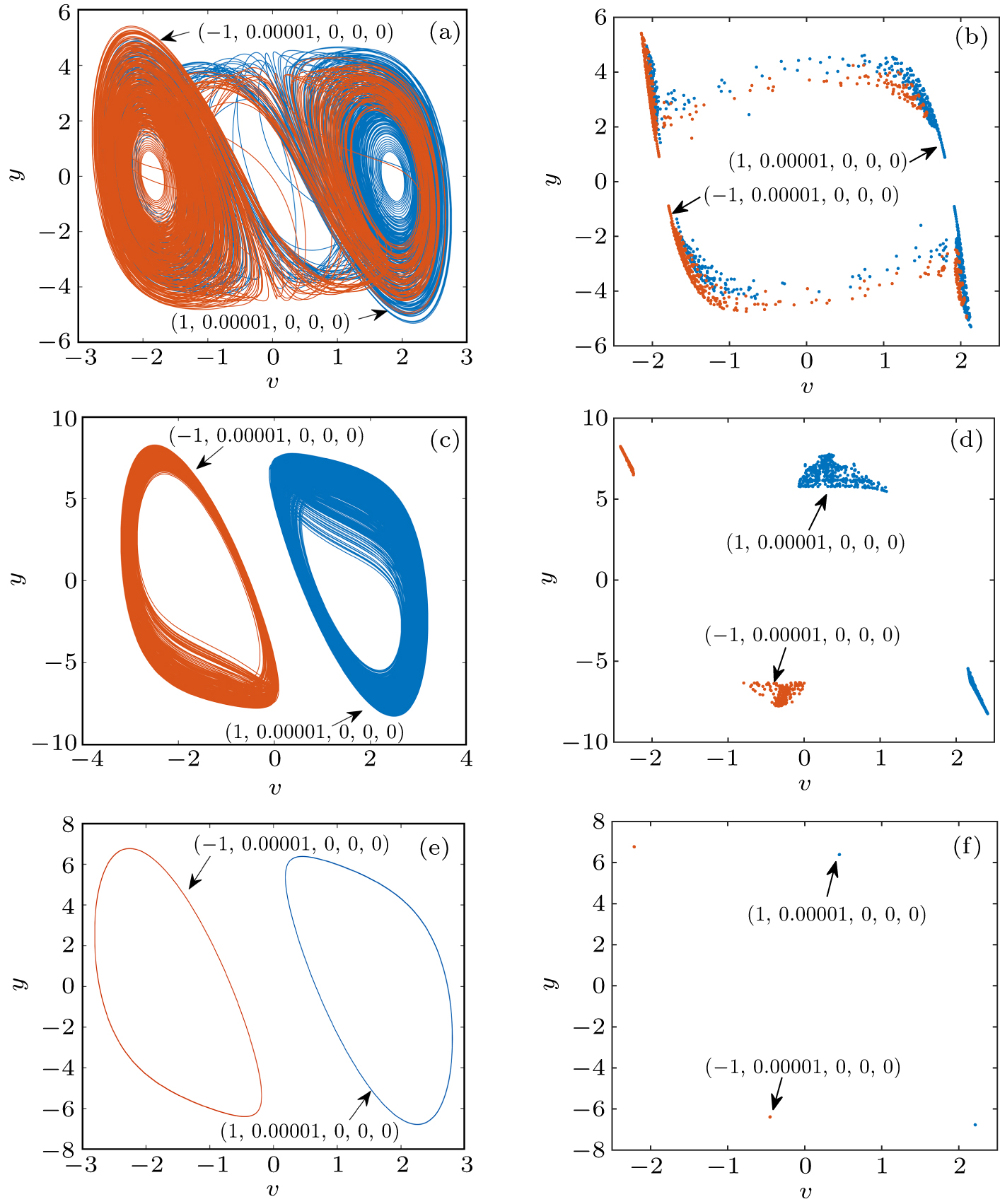
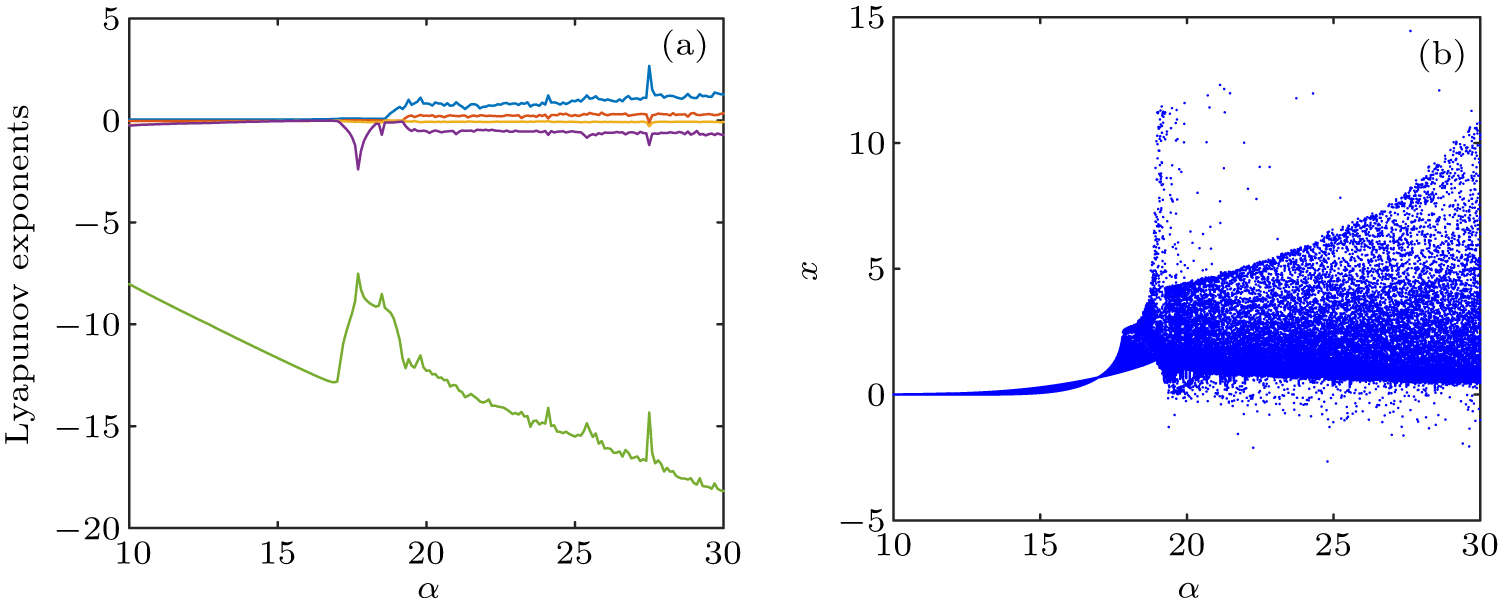
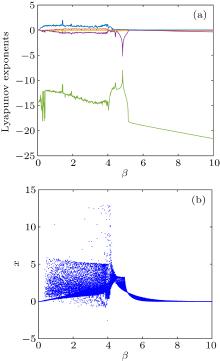
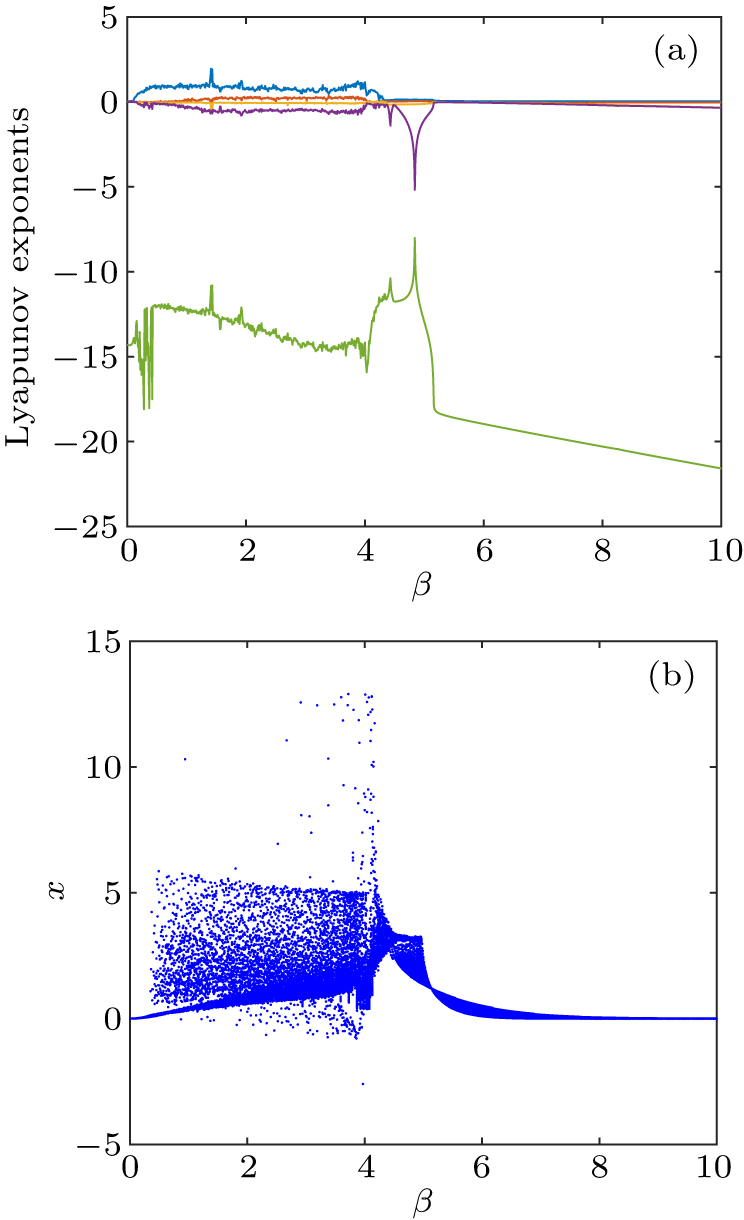
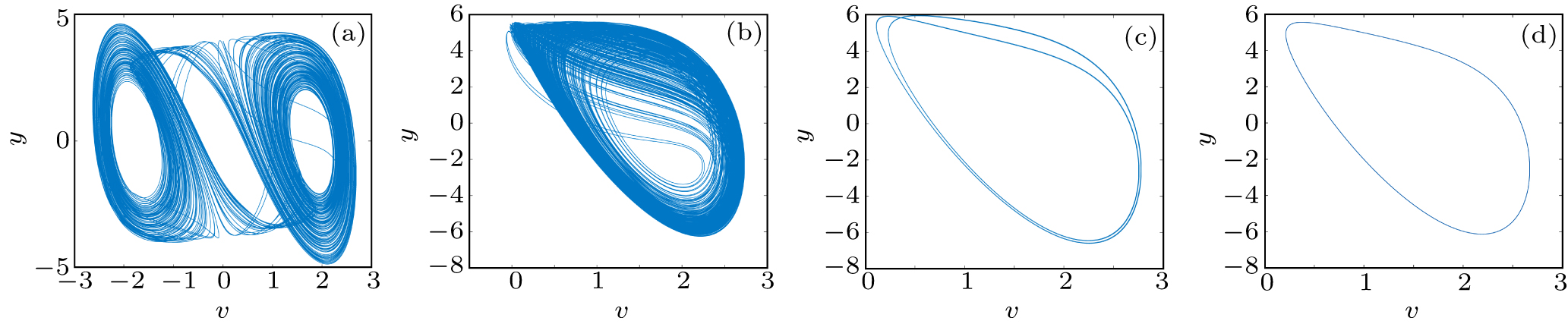
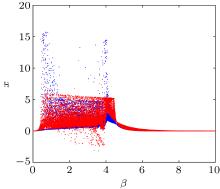
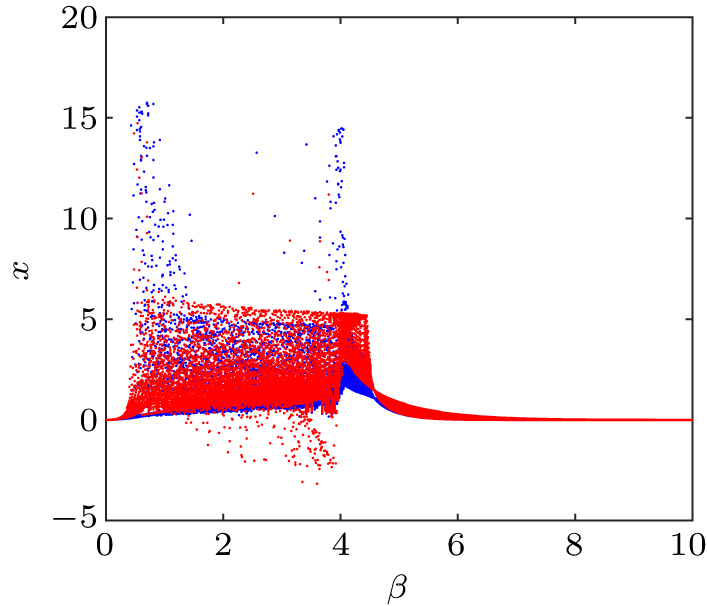
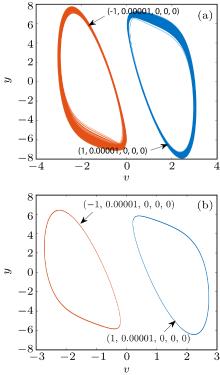
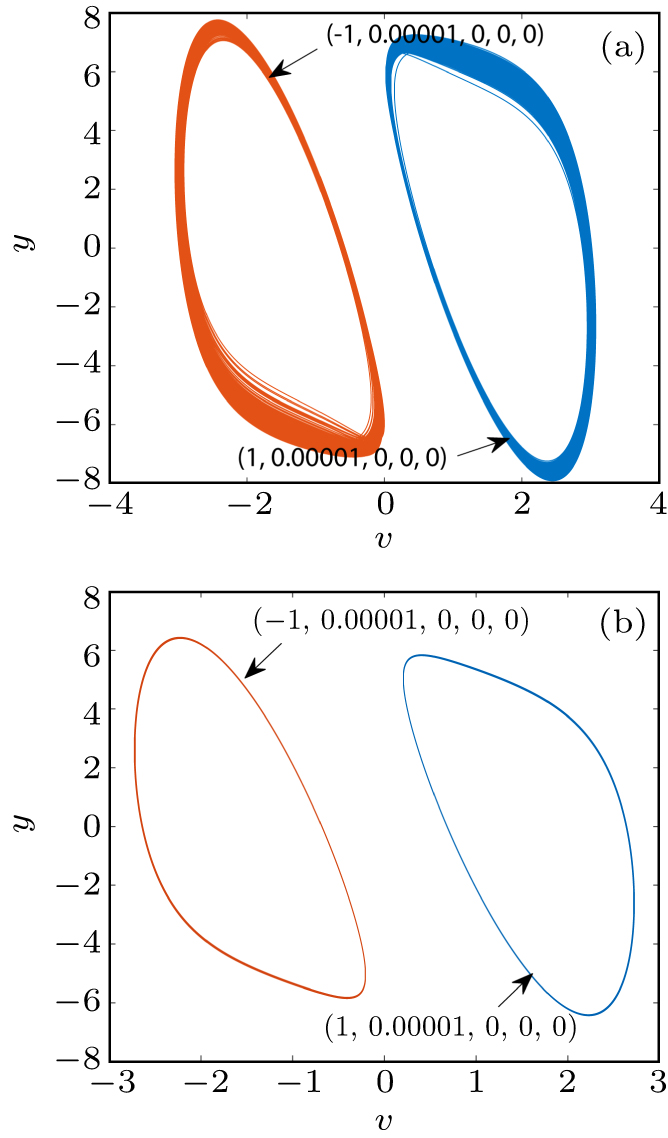
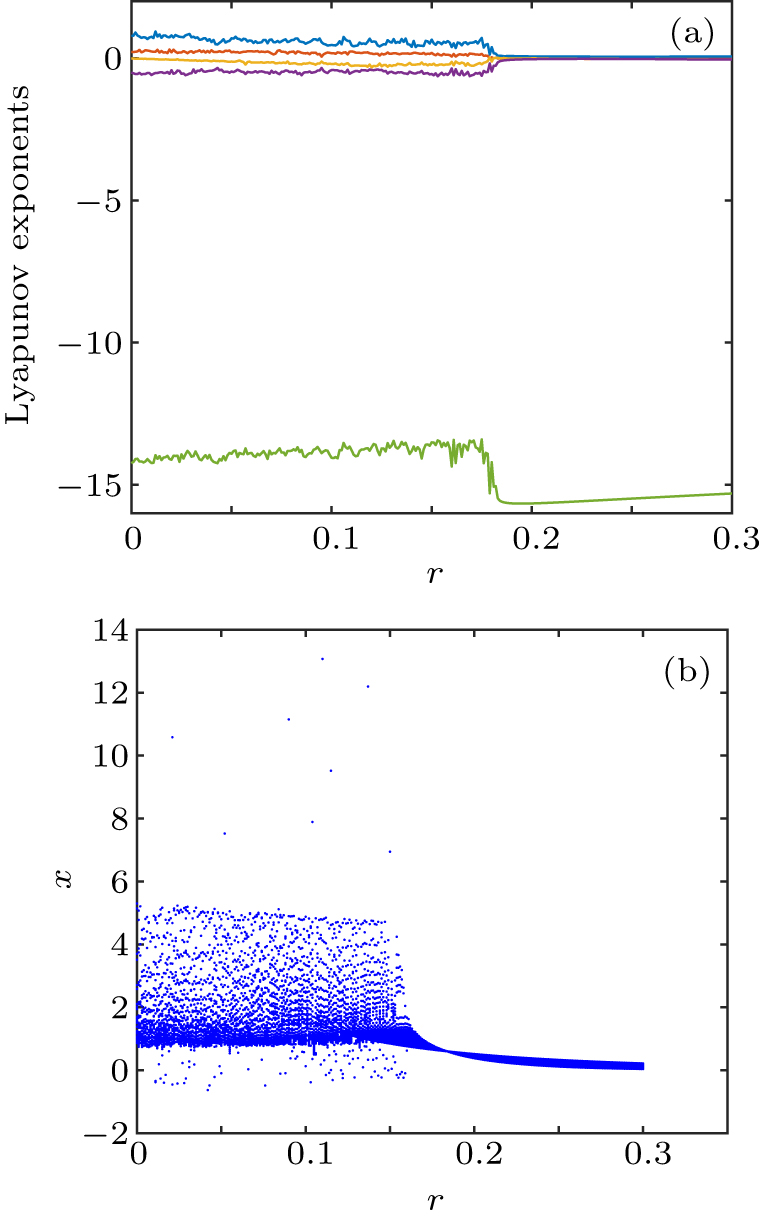
Dynamics of the two-SBT-memristor-based chaotic circuit

doi:10.1088/1674-1056/abbbe3

中国物理B ›› 2020, Vol. 29 ›› Issue (11): 110505-.doi: 10.1088/1674-1056/abbbe3

Mei Guo(郭梅)¹, Meng Zhang(张萌)¹, Ming-Long Dou(窦明龙)¹, Gang Dou(窦刚)¹^,†(), Yu-Xia Li(李玉霞)¹^,^‡()

收稿日期:2020-08-15 修回日期:2020-09-04 接受日期:2020-09-28 出版日期:2020-11-05 发布日期:2020-11-03

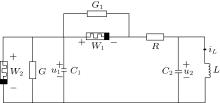
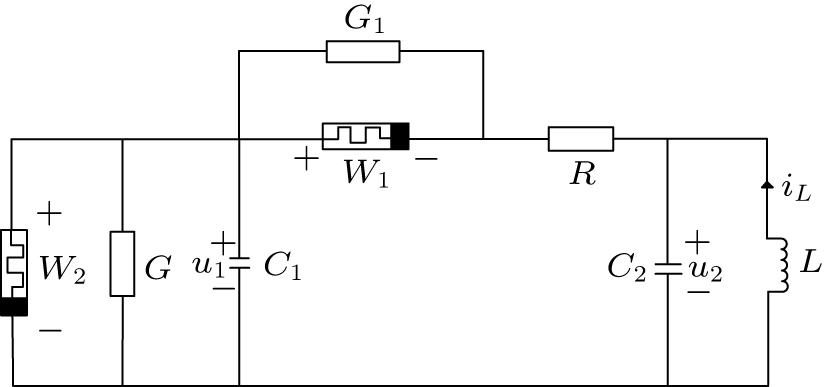
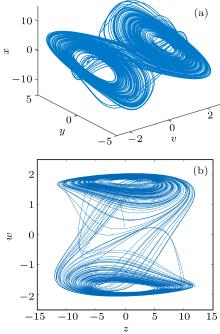
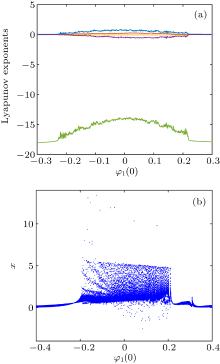
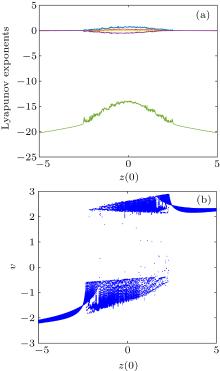
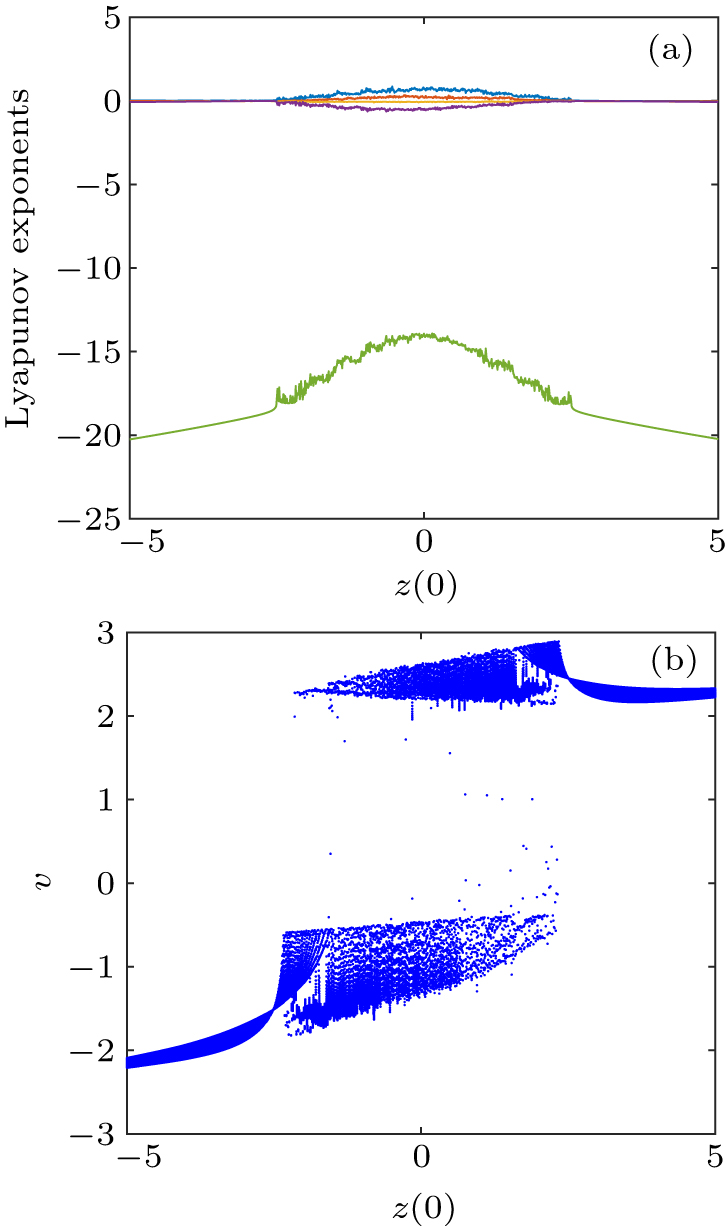
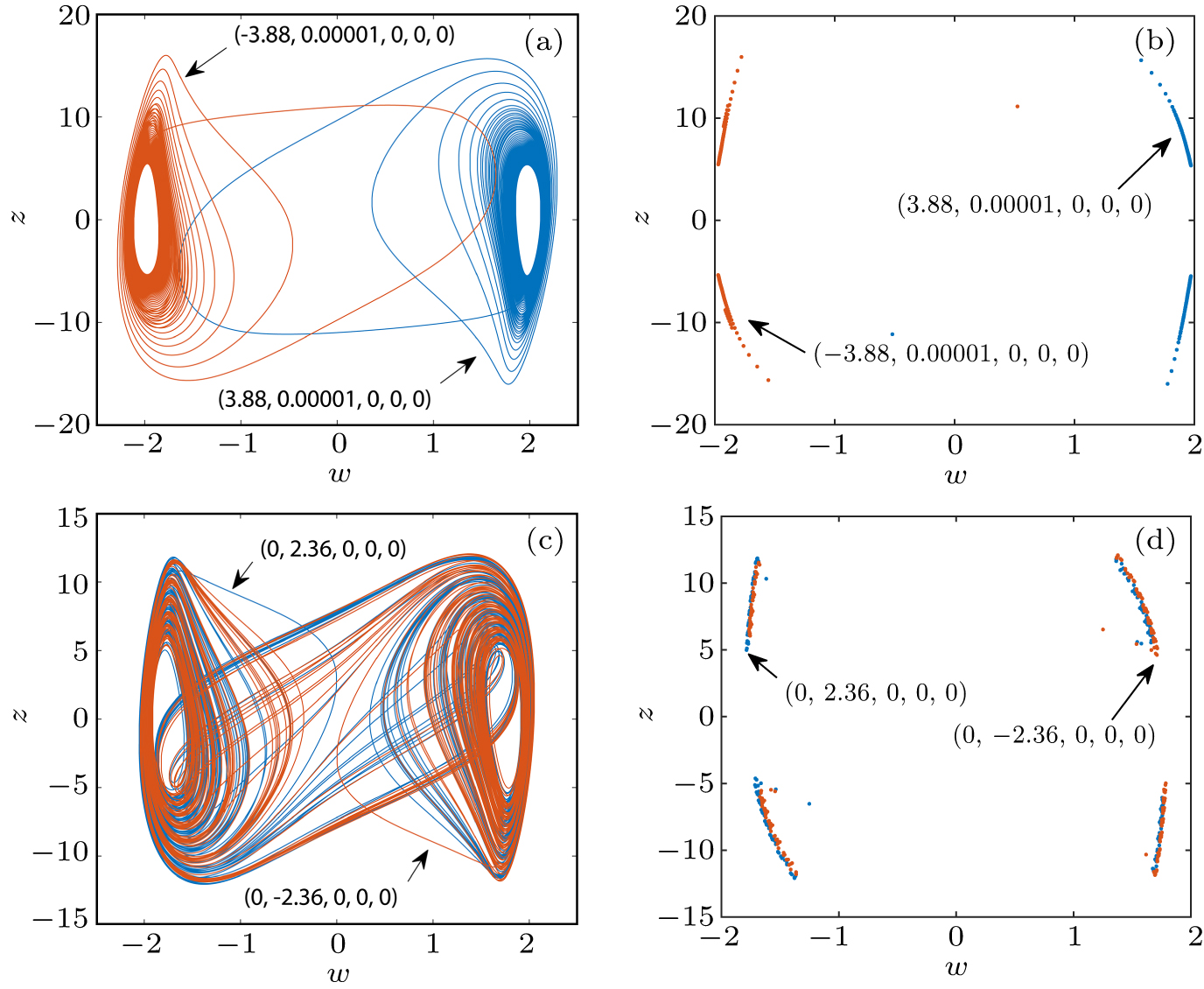
Dynamics of the two-SBT-memristor-based chaotic circuit

Mei Guo(郭梅), Meng Zhang(张萌), Ming-Long Dou(窦明龙), Gang Dou(窦刚)^†, and Yu-Xia Li(李玉霞)^‡

College of Electrical Engineering and Automation, Shandong University of Science and Technology, Qingdao 266590, China

Received:2020-08-15 Revised:2020-09-04 Accepted:2020-09-28 Online:2020-11-05 Published:2020-11-03
Contact: ^†Corresponding author. E-mail: dougang521@sdust.edu.cn ^‡Corresponding author. E-mail: yuxiali2004@sdust.edu.cn
Supported by:
the National Natural Science Foundation of China (Grant Nos. 61703247 and 61703246), the Qingdao Science and Technology Plan Project, China (Grant No. 19-6-2-2-cg), the Elite Project of Shandong University of Science and Technology, and the Taishan Scholar Project of Shandong Province of China.

摘要/Abstract

Abstract:

A two-SBT-memristor-based chaotic circuit was proposed. The stability of the equilibrium point was studied by theoretical analysis. The close dependence of the circuit dynamic characteristics on its initial conditions and circuit parameters was investigated by utilizing Lyapunov exponents spectra, bifurcation diagrams, phase diagrams, and Poincaré maps. The analysis showed that the circuit system had complex dynamic behaviors, such as stable points, period, chaos, limit cycles, and so on. In particular, the chaotic circuit produced the multistability phenomenon, such as coexisting attractors and coexisting periods.

Key words: memristor, chaotic circuit, multistability, coexisting attractors

Mei Guo(郭梅), Meng Zhang(张萌), Ming-Long Dou(窦明龙), Gang Dou(窦刚), Yu-Xia Li(李玉霞). [J]. 中国物理B, 2020, 29(11): 110505-.

Mei Guo(郭梅), Meng Zhang(张萌), Ming-Long Dou(窦明龙), Gang Dou(窦刚), and Yu-Xia Li(李玉霞). Dynamics of the two-SBT-memristor-based chaotic circuit[J]. Chin. Phys. B, 2020, 29(11): 110505-.

图/表 28

参考文献 37

[1]	Chua L O 1971 IEEE Trans. Circ. Theory 18 507 DOI: 10.1109/TCT.1971.1083337
[2]	Strukov D B, Snider G S, Stewart D R, Williams R S 2008 Nature 453 80 DOI: 10.1038/nature06932
[3]	Duan S K, Hu X F, Wang L D, Li C D 2012 Sci. China: Inf. Sci. 55 1446 DOI: 10.1007/s11432-012-4572-0
[4]	Shin S, Kim K, Kang S M 2011 IEEE Trans. Nanotechnology 10 266 DOI: 10.1109/TNANO.2009.2038610
[5]	Borghetti J, Snider G S, Kuekes P J, Yang J J, Stewart D R, Williams R S 2010 Nature 464 873 DOI: 10.1038/nature08940
[6]	Pershin Y V, Di V M 2010 Neural Networks 23 881 DOI: 10.1016/j.neunet.2010.05.001
[7]	Tour J M, He T 2008 Nature 453 42 DOI: 10.1038/453042a
[8]	Li C L, Li Z Y, Feng W, Tong Y N, Du J R, Wei D Q 2019 Int. J. Electron. Commun. 110 152861 DOI: 10.1016/j.aeue.2019.152861
[9]	Bao B C, Xu J P, Zhou G H, Ma Z H, Zou L 2011 Chin. Phys. B 20 120502 DOI: 10.1088/1674-1056/20/12/120502
[10]	Guo M, Xue Y B, Gao Z H, Zhang Y M, Dou G, Li Y X 2017 Int. J. Bifurc. Chaos 27 1730047 DOI: 10.1142/S0218127417300476
[11]	Liu C X, Liu L 2008 Chin. Phys. B 18 02188 DOI: 10.1088/1674-1056/18/6/013
[12]	Guo M, Gao Z H, Xue Y B, Dou G, Li Y X 2018 Nonlinear Dyn. 93 1681 DOI: 10.1007/s11071-018-4284-0
[13]	Dou G, Duan H Y, Yang W Y, Yang H, Guo M, Li Y X 2019 Int. J. Bifurc. Chaos 29 1950171 DOI: 10.1142/S0218127419501712
[14]	Muthuswamy B 2010 Int. J. Bifurc. Chaos 20 1335 DOI: 10.1142/S0218127410026514
[15]	Hoff A, Silva D T D, Manchein C, Albuquerque H A 2014 Phys. Lett. A 378 171 DOI: 10.1016/j.physleta.2013.11.003
[16]	Lin T C, Huang F Y, Du Z B, Lin Y C 2015 Int. J. Fuzzy Systems 17 206 DOI: 10.1007/s40815-015-0024-5
[17]	Li G L, Chen X Y 2010 Chin. Phys. B 19 030507 DOI: 10.1088/1674-1056/19/3/030507
[18]	Rocha R, Ruthiramoorthy J, Kathamuthu T 2017 Nonlinear Dyn. 88 2577 DOI: 10.1007/s11071-017-3396-2
[19]	Fozin T F, Ezhilarasu P M, Tabekoueng Z N, Leutcho G D, Kengne J, Thamilmaran K, Mezatio A B, Pelap F B 2019 Chaos 29 113105 DOI: 10.1063/1.5121028
[20]	Bao B C, Shi G D, Xu J P, Liu Z, Pan S H 2011 Sci. China Tech. Sci. 54 2180 DOI: 10.1007/s11431-011-4400-6
[21]	Buscarino A, Fortuna L, Frasca M, Gambuzza L V 2012 Chaos 22 023136 DOI: 10.1063/1.4729135
[22]	Li C, Min F H, Li C B 2018 Nonlinear Dyn. 94 2785 DOI: 10.1007/s11071-018-4524-3
[23]	Ye X L, Wang X Y, Gao S, Mou J, Wang Z S, Yang F F 2020 Nonlinear Dyn. 99 1489 DOI: 10.1007/s11071-019-05370-2
[24]	Hong Q H, Zeng Y C, Li Z J 2013 Acta Phys. Sin. 62 230502 in Chinese DOI: 10.7498/aps.62.230502
[25]	Mou J, Sun K H, Ruan J Y, He S B 2016 Nonlinear Dyn. 86 1735 DOI: 10.1007/s11071-016-2990-z
[26]	Pisarchik A N, Feudel U 2014 Phys. Rep. 540 167 DOI: 10.1016/j.physrep.2014.02.007
[27]	Shahzad M, Pham V T, Ahmad M A, Jafari S, Hadaeghi F 2008 Eur. Phys. J. Spec. Top. 224 1637 DOI: 10.1140/epjst/e2015-02485-8
[28]	Li H M, Yang Y F, Li W, He S B, Li C L 2020 Eur. Phys. J. Plus 135 579 DOI: 10.1140/epjp/s13360-020-00569-4
[29]	Peng G Y, Min F H 2017 Nonlinear Dyn. 90 1607 DOI: 10.1007/s11071-017-3752-2
[30]	Guo M, Yang W Y, Xue Y B, Gao Z H, Yuan F, Dou G, Li Y X 2019 Chaos 29 043114 DOI: 10.1063/1.5089293
[31]	Hu X Y, Liu C X, Liu L, Yao Y P, Zheng G C 2017 Chin. Phys. B 26 110502 DOI: 10.1088/1674-1056/26/11/110502
[32]	Dang X Y, Li C B, Bao B C, Wu H G 2015 Chin. Phys. B 24 050503 DOI: 10.1088/1674-1056/24/5/050503
[33]	Li C B, Lu T A, Chen G R, Xing H Y 2019 Chaos 29 051102 DOI: 10.1063/1.5097998
[34]	Tang Y X, Khalaf A J M, Rajagopal K, Pham V T, Jafari S, Tian Y 2018 Chin. Phys. B 27 040502 DOI: 10.1088/1674-1056/27/4/040502
[35]	Sprott J C, Li C B 2017 Acta Phys. Pol. B 48 97 DOI: 10.5506/APhysPolB.48.97
[36]	Dou G, Yu Y, Guo M, Zhang Y M, Sun Z, Li Y X 2017 Chin. Phys. Lett. 34 038502 DOI: 10.1088/0256-307X/34/3/038502
[37]	Zhang Y M, Dou G, Sun Z, Guo M, Li Y X 2017 Int. J. Bifurc. Chaos 27 1750148 DOI: 10.1142/S0218127417501486

Initial conditions φ₂(0)	Interval	Dynamics
	[-3.00, -1.77]	Stable point
	[-1.76, -0.64]	Transient chaos
	[−0.63, -0.40]	Period
	[−0.39, -0.33]	Single-scroll attractor
	[−0.32, 0.32]	Double-scroll attractor
	[0.33, 0.39]	Single-scroll attractor
	[0.40, 0.73]	Period
	[0.74, 1.76]	Sink
	[1.77, 3.00]	Stable point

Initial conditions	Interval	Dynamics
x(0)	[−6.00, −3.93]	Stable point
	[−3.92, 3.92]	Chaotic attractor
	[3.93, 6.00]	Stable point
y(0)	[−15.00, 15.00]	Chaotic attractor
z(0)	[−5.00, −2.55]	Stable point
	[−2.54, 2.54]	Chaotic attractor
	[2.55, 5.00]	Stable point

Initial conditions	Interval	Dynamics
α	[10.00, 17.02]	Stable point
	[17.03, 17.37]	Period
	[17.38, 17.41]	Stable point
	[17.42, 18.90]	Period
	[18.91, 19.25]	Single-scroll attractor
	[19.26, 30.00]	Double-scroll attractor
β	[0.00, 0.55]	Limit cycle
	[0.56, 4.08]	Double-scroll attractor
	[4.09, 4.21]	Single-scroll attractor
	[4.22, 5.13]	Period
	[5.14, 10.00]	Stable point
r	[0.000, 0.178]	Chaotic attractor
	[0.179, 0.300]	Stable point

Dynamics of the two-SBT-memristor-based chaotic circuit

RichHTML

PDF (PC)

赞

可视化

摘要/Abstract

引用本文

使用本文

图/表 28

参考文献 37

相关文章 0

Metrics

本文评价

推荐阅读 0