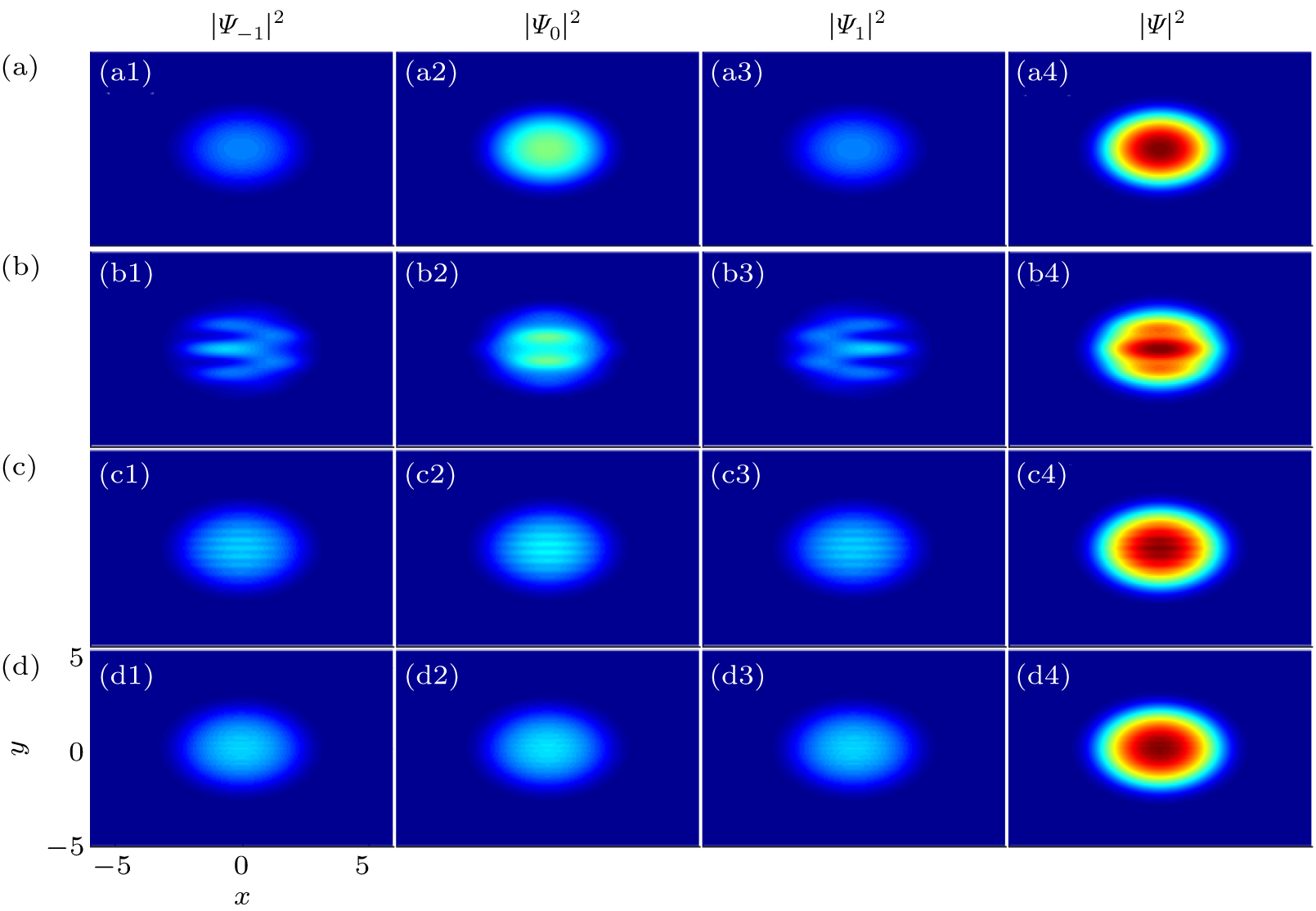
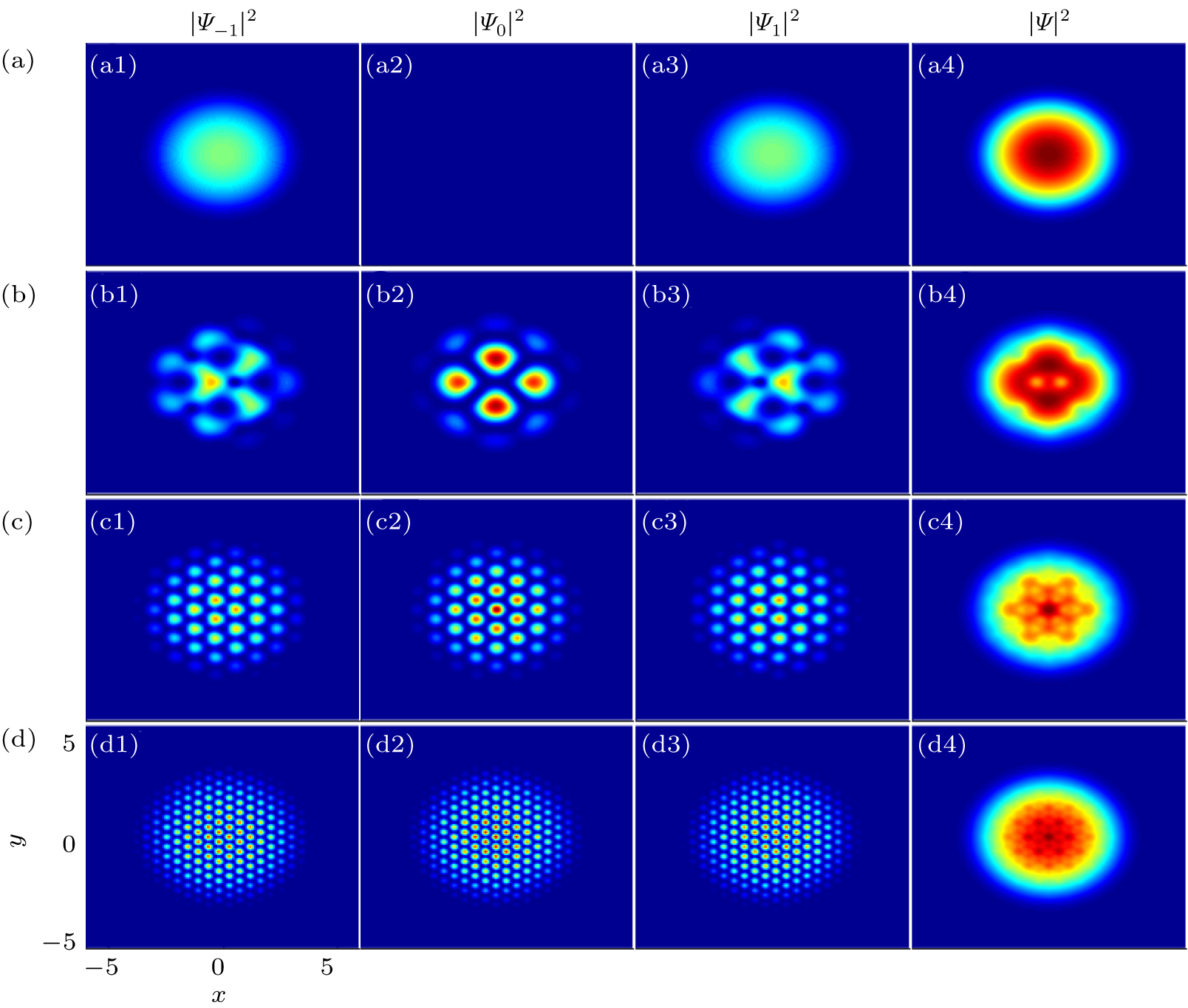
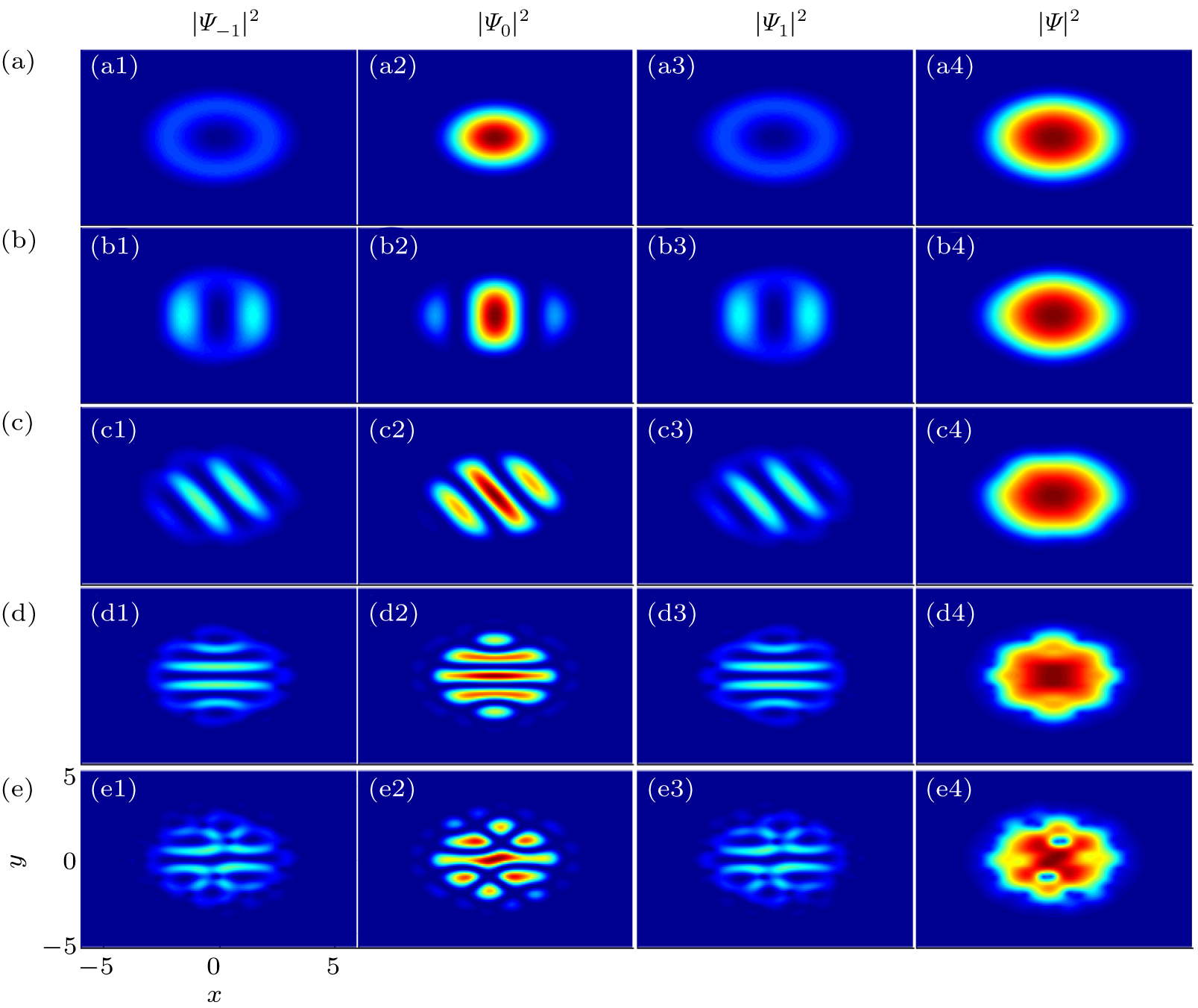
Lattice configurations in spin-1 Bose–Einstein condensates with the SU(3) spin–orbit coupling

doi:10.1088/1674-1056/abab72

中国物理B ›› 2020, Vol. 29 ›› Issue (10): 100304-.doi: 10.1088/1674-1056/abab72

Ji-Guo Wang(王继国)¹^,²^,†(), Yue-Qing Li(李月晴)¹^,², Yu-Fei Dong(董雨菲)¹^,²

收稿日期:2020-04-06 修回日期:2020-07-02 接受日期:2020-08-01 出版日期:2020-10-05 发布日期:2020-10-05
通讯作者: Ji-Guo Wang(王继国)

Lattice configurations in spin-1 Bose–Einstein condensates with the SU(3) spin–orbit coupling

Ji-Guo Wang(王继国)^1,2,†, Yue-Qing Li(李月晴)^1,2, and Yu-Fei Dong(董雨菲)^1,2

¹ Department of Mathematics and Physics, Shijiazhuang TieDao University, Shijiazhuang 050043, China
² Institute of Applied Physics, Shijiazhuang TieDao University, Shijiazhuang 050043, China

Received:2020-04-06 Revised:2020-07-02 Accepted:2020-08-01 Online:2020-10-05 Published:2020-10-05
Contact: ^†Corresponding author. E-mail: wangjiguo@stdu.edu.cn
About author:
†Corresponding author. E-mail: wangjiguo@stdu.edu.cn
* Project supported by the National Natural Science Foundation of China (Grant No. 11904242) and the Natural Science Foundation of Hebei Province, China (Grant No. A2019210280).

摘要/Abstract

Abstract:

We consider the SU(3) spin–orbit coupled spin-1 Bose–Einstein condensates in a two-dimensional harmonic trap. The competition between the SU(3) spin–orbit coupling and the spin-exchange interaction results in a rich variety of lattice configurations. The ground-state phase diagram spanned by the isotropic SU(3) spin–orbit coupling and the spin–spin interaction is presented. Five ground-state phases can be identified on the phase diagram, including the plane wave phase, the stripe phase, the kagome lattice phase, the stripe-honeycomb lattice phase, and the honeycomb hexagonal lattice phase. The system undergoes a sequence of phase transitions from the rectangular lattice phase to the honeycomb hexagonal lattice phase, and to the triangular lattice phase in spin-1 Bose–Einstein condensates with anisotrpic SU(3) spin–orbit coupling.

Key words: Bose-Einstein condensates, lattice configurations, SU(3) spin-orbit coupling

中图分类号: (Tunneling, Josephson effect, Bose-Einstein condensates in periodic potentials, solitons, vortices, and topological excitations)

03.75.Lm

03.75.Mn (Multicomponent condensates; spinor condensates) 67.85.Hj (Bose-Einstein condensates in optical potentials) 67.80.K- (Other supersolids)

Ji-Guo Wang(王继国), Yue-Qing Li(李月晴), Yu-Fei Dong(董雨菲). [J]. 中国物理B, 2020, 29(10): 100304-.

Ji-Guo Wang(王继国)†, Yue-Qing Li(李月晴), and Yu-Fei Dong(董雨菲). Lattice configurations in spin-1 Bose–Einstein condensates with the SU(3) spin–orbit coupling[J]. Chin. Phys. B, 2020, 29(10): 100304-.

图/表 7

参考文献 61

[1]	Lin Y J, Compton R L, Perry A R, Phillips W D, Porto J V, Spielman I B 2009 Phys. Rev. Lett. 102 130401 DOI: 10.1103/PhysRevLett.102.130401
[2]	Lin Y J, Compton R L, Jiménez-García K, Porto J V, Spielman I B 2009 Nature 462 628 DOI: 10.1038/nature08609
[3]	Lin Y J, Jiménez-García K, Spielman I B 2011 Nature 471 83 DOI: 10.1038/nature09887
[4]	Lin Y J, Compton R L, Jiménez-García K, Phillips W D, Porto J V, Spielman I B 2011 Nat. Phys. 7 531 DOI: 10.1038/nphys1954
[5]	Anderson B M, Juzeliunas G, Galitski V M, Spielman I B 2012 Phys. Rev. Lett. 108 235301 DOI: 10.1103/PhysRevLett.108.235301
[6]	Wu Z, Zhang L, Sun W, Xu X T, Wang B Z, Ji S C, Deng Y, Chen S, Liu X J, Pan J W 2016 Science 354 83 DOI: 10.1126/science.aaf6689
[7]	Huang L, Meng Z, Wang P, Peng P, Zhang S L, Chen L, Li D, Zhou Q, Zhang J 2016 Nat. Phys. 12 540 DOI: 10.1038/nphys3672
[8]	Sun W, Wang B Z, Xu X T, Yi C R, Zhang L, Wu Z, Deng Y, Liu X J, Chen S, Pan J W 2018 Phys. Rev. Lett. 121 150401 DOI: 10.1103/PhysRevLett.121.150401
[9]	Wang C, Gao C, Jian C M, Zhai H 2010 Phys. Rev. Lett. 105 160403 DOI: 10.1103/PhysRevLett.105.160403
[10]	Wu C, Mondragon-Shem I 2011 Chin. Phys. Lett. 28 097102 DOI: 10.1088/0256-307X/28/9/097102
[11]	Li Y, Pitaevskii L P, Stringari S 2012 Phys. Rev. Lett. 108 225301 DOI: 10.1103/PhysRevLett.108.225301
[12]	Chen X, Rabinovic M, Anderson B M, Santos L 2014 Phys. Rev. A 90 043632 DOI: 10.1103/PhysRevA.90.043632
[13]	Wilson R M, Anderson B M, Clark C W 2013 Phys. Rev. Lett. 111 185303 DOI: 10.1103/PhysRevLett.111.185303
[14]	Achilleos V, Frantzeskakis D J, Kevrekidis P G, Pelinovsky D E 2013 Phys. Rev. Lett. 110 264101 DOI: 10.1103/PhysRevLett.110.264101
[15]	Kasamatsu K 2015 Phys. Rev. A 92 063608 DOI: 10.1103/PhysRevA.92.063608
[16]	Zhang D W, Fu L B, Wang Z D, Zhu S L 2012 Phys. Rev. A 85 043609 DOI: 10.1103/PhysRevA.85.043609
[17]	Xu Y, Zhang Y P, Wu B 2013 Phys. Rev. A 87 013614 DOI: 10.1103/PhysRevA.87.013614
[18]	Sakaguchi H, Li B, Malomed B A 2014 Phys. Rev. E 89 032920 DOI: 10.1103/PhysRevE.89.032920
[19]	Zhong R X, Chen Z P, Huang C Q, Luo Z H, Tan H S, Malomed B A, Li Y Y 2018 Front. Phys. 13 130311 DOI: 10.1007/s11467-018-0778-y
[20]	Li Y Y, Zhang X L, Zhong R X, Luo Z H, Liu B, Huang C Q, Pang W, Malomed B A 2019 Commun Nonlinear Sci Numer Simulat 73 481 DOI: 10.1016/j.cnsns.2019.01.031
[21]	Ho T L 1998 Phys. Rev. Lett. 81 742 DOI: 10.1103/PhysRevLett.81.742
[22]	Ciobanu C V, Yip S K, Ho T L 2000 Phys. Rev. A 61 033607 DOI: 10.1103/PhysRevA.61.033607
[23]	Song J L, Semenoff G W, Zhou F 2007 Phys. Rev. Lett. 98 160408 DOI: 10.1103/PhysRevLett.98.160408
[24]	Turner A M, Barnett R, Demler E, Vishwanath A 2007 Phys. Rev. Lett. 98 190404 DOI: 10.1103/PhysRevLett.98.190404
[25]	Martone G I, Pepe F V, Facchi P, Pascazio S, Stringari S 2016 Phys. Rev. Lett. 117 125301 DOI: 10.1103/PhysRevLett.117.125301
[26]	Campbell D L, Price R M, Putra A, Valdés-Curiel A, Trypogeorgos D, Spielman I B 2016 Nat. Commun. 7 10897 DOI: 10.1038/ncomms10897
[27]	Luo X, Wu L, Chen J, Guan Q, Gao K, Xu Z F, You L, Wang R 2016 Sci. Rep. 6 18983 DOI: 10.1038/srep18983
[28]	Wen L, Sun Q, Wang H Q, Ji A C, Liu W M 2012 Phys. Rev. A 86 043602 DOI: 10.1103/PhysRevA.86.043602
[29]	Lan Z H, Öhberg P 2014 Phys. Rev. A 89 023630 DOI: 10.1103/PhysRevA.89.023630
[30]	Natu S S, Li X P, Cole W S 2015 Phys. Rev. A 91 023608 DOI: 10.1103/PhysRevA.91.023608
[31]	Sun K, Qu C L, Xu Y, Zhang Y P, Zhang C W 2016 Phys. Rev. A 93 023615 DOI: 10.1103/PhysRevA.93.023615
[32]	Yu Z Q 2016 Phys. Rev. A 93 033648 DOI: 10.1103/PhysRevA.93.033648
[33]	Hurst H M, Wilson J H, Pixley J H, Spielman I B, Natu S S 2016 Phys. Rev. A 94 063613 DOI: 10.1103/PhysRevA.94.063613
[34]	Wang J G, Xu L L, Yang S J 2017 Phys. Rev. A 96 033629 DOI: 10.1103/PhysRevA.96.033629
[35]	Huang X Y, Sun F X, Zhang W, He Q Y, Sun C P 2017 Phys. Rev. A 95 013605 DOI: 10.1103/PhysRevA.95.013605
[36]	Wang J G, Yang S J 2018 J. Phys.: Condens. Matter 30 295404 DOI: 10.1088/1361-648X/aacc42
[37]	Wang J G, Yang S J 2018 Eur. Phys. J. Plus 133 441 DOI: 10.1140/epjp/i2018-12331-4
[38]	Peng P, Li G Q, Zhao L C, Yang W L, Yang Z Y 2019 Phys. Lett. A 383 2883 DOI: 10.1016/j.physleta.2019.06.006
[39]	Xu Z F, Lü R, You L 2011 Phys. Rev. A 83 053602 DOI: 10.1103/PhysRevA.83.053602
[40]	Kawakami T, Mizushima T, Machida K 2011 Phys. Rev. A 84 011607 DOI: 10.1103/PhysRevA.84.011607
[41]	Wang J G, Wang W, Yang S J 2019 Phys. Lett. A 383 566 DOI: 10.1016/j.physleta.2018.11.023
[42]	Wan N S, Li Y E, Xue J K 2019 Phys. Rev. E 99 062220 DOI: 10.1103/PhysRevE.99.062220
[43]	Gautam S, Adhikari S K 2015 Phys. Rev. A 91 013624 DOI: 10.1103/PhysRevA.91.013624
[44]	Gautam S, Adhikari S K 2015 Phys. Rev. A 91 063617 DOI: 10.1103/PhysRevA.91.063617
[45]	Grab T, Chhajlany R W, Muschik C A, Lewenstein M 2014 Phys. Rev. B 90 195127 DOI: 10.1103/PhysRevB.90.195127
[46]	Barnett R, Boyd G R, Galitski V 2012 Phys. Rev. Lett. 109 235308 DOI: 10.1103/PhysRevLett.109.235308
[47]	Han W, Zhang X F, Song S W, Saito H, Zhang W, Liu W M, Zhang S G 2016 Phys. Rev. A 94 033629 DOI: 10.1103/PhysRevA.94.033629
[48]	Li H, Chen F L 2019 Chin. Phys. B 28 070302 DOI: 10.1088/1674-1056/28/7/070302
[49]	Yue H X, Liu Y K 2020 Commun. Theor. Phys. 72 025501 DOI: 10.1088/1572-9494/ab6907
[50]	Kawaguchi Y, Ueda M 2012 Phys. Rep. 520 253 DOI: 10.1016/j.physrep.2012.07.005
[51]	Bao W, Jin S, Markowich P A 2002 J. Comput. Phys. 175 487 DOI: 10.1006/jcph.2001.6956
[52]	Bao W, Jaksch D, Markowich P A 2003 J. Comput. Phys. 187 318 DOI: 10.1016/S0021-9991(03)00102-5
[53]	Bao W, Jaksch D, Markowich P A 2004 Multiscale Model. Simul. 2 210 DOI: 10.1137/030600209
[54]	Bao W, Chern I L, Zhang Y Z 2013 J. Comput. Phys. 253 189 DOI: 10.1016/j.jcp.2013.06.036
[55]	Wang H 2007 Int. J. Comput. Math. 84 925 DOI: 10.1080/00207160701458369
[56]	Lim F Y, Bao W 2008 Phys. Rev. E 78 066704 DOI: 10.1103/PhysRevE.78.066704
[57]	Bao W, Lim F Y 2008 Siam J. Sci. Comp. 30 1925 DOI: 10.1137/070698488
[58]	Gautam S, Adhikari S K 2014 Phys. Rev. A 90 043619 DOI: 10.1103/PhysRevA.90.043619
[59]	Gautam S, Adhikari S K 2017 Phys. Rev. A 95 013608 DOI: 10.1103/PhysRevA.95.013608
[60]	Peng P, Li G Q, Yang W L, Yang Z Y 2018 Phys. Lett. A 382 2493 DOI: 10.1016/j.physleta.2018.07.026
[61]	Kasamatsu K, Tsubota M, Ueda M 2004 Phys. Rev. Lett. 93 250406 DOI: 10.1103/PhysRevLett.93.250406

Lattice configurations in spin-1 Bose–Einstein condensates with the SU(3) spin–orbit coupling

RichHTML

PDF (PC)

赞

可视化

摘要/Abstract

引用本文

使用本文

图/表 7

参考文献 61

相关文章 15

Metrics

本文评价

推荐阅读 0

[1]	Xiao-Qiang Su(苏晓强), Zong-Ju Xu(许宗菊), and You-Quan Zhao(赵有权). Entanglement and thermalization in the extended Bose-Hubbard model after a quantum quench: A correlation analysis[J]. 中国物理B, 2023, 32(2): 20506-020506.
[2]	Liangwei Wang(王良伟), Kai Wen(文凯), Fangde Liu(刘方德), Yunda Li(李云达), Pengjun Wang(王鹏军), Lianghui Huang(黄良辉), Liangchao Chen(陈良超), Wei Han(韩伟), Zengming Meng(孟增明), and Jing Zhang(张靖). Experimental realization of two-dimensional single-layer ultracold gases of ⁸⁷Rb in an accordion lattice[J]. 中国物理B, 2022, 31(10): 103401-103401.
[3]	Geng Li(李更), Shiyu Zhu(朱诗雨), Peng Fan(范朋), Lu Cao(曹路), and Hong-Jun Gao(高鸿钧). Exploring Majorana zero modes in iron-based superconductors[J]. 中国物理B, 2022, 31(8): 80301-080301.
[4]	S Wang(王双), Y H Liu(刘元慧), and T F Xu(徐天赋). Gap solitons of spin-orbit-coupled Bose-Einstein condensates in $\mathcal{PT}$ periodic potential[J]. 中国物理B, 2022, 31(7): 70306-070306.
[5]	Hao Zhu(朱浩), Shou-Gen Yin(印寿根), and Wu-Ming Liu(刘伍明). Vortex chains induced by anisotropic spin-orbit coupling and magnetic field in spin-2 Bose-Einstein condensates[J]. 中国物理B, 2022, 31(6): 60305-060305.
[6]	Juewen Fan(范珏雯), Bingyan Jiang(江丙炎), Jiaji Zhao(赵嘉佶), Ran Bi(毕然), Jiadong Zhou(周家东), Zheng Liu(刘政), Guang Yang(杨光), Jie Shen(沈洁), Fanming Qu(屈凡明), Li Lu(吕力), Ning Kang(康宁), and Xiaosong Wu(吴孝松). Asymmetric Fraunhofer pattern in Josephson junctions from heterodimensional superlattice V₅S₈[J]. 中国物理B, 2022, 31(5): 57402-057402.
[7]	Hao Zhu(朱浩), Shou-Gen Yin(印寿根), and Wu-Ming Liu(刘伍明). Manipulating vortices in F=2 Bose-Einstein condensates through magnetic field and spin-orbit coupling[J]. 中国物理B, 2022, 31(4): 40306-040306.
[8]	Chao Zeng(曾超), Zhiwei Guo(郭志伟), Kejia Zhu(祝可嘉), Caifu Fan(范才富), Guo Li(李果), Jun Jiang(江俊), Yunhui Li(李云辉), Haitao Jiang(江海涛), Yaping Yang(羊亚平), Yong Sun(孙勇), and Hong Chen(陈鸿). Efficient and stable wireless power transfer based on the non-Hermitian physics[J]. 中国物理B, 2022, 31(1): 10307-010307.
[9]	Lupei Qin(秦陆培), Wei Feng(冯伟), and Xin-Qi Li(李新奇). Cross correlation mediated by distant Majorana zero modes with no overlap[J]. 中国物理B, 2022, 31(1): 17402-017402.
[10]	Li Wang(王力), Ji Li(李吉), Xiao-Lin Zhou(周晓林), Xiang-Rong Chen(陈向荣), and Wu-Ming Liu(刘伍明). Adjustable half-skyrmion chains induced by SU(3) spin-orbit coupling in rotating Bose-Einstein condensates[J]. 中国物理B, 2021, 30(11): 110312-110312.
[11]	Hui Guo(郭慧), Xu Qiu(邱旭), Yan Ma(马燕), Hai-Feng Jiang(姜海峰), and Xiao-Fei Zhang(张晓斐). Dynamics of bright soliton in a spin-orbit coupled spin-1 Bose-Einstein condensate[J]. 中国物理B, 2021, 30(6): 60310-060310.
[12]	Sheng-Hui Zhao(赵生辉), Wang-Hao Tian(田王昊), Xue-Lian Liang(梁雪连), Ze He(何泽), Pei Wang(王培), Lu Ji(季鲁), Ming He(何明), and Hua-Bing Wang(王华兵). Transport properties of Tl₂Ba₂CaCu₂O₈ microbridges on a low-angle step substrate[J]. 中国物理B, 2021, 30(6): 60308-060308.
[13]	Ji-Li Ma(马吉利), Xiao-Xun Li(李晓旬), Rui-Jin Cheng(程瑞锦), Ai-Xia Zhang(张爱霞), and Ju-Kui Xue(薛具奎). Dynamical stability of dipolar condensate in a parametrically modulated one-dimensional optical lattice[J]. 中国物理B, 2021, 30(6): 60307-060307.
[14]	. [J]. 中国物理B, 2021, 30(3): 30302-.
[15]	. [J]. 中国物理B, 2020, 29(12): 120501-.