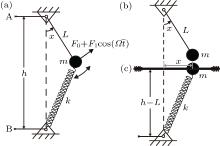
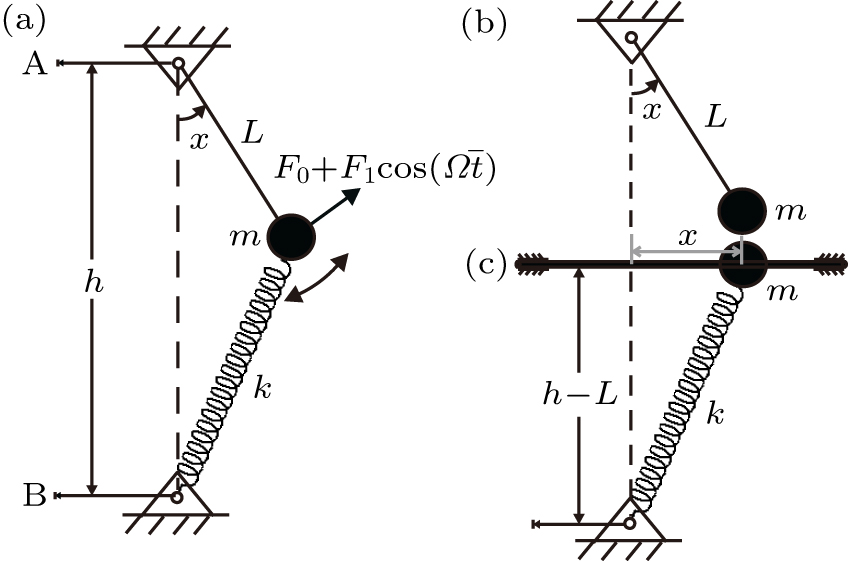
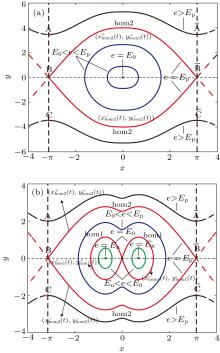
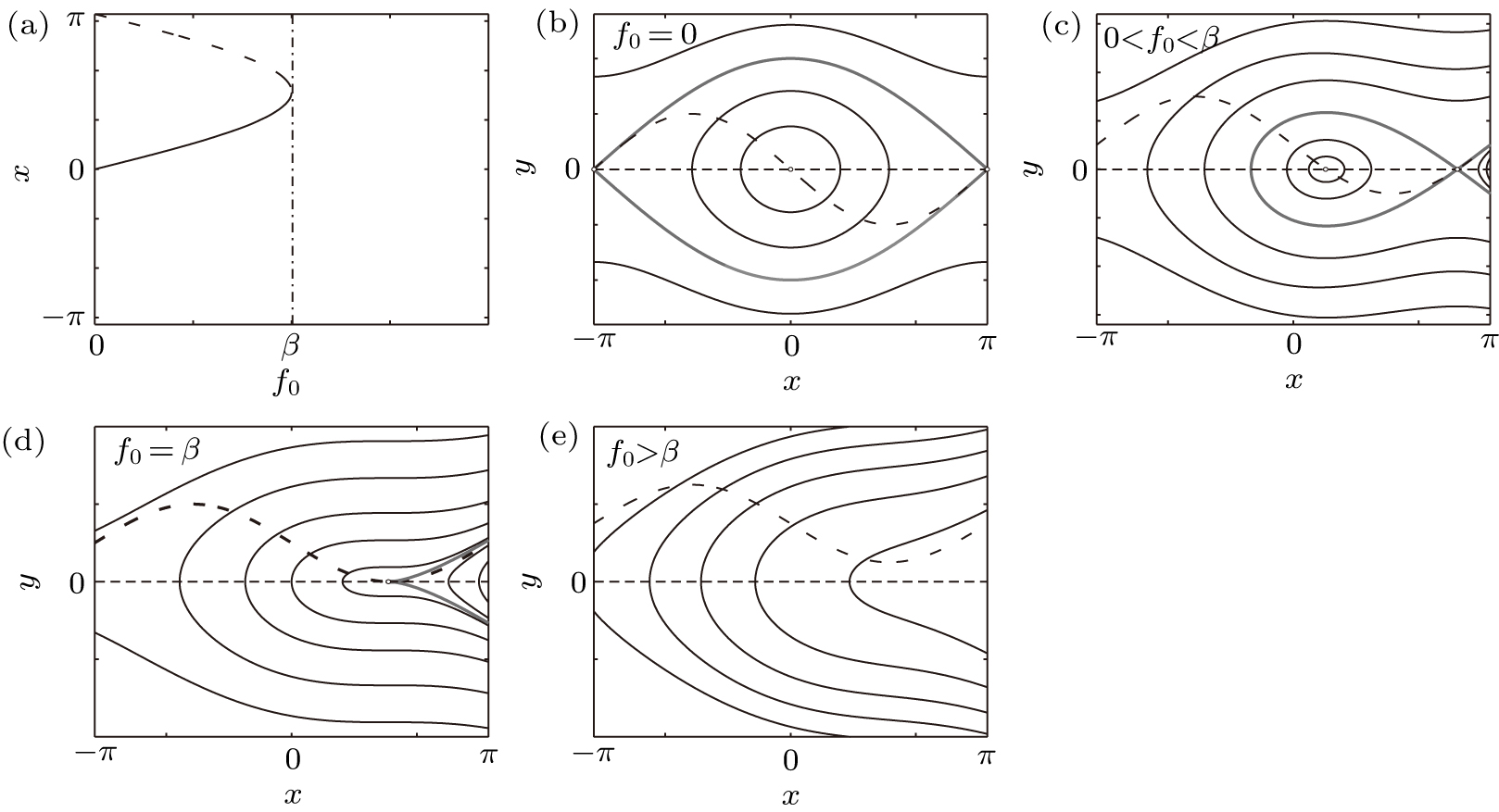
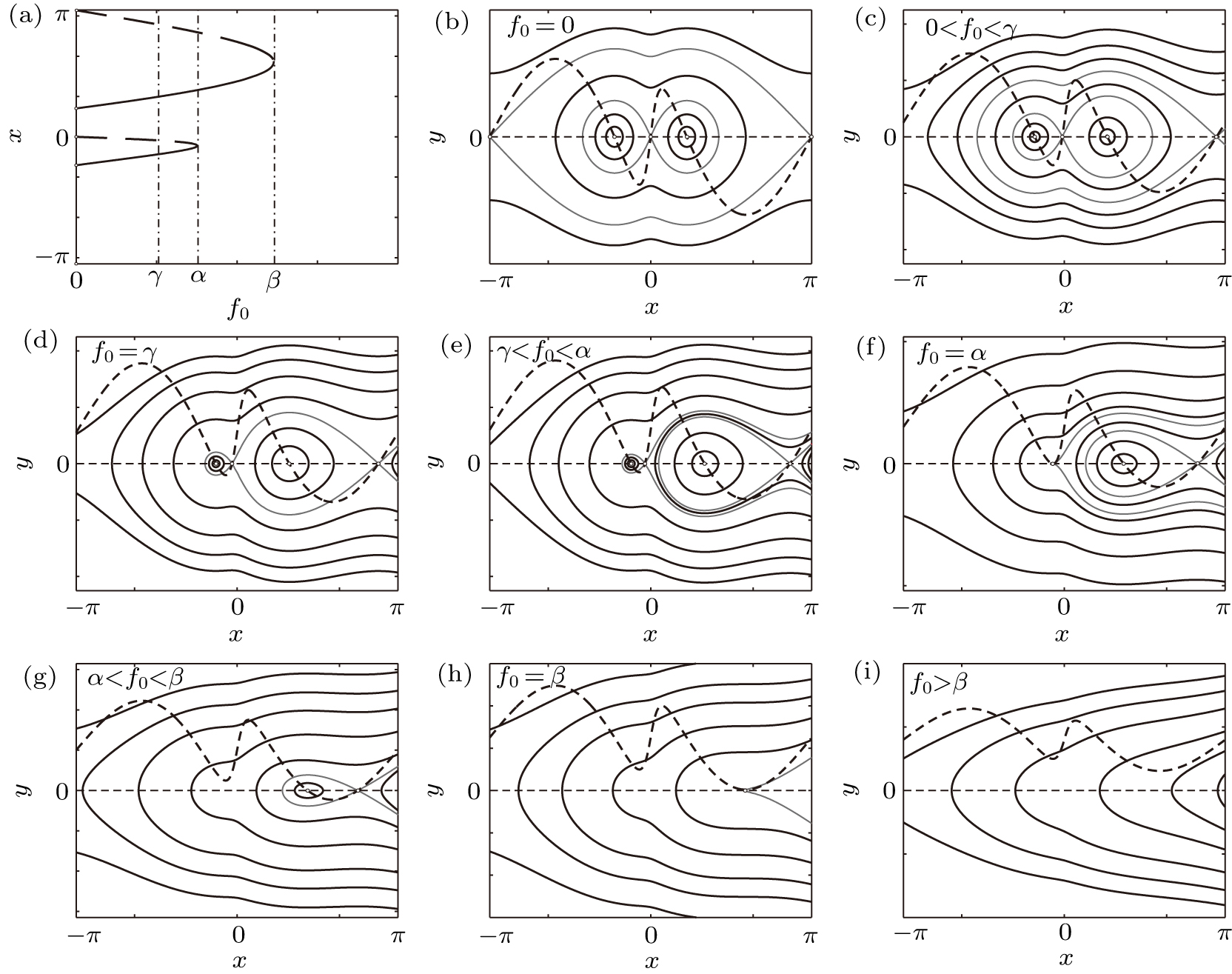
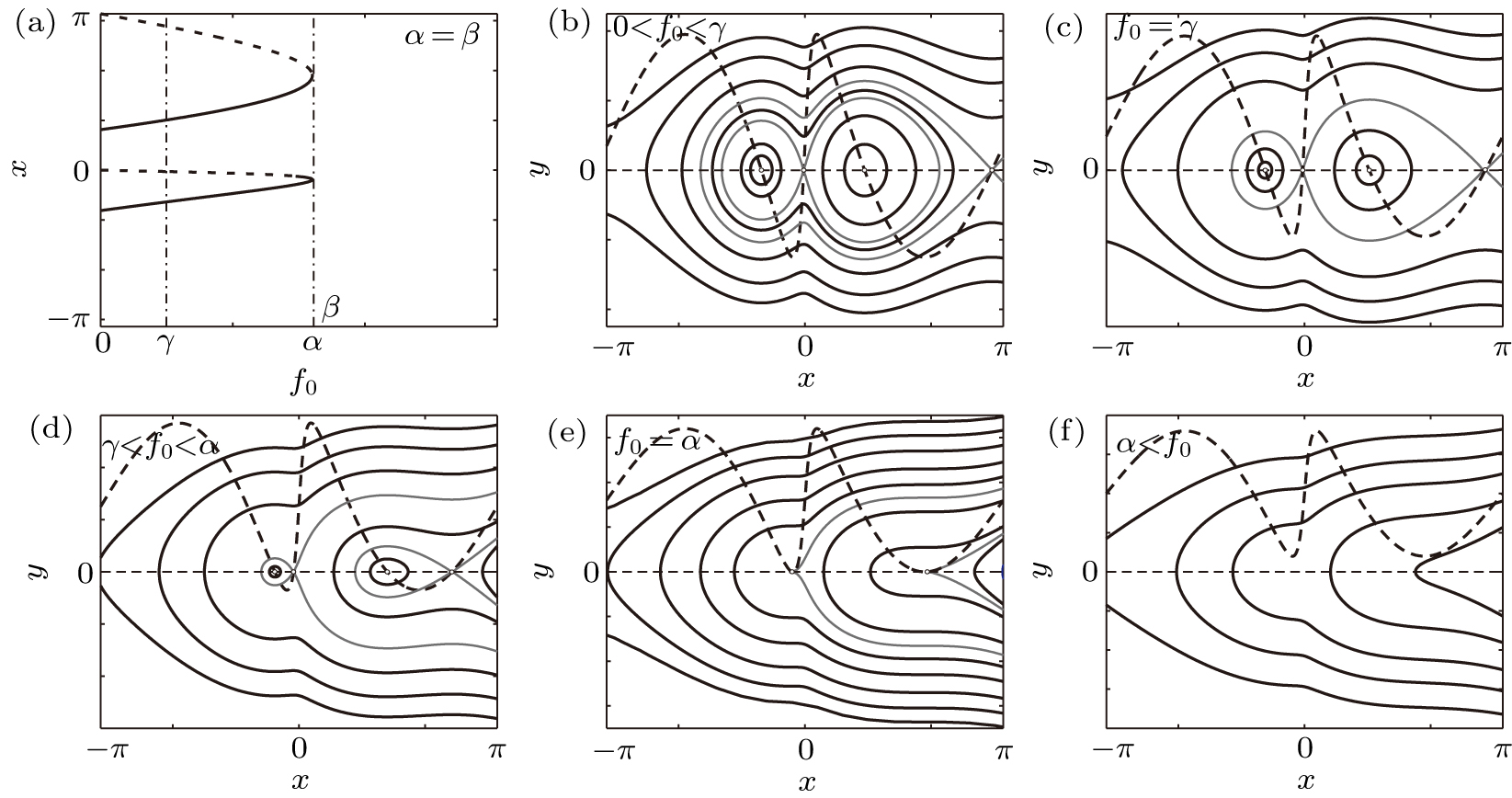
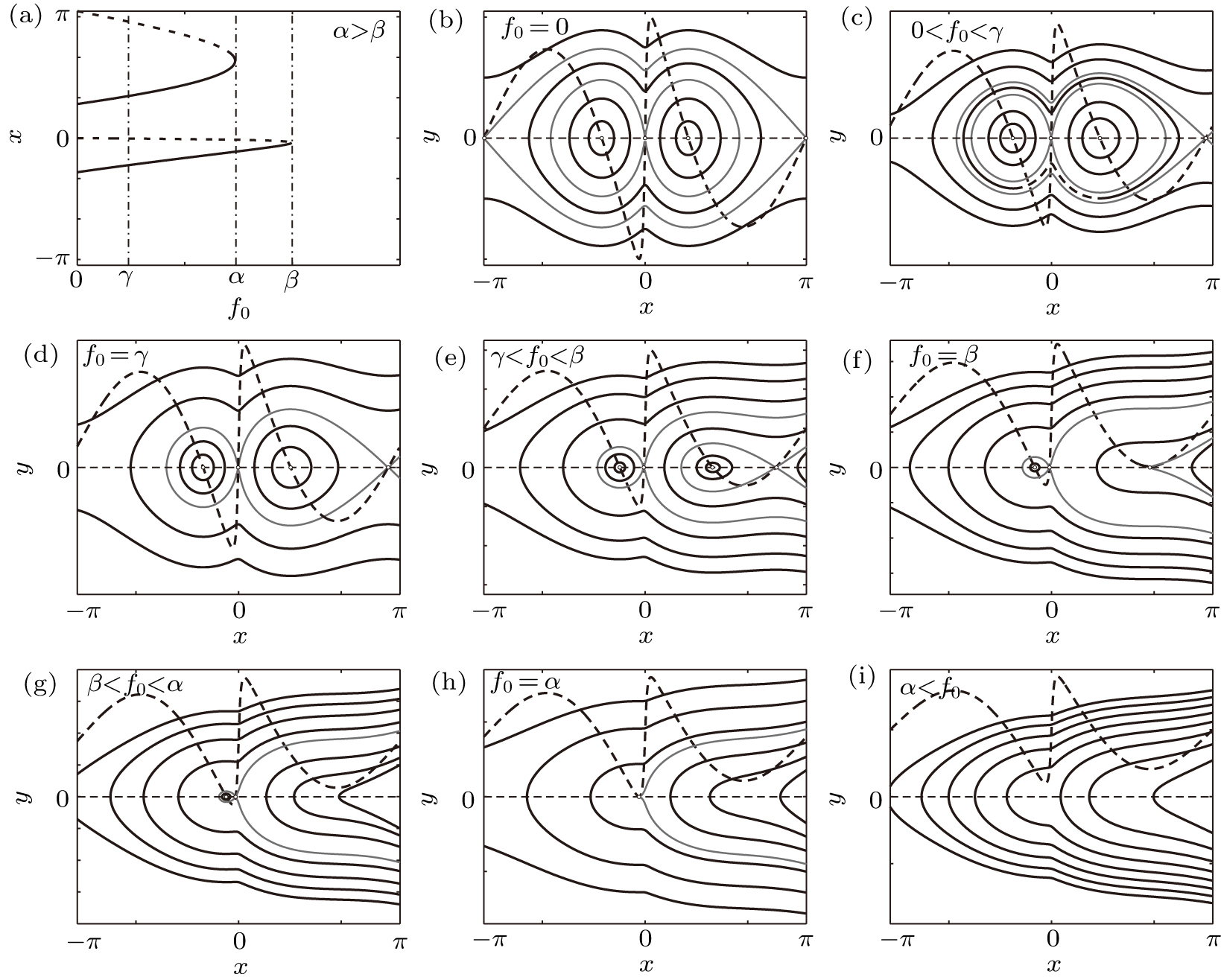
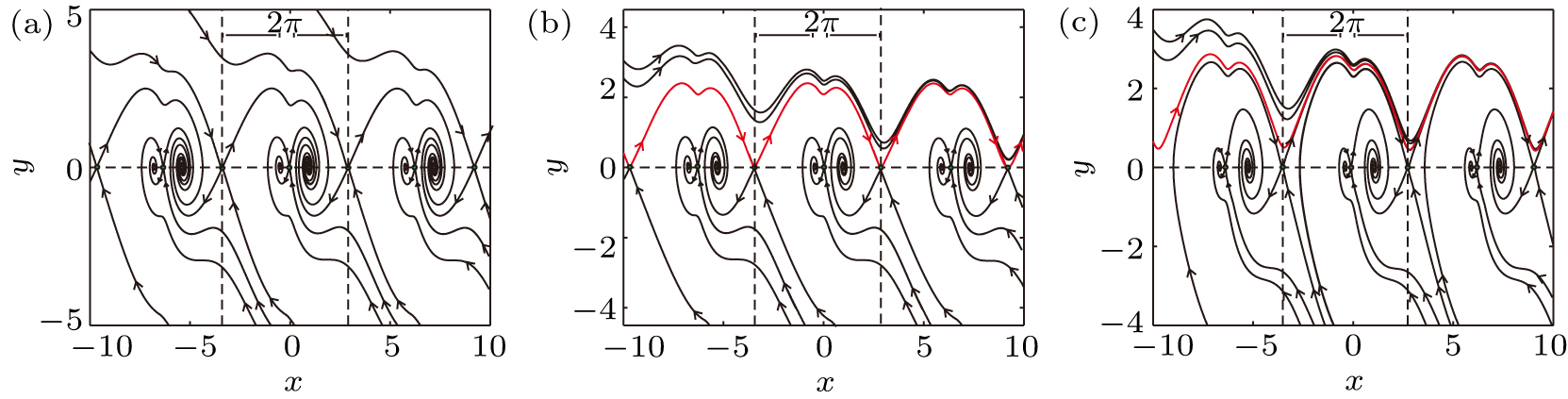
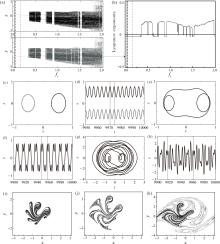
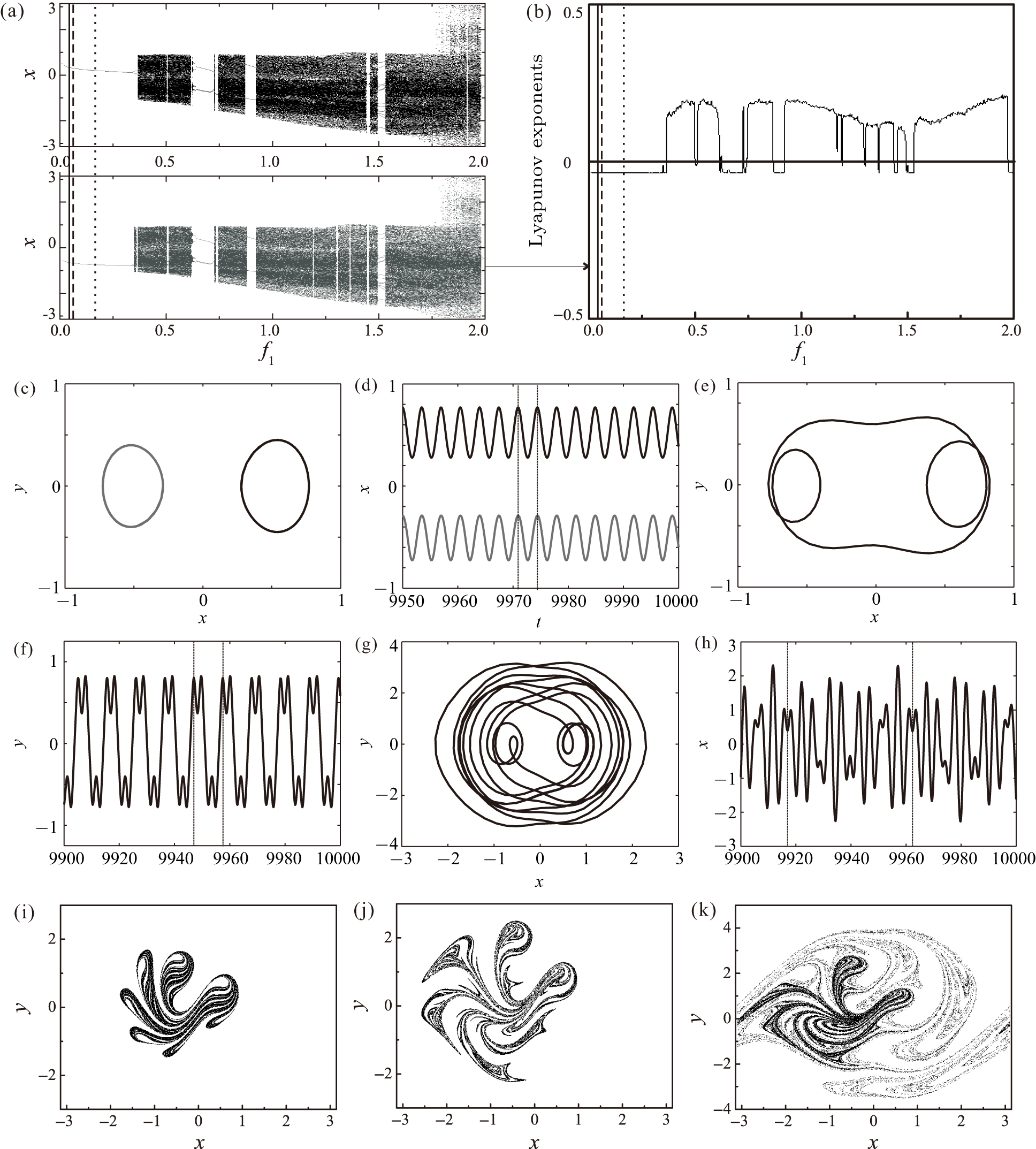
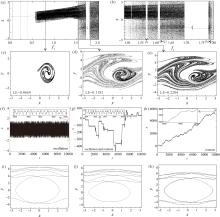
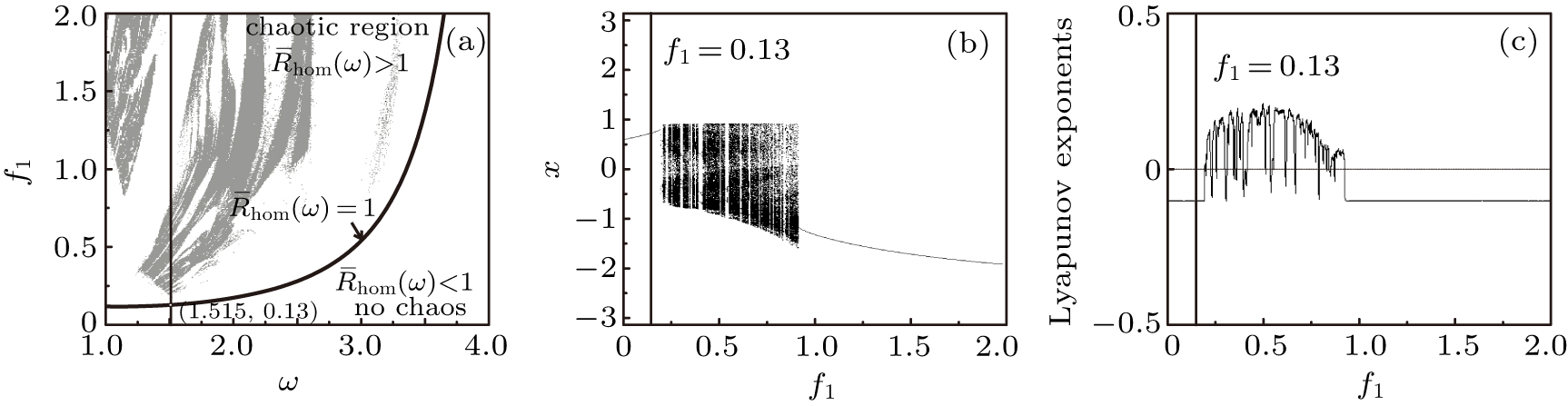
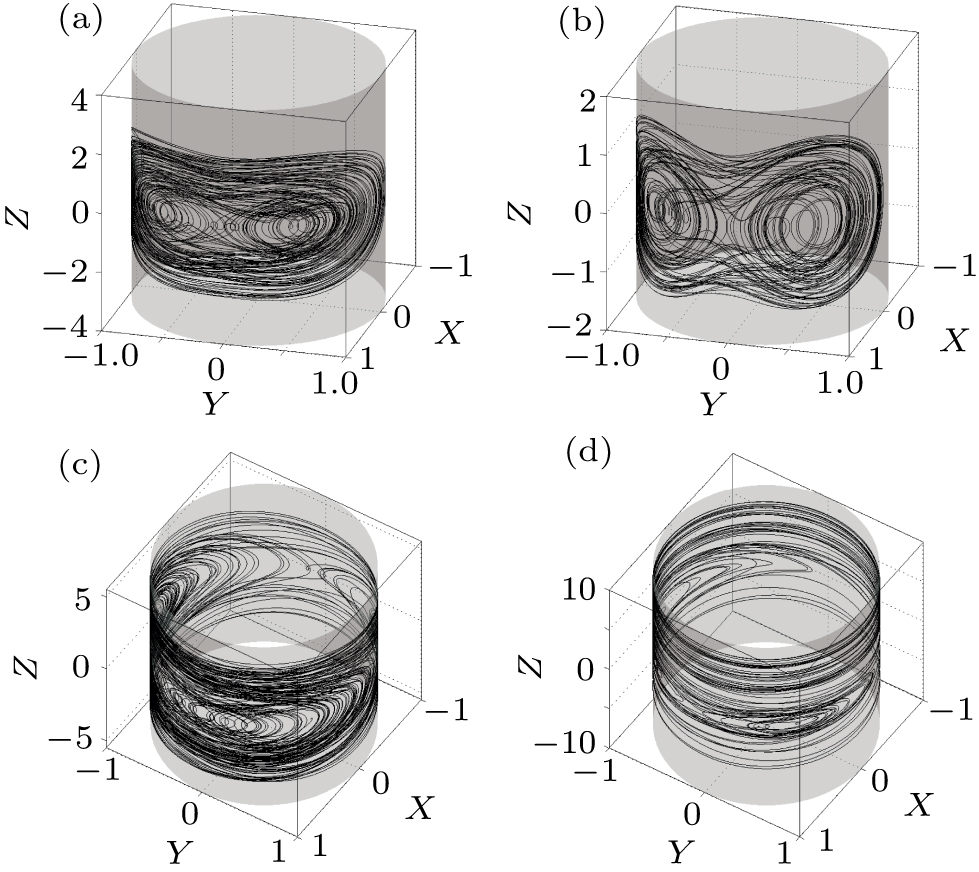
| [1] |
|
| [2] |
Matthews M R, Gauld C F, Stinner A 2005 The Pendulum: Scientific, Historical, Philosophical and Educational Perspectives Dordrecht Springer 139
|
| [3] |
|
| [4] |
|
| [5] |
|
| [6] |
|
| [7] |
|
| [8] |
|
| [9] |
|
| [10] |
|
| [11] |
|
| [12] |
|
| [13] |
|
| [14] |
|
| [15] |
|
| [16] |
|
| [17] |
|
| [18] |
|
| [19] |
|
| [20] |
|
| [21] |
|
| [22] |
|
| [23] |
|
| [24] |
|
| [25] |
|
| [26] |
|
| [27] |
Jia M M, Jiang H G, Li W J 2019 Acta Phys. Sin. 68 130503 in Chinese
|
| [28] |
Cao Q J, Han N, Tian R L 2011 Chin. Phys. Lett. 28 0605021
|
| [29] |
|
| [30] |
Han N, Cao Q J 2016 Commun. Nonlinear Sci. Numer. Simulat. 36 431
|
| [31] |
|
| [32] |
|
| [33] |
Hong L, Xu J 2004 Commun. Nonlinear Sci. Numer. Simulat. 9 313
|
| [34] |
|
| [35] |
|
| [36] |
|
| [37] |
|
| [38] |
Cao Q J, Wiercigroch M, Pavlovskaia E E, Grebogi C, Thompson J M T 2008 Philos. Trans. R. Soc. A 366 635 DOI: 10.1098/rsta.2007.2115 |
| [39] |
Guckenheimer J, Holmes P 1983 Nonlinear Oscillations, Dynamical Systems, and Bifurcations of Vector Fields New York Springer 184
|
| [40] |
Melnikov V K 1963 Trans. Moscow. Math. Soc. 12 1
|
| [41] |
Hu H Y 2000 Applied Nonlinear Dynamics Beijing Aviation Industry Press of China 38 in Chinese
|
| [42] |
Strogatz S H 2015 Nonlinear Dynamics and Chaos: With Applications to Physics, Biology, Chemistry, and Engineering Beijing China Machine Press 272
|
| [43] |
|
| [44] |
|
| [45] |
|
| [46] |
|
| [47] |
Albert Luo C J 2017 Resonance and Bifurcation to Chaos in Pendulum Beijing Higher Education Press of China 220
|
| [48] |
Liu Y Z, Chen L Q 2001 Nonlinear Vibrations Beijing Aviation Industry Press of China 286 in Chinese
|
| [49] |
Feng J Q 2018 Nonlinear Dynamics of a Typical Vibro-Impact System Beijing Science Press of China 95 in Chinese
|
 ), Pei-Pei Lu(鲁佩佩)1
), Pei-Pei Lu(鲁佩佩)1