† Corresponding author. E-mail:
Project supported by the National Natural Science Foundation of China (Grant No. 11664001).
The spin-1/2 Heisenberg chain coupled to a spin-S impurity moment with anti-periodic boundary condition is studied via the off-diagonal Bethe ansatz method. The twisted boundary breaks the U(1) symmetry of the system, which leads to that the spin ring with impurity can not be solved by the conventional Bethe ansatz methods. By combining the properties of the R-matrix, the transfer matrix, and the quantum determinant, we derive the T–Q relation and the corresponding Bethe ansatz equations. The residual magnetizations of the ground states and the impurity specific heat are investigated. It is found that the residual magnetizations in this model strongly depend on the constraint of the topological boundary condition, the inhomogeneity of the impurity comparing with the hosts could depress the impurity specific heat in the thermodynamic limit. This method can be expand to other integrable impurity models without U(1) symmetry.
Recently, considerable attention has been drawn by the theory of impurities in both the Kondo problem and the topological state, and many new developments have been reported.[1–6] We know that the impurities play an important role in the strongly correlated electron systems, and even a small amount of defects may change the properties of the electron systems.[7–10] Then it is very important to construct the integrable systems including the impurities. Many impurity models with free end boundary conditions have been considered previously. Frahm and Zvyagin studied the effects of a boundary magnetic field and an impurity spin coupled to an open spin chain by means of Bethe ansatz method.[11] Wang proposed an open spin-1/2 Heisenberg chain coupled with two impurity spins sited at the ends, and exactly solved this model for arbitrary impurity spin and arbitrary exchange constants between the bulk and the impurities using Bethe ansatz method.[12] The integrable impurity problem of the Heisenberg chain with periodic boundary condition was first considered by Andrei and Johannesson.[13] They studied the integrable case of a spin S > 1/2 embedded in a spin-1/2 Heisenberg chain. Subsequently, the problem was generalized to arbitrary spins by Lee and Schlottmann.[14] In contrast to the periodic boundary conditions in the integrable impurity models, the anti-periodic boundary conditions are especially interesting, which are tightly related to the recent study on the topological states of matter.[15,16]
Since the Yang and Baxter’s pioneering works,[17–19] the quantum Yang–Baxter equation, which defines the underlying algebraic structure, has become a cornerstone for constructing and solving the integrable models. The T–Q relation method and the algebraic Bethe ansatz method developed from the Yang–Baxter equation have become two very popular methods for dealing with the exact solutions of the known integrable models.[20] Although the algebraic Bethe ansatz method is a powerful tool exhibiting a rich mathematical structure, its implementation for models without U(1) symmetry still has an obstacle. The main obstacle applying the algebraic Bethe ansatz to these models lies in the absence of a trivial reference state. Significant progress has been made recently for the integrable models without U(1) symmetry. For example, a promising method for approaching such kind of problems is Sklyanin’s separation variables method which has been applied to some integrable models.[21,22] Another important advance was made by Cao et al.,[23–25] who proposed the off-diagonal Bethe ansatz method to approach the exact solutions of generic integrable models either with or without U(1) symmetry. The central step of this method lies in the construction of the T–Q ansatz with an extra off-diagonal term. For the integrable models with impurities, on one hand the twisted boundary breaks the U(1) symmetry of the system, on the other hand the introduction of the impurity adds the freedom degree of the system, which make it difficult to resolve the impurity model without U(1) symmetry. A systematic method is still absent to derive the Bethe ansatz equations for integrable impurity models without U(1) symmetry.
It is known that the behavior of a single magnetic impurity in the one-dimensional spin-1/2 Heisenberg model as well as the behavior of a single Kondo impurity in a three-dimensional free electron host is described by similar Bethe ansatz theories.[26] The spin-1/2 magnetic impurity manifests the total Kondo screening with Fermi liquids-like low temperature behavior of the magnetic susceptibility and specific heat.[27] The effects of impurity scattering on the surface of topological insulators have been investigated extensively.[28–30] A key issue is to determine the conditions for the stability of the current-carrying states at the edge of the sample,[31,32] as this is the feature that most directly impacts prospect for future applications in electronics and spintronics.[33] Therefore, it is worthwhile to investigate the effects of impurity when the impurity locates at the twisted boundary. In this paper, we consider the Heisenberg spin ring with a Möbius-like topological boundary condition coupled to a spin-S impurity. The Hamiltonian of the model is H = H0 + Himp, which was first solved by Andrei and Johannesson,[13]
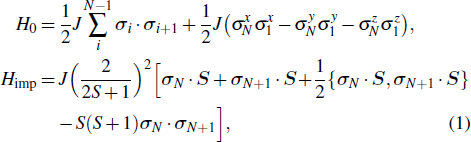
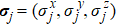
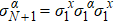
This paper is organized as follows. Section
Let V0, V1, …, VN be 2D vector spaces over complex numbers C. The V0 indicates the auxiliary space and the tensor product V1 ⊗ ⋯ ⊗ VN indicates the quantum space, where Vj corresponds to the j-th site of the spin chain with j = 1,2,…,N. On the tensor product V0⊗ Vj, we define the R-matrix
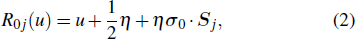
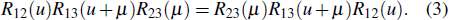
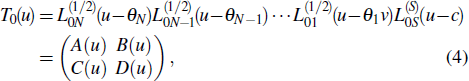
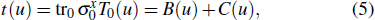
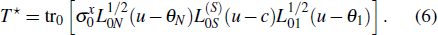
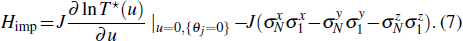
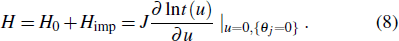
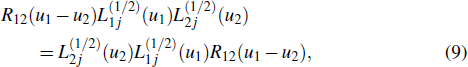
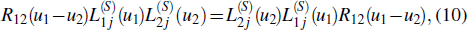
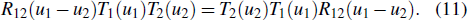
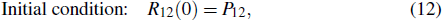
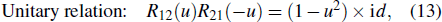
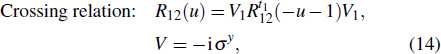
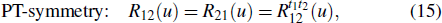
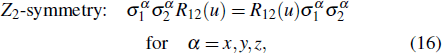
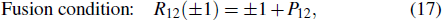
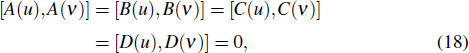
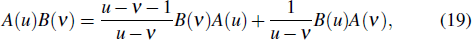
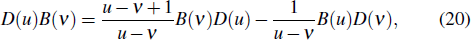
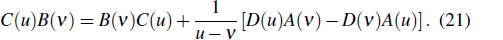
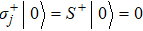
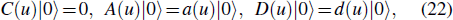
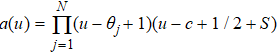

We suppose that |Ψ〉 is an eigenstate of t(u) and independent of u, and has t(u)|Ψ〉 = Λ(u)|Ψ〉. Base on the commutation relations Eqs. (
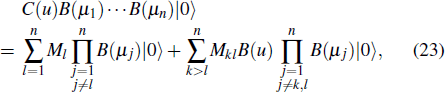
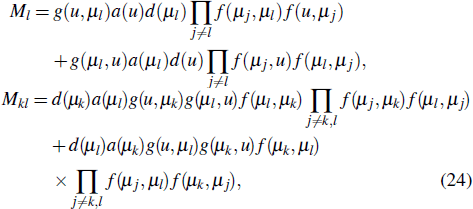
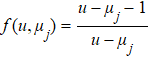
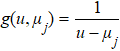
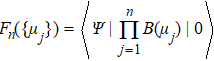
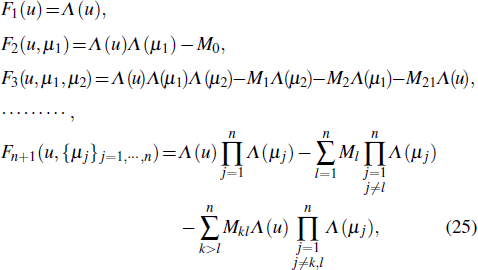
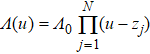
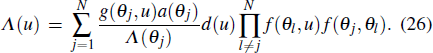
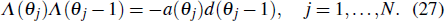
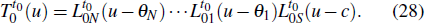
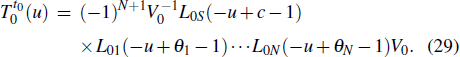
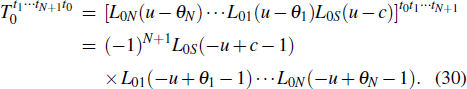
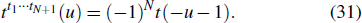
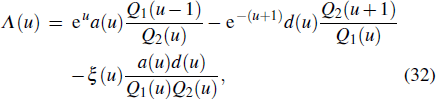
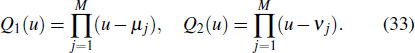
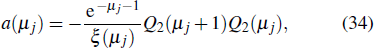
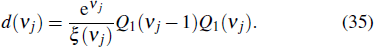
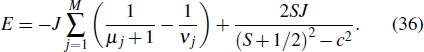
The interaction between the impurity and the spin ring depends on the parameter c, and c could be real or imaginary values. Therefore, we discuss the ground state properties for different c values with the standard method proposed by Yang and Yang.[17] Note that the distributions of the roots { μj } and { νj } are not independent, which essentially are decided by the selection rules of elementary excitation at the anti-periodic boundary condition of the system. In the homogeneous limits θj → 0, with the help of Eqs. (
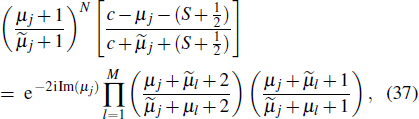
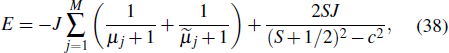
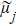
First, we consider the case of imaginary c = iζ. The exchange interaction between the impurity and the spin ring is always positive corresponding to antiferromagnetic coupling. All the modes of { μj} take imaginary values in the ground state to minimize the energy. We set μj = λj + iγj, and introduce the following function:
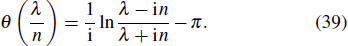
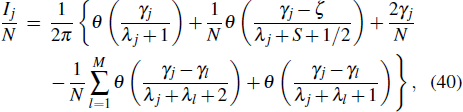
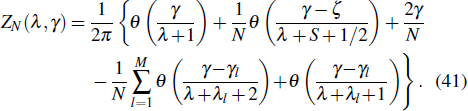
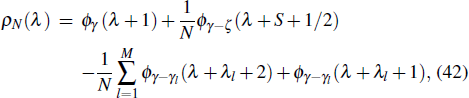
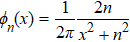
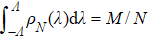
For the case of real value c, the coupling between the impurity and the spin ring can be ferromagnetic or antiferromagnetic for |c| < S + 1/2 and S+1/2 < |c|, respectively. Obviously, there exists a critical point |c| = S+1/2, which corresponds to a quantum phase transition to a non-Fermi liquid quantum critical region. When S + 1/2 < |c|, the real modes of μ at Re {μj} = c – (S + 1/2) can exist in the ground state. With a similar discuss, the self magnetization of the ground state is Mg = S, which means that the impurity can not be screened. When S + 1/2 > |c|, the exchange interaction between the impurity and the bulk is in the antiferromagnetic region, and no bound state can exist in the ground state. In this case, the residual magnetization of the ground state Mg = S–1/2, which means that the impurity moment is partially screened (for S > 1/2).
In order to investigate the impurity effects in the thermodynamic limit, we consider a special case of c = iζ and μj = iγj to investigate the thermodynamic of the present model. In the thermodynamic limit, in the leading order of O(N–1), equation (
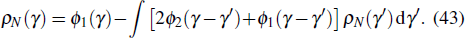
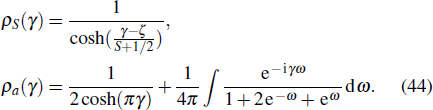
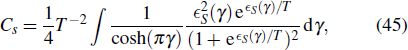
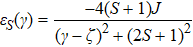
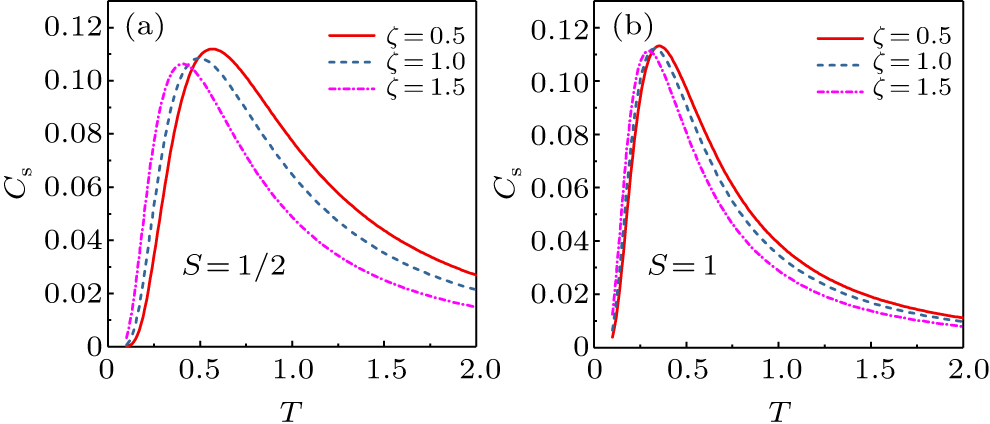 | Fig. 1. The impurity specific heat Cs as a function of temperature T for the system with the impurity spin-1/2 (a) and the impurity spin-1 (b), where the impurity parameter ζ = 0.5, 1, 1.5. |
From the above discussion, we conclude that the impurity can not be screened for the antiferromagnetic cases. The impurity moments only can be partially screened for the ferromagnetic case. The coupling interaction of the impurity is different to that in the one-dimensional quantum system with U(1) system.[12] The topological boundary condition will produce a finite boundary energy described by a phase factor, which constraints the interaction between the impurity and the spin kink. The screen behaviors of the impurity strongly depend on the competition between the quantum fluctuation and the constraint of the topological boundary condition. When the impurity parameter c is a pure imaginary value, the contribution of the impurity on the specific heat mainly depends on the impurity spin, and the effects of the anti-periodic boundary are depressed in the thermodynamic limit. From Fig.
The spin-1/2 Heisenberg chain described by the Hamiltonian (
| [1] | |
| [2] | |
| [3] | |
| [4] | |
| [5] | |
| [6] | |
| [7] | |
| [8] | |
| [9] | |
| [10] | |
| [11] | |
| [12] | |
| [13] | |
| [14] | |
| [15] | |
| [16] | |
| [17] | |
| [18] | |
| [19] | |
| [20] | |
| [21] | |
| [22] | |
| [23] | |
| [24] | |
| [25] | |
| [26] | |
| [27] | |
| [28] | |
| [29] | |
| [30] | |
| [31] | |
| [32] | |
| [33] | |
| [34] | |
| [35] | |
| [36] | |
| [37] | |
| [38] | |
| [39] |


