† Corresponding author. E-mail:
Project supported by the Ningbo Natural Science Foundation, China (Grant Nos. 2019A610151 and 2018A610081), the Natural Science Foundation of Zhejiang Province, China (Grant Nos. LY17A020001 and LY20A020002), the National Natural Science Foundation of China (Grant No. 11402063), and the K C Wong Magna Fund in Ningbo University, China.
A homogenization theory is developed to predict the influence of spherical inclusions on the effective thermoelectric properties of thermoelectric composite materials based on the general principles of thermodynamics and Mori–Tanaka method. The closed-form solutions of effective Seebeck coefficient, electric conductivity, heat conductivity, and figure of merit for such thermoelectric materials are obtained by solving the nonlinear coupled transport equations of electricity and heat. It is found that the effective figure of merit of thermoelectric material containing spherical inclusions can be higher than that of each constituent in the absence of size effect and interface effect. Some interesting examples of actual thermoelectric composites with spherical inclusions, such as insulated cavities, inclusions subjected to conductive electric and heat exchange and thermoelectric inclusions, are considered, and the numerical results lead to the conclusion that considerable enhancement of the effective figure of merit is achievable by introducing inclusions. In this paper, we provide a theoretical foundation for analytically and computationally treating the thermoelectric composites with more complicated inclusion structures, and thus pointing out a new route to their design and optimization.
Thermoelectric materials directly convert heat into electricity and vice versa due to the Seebeck effect and Peltier effect, and thus they are widely used for power generation in harvesting wasted heat, refrigeration, solar energy harvesting, and carbon reduction.[1–9] The thermoelectric devices have unique advantages: they are more compact, robust, and noiseless than the conventional mechanical providers of power generation and refrigeration because the thermoelectric devices are solid state heat engines and have no moving parts.[10–13] However, thermoelectric materials have a critical weakness of low conversion efficiency which is governed by a dimensionless figure of merit ZT = α2 σ T/k, where α, σ, and k are the Seebeck coefficient, electric conductivity, and heat conductivity, respectively, T being the absolute temperature.[3,14] Thermoelectric materials with high figure of merit at a temperature T should combine with a large Seebeck coefficient α to provide a large electric potential difference, a large electric conductivity σ to reduce the Joule heat loss, and a small heat conductivity k to maintain a large temperature gradient.[15] As a result, most efforts dedicating to improving the conversion efficient have involved alloying, doping, quantum, and other size effects in thin thermoelectric films and mesoscopic systems.[16–27]
Lots of papers have focused on improving the properties of thermoelectric materials by using size effect and interface effect, but very few researchers have examined the classical composite mixtures as a route to enhancing the thermoelectric characteristics. In such thermoelectric composites, the granularity and heterogeneity are on a large enough scale that the physical processes can be well described in each individual phase by the classical thermodynamic theory.[28] The problem of thermoelectric properties of a composite medium was studied by Bergman and Levy,[29] and they found that the effective figure of merit of a thermoelectric composite material was bounded by its constituents from the micromechanical analysis. However, this conclusion was drawn based on the assumption that both ασT and α2σT are material constants independent of temperature T, which linearizes the constitutive equations. In fact, both the effective figure of merit and the effective conversion efficiency of layered heterogeneous medium can be higher than all of its constituents, indicated by solving the rigorous nonlinearly coupled transport equations of electricity and heat.[30,31] The closed-form solutions of effective properties of a thermoelectric composite containing an elliptic inhomogeneity or an arbitrarily shaped hole were provided in Refs. [32,33]. A theoretical model to analyze the energy conversion efficiency of a cracked thermoelectric material with finite height and width based on the nonlinearly coupled transport equations of electricity and heat was developed by Zhang et al.[34] The three-dimensional ellipsoidal inclusion problem in thermoelectric materials and the effective material properties of the matrix–inclusion system were studied by Wang et al.[35] From Refs. [30,32–35], it is also found that the effective figure of merit can exceed that of each constituent. A continuum linear theory for thermoelectric materials was developed by Liu under the conditions of small variations of temperature, electric potential, and their gradients, and this linear theory is further used to predict effective properties of thermoelectric composites.[36] An asymptotic homogenization model for three-dimensional thermoelectric composites was proposed and applied to the finite element simulations by Yang et al.[37] Most of the above papers have paid attention to the two-dimensional problem, these studies motivate us to developing a rigorous homogenization theory based on the three-dimensional thermoelectric constitutive model which can predict the macroscopic behavior of thermoelectric composites and furnish the design strategies for desired effective properties.
In this paper, we develop a homogenization theory to analyze the effective properties of thermoelectric composites based on the Mori–Tanaka method.[38] The rest of the present paper is organized as follows. The general framework of homogenization for thermoelectric composites is provided in Section
The properties of thermoelectric materials can be described by considering an isotropic homogeneous body V ∈ Rn, where n = 2 or 3 is the dimension of space. The thermodynamic state of the body is described by the absolute temperature T and electrochemical potential μ. The usual suffix notation is used in this paper, a repeated suffix is summed over x, y, and z, and suffixes preceded by a comma denote differentiation. The absolute temperature T, electrochemical potential μ, electric current density ei, and heat flux density qi are related by[2]








It should be noted that the total electrochemical potential F in Eq. (

The general framework of homogenization for thermoelectric composites is developed based on the Mori–Tanaka method in this Subsection.[38] Consider a three-dimensional thermoelectric material containing uniformly distributed spherical inclusions. The geometrical and physical quantities with superscripts “m” and “s” refer to those for the thermoelectric matrix material and internal inclusions, respectively, and the full quantities without a superscript refer to those for the entire thermoelectric composite material. The total volume of the solid can be expressed as V = Vm + Vs, and the volume fraction of the inclusions to the thermoelectric composite material is defined as f = Vs/V. The overall quantities defined are based on a volume-averaged concept, therefore the average electrochemical potential 








The average quantities of the inclusions and thermoelectric matrix are related to those of the entire solid by volume fraction rule, that is




If the thermoelectric transport process in the inclusions and thermoelectric material matrix follows Eqs. (








For a thermoelectric material with internal inclusions subject to homogeneous boundary conditions, in general, the electric current density and energy flux density inside the inclusions can be expressed as


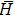
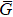




The effective electric resistivity 





As a result, the electric conductivity 



It should be noted that 



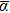


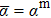
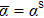
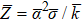
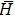
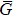
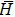
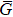
The local concentration factors 



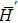
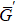
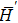
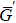
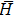
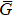


The practical use of the homogenization theory for the thermoelectric composites is illustrated by considering the case of internal spherical inclusions (see Fig. 



The incoming electric current density e0 and energy flux density u0 in a three-dimensional thermoelectric material are assumed to be disturbed by spherical thermoelectric inclusions with an average radius a, and the applied e0 and u0 are aligned to be in the z′ direction (Fig.











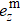
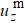











Referring to Eqs. (

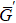



From Eqs. (




The influence of the type of spherical inclusion on the effective properties of thermoelectric composite material will be studied in this section. The following dimensionless parameters such as α0 = αs/αm, σ0 = σs/σm, k0 = ks/km are introduced for the convenience of discussion.
The electric and heat properties of cavities filled with air or vacuum are always neglected in the theoretical analysis because air is a very good insulator. Therefore α0, σ0, and k0 are all equal to zero, and the effective thermoelectric properties from Eq. (




From Eq. (


The dependence of the effective properties of thermoelectric material containing insulated spherical cavities on f is plotted in Fig. 
The conductive electric and heat transfer across the spherical inclusion are considered to examine the influence of inclusions on the effective thermoelectric properties. For this case, α0 = 0, σ0 and k0 are no longer equal to zero, and thus the effective Seebeck coefficient, electric conductivity, heat conductivity, and figure of merit are given as




Figures 

 | Fig. 4. Effective properties of thermoelectric material containing spherical-inclusions (k0 = 0.5): (a) Seebeck coefficient    |
The thermoelectric effect of spherical inclusions will be considered based on the analysis in Subsection


From Eq. (
It seems that the figure of merit of two-phase thermoelectric composite is bounded from above by the larger one of the constituent phases. However, what we calculate is the effective figure of merit of two groups of Bi2Te3-based thermoelectric composites, the inclusion phases are Bi0.5Sb1.5Te3 and BiSbTe, respectively. The material constants used in the calculation are listed in Table 
| Table 1. Material properties of thermoelectric materials.[42] . |
Finally, we point out that it is very difficult to analytically determine the optimized relationship between f and (α0, σ0, k0) under the condition of 
In this paper, we proposed a micro-mechanical model to estimate the effective properties of the thermoelectric material containing spherical inclusions based on the Mori–Tanaka method. The general framework of homogenization for thermoelectric composites is developed by following the thermodynamic principle. Solutions of a spherical thermoelectric inclusion embedded in a thermoelectric material matrix subjected to uniform electric current density and energy flux density are derived, and the closed-form expressions of effective Seebeck coefficient, electric conductivity, heat conductivity, and figure of merit are obtained. Numerical results show that the effective properties are significantly degraded when the spherical cavities are produced inside the thermoelectric material. The effective figure of merit of thermoelectric composites can be improved by appropriately introducing (thermoelectric) inclusions, and it can be higher than that of each constituent.
| [1] | |
| [2] | |
| [3] | |
| [4] | |
| [5] | |
| [6] | |
| [7] | |
| [8] | |
| [9] | |
| [10] | |
| [11] | |
| [12] | |
| [13] | |
| [14] | |
| [15] | |
| [16] | |
| [17] | |
| [18] | |
| [19] | |
| [20] | |
| [21] | |
| [22] | |
| [23] | |
| [24] | |
| [25] | |
| [26] | |
| [27] | |
| [28] | |
| [29] | |
| [30] | |
| [31] | |
| [32] | |
| [33] | |
| [34] | |
| [35] | |
| [36] | |
| [37] | |
| [38] | |
| [39] | |
| [40] | |
| [41] | |
| [42] |



























