† Corresponding author. E-mail:
Project supported by the Science Fund for Creative Research Groups of the National Natural Science Foundation of China (Grant No. 51621062) and the National Natural Science Foundation of China (Grant No. 51802144).
Silicone rubber is widely used as a kind of thermal interface material (TIM) in electronic devices. However few studies have been carried out on the thermal conductivity mechanism of silicone rubber. This paper investigates the thermal conductivity mechanism by non-equilibrium molecular dynamics (NEMD) in three aspects: chain length, morphology, and temperature. It is found that the effect of chain length on thermal conductivity varies with morphologies. In crystalline state where the chains are aligned, the thermal conductivity increases apparently with the length of the silicone-oxygen chain, the thermal conductivity of 79 nm-long crystalline silicone rubber could reach 1.49 W/(m⋅K). The thermal conductivity of amorphous silicone rubber is less affected by the chain length. The temperature dependence of thermal conductivity of silicone rubbers with different morphologies is trivial. The phonon density of states (DOS) is calculated and analyzed. The results indicate that crystalline silicone rubber with aligned orientation has more low frequency phonons, longer phonon MFP, and shorter conducting path, which contribute to a larger thermal conductivity.
Highly integrated circuits inevitably lead to high heat flux, which is a bottleneck of Moore’s law. If the heat cannot be released in time, the temperature of the electronic components will rise sharply, leading to short working life, poor performance, as well as unguaranteed stability and reliability. According to statistic data, thermal invalidation is the main failure form of electronic equipments, more than half of electronic equipment failures are caused by poor cooling systems.[1] The invalidation rate of some electronic components conforms to the 10 °C law, i.e., for every 10 °C rise in temperature, the failure rate doubles.[2] Electronic components are extremely sensitive to temperature and high temperature is extremely unfavorable to electronic devices, so it is necessary to cool the electronic devices.
A reasonable layout can reduce the temperature of chips under the circumstances of low heat flux. However when the heat flux is high, an effective heat transfer technology needs to be introduced to cool the electronic devices. The heat flow transfers to the package shell first and then is took away by air or other refrigerants.[3] Materials of the packaging shell selected should be of high thermal conductivity to reduce the internal thermal resistance, such as copper and aluminum. Due to the air gap between heat dissipation devices and packaging shells, thermal interface materials (TIMs) are needed to improve the heat dissipation through the gap. TIMs are usually insulating materials. Polymer materials in shims are most often used as TIMs due to their softness and insulation. Among polymer shim materials, silicone rubber stands out for its relative higher thermal conductivity and good elasticity. Most of conventional TIMs on market are made of silicone oil and silicone rubber.[4] However, the thermal conductivity of these insulating polymer materials is usually on the order of 10−1 –100 W/(m⋅K).[4] Therefore, it is necessary to further improve the thermal conductivity of silicone rubber to improve the heat dissipation and meet the increasing requirements of electronic devices. Un-doped silicone rubber has low thermal conductivity of only 0.2 W/(m⋅K). The composites of silicone rubber are popularly applied, whose thermal conductivity can be increased by an order of magnitude.[5–8] Previous reports on the improvement of their thermal conductivity have shown that the processing method, formulas, sizes, and surface treatments of the doped particles contribute to the thermal conductivity. Higher amount of filler[5,9,10] and moderately larger particle size[6,7,11–13] are beneficial for higher thermal conductivity. Different types of fillers[9,14–16] and different manufacturing procedures[9,17,18] have a great influence on the thermal conductivity. However, the reported researches on improving the thermal conductivity of silicone rubber are mainly on the doped silicone rubber, few researches are about the structure of the un-doped silicone rubber,[18] which is important too.
The main structure factors affecting the thermal conductivity of the un-doped silicone rubber include the silicone chain orientation and relative molecular mass. In this paper, non-equilibrium molecular dynamics (NEMD) simulation is used to study these factors. At present, there are few studies on the thermal conductivity of silicone rubber by using molecular dynamics simulation (MDS). Previous studies carried out by Luo et al.[19] (2011) investigated the thermal conductivity of single-chain, double-chain, and crystalline silicone-oxygen chain. However, there exist some queries in the paper that are worthy of further discussion. Firstly, the effective cross-section area used was not appropriate. According to the definition,[20] the effective cross-section area of a single chain should be 54 Å2, not 27 Å2. Fourier’s law tells us that if the cross-sectional area is underestimated, the calculated thermal conductivity will be greater than its actual value. Secondly, the density quoted by the authors was 0.92 g/cm3 that was different from the original document[21] of 0.97 g/cm3. If the density is underestimated, the number of atoms in the same volume decreases, the heat capacity per unit volume will decrease, and the thermal conductivity thus obtained will be lower than its actual value (detailed discussion in Subsection
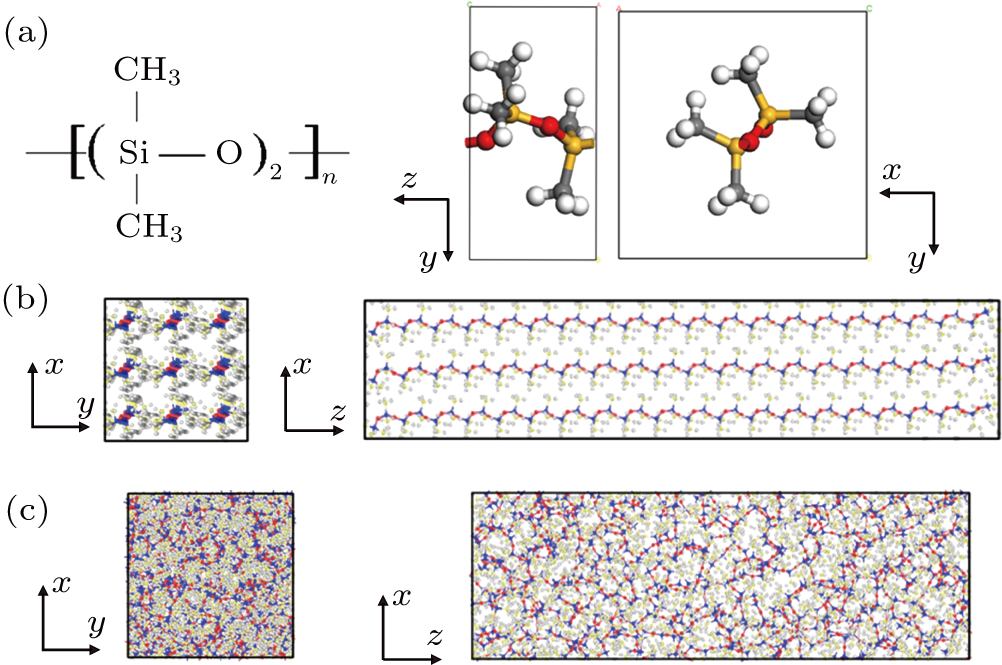
Considering the instability and the very limited application scenarios of a single-chain in practical applications, as well as inspired by the works of Cao et al.[22] and Xu et al.,[23] we investigate the crystalline silicone rubber. A crystalline silicone rubber model with the same density as experiment is constructed, and then the thermal conductivity is calculated by NEMD simulation to study the effect of the chain length. Amorphous silicone rubber is also calculated and compared with crystalline silicone rubber to demonstrate the effect of orientation on the thermal conductivity.
MDS can be roughly divided into two types,[24] equilibrium molecular dynamics (EMD) and NEMD. For NEMD, it is essential to set up a steady temperature gradient in the system, then Fourier’s law is used to obtain the thermal conductivity by calculating heat flow and temperature gradient. There are two kinds of commonly used NEMD methods.[25] One is to establish a temperature distribution and then calculate the heat flux, such as the dual-thermostat method.[26] The other is to apply a heat flux to get a temperature distribution, which is called inverse NEMD. A typical one is the Müller–Plathe method.[27,28]
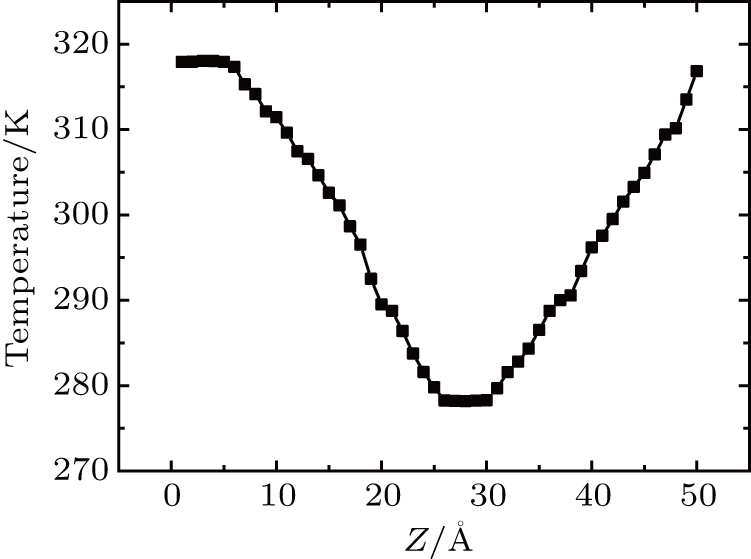
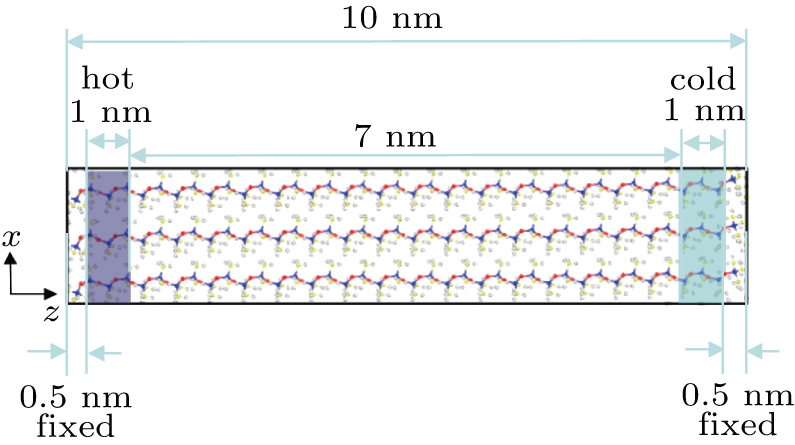
In this paper, the dual-thermostat NEMD method[29,30] is adopted to calculate the thermal conductivity of silicone rubber. The boundary condition is the thermal bath boundary, so that one end of the model is at high temperature and the other end is at low temperature, and the two ends are named as hot and cold regions, respectively. The energy entering the hot region and the energy leaving the cold region are averaged to obtain the heat flux. The system is divided into 40–50 slices. There are more slices when the length of the simulation system is longer. The temperature of each slice along the heat flux direction is calculated and plotted to obtain the temperature gradient. Then Fourier’s law is used to get the thermal conductivity


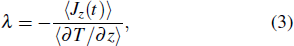
Firstly, a silicone-oxygen monomer [–(Si(CH3)2–O)2–] (as shown in Fig.
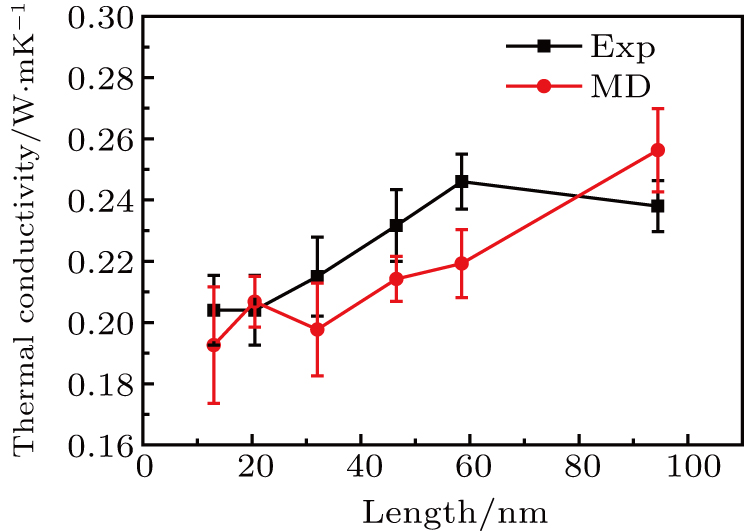
Since ideal crystalline state is difficult to prepare in experiment, and the thermal conductivity data of amorphous silicone rubber in literature[21] lacks corresponding relative molecular mass, a direct comparison is made between the calculation value and the experimental value of the amorphous silicone rubber that had exact relative molecular mass. It is difficult to directly determine the length of the molecular chain of the polymer. Its length is calculated according to the relative molecular mass of the polymer, the mass of a monomer and its length. The silicone oil used in the experiment is from Dow Corning Company as shown in Table
| Table 1. Relationship between viscosity and relative molecular mass. . |
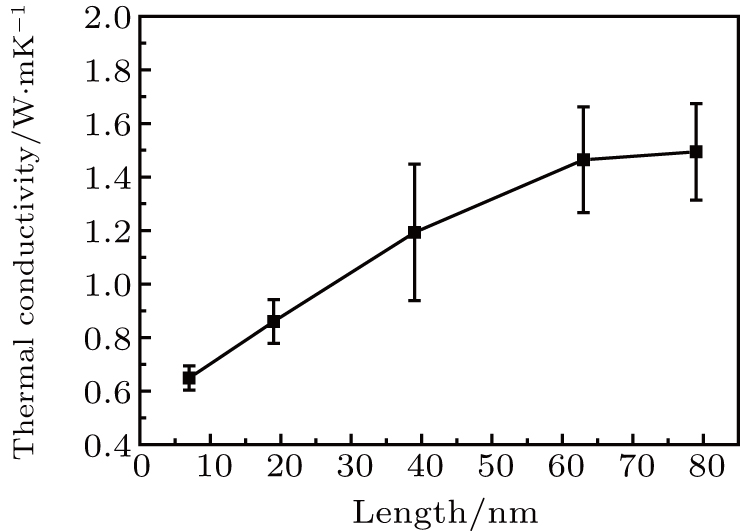
The crystalline silicone rubbers with lengths of 10 nm, 25 nm, 50 nm, 80 nm, and 100 nm in heat flux direction are simulated. The length includes that used to fix the atom to form an adiabatic wall and the length used to control the temperature of the cold and hot regions. The remaining is the length of the temperature gradient, as shown in Fig.
It is clear that the thermal conductivity of the crystalline structure with aligned chains increases with the chain length, but whether there is a similar phenomenon in amorphous state is not known. The latter is investigated by combining experimental measurement and MD simulation. Since the sample to be measured is liquid, a TIM thermal performance measurement instrument (LW-9389, Longwin, Taiwan, China) equipped with a container for liquid testing is used to measure the thermal conductivity of the samples. The container is a plastic one specially designed to prevent heat exchange between sample and surroundings. There is a square hole in the middle of the container in which the sample is filled and directly contacts with the hot and cold meter bars. The scheme of the experiment device to measure the thermal conductivity is shown in Fig. S1 (detailed information in the
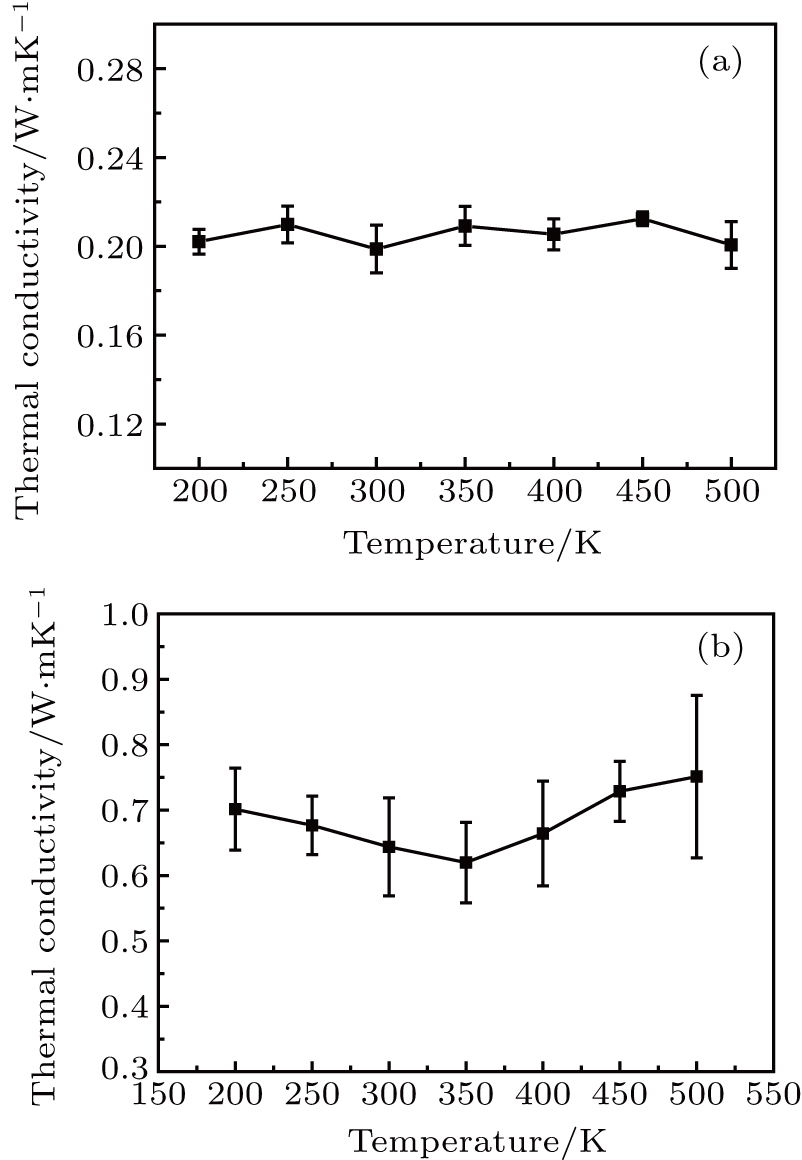
Temperature is also thought to affect the thermal conductivity. Currently, there are few studies on the effect of temperature on thermal conductivity of amorphous silicone rubber. In this paper, the thermal conductivity of silicone rubber both in amorphous state and crystalline state is calculated at different temperatures. The results, as shown in Fig.
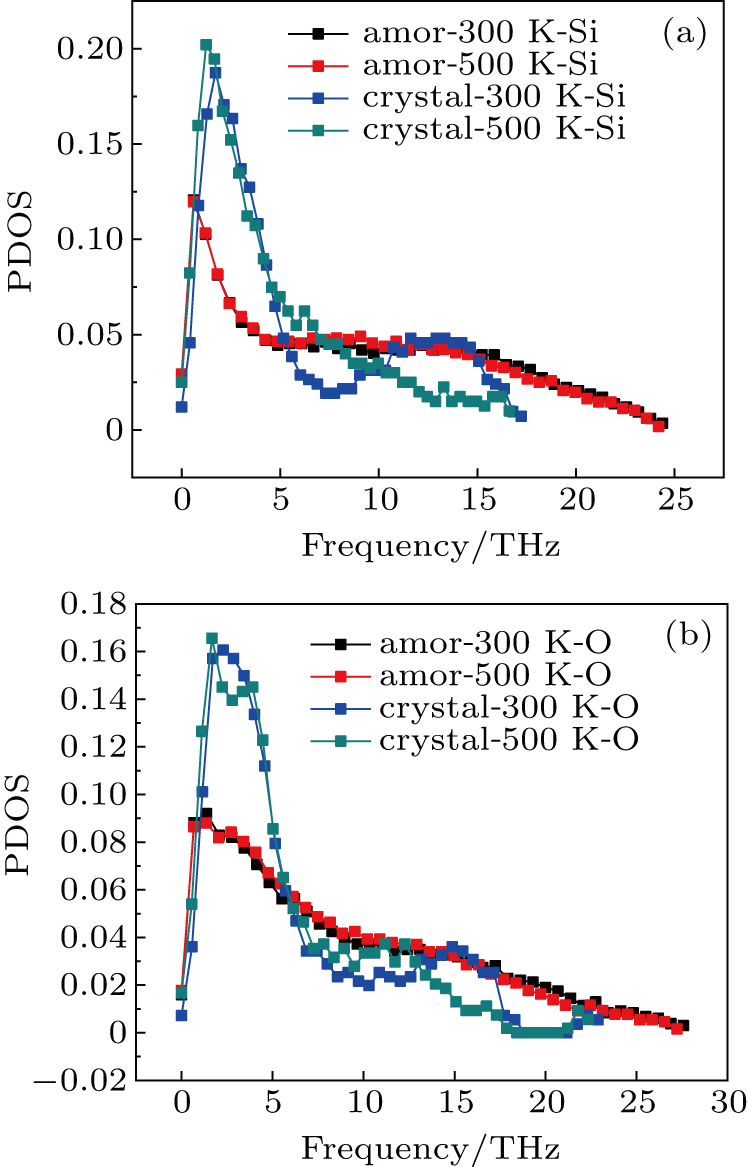
In this sub-section, information from MDS is used to calculate the phonon DOS. The conventionally used method is the Fourier transform of the velocity auto-correlation function.[47,48] Another method is based on the vibration scattering phonon theory[49,50] that uses the displacement information in MDS to obtain the Green’s function in the inverse space through Fourier transform.[51] The phonon DOS is obtained by calculating the eigenvalues of the dynamic matrix. The phonon DOSs for crystalline and amorphous silicone rubbers are obtained by the method of phonon information based on the vibration scattering theory, and the results are shown in Fig.
It can be seen from Fig.
By comparing Fig.
NEMD method is used to study the effect of chain length on the thermal conductivity of crystalline silicone rubber and amorphous silicone rubber. It is found that there is an apparent increase in thermal conductivity of crystalline state with the chain length, but the chain length has less effect on thermal conductivity in amorphous state, which indicates that morphology plays an important role in thermal transport. In addition, the thermal conductivities of both amorphous state and crystalline state at different temperatures are calculated. It is observed that in the temperature range from 200 K to 500 K, the temperature has almost no effect on the thermal conductivity. The crystalline state of silicone rubber has larger MFP and shorter conducting path due to its orientation. Therefore, its thermal conductivity is higher than that of amorphous state.
| [1] | |
| [2] | |
| [3] | |
| [4] | |
| [5] | |
| [6] | |
| [7] | |
| [8] | |
| [9] | |
| [10] | |
| [11] | |
| [12] | |
| [13] | |
| [14] | |
| [15] | |
| [16] | |
| [17] | |
| [18] | |
| [19] | |
| [20] | |
| [21] | |
| [22] | |
| [23] | |
| [24] | |
| [25] | |
| [26] | |
| [27] | |
| [28] | |
| [29] | |
| [30] | |
| [31] | |
| [32] | |
| [33] | |
| [34] | |
| [35] | |
| [36] | |
| [37] | |
| [38] | |
| [39] | |
| [40] | |
| [41] | |
| [42] | |
| [43] | |
| [44] | |
| [45] | |
| [46] | |
| [47] | |
| [48] | |
| [49] | |
| [50] | |
| [51] | |
| [52] | |
| [53] |