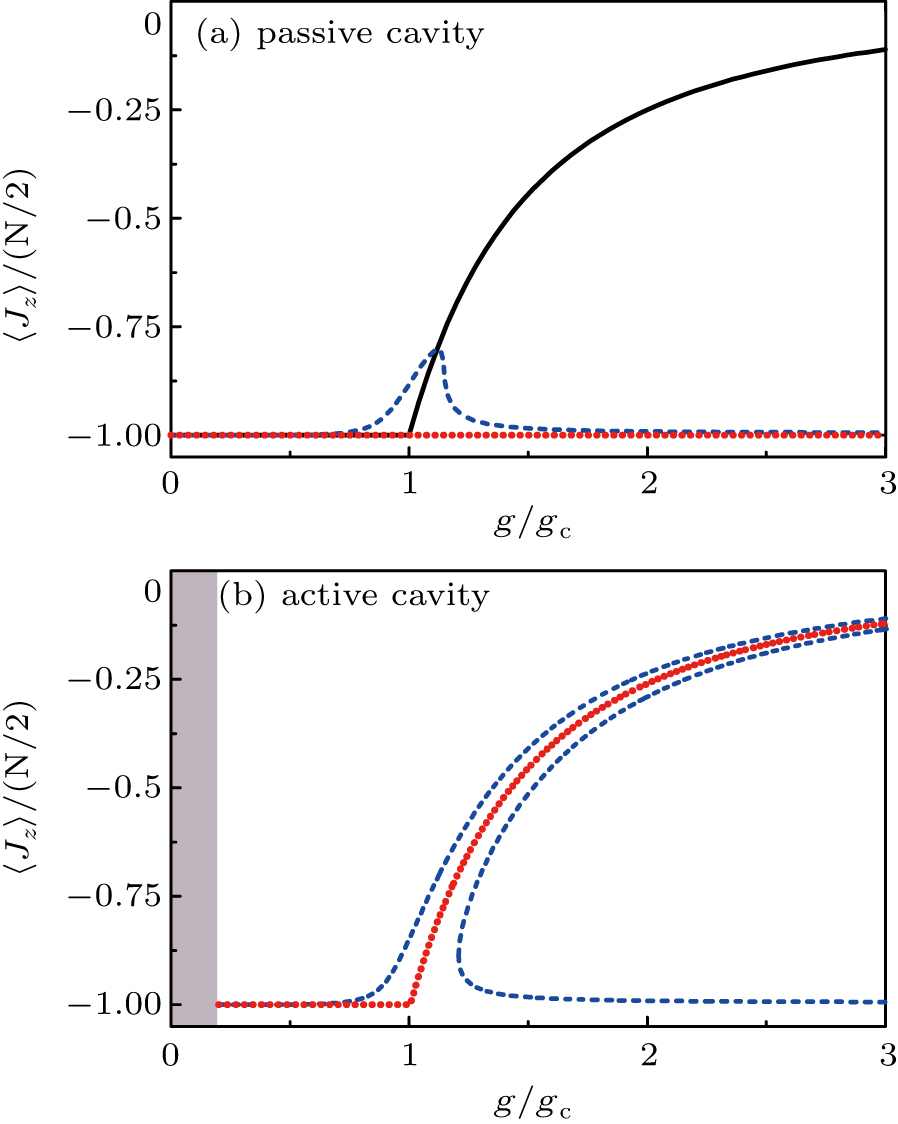
Numerical results of ⟨Jz⟩/(N/2) versus the coupling strength g/gc for different amplitudes
Ωd/N
of the drive field. (a) ⟨Jz⟩/(N/2) versus g/gc without the gain, calculated using Eq. (8). Here κc = γ = 0 and
Ωd/N→0
for the (black) solid curve; κc/γ = 0.5 and
Ωd/N→0
for the (red) dotted curve; and κc/γ = 0.5 and
(Ωd/N)/γ=0.5
for the (blue) dashed curve. (b) ⟨Jz⟩/(N/2) versus g/gc with the gain, calculated using Eq. (11). There is no ⟨Jz⟩/(N/2) when g/gc < 0.2 (grey region) because
g4(1−2χ)2−4γ2Δc2<0
in this region [cf. Eq. (13)]. The gain rate κg in Eq. (13) is determined with
Ωd/N→0
and the same value of κg is used for the two curves. Here
Ωd/N→0
for the (red) dotted curve and
(Ωd/N)/γ=0.5
for the (blue) dashed curve. In both (a) and (b), we choose Δc = Δs and g/γ = 10. |