† Corresponding author. E-mail:
Due to the unique magnetic, mechanical and thermal properties, magnetic nanoparticles (MNPs) have comprehensive applications as the contrast and therapeutic agents in biomedical imaging and magnetic hyperthermia. The linear and nonlinear magnetoacoustic responses determined by the magnetic properties of MNPs have attracted more and more attention in biomedical engineering. By considering the relaxation time of MNPs, we derive the formulae of second harmonic magnetoacoustic responses (2H-MARs) for a cylindrical MNP solution model based on the mechanical oscillations of MNPs in magnetoacoustic tomography with magnetic induction (MAT-MI). It is proved that only the second harmonic magnetoacoustic oscillations can be generated by MNPs under an alternating magnetic excitation. The acoustic pressure of the 2H-MAR is proportional to the square of the magnetic field intensity and exhibits a linear increase with the concentration of MNPs. Numerical simulations of the 2H-MAR are confirmed by the experimental measurements for various magnetic field intensities and solution concentrations using a laser vibrometer. The favorable results demonstrate the feasibility of the harmonic measurements without the fundamental interference of the electromagnetic excitation, and suggest a new harmonic imaging strategy of MAT-MI for MNPs with enhanced spatial resolution and improved signal-to-noise ratio in biomedical applications.
Nanoparticles with specific biological properties have been applied to imaging macrophages[1] in human diseases, and in-vivo imaging clinical tools are expected to define treatment options and to monitor responses to therapy. Characterized by the quick magnetic response,[2] unique magnetic property,[3] good biological compatibility,[4] low remanence and coercive force, and remarkable specific distribution in a magnetic field, magnetic nanoparticles (MNPs)[5–7] have been widely used as the contrast and therapeutic agents in clinical imaging and magnetic hyperthermia by coating MNPs with appropriate tumor/tissue specific markers[8, 9] bounded to the tumor region. Besides the electromagnetic and thermal effects, a great deal of attention has been focused on the magnetoacoustic responses (MARs) of MNPs based on the magnetically induced acoustic oscillations of particles in biomedical tissues. By employing the interaction between the eddy current and the static magnetic field, the new imaging methodology of magnetoacoustic tomography with magnetic induction (MAT-MI)[10–14] was developed to reconstruct the conductivity distribution inside conductive tissues using the Lorentz force induced magnetoacoustic signals. Then, a new imaging technology of MAT-MI incorporating MNPs was proposed by Hu et al.[15] and a clear tissue boundary image for the tissue phantom containing superparamagnetic nanoparticles was achieved, demonstrating the feasibility of MNP imaging. By introducing MNPs to MAT-MI under an alternating magnetic excitation,[16] numerical simulations of the electromagnetic and acoustic fields proved that the acoustic sources of MNPs generated by the magnetic forces are much higher than those produced by the Lorentz forces in biological tissues, facilitating potential applications in drug tracing and imaging. A transducer was employed to collect magnetoacoustic signals at the identical frequency of the short-pulse magnetic excitation[17] in MAT-MI. With the impulse response consideration of the transducer, the distribution of MNPs was reconstructed using the MARs collected around the model at the fundamental frequency.
The thermodynamics of electromagnetic energy absorption[18–22] of MNPs was often employed for magnetic hyperthermia in past decades. Kellnberger et al.[23] proposed the concept of magnetoacoustic sensing for MNPs and demonstrated the existence of the second harmonics generated in response to a continuous electromagnetic excitation based on the energy absorption of MNPs. The second harmonic magnetoacoustic responses (2H-MARs) were confirmed by acoustic measurements using a fiber-interferometer detector. Carrey et al.[24] derived the expressions of the magnetic force and the vibration velocity of a single MNP based on the calculation of the dissipated power. The behavior of the second harmonic acoustic oscillations was described by analyzing the magnetic force, the magnetic moment, and the magnetic gradient undergone by an MNP during the four quadrants of the simplified rectangular magnetic hysteresis loop. It was reported that the second harmonic mechanical oscillations happened only when the relaxation time of particles was longer than the period of the alternating magnetic field. However, the energy dissipation based magnetoacoustic responses with a long-time continuous magnetic excitation cannot be applied to achieve an acceptable spatial resolution for image reconstruction in MAT-MI. With a short-time alternating magnetic excitation, the behavior of MNPs based on the mechanical vibrations and the corresponding 2H-MAR of an MNP distribution model exhibit great significance in further studies of the monitoring and imaging of MNPs.
In this paper, embarking from the relaxation time of MNPs, the mechanism of the 2H-MAR under an alternating magnetic excitation is investigated based on the mechanical vibrations of particles. The particle velocity of a single MNP with a general complex AC magnetic susceptibility is solved using the differential motion equation by considering the exerted magnetic force. The formulae of the 2H-MAR for a cylindrical MNP solution model is derived based on the principle of MAT-MI. The second harmonic mechanical oscillations of MNPs are demonstrated by the theoretical results of the magnetic force and the corresponding particle velocity. Numerical simulations are verified by the experimental measurements of magnetoacoustic vibrations on the model surface using a laser vibrometer. The acoustic pressure of the 2H-MAR is proved to be proportional to the square of the excitation current (magnetic intensity) with a linear increase with respect to the concentration of the MNP solution. The favorable results demonstrate the feasibility of high-precision second harmonic acoustic measurements without the electromagnetic interference produced by the solenoid, and suggest a new harmonic imaging strategy of MAT-MI for MNPs with enhanced spatial resolution and improved signal-to-noise ratio in biomedical engineering.
As shown in Fig. 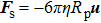




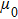
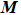
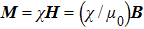
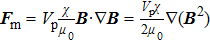
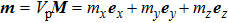
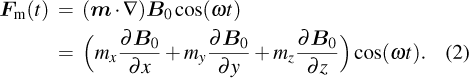


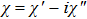
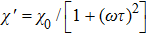
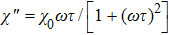

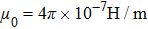
 | Fig. 1. Schematic diagram of the 2H-MAR measurement system with the inset of magnetic force analysis for a single MNP. |
Thus, in order to investigate the motion characteristics of the MNP in the alternating magnetic field 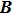

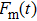
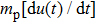

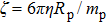
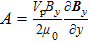

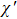

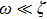

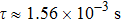
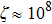


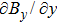
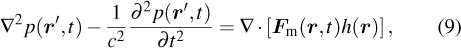
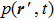
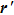
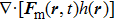

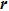


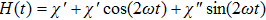
Based on the theory of MAT-MI, the acoustic pressure detected at 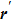

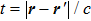
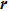
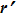
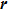
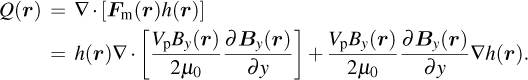
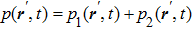
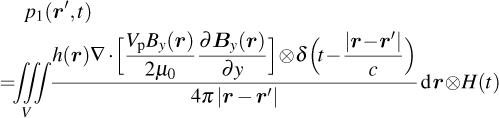
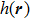

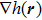
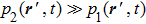
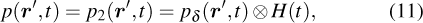

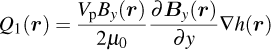

To establish an alternating magnetic field with a high intensity, a solenoid was fabricated with the turns, length and diameter of 300, 40 mm and 20 mm, respectively. By measuring the inductance (1.2 mH) and the resistance (4.7 Ω) of the solenoid using an impedance analyzer (Agilent 4294 A, Agilent Technologies, USA), a resonant circuit at the frequency of 4.86 kHz was designed, which satisfies the frequency requirements for Eq. (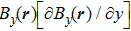
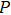
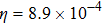
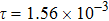


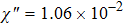
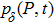


By integrating all the sources inside the entire MNP solution model, the impulse responses produced by the boundary and inner acoustic sources are illustrated in Fig. 
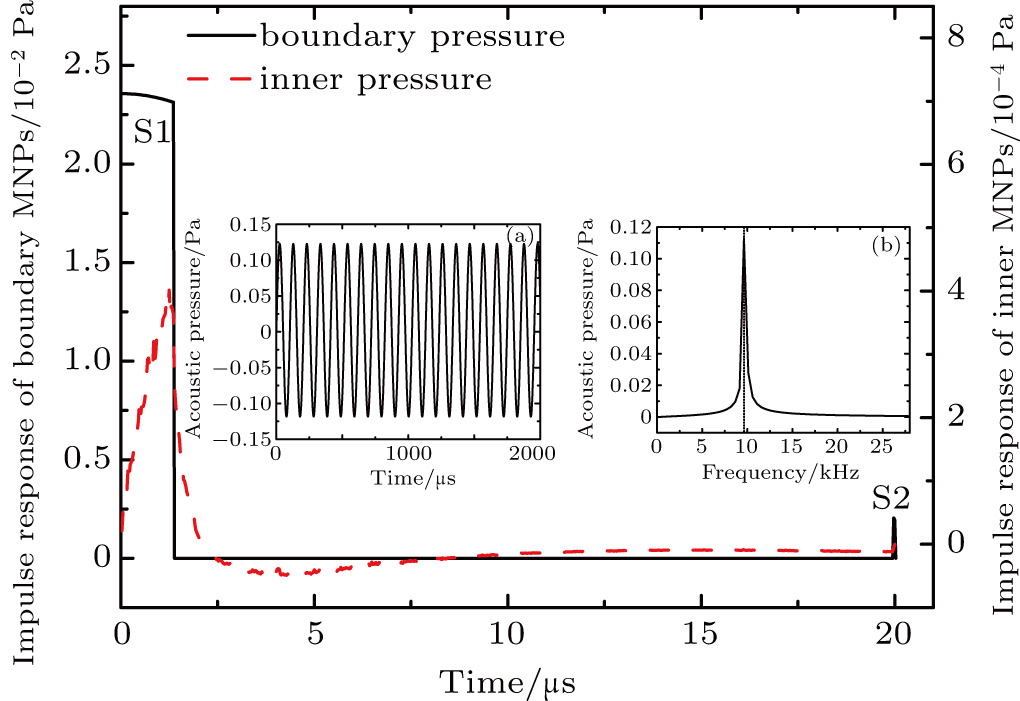 | Fig. 2. Impulse responses of the boundary and inner MNPs with the insets of (a) the 2H-MAR and (b) the corresponding spectrum under a sinusoidal magnetic excitation. |
In order to optimize the 2H-MAR of the system, pressure dependences of the magnetic field intensity and the concentration of MNPs on 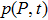
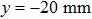

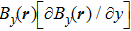
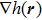
 | Fig. 3. Acoustic pressure distributions of the 2H-MAR with respect to (a) the excitation current and (b) the concentration of the cylindrical MNP solution model. |
As shown in Fig. 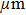
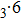
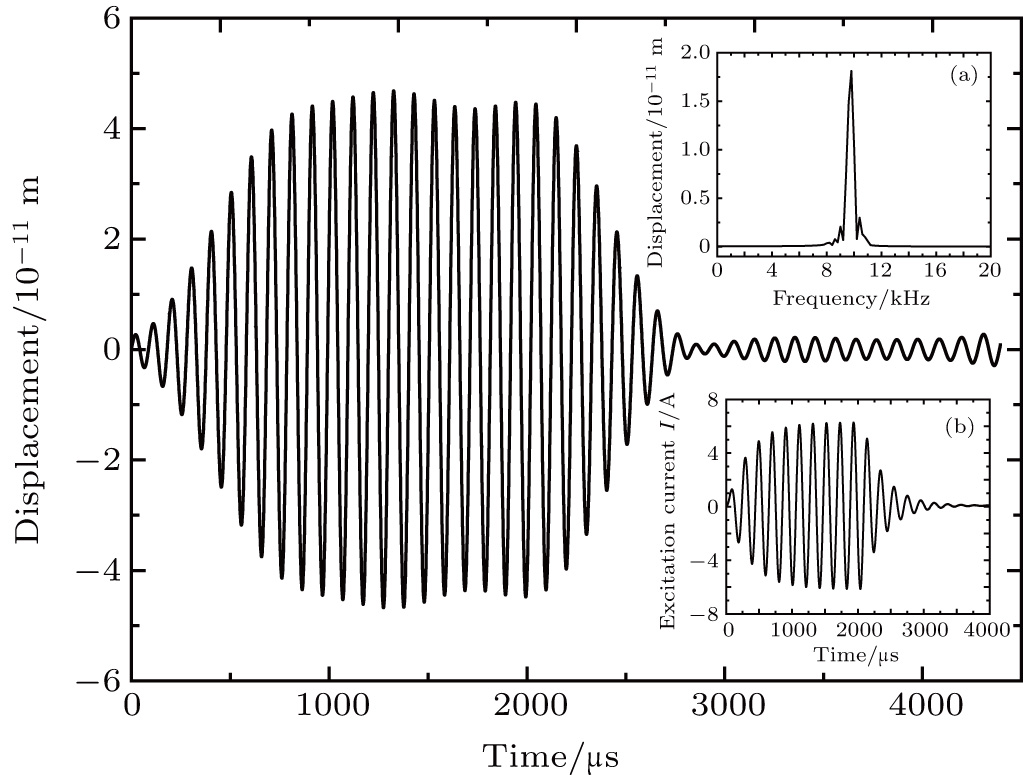
The experimental vibration displacement of the model with the concentration of 30 mg/ml at I = 6 A is illustrated in Fig. 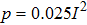

In the previous study of Kellnberger et al.,[23] the internal energy of MNPs evoked by a continuous electromagnetic excitation was derived to describe the power dissipation, and the appearance of the second harmonic magnetoacoustic resonance was theoretically demonstrated. However, except for the energy absorption of medium magnetization in the period of an alternating magnetic field, the 2H-MAR of the MNP solution model in terms of the shape and size was not taken into account, which might introduce significant influences on magnetoacoustic measurement and image reconstruction with the long-time continuous alternating magnetic excitation in MAT-MI. By considering the second harmonic mechanical oscillations and the integral effects of all MNPs with a short-time pulsed high-frequency magnetic excitation in our study, the structure characteristics of the solution model can be extracted from the 2H-MAR to reconstruct the distribution of MNPs. Meanwhile, the acoustic pressure of the 2H-MAR is much higher than that produced by a single MNP, which is easy to be verified by experimental measurements.
For the MAT-MI studies carried out by Hu and He[15] and Mariappan et al.,[17] magnetoacoustic signals generated by superparamagnetic iron oxide nanoparticles were collected around the phantom by a transducer at the fundamental frequency (0.5 MHz) of the short-time pulsed magnetic excitation, which might be caused by the experimental factors stated in the following. The relaxation time of the superparamagnetic nanoparticles was much smaller than the period of the alternating magnetic field, only mechanical vibrations at the fundamental frequency of the electromagnetic excitation could be produced by the magnetizations of MNPs. Meanwhile, due to the narrow bandwidth of the transducer (TRS ceramic, peak frequency 0.5 MHz, bandwidth 60%),[17] only the fundamental component around 0.5 MHz could be detected for image reconstruction. Nevertheless, the pilot investigations on MAT-MI with MNPs can still provide a solid experimental basis for the harmonic imaging of MAT-MI based on the 2H-MAR of MNPs.
The 2H-MAR of MNPs is demonstrated by theoretical simulations and experimental measurements, and only the second harmonic mechanical oscillations are detected by the laser vibrometer in our study. However, due to the linear and nonlinear electromagnetic interference of the coil, the 2H-MAR of MNPs may be influenced by the mechanical vibrations at the fundamental and the second harmonic frequencies. In order to reduce the interference between the electromagnetic excitation and the magnetoacoustic measurements in practical applications, an accurate sinusoidal excitation should be applied to minimize the second-harmonic electromagnetic excitation and a narrow bandwidth transducer with the central frequency at the second-harmonic frequency should be used to detect the 2H-MAR of MNPs. In addition, the systems of the electromagnetic excitation and the magnetoacoustic measurement should be fixed on two damping platforms to block the mutual interference of mechanical vibrations.
In conclusion, embarking from the relaxation time of MNPs, the mechanism of magnetoacoustic oscillations under an alternating magnetic excitation is investigated based on mechanical effects. The 2H-MAR of a cylindrical MNP solution model is derived using the principle of MAT-MI. Numerical simulations and experimental measurements of magnetoacoustic vibration using a laser vibrometer prove that only the second harmonic magnetoacoustic vibrations of MNPs can be generated by the exerted magnetic force due to the interaction of the alternating magnetic field intensity and the corresponding intensity gradient. The acoustic pressure of the 2H-MAR is proportional to the square of the magnetic field intensity and exhibits a linear increase with the concentration of the MNP solution. The favorable results demonstrate the feasibility of magnetoacoustic measurements at the second harmonic frequency without the fundamental interference of the electromagnetic excitation, and suggest a new harmonic imaging strategy of MAT-MI for MNPs with enhanced spatial resolution and improved signal-to-noise ratio in biomedical applications.
| [1] | |
| [2] | |
| [3] | |
| [4] | |
| [5] | |
| [6] | |
| [7] | |
| [8] | |
| [9] | |
| [10] | |
| [11] | |
| [12] | |
| [13] | |
| [14] | |
| [15] | |
| [16] | |
| [17] | |
| [18] | |
| [19] | |
| [20] | |
| [21] | |
| [22] | |
| [23] | |
| [24] | |
| [25] | |
| [26] | |
| [27] | |
| [28] | |
| [29] | |
| [30] |