† Corresponding author. E-mail:
Project supported by the National Natural Science Foundation of China (Grant No. 11975089), the Program for National Defense Science and Technology Innovation Special Zone of China, and the Program for Young Top-Notch Talents of Hebei Province of China.
Directional motion of dust particles in a dusty plasma ratchet is observed experimentally. The dusty plasma ratchet consists of two concentric gears with asymmetric sawtooth. It is found that the sawtooth number affects the directional motion of dust particles along the saw channel. With the increase of the sawtooth number, the particle velocity increases firstly and then decreases, and there is an optimum number of the sawtooth which could induce fast rotation of dust particles. The velocities of dust particles change as they are flowing along the saw channel. We also explore the force acting on the dust particle experimentally.
Rectifying motion in random environments is a long standing problem. In the absence of any net macroscopic forces, asymmetric potentials can be used to induce a particle flow when subjected to external fluctuations.[1] Referred to as Brownian ratchet, its study has flourished as one of the most active and diverse fields such as physics,[2–5] chemistry,[6,7] and biology.[8,9] Symmetry breaking mechanisms are demanded for operation of Brownian ratchet. Three possibilities inducing the symmetry-breaking have been proposed:[1] (1) unbiased driving force, (2) asymmetric ratchet potential, and (3) collective effect. Experimental explores to these possibilities have been performed in several experiments, such as tunneling ratchet in a semiconductor nanostructure[10] and vortex ratchet in a superconductor.[4] As demonstrated in Ref. [5], asymmetric ratchet and unbiased driving force are two necessary conditions for operation of Brownian ratchet in one-dimensional pores of silicon membrane. Experimental investigation of ratchet effect in an easy way at the kinetic level is still highly sought until now.
Dusty plasma consists of electrons, ions, neutral gas atoms, and dust particles.[11–20] Due to the collection of electrons and ions from the plasma, these particles gain more than thousands of elementary charges and often become strongly coupled. Typical time scales of dynamical processes for the dust subsystem are in the range of 10–100 Hz. Therefore, micron-sized dust particles can be visualized individually and tracked easily in experiments at the kinetic level. This makes it an ideal model system for the study of phase transitions, strong coupling, and nonlinear waves phenomena, etc.[21–25]
In a recent experimental study, we designed a dusty plasma ratchet and realized the rectification of dust particles into directional motion in plasma environment.[26] We explored the fundamental mechanisms inducing the directional movement of dust particles: asymmetric ratchet potential and collective effect. In the previous experiments, we found that the sawtooth number on each gear could affect the directional movement of particles. The change of the sawtooth number modifies the degree of asymmetry of the ratchet potential. In the present study, we report the directional motion of dust particles in the saw channel with different sawtooth numbers in detail. Too many or too few sawtooth numbers will not cause the directional movement of dust particles. There exists an optimal sawtooth number which can give rise to fast motion of dust particles in the saw channel. We also explore the nonuniform motion of dust particles in the saw channel. Our results are helpful for understanding well the mechanisms of rectification of dust particles in the dusty plasma ratchet.
The experiments are performed in a vacuum chamber filled with argon as shown by the schematic diagram in Fig.
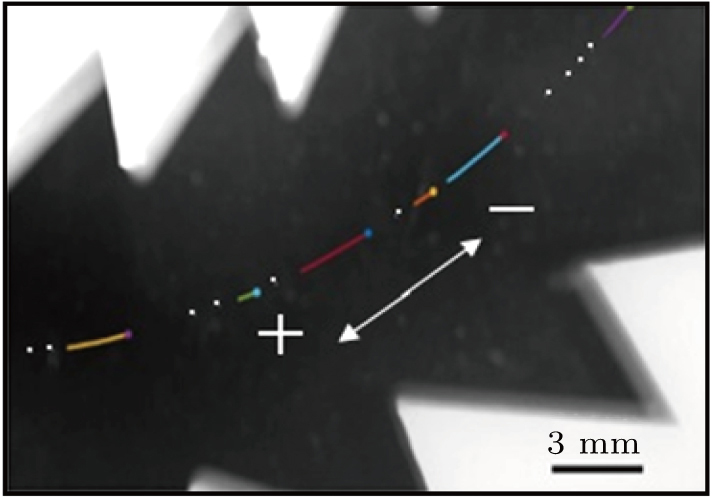
After dropped into the saw channel from one side, the charged dust particles flow along the saw channel due to the strong repulsive action among them. They form a dust particle chain after a transient about several seconds as shown in Fig.
In order to explore the effect of the sawtooth number of gears on dust particle motion, we design a set of gears with the same radius, but different sawtooth numbers changing from n = 28 to n = 72. As we have shown that the number of the dust particles affects the average velocity of dust particles,[26] here we have to keep the number of dust particles as constant as possible after we replace the gears in different experiments. In the present work, the number of dust particles is set to N0 ≈ 340. In this case, the dust particles arrange themselves into a chain along the center of the saw channel as shown in Fig.
Figure
When the sawtooth number n > 44, the average angular velocity decreases with the sawtooth number. When n = 70, the average angular velocity reduces to one order of magnitude of the maximum. It is believed that in the limitation case of n → ∞, the asymmetrical gears would also degenerate to circular rings. Therefore, the asymmetry of the system disappears in this case, which leads to zero flow of dust particles.
To further explore the effect of the sawtooth number on the rectification of dust particles, we consider the couple between the dust particles and the gears in the saw channel. As have been shown in Ref. [26], ratchet potential and collective effects are two keys to the rectification of dust particles. Along the saw channel, the ratchet potential is asymmetric and periodic with a ratchet length L = 2πr/n, here r is the radius of the circular trajectories dust particles following. The negatively-charged dust particles are separated well along the saw channel due to their strongly repulsive action with an average inter particle distance d = 0.75–0.98 mm. Then, one can obtain the number of dust particles within each ratchet well N/n = L/d, here N is the total number of dust particles. Too many or too few dust particles within each ratchet well lead to slow motion of dust particles. A peak appears at L/d ≈ 7, which means that the optimal coupling between the dust particles and the gears is that each ratchet well accommodates ∼7 dust particles. The average angular velocity could reach 0.5 rad/s in this case.
Due to the periodic variation on the width of the saw channel, the angular velocity of the directional motion of dust particles changes as the dust particles are flowing along the center of the saw channel. Figure
Figure
Figure
 | Fig. 5. Dependence of the ratio of the maximum to minimum angular velocities on the sawtooth number. Gas pressure: 30 Pa, RF power: 30 W. |
We further explore the force acting on the dust particle. Based on Fig.
Although the force is variable along the saw channel, its integral over one period of the saw channel is zero. Therefore, the dust particles would perform persistent flow along the saw channel. Our numerical simulations in Ref. [26] showed a clear physical picture of the directional movement of dust particles in the dusty plasma ratchet:[2] under a non-equilibrium driving force of ion drag (∼10−13 N) which could overcome the resistance of neutral gas (∼10−13 N), a large number of dust particles can perform directional motion along the periodic and asymmetrical ratchet potential. In this work, we measure that the resultant forces of dust particles are in the order of 10−14 N.
We have studied the effect of the sawtooth number on the average angular velocity of dust particles and explored the directional movement of dust particles in the saw channel. The change in the sawtooth number of gears affects the structure parameters of gears and then the coupling between the dust particle and the gears. The average angular velocity of the dust particles first increases and then decreases with the increasing sawtooth number. The distribution of angular velocity of dust particles is very nonuniform in the saw channel when the sawtooth number is less than 44. The distribution of the force acting on the dust particle is also nonuniform. Our results are important to finding optimal dust plasma ratchet for further control of the directional motion of dust particles in a plasma.
| [1] | |
| [2] | |
| [3] | |
| [4] | |
| [5] | |
| [6] | |
| [7] | |
| [8] | |
| [9] | |
| [10] | |
| [11] | |
| [12] | |
| [13] | |
| [14] | |
| [15] | |
| [16] | |
| [17] | |
| [18] | |
| [19] | |
| [20] | |
| [21] | |
| [22] | |
| [23] | |
| [24] | |
| [25] | |
| [26] |