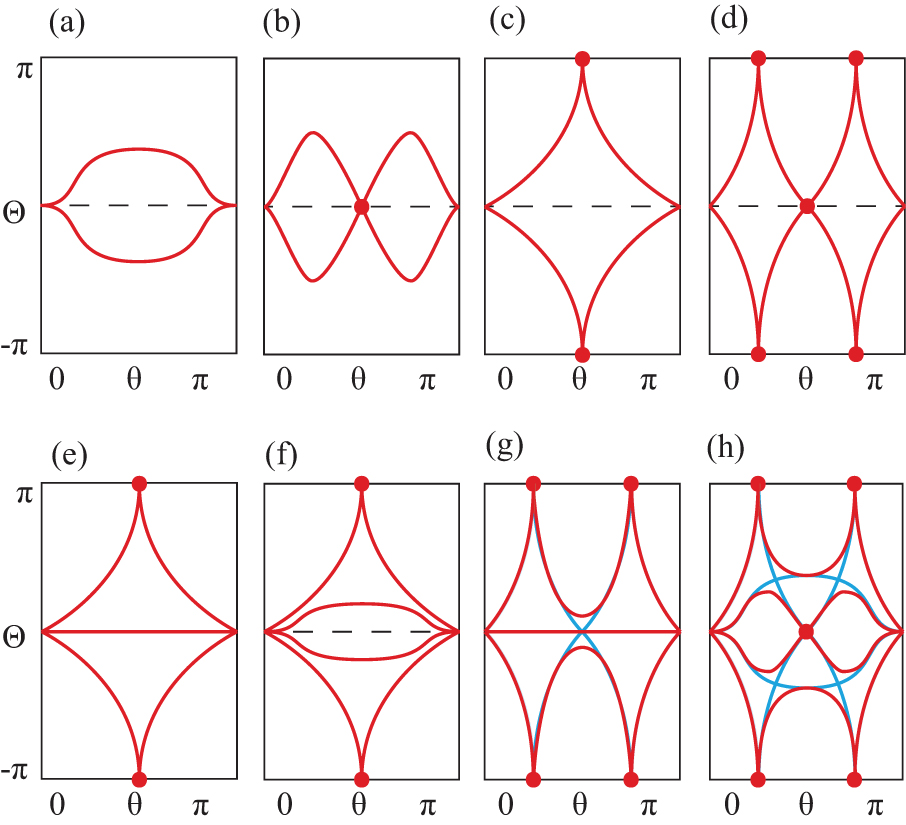
Wilson loop spectra on a sphere. The Wilson loop operator is calculated along the azimuthal direction with a fixed polar angle θ. (a)–(d) When the number of occupied bands N_occ is two. The winding number is (a), (b) zero, (c) one, and (d) two. (b) can be adiabatically deformed to (a) as we push the crossing point on Θ = 0 out of the boundary at θ = 0 or θ = π. (e)–(h) When N_occ = 3 or N_occ = 4. (e) has a flat spectrum on Θ = 0 in addition to (c). Adding a small perturbation to (e) does not deform the spectrum when N_occ = 3, while it deforms the spectrum to (f) when N_occ = 4. Panels (g) and (h) show the Z₂ nature of the Wilson loop spectrum for N_occ = 3 and N_occ = 4, respectively. Blue lines in (g) and (h) are obtained after adding one and two flat bands to (d), respectively, whereas red lines are the spectrum after adding a PT-preserving deformation which eliminates non-protected crossing points. The crossing points on Θ = π can always be pair-annihilated after this deformation. Figures are adopted from the supplemental materials in Ref. [7].