White light-emitting diodes (WLEDs) have exhibited more extensive applications in liquid crystal display backlighting and solid-state general lighting in recent years.^[1–5] However, the most common WLEDs are LED-plus-phosphor-based, and they suffer an unavoidable Stokes energy loss and have a low color-rendering index (CRI).^[2,6,7] Therefore, it is necessary to find a new method of preparing prototype phosphor-free monolithic WLEDs as an alternative to phosphor-based WLEDs. The entire spectral range of a nitride system can be obtained by tuning the indium composition in an InGaN alloy, so the InGaN alloys have become materials having significant potential to be used as active layers in LEDs.^[8–11] It has been reported that monolithic WLEDs can be achieved by stacking dual-wavelength emitting InGaN/GaN multiple quantum wells (MQWs) in GaN p–n junctions.^[12,13]

Currently, the preparation technology of InGaN/GaN MQW-based blue LEDs has become relatively mature, and a high-efficiency (84.3%) blue LED based on InGaN/GaN MQWs has been achieved.^[14] However, the InGaN/GaN MQW-based long-emitting-wavelength LED, such as green LED, is still in its inception in research and development terms; moreover, the optimal parameters for the growth of a high-In-content InGaN epilayer on GaN remain uncertain. The difficulty in preparing high-In-content InGaN epilayers is due mainly to the fact that indium and gallium have a significant discrepancy in atomic size, and the lattice mismatch between InN and GaN is as high as 11%, which may cause slight compositional fluctuations or strong phase separation.^[8] These In-rich regions in this kind of structure serve as localization centers of carriers to improve the radiation recombination rate of the carriers, but at the same time they also lead the structural defects to be generated and light emission efficiency to be reduced.^[9] Moreover, a quantum-confined Stark effect (QCSE) triggered by a piezoelectric polarization field in the high-In-content InGaN/GaN QW due to the large lattice mismatch between GaN and InGaN, also results in an increase in the spatial separation of electrons and holes and a decrease in the radiative recombination efficiency of the carriers in the MQW. Therefore, although monolithic WLEDs simultaneously emitting two colors have been achieved by growing two InGaN/GaN MQW-based active regions with different indium content on a single substrate, the long-wavelength components demonstrated quite a low internal quantum efficiency (IQE).^[12,13] Hence, it is necessary to improve the understanding of the emission mechanism of blue and green dual-wavelength InGaN/GaN MQW epitaxial structures, thus preparing high-performance phosphor-free monolithic WLEDs that emit photons at different wavelengths with an appropriate power ratio.

Photoluminescence (PL) measurements are an effective method to analyze the characteristics of InGaN/GaN MQWs. They can clearly reflect the effects of impurities/defects, composition fluctuation, and strain on optical properties of the MQWs. Here, in order to ascertain the effects of the indium content on the emission mechanism in InGaN/GaN MQWs and the mechanism of interrelation between two active regions grown on a single sapphire substrate, an InGaN/GaN MQW structure containing two active regions with lower (blue MQWs) and higher (green MQWs) indium content, is grown, while dependence of the PL spectrum on temperature (T) and excitation power for the two active regions are investigated in a large range of temperature and excitation power.

Through using the metal-organic chemical vapor deposition (MOCVD), one has grown an InGaN/GaN MQW sample on a sapphire substrate oriented to (0001). Before the process, the substrates were thermally annealed at 1100 °C in a hydrogen atmosphere to remove surface contamination from the sapphire substrate. In the process of growth, a 25-nm-thick GaN nucleation layer was deposited at 530 °C, followed by forming a 4- -thick undoped GaN layer and a 3- -thick Si-doped GaN layer at 1080 °C. Then, three pairs of In_xGa_1−xN/GaN ( ) strain relief layers (SRLs) with 1-nm-thick InGaN wells and 5-nm-thick GaN barriers were grown at 800 °C. Two InGaN/GaN MQW active regions, blue MQW (BMQW) and green MQW (GMQW), were formed at the same trimethylindium (TMIn) flow rate and in an N₂ ambient atmosphere after growing SRL: firstly, three pairs of In_xGa_1−xN/GaN ( ) BMQWs with 3-nm-thick InGaN wells (750 °C) and 17-nm-thick GaN barriers (850 °C) separately were grown, followed by growing nine pairs of In_yGa_1−yN/GaN ( ) GMQW with InGaN wells (725 °C, 3-nm thick) and GaN barriers (850 °C, 17-nm thick).

For the measurement of PL dependence on excitation power and temperature, the sample was placed in a closed-cycle He cryostat to control the temperature changing from 6 K to 300 K. A 405-nm continuous wave (CW) semiconductor laser with a spot size of approximately 300 was adopted to excite the PL signals, and the excitation power was varied between 0.005 mW and 100 mW. A Jobin–Yvon iHR320 monochromator and a thermo-electrical cooled Synapse charge-coupled device (CCD) detector were used for the dispersion and detection of PL signals from the sample.

Figure 1 shows a typical PL spectrum of sample obtained at 100-mW excitation power and 300 K. Two emission peaks related to InGaN matrix can be found at approximate 2.603 eV (476 nm) and 2.338 eV (530 nm), which are assigned to the near-band-edge transitions (denoted by P_B and P_G) related to the BMQW matrix and GMQW matrix, respectively. To reveal the transfer and recombination mechanism of carriers in the two active regions, the dependence of their PL spectra on temperature and excitation power are presented and discussed below.

Fig. 1.

	Figure Option ViewDownloadNew Window
	Fig. 1. Measured PL spectrum of sample at 100 mW and 300 K.

Figure 2 shows the dependence of peak energy for the two emissions (P_B and P_G) on temperature at 0.005-mW excitation power. It can be found from Fig. 2 that no dependence follows Varshniʼs equation^[15]

Fig. 2.

	Figure Option ViewDownloadNew Window
	Fig. 2. Temperature-dependent peak energy for PB and PG, measured at 0.005 mW.

Figure 3(a) and 3(b) show excitation-power-dependent P_B peak energy and line-width at 6 K and 300 K, respectively. As shown in Fig. 3(a), when the excitation power increases from 0.005 mW to 100 mW at 6 K, the peak energy tends to increase monotonically, showing a narrowing line-width below 2 mW and then a broadening line-width above 2 mW. The former is explained by the Coulomb screening of the QCSE in the BMQW, and the latter is mainly because of the band filling of the localized centers in the higher excitation power range due to the reduction of the QCSE.^[9,18–20] At 300 K, as seen in Fig. 3(b), comparing with the situation at 6 K, both initial increase in the peak energy and the initial narrowing in the peak line-width, which is a function of the excitation power, become less significant in a lower range of excitation power below ∼0.05 mW. This phenomenon may be attributed to the combined effect of Coulomb screening and non-radiative recombination, since the defect-related non-radiative centers in the BMQW are thermally activated at 300 K. Nevertheless, as the excitation power increases to greater than ∼0.05 mW, a trend similar to that observed in Fig. 3(a) is seen in Fig. 3(b) as non-radiative centers become saturated.

Fig. 3.

	Figure Option ViewDownloadNew Window
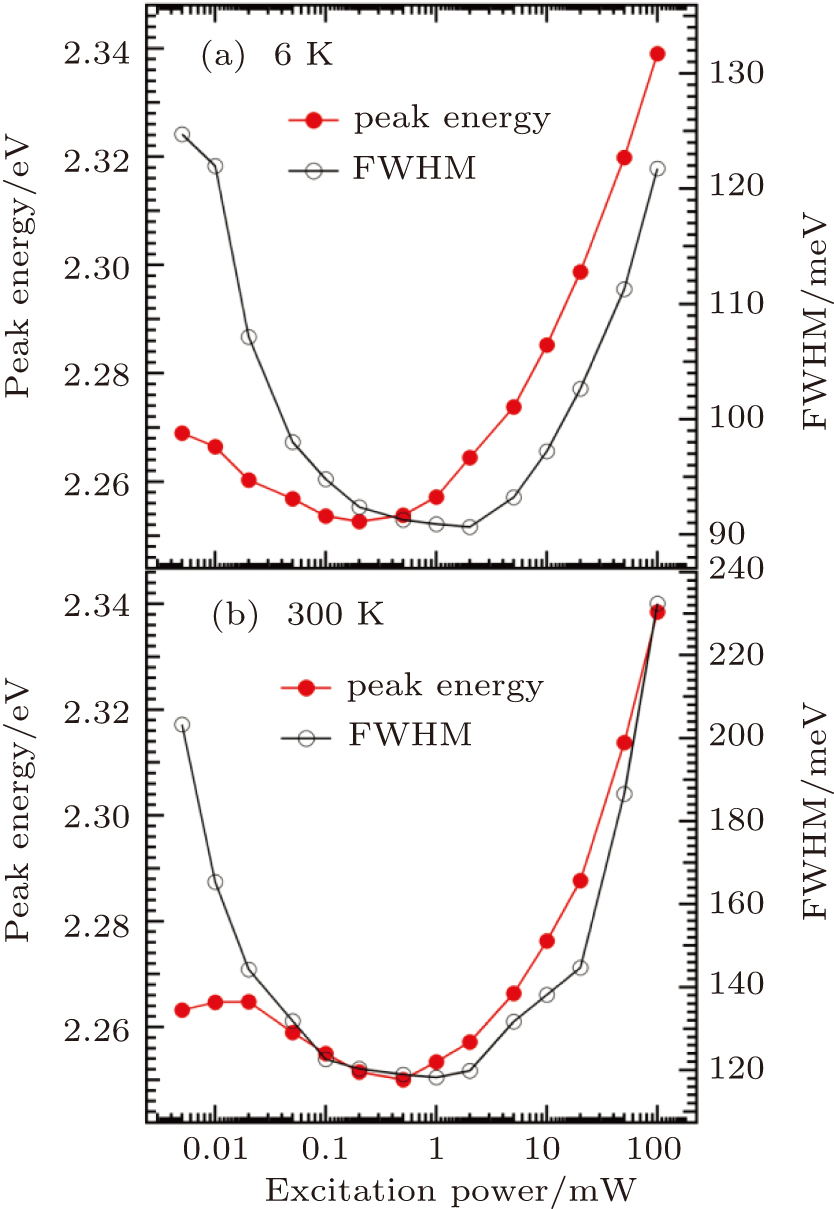
	Fig. 3. Dependence of PB peak energy and full-width at half-maximum (FWHM) on excitation power, measured at 6 K (a) and 300 K (b).

Figure 4(a) and 4(b) show the excitation-power-dependent P_G peak energy and line-width, measured at 6 K and 300 K, respectively. Figure 4(a) shows that at 6 K, unlike the case of the P_B peak energy at 6 K in Fig. 3(a), when the excitation power rises within an excitation power range below ∼0.2 mW, both the P_G peak energy and line-width significantly decrease. This happens because of an strengthened scattering effect of carriers, which results in carriers being transferred from shallow localized states downwards deeper states through tunneling effect when the excitation power is increased.^[21,22] The aforementioned experimental result (Fig. 2) evinces this point: a GMQW has a stronger potential inhomogeneity and localized characteristic for recombination of carriers than a BMQW; however, when the excitation power exceeds 0.2 mW, the scattering effect of carriers is inhibited because deep localized states are saturated, so a similar phenomenon (Fig. 3(a) for P_B) is observed across a higher excitation power range above ∼0.2 mW in Fig. 4(a) for P_G. However, at 300 K, it can be seen from Fig. 4(b) that unlike the situation at 6 K (Fig. 4(a)), the P_G peak energy increases slightly as the excitation power increases from 0.005 mW to ∼0.02 mW. Apart from this, the peak line-width exhibits that it narrows down to 59.0 meV at 300 K, which is markedly larger than that (17.6 meV) at 6 K in the range of evaluating excitation power. This happens because at the higher temperature (300 K), localized carriers in the GMQW are thermally activated; activated carriers partially occupy the localized states at higher energy levels and partially shift from localized states into conduction and valence bands. As a result, when the excitation power is increased from 0.005 mW to 0.02 mW, the scattering effect of localized carriers and Coulomb screening effect of free carriers together play a dominant role in the recombining of the GMQW, and this leads to a very slight increase in the peak energy, in addition to significant narrowing of the line-width. Nevertheless, if the excitation power exceeds ∼0.02 mW, the Coulomb screening effect of free carriers gradually diminishes and scattering effect of localized carriers is enhanced since the conduction and valence band of the GMQW structure gradually become flat. Hence, a phenomenon similar to that described in Fig. 4(a) occurs at excitation powers above 0.02 mW (Fig. 4(b)).

Fig. 4.

	Figure Option ViewDownloadNew Window
	Fig. 4. Dependence of PG peak energy and FWHM on excitation power at 6 K (a) and 300 K (b).

For assessing and comparing the strengths of QCSE in the two aforementioned MQWs, the excitation-power-dependent PL peak energy and line-widths shown in Figs. 3 and 4, will be further analyzed. As discussed above for both MQWs in Figs. 3 and 4, the dominant carrier recombination mechanism is excitation-power-dependent, and the carrier recombination process contains several possible mechanisms combining dominant and non-dominant positions, such as i) Coulomb screening of QCSE, ii) band filling for localized states, iii) carrier-scattering, and iv) defect-related non-radiative recombination, as mentioned above. Although the different recombination mechanisms result in the different excitation-power-dependent behaviors of the PL spectra, i.e., the above mechanism i) (mechanism ii)) results in a peak blue-shift (blue-shift) together with the line-width narrowing (broadening), and the above mechanism iii) (mechanism iv)) results in a peak red-shift (red-shift) together with the line-width narrowing (broadening), the strengths of QCSE of these two MQWs can be compared with each other based on the experimental fact that when the excitation power is increased from 0.005 mW to 100 mW, the total blue-shift (narrowing) of peak position (line-width) is about 70 meV (3.0 meV) for the P_G emission, and 37 meV (−10.3 meV) for the P_B emission at 6 K, and is about 75 meV (−29.1 meV) for the P_G emission and 37 meV (−49.5 meV) for the P_B emission at 300 K. That is, the total blue-shift (narrowing) of peak positions (line-width) for the P_G emission is more significant than that for the P_B emission in the range of excitation powers evaluated at both 6 K and 300 K. The results show that the GMQW has a stronger QCSE than the BMQW.

Figure 5(a) shows integrated PL intensity (I) varying with excitation power (P) for P_B emission and P_G emission at 300 K. Usually, the relationship between I and P is expressed as^[8,9]

Fig. 5.

	Figure Option ViewDownloadNew Window
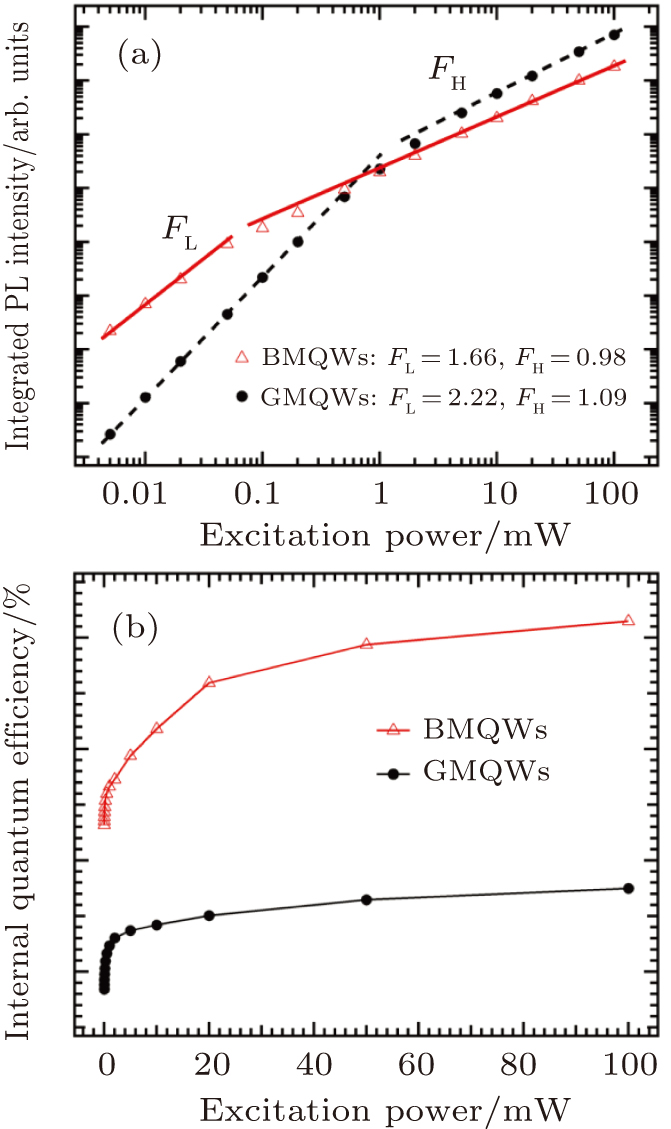	Fig. 5. Integrated PL intensities (a) and internal quantum efficiencies (b) for the BMQW and the GMQW (shown as functions of excitation power).

Figure 5(b) shows the excitation-power-dependent IQE of the BMQW and GMQW. IQE is defined here as the ratio of integrated PL intensity at 300 K to that at 6 K.^[9,23] As shown in Fig. 5(b), IQE for the BMQW and GMQW gradually increase as the excitation power is increased from 0.005 mW to 100 mW. This behavior is attributed to the fact that at 300 K, when the excitation power increases, non-radiative recombination gradually weakens but radiative recombination gradually increases because the non-radiative centers gradually become saturated. This leads the IQE to gradually increase for both the BMQW and the GMQW. In addition, as shown in Fig. 5(b), the GMQW demonstrates lower IQE within the selected measurement range than the BMQW. This can be mainly attributed to the more non-radiative centers in the GMQW than in the BMQW, due to the marked deterioration of the crystalline quality of the GMQW with higher indium content.

In this work, an InGaN/GaN MQW sample containing two active regions, BMQW and GMQW (the latter is deposited on the former), is grown. In addition, the PL spectra of the sample are studied at excitation powers in a range between 0.005 mW and 100 mW and temperatures ranging from 6 K to 300 K. It is found that the peak energy of P_G emission demonstrates stronger dependence in an “S-shape” on temperature than that of P_B emission, and an excitation-power-dependent carrier-scattering effect is observed only in the P_G emission; when the excitation power is increased from 0.005 mW to 100 mW separately at 6 K and 300 K, the total blue-shift (narrowing) of peak position (line-width) for the P_G emission is more significant than that for P_B emission; the GMQW has a lower IQE than the BMQW. All of these results can be attributed to the fact that the GMQW has higher indium content than the BMQW, and the higher indium content in the GMQW induces a more significant compositional fluctuation and localized character of the carrier recombination, a stronger QCSE, and more non-radiative centers. The reason why more indium elements are incorporated into the GMQW is considered mainly because the GMQW is grown at a lower temperature (725 °C). Additionally, the BMQW underlying layer, acting as a layer for strain release, also facilitates the incorporation of In into the GMQW and the In compositional fluctuation therein.