1. IntroductionHigh-order harmonic generation (HHG) which is a useful tool to monitor quantum dynamics has been intensively investigated.[1–7] HHG could be used to produce attosecond pulse[8–12] that is a powerful tool to probe, track, control the dynamics of electron in ultrafast process.[13–16] Recently, linearly polarized attosecond pulse could be obtained from linear polarized harmonics in experiments,[17,18] and the HHG process is well described by semiclassical three-step model.[13] In circular or highly elliptical field, the atoms or molecules have a probability to be ionized but the electron scarcely ever recombines to the parent ion, thus circular-polarized harmonic (CPH) is hardly obtained by using a circular driver fields.[19,20] However, a CPH signal is needed to well understand the chiral-sensitive phenomena such as ultrafast chiral-specific dynamics in molecules, the character of magnetic materials and nanostructures, and so on.[21–23] So that the CPH generation[24–27] and its potential application[28] are a hot topic in very recent years.
Counter rotating bicircular (CRB) field scheme has been proposed to generate CPH or highly elliptical light pulses. Generally, this scheme combines a right-circular-polarized (RCP) pulse with a left-circular-polarized (LCP) second harmonics, first proposed in Ref. [29]. This scheme was proven experimentally[30] and it has been applied to generate an attosecond pulse recently.[31,32] For the combination field, a trefoil structure would be obtained in Lissajous curve with a threefold symmetry: the system remains constant by the combination of 120° rotation and a time delay of one-third of the fundamental frequency field period.[33] For centrally symmetric system such as atom targets, the harmonic orders of 3n+1 and 3n+2, which correspond to RCP and LCP harmonics respectively can be generated. The harmonic orders of 3n are absent because of the symmetry.[24,31,32,34–36] For the molecule targets, odd–even harmonics can also be emitted due to the asymmetry of the initial-state electron wave function,[37,38] hence the selection rules are more elaborate because it based on both the symmetry of the target and the symmetry of the field.[39–42] A linearly attosecond pulse can be obtained when the intensities of RCP and LCP harmonics are equal. Consequently, it would be meaningful if the high elliptically or circularly attosecond pulse can be obtained which means the ellipticity of the attosecond pulse can be controlled.
CPH generation is investigated from rare gas, such as helium, neon, argon, both in experiments and in theory. As we all know, the ground state of those gases is p orbital except helium and has three degenerate orbitals sign as
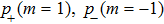 , p0 (m=0). In this paper, the HHG from the p orbital of neon atom by the CRB field is theoretically investigated. Since the Ne atoms with closed electron shells, a hydrogen-like model to simulate Ne atoms can also satisfy the selection rules as the p orbital:[43]
, p0 (m=0). In this paper, the HHG from the p orbital of neon atom by the CRB field is theoretically investigated. Since the Ne atoms with closed electron shells, a hydrogen-like model to simulate Ne atoms can also satisfy the selection rules as the p orbital:[43]
 (n is an integer). But it cannot simulate the intensity regularities between adjacent harmonics well.[24,43] Our work intends to focus on the selection of the same helicity, thus choosing the p orbital as the initial state is necessary. The helicity of HHG is studied by calculating the phase differences between x and y components. The right-circular-polarized harmonics could be selected in the p orbital of the neon atom by adjust the relative intensity of the pulses. Time–frequency analysis and the semiclassical three-step model are used to study the short and long paths.
(n is an integer). But it cannot simulate the intensity regularities between adjacent harmonics well.[24,43] Our work intends to focus on the selection of the same helicity, thus choosing the p orbital as the initial state is necessary. The helicity of HHG is studied by calculating the phase differences between x and y components. The right-circular-polarized harmonics could be selected in the p orbital of the neon atom by adjust the relative intensity of the pulses. Time–frequency analysis and the semiclassical three-step model are used to study the short and long paths.
3. Results and discussionTo simulate the HHG from 2p orbital of neon, we numerically solve Eq. (1) with the initial state in two cases by the method used in Ref. [24]. The initial states for m=±1 are defined as
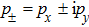 , the p0 orbital is neglected because it has a node in the polarization plane and the contribution to the HHG is too weak from the p0 orbital.[40] In our simulation, the CRB field is combined by a
, the p0 orbital is neglected because it has a node in the polarization plane and the contribution to the HHG is too weak from the p0 orbital.[40] In our simulation, the CRB field is combined by a
 -nm RCP pulse with
-nm RCP pulse with
 -nm LCP pulse, and the intensity of each field is
-nm LCP pulse, and the intensity of each field is
 . First, we investigate HHG for the initial state in p+ orbital, as shown in the left column of Fig. 1. To clearly show the harmonic orders in the plateau area, Figure 1(a) shows the harmonic spectra from 30th order to 90th order. The overall character of the HHG is shown in the inset of Fig. 1(a). The following characters are obviously observed from harmonic spectra: (i) The harmonic orders of 3n+1 and 3n+2 are obtained in the whole plateau area and the intensities of 3n+2 orders are lower than the 3n+1 orders. (ii) The intensity of the spectrum in x component is approximately equal to the intensity in y component at each 3n+1 order. We also calculate the phase difference of the HHG by Eq. (10), and the result is shown in Fig. 1(c), the orders of 3n+1 and 3n+2 are marked by the red solid circle and the blue solid square, respectively. The phase differences are stable near π/2 for harmonic orders of 3n+1 while fluctuant near the −π/2 for the orders of 3n+2. Because the harmonic intensities are equal at the x and y components and the phase differences are nearly π/2, the harmonic orders of 3n+1 correspond to perfect RCP harmonic.
. First, we investigate HHG for the initial state in p+ orbital, as shown in the left column of Fig. 1. To clearly show the harmonic orders in the plateau area, Figure 1(a) shows the harmonic spectra from 30th order to 90th order. The overall character of the HHG is shown in the inset of Fig. 1(a). The following characters are obviously observed from harmonic spectra: (i) The harmonic orders of 3n+1 and 3n+2 are obtained in the whole plateau area and the intensities of 3n+2 orders are lower than the 3n+1 orders. (ii) The intensity of the spectrum in x component is approximately equal to the intensity in y component at each 3n+1 order. We also calculate the phase difference of the HHG by Eq. (10), and the result is shown in Fig. 1(c), the orders of 3n+1 and 3n+2 are marked by the red solid circle and the blue solid square, respectively. The phase differences are stable near π/2 for harmonic orders of 3n+1 while fluctuant near the −π/2 for the orders of 3n+2. Because the harmonic intensities are equal at the x and y components and the phase differences are nearly π/2, the harmonic orders of 3n+1 correspond to perfect RCP harmonic.
Second, we investigate HHG for the initial state in p− orbital, as shown in the right column of Fig. 1. Contrary to the situation of p+: (I) The intensities of 3n+2 orders are higher than the 3n+1 orders. (II) The harmonic intensities between the x and y components are almost equal at each 3n+2 order. (III) The phase differences are stable near −π/2 for harmonic orders of 3n+2 while fluctuant near the π/2 for the orders of 3n+1. Based on the above phenomenon and analyses, perfect LCP harmonic could be obtained at the orders of 3n+2 in p− orbitals. From Figs. 1(b) and 1(d), the phase differences are not stable for the 3n+2 or 3n+1 orders, thus left-handed or right-handed elliptically polarized harmonics can be obtained in p+, p− orbitals. However, the real initial state of neon atom is coupled by p+ and p− orbitals, it is necessary to calculate the total harmonic spectrum to simulate the phenomenon in the experiment.
The total spectra of HHG are calculated by Eqs. (7)–(9), and the total dipole acceleration in
 component is obtained by
component is obtained by
 (i is x or y). Figure 2(a) and 2(b) show the total HHG, the orders of 3n+1 and 3n+2 appear in the whole plateau region. The intensities of 3n+1 orders are higher than 3n+2 orders, the electron in continuum state is affected more strongly by the lower-frequency field, thus the polarization of the harmonics is affected more strongly by the fundamental field, that is the RCP. From Fig. 2(c), the phase differences in the plateau region are stable at π/2 and −π/2 for the harmonic orders of 3n+1 and 3n+2, (i.e., a pair of perfect circular-polarized harmonics with RCP and LCP are obtained, respectively. As mentioned in Ref. [19], linearly attosecond pulses will be synthesized if RCP and LCP harmonics have the same intensities. Therefore, while keeping RCP and LCP harmonics in perfect circular-polarization, RCP or LCP could be selected and a simple and straightforward method to generate highly elliptically or circularly attosecond pulse trains is desirable.
(i is x or y). Figure 2(a) and 2(b) show the total HHG, the orders of 3n+1 and 3n+2 appear in the whole plateau region. The intensities of 3n+1 orders are higher than 3n+2 orders, the electron in continuum state is affected more strongly by the lower-frequency field, thus the polarization of the harmonics is affected more strongly by the fundamental field, that is the RCP. From Fig. 2(c), the phase differences in the plateau region are stable at π/2 and −π/2 for the harmonic orders of 3n+1 and 3n+2, (i.e., a pair of perfect circular-polarized harmonics with RCP and LCP are obtained, respectively. As mentioned in Ref. [19], linearly attosecond pulses will be synthesized if RCP and LCP harmonics have the same intensities. Therefore, while keeping RCP and LCP harmonics in perfect circular-polarization, RCP or LCP could be selected and a simple and straightforward method to generate highly elliptically or circularly attosecond pulse trains is desirable.
The mechanism of HHG is further discussed by the time-frequency analysis in synchro squeezing transforms (SST) method. The SST can solve the problem of spectrum ambiguity and analyze lower orders harmonic more clearly.[45,46] Figure 3(a) shows the time–frequency analysis by transforming the total dipole acceleration in x component. Three quantum paths are obtained in each optical cycle. The HHG is mainly contributed by short paths which are marked by Ax, Bx, and Cx in the optical cycle of [2.8T1, 3.8T1]. The intensity of Ax is stronger than those of Bx and Cx in the above-threshold region (above 28th order). In the below-threshold region, the intensities of the three peaks are weak, corresponding to the weak spectra in this region as shown in the inset of Fig. 1. For the case of y component, the time–frequency analysis is shown in Fig. 3(b). There are also three quantum paths in each optical cycle. However, the intensity of Ay is very weak and the intensity of By is stronger than the peak of Cy in the region of above threshold. Why are the intensities of three peaks different on x component and y component? We will discuss the reason by the semiclassical three-step model.
As everyone knows, the field is threefold symmetry in one cycle for the case of
 . The combined CRB field is shown in Fig. 4(a), the inset is Lissajous curves of the combination field in plateau region of the trapezoidal envelope, the lobes A, B, C are marked with background colors of gray, red, and green in one-cycle electric field of the pulse. The threefold symmetry leads to the three paths[35,47] as discussed in Fig. 3. To give a clear picture of the HHG, we solve the Newtonʼs equation under the CRB field.[48–50] The initial velocity in x component (i.e., OA direction) is set to zero but the initial velocity in y component is nonzero when the ionization time is in lobe A, as discussed in Ref. [44]. For the case of ionization time in lobe B or lobe C, we set the initial velocity to zero at the OB or OC direction, and set to non-zero at the direction perpendicular to OB or OC, the similar results can be obtained due to the symmetry of the threefold. Figure 4(b) shows kinetic energy in unit of
. The combined CRB field is shown in Fig. 4(a), the inset is Lissajous curves of the combination field in plateau region of the trapezoidal envelope, the lobes A, B, C are marked with background colors of gray, red, and green in one-cycle electric field of the pulse. The threefold symmetry leads to the three paths[35,47] as discussed in Fig. 3. To give a clear picture of the HHG, we solve the Newtonʼs equation under the CRB field.[48–50] The initial velocity in x component (i.e., OA direction) is set to zero but the initial velocity in y component is nonzero when the ionization time is in lobe A, as discussed in Ref. [44]. For the case of ionization time in lobe B or lobe C, we set the initial velocity to zero at the OB or OC direction, and set to non-zero at the direction perpendicular to OB or OC, the similar results can be obtained due to the symmetry of the threefold. Figure 4(b) shows kinetic energy in unit of
 , two kinetic energy peaks which ionized in lobe C are marked with
, two kinetic energy peaks which ionized in lobe C are marked with
 and
and
 , and the corresponding emission times in lobe A and lobe B are marked with
, and the corresponding emission times in lobe A and lobe B are marked with
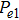 and
and
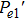 respectively. Although short and long paths are obtained from classical model, HHG is mainly contributed by short path in fact,[35,44] and this character can also be found from Fig. 3. The intensities of HHG contributed from long path may lower due to the wave function diffusion in the laser field. So in order to understand characteristics of HHG more clearly, short path is mainly studied. Moreover, the time of electron oscillation is longer for the peak
respectively. Although short and long paths are obtained from classical model, HHG is mainly contributed by short path in fact,[35,44] and this character can also be found from Fig. 3. The intensities of HHG contributed from long path may lower due to the wave function diffusion in the laser field. So in order to understand characteristics of HHG more clearly, short path is mainly studied. Moreover, the time of electron oscillation is longer for the peak
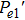 than
than
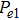 , thus the electron mainly recombines to the parent nucleus in peak
, thus the electron mainly recombines to the parent nucleus in peak
 for the case ionized from lobe C. Similarly, for the ionization time in the region of lobe A or lobe B, HHG are mainly contributed by the peaks
for the case ionized from lobe C. Similarly, for the ionization time in the region of lobe A or lobe B, HHG are mainly contributed by the peaks
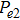 and
and
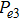 . Those three peaks are corresponding to the quantum paths
. Those three peaks are corresponding to the quantum paths
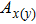 ,
,
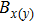 , and
, and
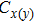 , respectively.
, respectively.
The emission time of short quantum path is analyzed to explain the relative intensity of the quantum paths. In the time range of peak
 ,
,
 , which is corresponding to time range marked by gray background in Fig. 4(a). So that the electron recombines to the parent nucleus in x component is stronger, this maybe the reason the intensity of quantum path Ax is much larger than that of the path Ay. The phenomenon could also be verified for the paths
, which is corresponding to time range marked by gray background in Fig. 4(a). So that the electron recombines to the parent nucleus in x component is stronger, this maybe the reason the intensity of quantum path Ax is much larger than that of the path Ay. The phenomenon could also be verified for the paths
 and
and
 . In the time range of peaks
. In the time range of peaks
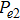 and
and
 , the electric field strength in x component is weaker than that in y component, thus the intensities of Bx and Cx are weaker than those of By and Cy. The semiclassical three-step model gives a clear physical picture, which qualitatively explains physical process and relative intensity of the quantum paths.
, the electric field strength in x component is weaker than that in y component, thus the intensities of Bx and Cx are weaker than those of By and Cy. The semiclassical three-step model gives a clear physical picture, which qualitatively explains physical process and relative intensity of the quantum paths.
In order to select right-circular-polarized harmonics, we keep the total intensities of RCP and LCP pulses unchanged and try to adjust the intensity ratio
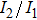 by changing the amplitude ratio γ. Figure 5(a) shows harmonic spectra when γ=0.8, compared with Fig. 2(a), we find that the intensities of harmonics are slightly decreased in the whole plateau region, and the intensities of 3n+2 orders get weaker. To clearly analyze the relation of the intesity, we define a quantitative parameter χ to show relative intensity between 3n+1 and 3n+2 orders of the harmonic:
by changing the amplitude ratio γ. Figure 5(a) shows harmonic spectra when γ=0.8, compared with Fig. 2(a), we find that the intensities of harmonics are slightly decreased in the whole plateau region, and the intensities of 3n+2 orders get weaker. To clearly analyze the relation of the intesity, we define a quantitative parameter χ to show relative intensity between 3n+1 and 3n+2 orders of the harmonic:
where S(i) is the intensity of the i-th order, i=3n+1 or 3n+2. From Fig. 5(c), contrasting the γ=1 and γ=0.8 for the p orbital, we note that the relative intensity χ is greater than 0.64, which means the intensities of 3n+1 orders are stronger than those of 3n+2 orders in both cases. The relative intensity for the case of γ=0.8 is higher than γ=1 obviously. Even the value of χ is close to 1 when n = 11, 13, indicating that the 3n+2 orders harmonics are almost suppressed. We also try other values of γ (not shown here) and find the harmonics of the same helicility as the fundamental field could be selected by changing the intensity ratio, but the intensities of the overall harmonic will decrease as the intensity ratio decreases.
Could the intensity ratio affect the harmonic generation from hydrogen-like model of the neon atom in three fold electric field? The hydrogen-like model whose potential is taken from
 is used to simulate the neon atom. The harmonic spectra of the neon atom with s orbital as the initial state under the same laser pulses irradiation is shown in Fig. 5(b). The intensities of 3n+1 orders harmonics are a little stronger than those of 3n+2 orders for the case of γ=0.8, and they are decreased in pair after 70th order. As shown in Fig. 5(c), the relative intensity χ(n) of harmonics is less than 0.54. By analyzing s orbital, the right-circular-polarized harmonics can also be selected by changing the intensity ratio, while the effect of enhancing is really weak. From the above discussion, we can achieve the selection of right-circular-polarized harmonics by laser field action on the p orbital of the neon atom with increasing the intensities of RCP pulse. We atrribute the selection of the right-circular-polarized harmonics to the stronger effect of the fundamental field, which leads to probability of recombination on p+. So when the intensities of RCP pulse are increased, the intensities of harmonic whose helicity direction is the same as RCP pulse could be enhanced.
is used to simulate the neon atom. The harmonic spectra of the neon atom with s orbital as the initial state under the same laser pulses irradiation is shown in Fig. 5(b). The intensities of 3n+1 orders harmonics are a little stronger than those of 3n+2 orders for the case of γ=0.8, and they are decreased in pair after 70th order. As shown in Fig. 5(c), the relative intensity χ(n) of harmonics is less than 0.54. By analyzing s orbital, the right-circular-polarized harmonics can also be selected by changing the intensity ratio, while the effect of enhancing is really weak. From the above discussion, we can achieve the selection of right-circular-polarized harmonics by laser field action on the p orbital of the neon atom with increasing the intensities of RCP pulse. We atrribute the selection of the right-circular-polarized harmonics to the stronger effect of the fundamental field, which leads to probability of recombination on p+. So when the intensities of RCP pulse are increased, the intensities of harmonic whose helicity direction is the same as RCP pulse could be enhanced.