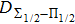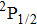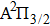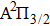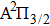† Corresponding author. E-mail:
Full quantum mechanical calculations are performed to determine the broadening in the far wings of the cesium D1 and D2 line shapes arising from elastic collisions of Cs atom with inert helium atoms. The potential energy curves of the low-lying CsHe molecular states, as well as the related transition dipole moments, are carefully computed from ab initio methods based on state-averaged complete active space self-consistent field–multireference configuration interaction (SA-CASSCF–MRCI) calculations, involving the spin–orbit effect, and taking into account the Davidson and BSSE corrections. The absorption and emission reduced coefficients are determined in the temperature and wavelength ranges of 323–3000 K and 800–1000 nm, respectively. Both profiles of the absorption and the emission are dominated by the free–free transitions, and exhibit a satellite peak in the blue wing near the wavelength 825 nm, attributed to 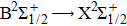
The principal and dominant alkali-metal D1 and D2 lines, broadened by elastic collisions with rare-gases, have been a subject of many theoretical and experimental studies. More precisely, the determination of the profiles and satellite features in the wings of the heavy alkaline atoms evolving in the bath of He atoms has been, very recently, the goal of several theoretical[1–7] and experimental[5,8,9] works.
On the other hand, the spectroscopic studies of the alkali–rare-gas system have demonstrated their significance in a few fields, such as astrophysics and laser physics. In fact, the experimental measurement or theoretically simulation of the absorption–emission spectra must be a crucial tool for determining the physical and chemical properties of the environments of extrasolar giant planets and brown dwarfs.[10–16] Furthermore, the analysis of the collisionally induced spectral broadening of the D1 and D2 lines has played a very significant role in the conceptual design and manufacturing of several new type and efficient lasers, namely, the diode-pumped alkali lasers (DPAL),[7,17–20] and the excimer-pumped alkali vapor lasers (XPAL),[21–24] which was proposed as an alternative to high-power diode-pumped solid-state lasers, and could be used not only for technological applications but also for very important medical application in magnetic resonance imaging.
As far as we know, the photoabsorption profiles generated by the pressure-broadening of D1 and D2 lines of cesium atom immersed in a bath of diluted helium gas has been studied theoretically, in the frame work of classical theory, by Allard et al.[1] and Hager et al.[5] using the unified and Anderson–Tallman theories, respectively. In addition, the photoemission profiles are experimentally realized by Hedges et al.,[8] however, the photoabsorption spectra are very recently measured by Hager et al.[5]
The primary focus of the current work is to determine, in the framework of purely quantum mechanical study, the photoabsorption and photoemission profiles of the D1 and D2 lines of the cesium atom perturbed by the helium one, and the satellite features appearing in the wings. We also analyze carefully the behavior of the shape profiles, the form of the satellites, and their positions at certain well-defined temperature values.
For this purpose, we start with calculating the potential-energy curves (PECs) of the low-lying CsHe molecular states, namely, the ground 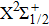
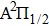
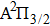


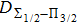

We are interested, in this work, in the far-wing profiles arising from 
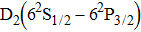
We must precise that during the transition process, the first broadened D1 line of absorber–perturber is attributed to the transitions between the ground 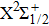




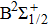




In the following, we will especially focus on the determination of reduced coefficients of the photoabsorption and the photoemission.
One can characterize the pressure-broadened profile of the cesium D1 or D2 resonance line in the presence of the helium He(1s2) atoms by defining the temperature-dependent reduced absorption coefficient 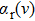

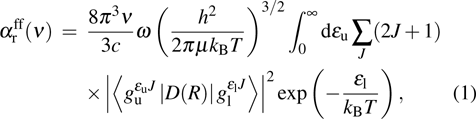 |
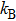
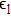
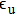
 |
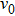
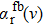
 |
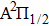

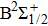
We notice that the rotational quantum numbers J involved in the computations are generally very large. It is, therefore, possible to assume 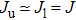
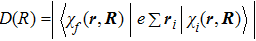



Furthermore, the radial-wave functions 
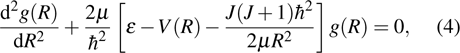 |

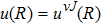
For the emission spectra, we consider for the D1 line the bound–free and free–free transitions between the 



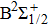

On one hand, the free–free reduced emission coefficients 
 |
 |
We investigate the fourth low-lying doublet electronic states of the molecule CsHe using complete active space self consistent field (CASSCF) procedure followed by a multireference configuration interaction with Davidson correction treatment for the electron correlation. The energies for Ω states have been obtained using the state-interacting method, which means that the spin–orbit eigenstates are obtained by diagonalizing 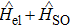


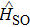

We use the aug-cc-pCV5z[32] basis set for helium atom while the small Stugrart relativistic pseudopotential is used for Cs atom,[33] with its corresponding basis set for s, p, d, and f, and after small modifications for the last four exponents p basis set that become: 0.121000,0.065500,0.016200,0.0061 with two additional ones which are 0.0026,0.0016 and leaving intact their coefficients. The change are also made on the last two sets of three parameters that belong to the term series of p spin–orbit pseudopotential. These two sets of parameters are: (2,2.280961580,−23.7438456037161) and (2,2.103490505,23.7485580204565).
In this pseudo potential, only 9 electrons are explicitly considered. In CsHe molecule, the s and p inner orbitals of Cs and the 1s of He are considered as closed orbitals and 1 valence electron is explicitly treated using 6 active orbitals in our CASSCF calculation. The correlations of inner electrons are treated at MRCI level of theory. All computations are performed without symmetry.
Table 

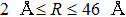
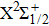




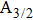
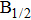







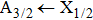

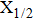
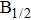


 | Fig. 1. CsHe potential-energy curves V(R) (in a.u.) for the ground 

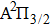
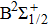
|
| Table 1.
Calculated atomic energy levels (in cm−1) of the Cs atom compared with results from National Institute of Standards and Technology (NIST) recommended data. . |
| Table 2.
Vibrational energy levels Ev (in cm−1) for the CsHe |
| Table 3.
CsHe equilibrium distances Re (in Å) and potential well depths De (in cm−1) compared with other published works. . |
the equilibrium distances for the 
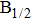

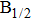
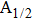
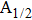
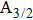
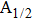
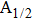

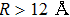

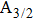




The potential-energy curves and transition dipole moments correctly built in the previous section can be utilized in the computation of the absorption and emission profiles. One has to primarily point out some important details.
The normalized wave functions appearing in Eqs. (
For a given temperature T, the profiles of the broadened lines at the far wings depend closely on the maximum value of the chosen rotational quantum number 

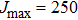
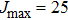

At this stage, one may predict the possible existence of satellites in the far wings by adopting the classical point of view based on potential-difference curves. Indeed, satellites might appear where the curves of the potential differences between the ground and excited states exhibit extrema. Accordingly, we display in Fig. 




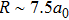
Generally, the photoabsorption profiles around the cesium D1 or D2 resonance lines are determined in the far wings by transitions from the ground to the excited CsHe molecular states, taking into account that the ground and excited molecular states are either repulsive or shallow. We may therefore consider just the transitions of the type free–free or free–bound.
More precisely, for the D1 resonance line broadened around the wavelength 

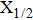





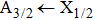
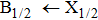
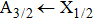
We may mention that in both cases, and for all considered temperatures, the calculations show that the CsHe absorption spectra of the broadened D1 and D2 lines are largely dominated by the free–free transitions.
Nevertheless, the partial reduced absorption coefficients of the broadened D1 line, in the range 800–1000 nm of the wavelength are presented in Fig.
 | Fig. 4. Theoretical reduced photoabsorption coefficients 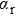
|
We have illustrated in Fig. 
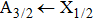

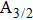
The total absorption profile of the quasi-molecular CsHe is the sum of the partial contributions of the broadened D1 and D2 lines, which can be seen in Fig.
We have at our disposal some results of previous works, as a theoretical study related to calculations of photoabsorption spectra, using a semi-classical method by Allard et al.[1] This study was carried out by Allard and his coworkers in the framework of the unified theory based on the potentials and dipole moments of the CsHe system calculated by Pascale.[42] They theoretically found at the temperature of 1000 K, with the low perturber density of about 1020 cm−3, a blue-wing satellite close to the wavelength 420 cm−1 corresponding to 820 nm. On the other hand, Gilbert and Ch’en[43] observed experimentally a satellite in the blue wing of the CsHe profiles located around 387 ± 9 cm−1 which corresponds to 755 ± 18 nm. In addition, we have presented in Figs.
 | Fig. 6. Comparison of our computed T = 3000 K photoabsorption profiles around the cesium D1 and D2 lines, presented in panels (a) and (b), respectively, with those obtained within the unified theory of Allard et al.[1] |
Moreover, Hager and his collaborator[5] have experimentally observed a satellite near the wavelength position 827 nm for Cs collisions with He at a high pressure of 2280 torr in the temperature range of 323–448 K. Figure
 | Fig. 7. Comparison of our computed photoabsorption profiles around the cesium D1 and D2 lines, presented in panels (a) and (b), respectively, at T = 323 K and T = 448 K, with those measured by Hager et al.[5] |
All these results confirm that our calculated photoabsorption spectra are in good agreement with experimental measurements and theoretical calculations, and illustrate in particular the sensitivity of the pressure broadening calculations to the quality of potentials and the accuracy transition dipole moments we have used.
The full quantum-theoretical photoemission profiles for both broadened D1 and D2 resonance lines are displayed in the wavelength interval from 800 nm to 1000 nm, at temperatures T = 323 K, 448 K, 500 K, 1000 K, 2000 K, and 3000 K. As a matter of first importance, one needs to underline as with the CsHe absorption profile calculations that only the free–free transitions contribute to building the D1 and D2 emission spectra. In particular, the shapes of the emission profiles of the broadened D1 line in the blue and red wings are constructed by 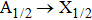
For the broadened D2 resonance line, the computations state that the 

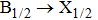
 | Fig. 9. Total reduced emission coefficients presented at temperatures ranging between 323 K and 3000 K. |
Very recently, Blank et al.[2] have used the classical quasistatic approximation, based on the 
To our knowledge, the only available experimental data of the CsHe emission profile is the normalized infinite-temperature emission spectra for 1019 cm−1 perturber density measured by Hedges et al.[8] To successfully reproduce the experimental spectrum at the infinite temperature 
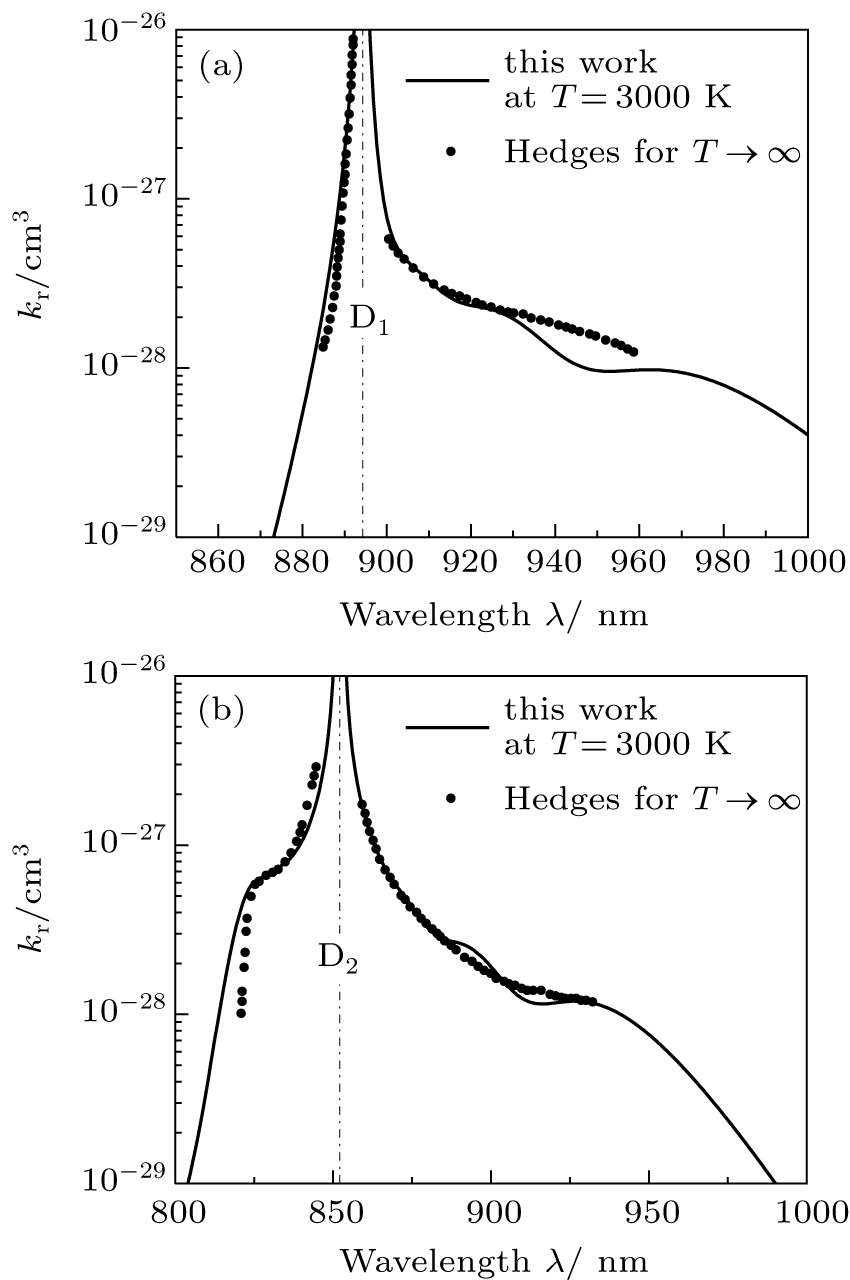 | Fig. 10. Comparison of our computed T = 3000 K photoemission profiles around the cesium D1 and D2 lines, presented in panels (a) and (b), respectively, with those measured at the infinite temperature by Hedges et al.[8] |
In this work, we have performed full quantum-mechanical calculations related to the CsHe absorption and emission profiles in the wavelength range of 800–1000 nm, at temperatures going from 323 K to 3000 K. For this purpose, we have computed accurate potential energy curves of ground 

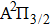

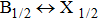
| [1] | |
| [2] | |
| [3] | |
| [4] | |
| [5] | |
| [6] | |
| [7] | |
| [8] | |
| [9] | |
| [10] | |
| [11] | |
| [12] | |
| [13] | |
| [14] | |
| [15] | |
| [16] | |
| [17] | |
| [18] | |
| [19] | |
| [20] | |
| [21] | |
| [22] | |
| [23] | |
| [24] | |
| [25] | |
| [26] | |
| [27] | |
| [28] | |
| [29] | |
| [30] | |
| [31] | |
| [32] | |
| [33] | |
| [34] | |
| [35] | |
| [36] | |
| [37] | |
| [38] | |
| [39] | |
| [40] | |
| [41] | |
| [42] | |
| [43] |