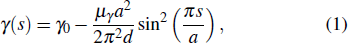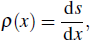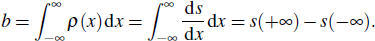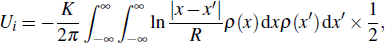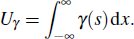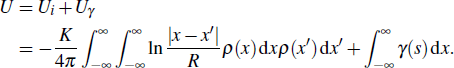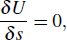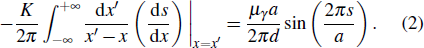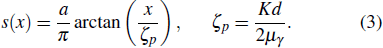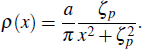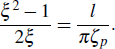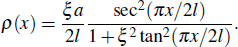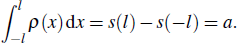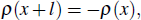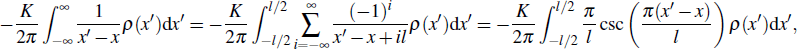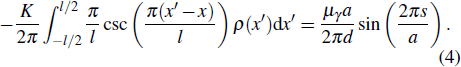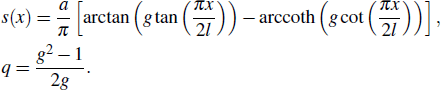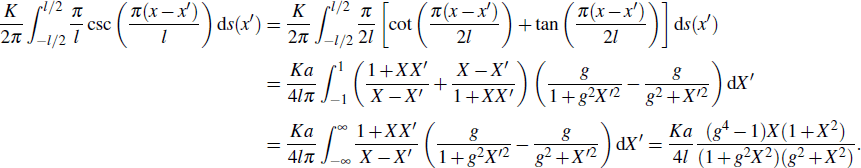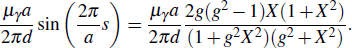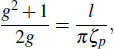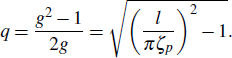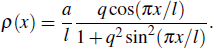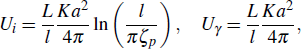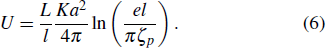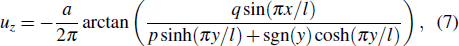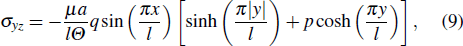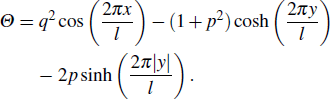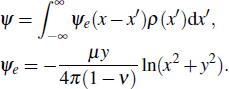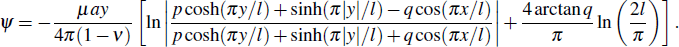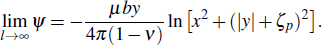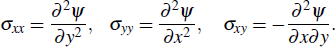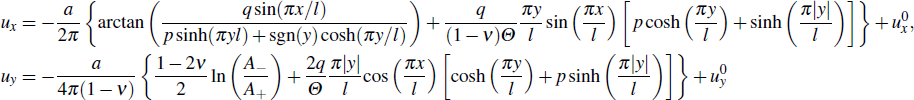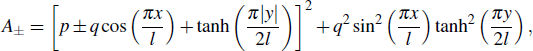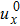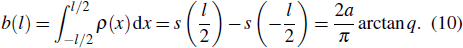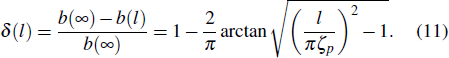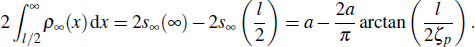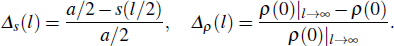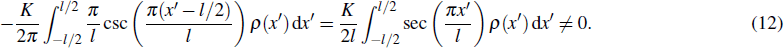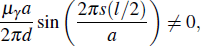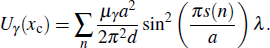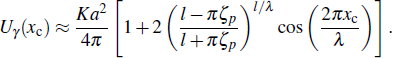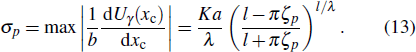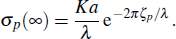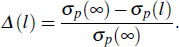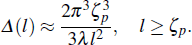1. IntroductionDislocation is a kind of topological defect characterized by Burgers vector b.[1] The Burgers vector b is a topology invariant (topology charge) that is similar to the electric charge in the electrics or the electric current in the magnetics. Due to discreteness of lattice, the Burgers vector of an isolated dislocation in crystals is quantized. For a given lattice, the minimum Burgers vectors are the primitive vectors of the lattice, and an arbitrary Burgers vector is an integer multiple of the minimum Burgers vector or a linear combining. Instead of a point-like defect, a dislocation possesses an extended structure, i.e., its topology charge does not concentrate at a point, but distributes extendedly. The major feature of the distribution of the topology charge (Burgers vector) can be well described by the Lorentz-type distribution, which has a characteristic length and a power-law asymptotic behavior inversely proportional to square of the distance. Usually, dislocations in materials are well-separated. The distance between the dislocations is so large that every dislocation can be suitably identified with an isolated dislocation and has a definite Burgers vector. However, in composite structures of dislocations such as dislocation dipoles and dislocation arrays, the distance between dislocations is comparable to the dislocation width. For example, the Stone–Wales defect existing in two-dimensional (2D) covalent materials is a dislocation dipole, in which the distance between the dislocation and the anti-dislocation is slightly larger than the width.[2–5] The dislocation array frequently used in the first principle calculation is a dislocation lattice in which the dislocation and the anti-dislocation aligned alternately and the distance is usually chosen to be about ten times the size of the width.[6–8] A natural question is what different when two dislocations approach each other. If the distance between the dislocations are not large enough, to what level the dislocation features including the dislocation interaction, dislocation response, etc. may be approximately invariant or otherwise may be quite different.[9] In particular, whether the topology feature of the dislocations changes as the distance between the dislocation is comparable to the dislocation width. In this paper, a self-organized one-dimensional array composed of dislocation and anti-dislocation is analytically studied in the frame of the Peierls theory.[10] From the exact solution of the Peierls equation, it is found that there exists strong neutralizing effect that makes the Burgers vector of each individual dislocation in the equilibrium array smaller than that of an isolated dislocation. Our results indicate that the topology feature of individual dislocation is not invariant in dislocation reactions while conservation of total Burgers vector is valid. For the dislocation dipole array frequently used in the first principle simulation, each individual dislocation in the array may be remarkably different from an isolated dislocation if the dislocations are not enough separated.
2. One-dimensional self-organized array of dislocationsA crystalline lattice can be viewed as a family of lattice planes which are parallel and equally spaced. A lattice plane family is usually labeled by their Miller index, or by the related reciprocal lattice vector. Dislocations are resulting from relative slip between lattice planes.[1] Let us divide a lattice into two symmetric halves by cutting lattice along a middle plane between two parallel lattice planes. If two halves have a rigid relative-slip along the cut plane, the energy changes periodically due to discreteness of the lattice. The energy per unit area as a function of the slip s is called generalized stacking fault (GSF) energy or the γ-surface, which is given in the leading term approximation[10,11]
where
γ0 is a constant,
a is period in the slip direction,
d is the space of the lattice planes,
μγ is a physical constant that is usually approximated to be the shear modulus.
If two halves have a spatially nonuniform relative-slip s(x) (slip field), dislocations appear as a result of the slip. It is Eshelby who recognized that the distribution of straight dislocations is identified with gradient of the slip field
the total Burgers vector is
The interaction energy (per unit length) of the distribution
ρ(
x) is
where
K is the energy factor,
K =
μ for the screw dislocation and
K =
μ/(1 −
ν) for the edge dislocation. If the slip field varies slowly, the interaction energy between two halves can be approximately given by
Thus, the total energy functional is
The equation of equilibrium distribution is obtained from the minimum energy principle
that is
This is the famous Peierls equation and it has an exact solution
[10]
The solution Eq. (
3) is called the Peierls solution that describes an isolated dislocation with a Lorentz-type distribution
The Peierls equation (
2) has periodic solution
[12,13]
where 2
l is the period, parameters
ξ describes the dislocation shape. The period
l and shape parameter
ξ satisfy the following equation
The density distribution is
This solution describes an array of dislocations and it is usually used in discussion of the misfit dislocations. The Burgers vector of each individual dislocation equals the period
a
It is significant to study a dislocation array composed of dislocation and anti-dislocation.
As illustrated in Fig. 1, for a periodic array in which dislocation and anti-dislocation aligned alternately, one has
where the length
l is the distance between the two neighboring dislocations. The period of the array is 2
l. By using the periodicity, the left-hand side of the Peierls Eq. (
2) can be written as follows:
and thus the Peierls equation becomes
Surprisingly, this equation has an exact solution
The key step to verify the solution is noting that the slip field can be decomposed into two terms
Introducing new variable
X = tan
πx/2
l, the left-hand side of Eq. (
4) is
and the right-hand side is
Therefore, the slip field equation (
5) is an exact solution provided that
or
The solution equation (
5) represents a self-organized structure in which dislocation and anti-dislocation are alternately aligned. It is interesting to see that the period
l has a lower limit. The minimum value is
l =
πζp. The dislocation density distribution is
In the limit,
l → ∞,
q →
l/(
πζp), and
q sin(
πx/
l) →
x/
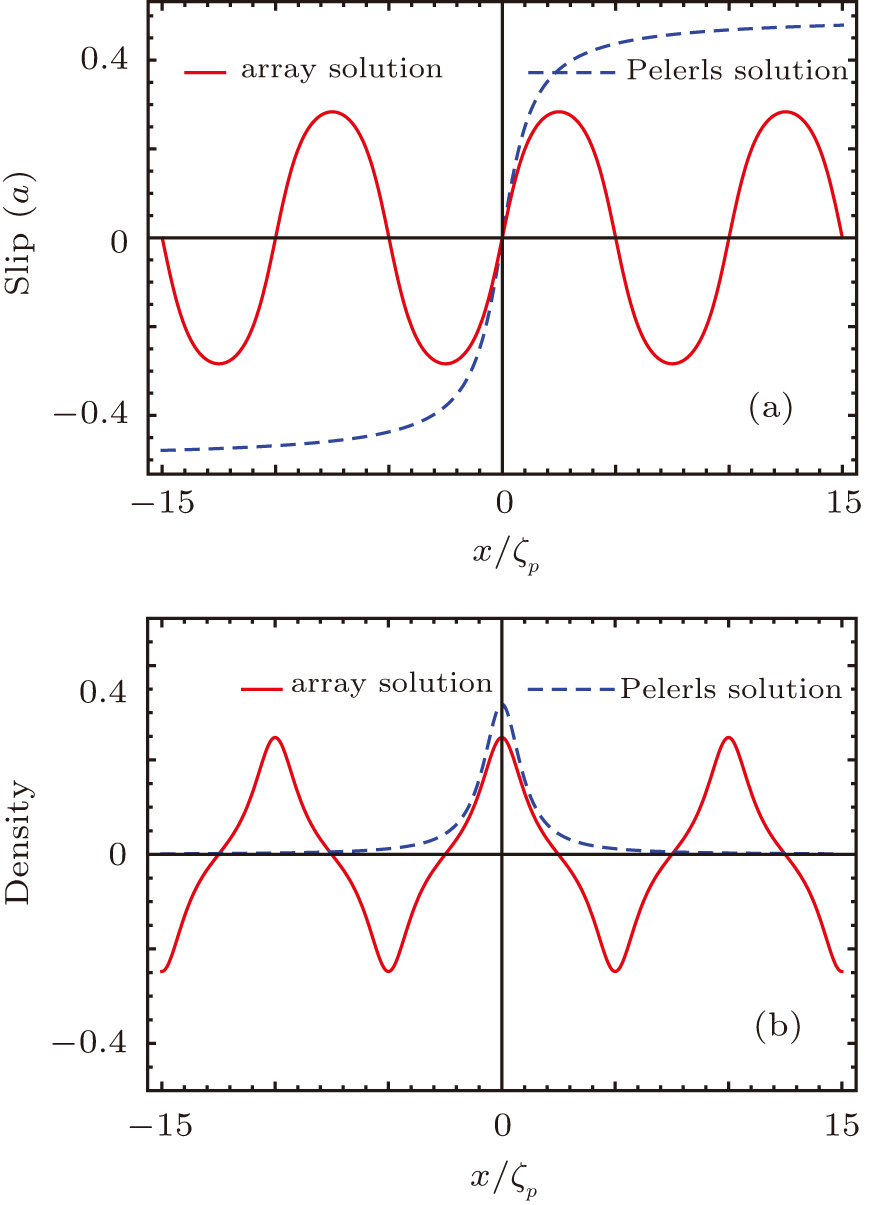
ζp, the new solution degenerates into the Peierls solution. In Fig.
2, the slip field and the density distributions are plotted for
l = 5
ζp. We see that the distribution in the region, −
l/2 <
x <
l/2, is remarkably different from that of an isolated dislocation.
For the solution Eq. (5), it is straightforward to evaluate the interaction energy and the misfit energy
where
L is the size of the array,
L/
l is the number of the dislocations in the array. The total energy is
The stress field and the displacement field can also be evaluated by using the superposition principle. For the screw dislocation, the displacement field is
where
p =
l/
πζp, and sgn(
y) is the sign function, sgn(
y) = 1 if
y > 0 and sgn(
y) = −1 if
y < 0. The stress field is
where
For the edge dislocation, the stress function can be obtained from the following integration
[1]
This integration has been carried out analytically for the self-organized array. The final expression of the stress function is
The stress function of an isolated dislocation is recovered in the limit
l → ∞,
The components of the stress field are given by
From the stress field, it is easy to obtain the strain field by using the Hook’s law. Furthermore, the displacement field can be evaluated by integration of the strain field. The explicit expression of the displacement field is
with
where
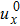
and
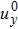
are integral constants.
3. Dislocation neutralizingThe self-organized structure presented in Section 2 is a periodic equilibrium-array in which dislocations and anti-dislocations are alternately aligned. It is observed that the Burgers vector of each individual dislocation in the array does not equal the minimum lattice vector. The Burgers vector of each individual dislocation is
It varies continuously in the range 0 <
b ≤
a. Therefore, in a self-organized equilibrium-array composed of dislocation and anti-dislocation, Burgers vector of the dislocation is not an integer times of the minimum lattice vector. There is neutralizing effect for two neighboring dislocation and anti-dislocation. The neutralizing effect makes the Burgers vector of a dislocation smaller than that of an isolated dislocation.
In order to measure the neutralizing effect, let us introduce a dimensionless factor
Obviously, the factor
δ is the fraction of the Burgers vector that is neutralized. For the distance
l = 10
ζp, it is reasonable to suppose that the two neighboring dislocations are well separated. However, it is found that,
δ ≈ 20%, about twenty percent Burgers vector is neutralized. Actually, for large
l,
δ ≈ 2
ζp/
l, the factor
δ falls off slowly. We see that there are strong neutralizing effect between the dislocation and the anti-dislocation. The strong neutralizing effect can be attributed to the structure feature of a dislocation. Although a dislocation has a core characterized by the width
ζp, it also has a long tail that falls off inversely proportional to square of the distance. The power-law asymptotic behavior enables two dislocations interfere even though they are well separated.
For the large period l ≫ ζp, the core of an individual dislocation in the array is nearly the same as that of an isolated dislocation. In fact, if the period is large enough, the density distribution is almost coincided with that of an isolated dislocation in its own region, ρ(x) ≈ ρ∞(x) for −l/2≤ x ≤ l/2. The major difference is that the tail of an isolated dislocation is cut at the position x = l/2. In a sense, a well separated array can be viewed as a chain composed of tail-cutting dislocations and anti-dislocations. The Burger vector in the tail of an isolated dislocation is
The dimensionless factor
δ estimated in this way is
δ ∼ 4
ζp/
πl. This result is similar to that given by Eq. (
11) provided
l ≫
ζp. Although a dislocation may have a quite different core in different materials, it has a universal asymptotic-behavior that is the same as that given by the Peierls solution. Therefore, the neutralizing effect is almost independent of the detail of the core structures of dislocations for large distance
l ≫
ζp. Usually, the characteristic width of an isolated dislocation is the same order of the lattice distance
ζp ∼
a. As a consequence, the separating distance between dislocation and anti-dislocation should be larger than 20
a to make the neutralizing effect negligible.
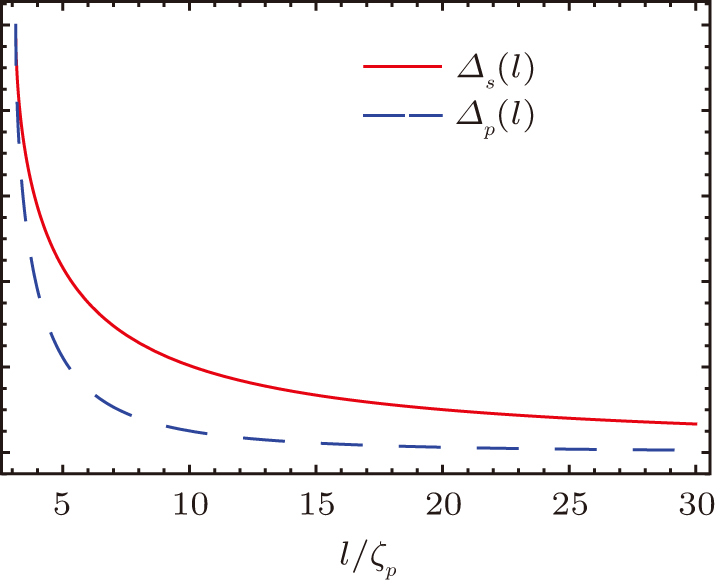
It is also observed that the maximum slip and the maximum density of an individual dislocation in the array are different from those of an isolated dislocation. Introducing the relative deviations of maximum slip value Δs and density value Δρ
as shown in Fig.
3, the deviations
Δs and
Δρ are large when the distance
l becomes small.
It is necessary to point out that at the middle point between two neighboring dislocation and anti-dislocation, the density is zero, but the slip is nonzero. In fact, for x = l/2, the left-hand side of Eq. (4) is
Therefore, the right-hand side of this equation must also be nonzero
i.e.,
s(
l/2) ≠ 0 and
s(
l/2) ≠
a/2. In conclusion, there is always a nonzero slip at the middle point between two neighboring dislocation and anti-dislocation.
In the self-organized dislocation array, the center of each individual dislocation, which is defined by the maximum point of the density distribution, locates at the unstable position at which the γ-surface takes maximum. Furthermore, due to discreteness of lattice there actually exists a periodic potential called the Peierls potential. If the distance between two neighboring dislocation and anti-dislocation is integer times of the intrinsic period λ of the lattice, l = nλ, (n = 1,2,3,…), and every dislocation locates at the valley bottom of the potential, the stability of the equilibrium array can be guaranteed by the Peierls barrier.
It is usually assumed that the continuous envelope is always identical with the solution of the Peierls equation when the dislocation is moved to new position xc.[14] Under this rigid dislocation assumption, the discrete slip field for moved dislocation array can be obtained by s(n) = s(x − xc)|x = nλ. The Peierls potential energy related to the γ-surface is given by the following expression
By using the Poison sum formula, we can obtain
The Peierls stress
σp is the critical stress given by
[1,10]
In the limit
l → ∞, the well-known formula is recovered as
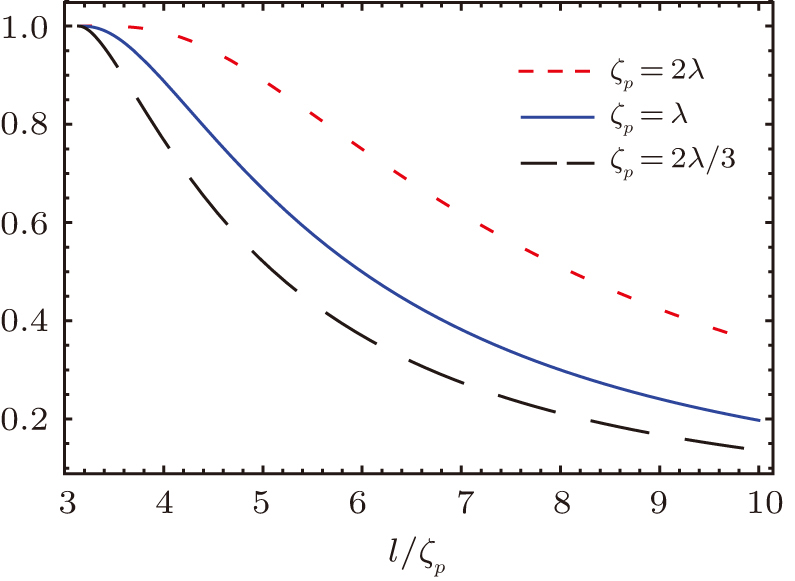
In order to measure the influence of the finite distance
l, it is helpful to introduce relative deviation of the Peierls stress
In Fig.
4, the relative deviation is plotted as a function of the period. We see that the wider dislocation is, the larger deviation. The relative deviation falls off as the period increases. For
l = 10
ζp the relative deviation is about 20% (
λ ∼
ζp) which approximately equals the deviation in the Burgers vector,
Δ ≈
δ. The asymptotical behavior is given by
4. Summary and discussionA 1D self-organized array composed of dislocation and anti-dislocation is analytically investigated in the frame of Peierls theory. A new exact solution of the Peierls equation is obtained, which describes the equilibrium structure of the dislocation array. If the distance between the two neighboring dislocation and anti-dislocation is integer times of the intrinsic period of the lattice, i.e., the periodicity of this array is commensurate with the lattice periodicity, the stability of the equilibrium array can be guaranteed by the Peierls barrier. The energy of the array is simply a logarithmic function of the period that has a lower-limit l ≫ πζp. The stress function and the displacement field are presented explicitly.
From the exact solution of the Peierls equation, it is found that there exists strong neutralizing effect that makes the Burgers vector of each individual dislocation in the equilibrium array smaller than that of an isolated dislocation. This neutralizing effect is not negligible even though dislocations are well separated. The neutralizing effect originates physically from the power-law asymptotic behavior that enables two dislocations interfere even though they are well separated. For the dislocations in real solids, the sinusoidal law used in the Peierls equation should be replaced by the generalized stacking fault energy and the lattice discreteness should be taken into account.[15–17] As a result, details of the above theoretical conclusions may be modified in some ways. However, it is rational to believe that the underlying physics will be maintained. In particular, although a dislocation may have a quite different core in different materials, it has a universal asymptotic-behavior that is the same as that given by the Peierls solution. Therefore, the neutralizing effect is almost independent of the detail of the core structures of dislocations for large distance l ≫ ζp. The neutralizing effect originated from the long-range feature of the elastic field will play significant role when the dislocation and the anti-dislocation are involved.
As is well known, the long-range feature of the dislocations makes it difficult to simulate the dislocation precisely. Usually, instead of a single dislocation, an array composed of dislocation and anti-dislocation is used in the ab initio simulation to study the properties of an isolated dislocation. Our theoretical results are useful in constructing the supercell and helpful in explanation of the data obtained from numerical simulations. The stability of the self-organized dislocation array critically depends on the height of the Peierls barrier of the material. For materials with low Peierls barrier, the self-organized dislocation array can only exist at low temperature. For materials with strong covalent bond, the Peierls barrier is usually high and the self-organized dislocation array can stably exist even at the room temperature. For instance, in graphene, the self-organized dislocation array (flower defect) had been reported by Lehtinen et al.[18] Naturally, appearance of the dislocation array will alter the physical and chemical properties of materials.