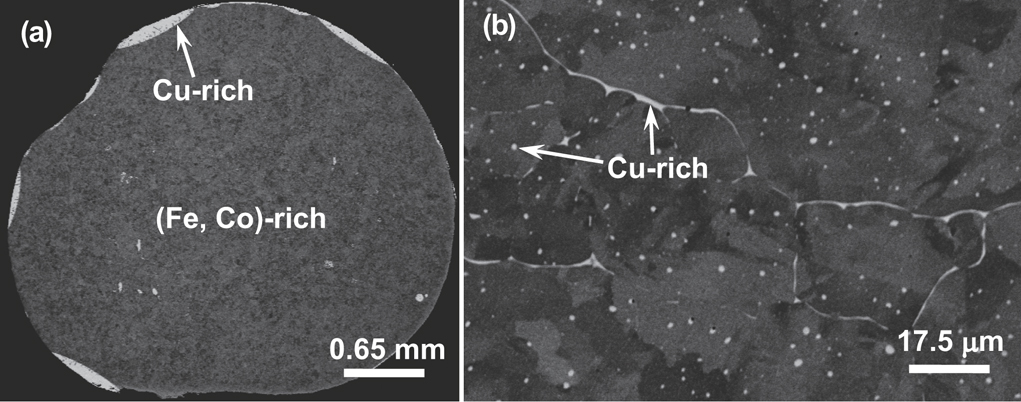
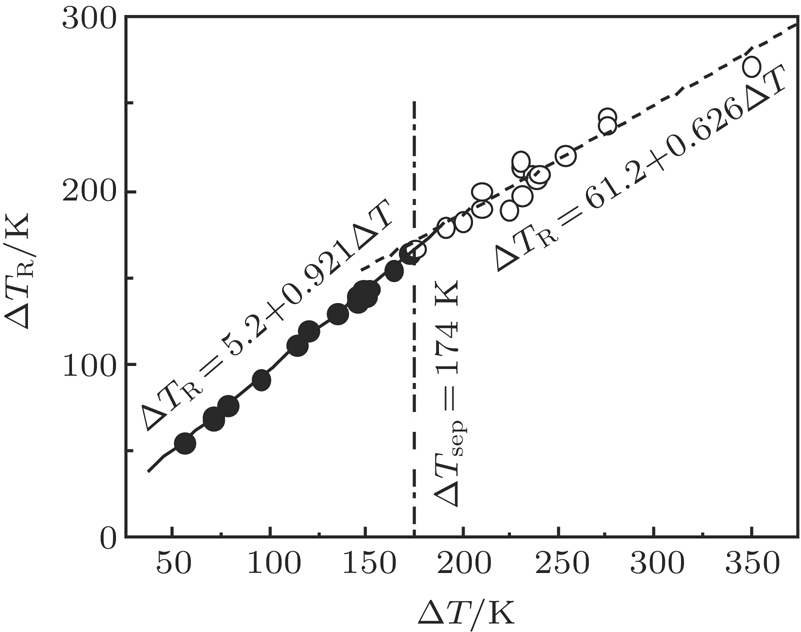
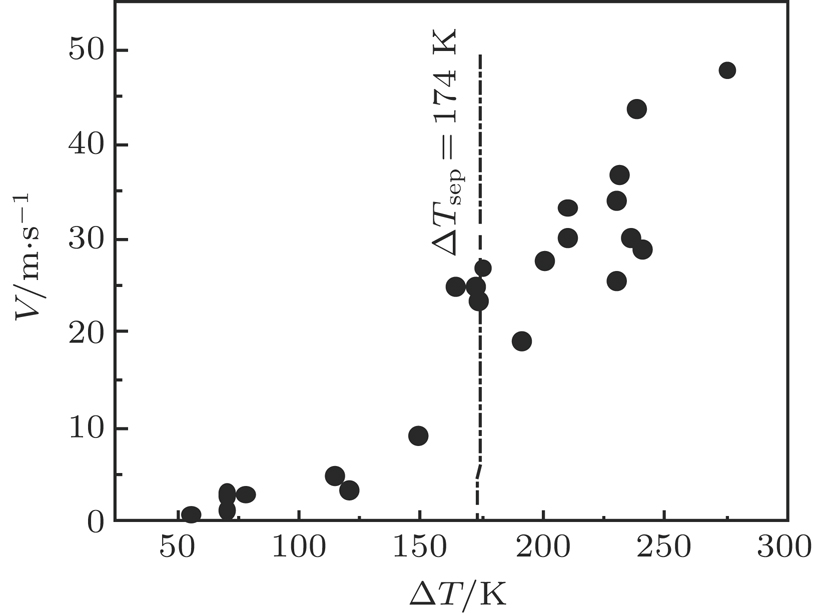
† Corresponding author. E-mail:
Project supported by the National Key Research and Development Program of China (Grant No. 2016YFB1100101), the National Natural Science Foundation of China (Grant No. 51471135), Shenzhen Science and Technology Program, China (Grant No. JCYJ20170815162201821), Shaanxi Provincial Key R&D Program, China (Grant No. 2017KW-ZD-07), and the Fundamental Research Funds for the Central Universities, China (Grant No. 31020170QD102).
The metastable liquid phase separation and rapid solidification behaviors of Co40Fe40Cu20 alloy were investigated by using differential thermal analysis (DTA) in combination with glass fluxing and electromagnetic levitation (EML) techniques. The critical liquid phase separation undercooling for this alloy was determined by DTA to be 174 K. Macrosegregation morphologies are formed in the bulk samples processed by both DTA and EML. It is revealed that undercooling level, cooling rate, convection, and surface tension difference between the two separated phases play a dominant role in the coalescence and segregation of the separated phases. The growth velocity of the (Fe,Co) dendrite has been measured as a function of undercooling up to 275 K. The temperature rise resulting from recalescence increases linearly with the increase of undercooling because of the enhancement of recalescence. The slope change of the recalescence temperature rise versus undercooling at the critical undercooling also implies the occurrence of liquid demixing.
The liquid phase separation phenomena have been extensively studied[1–5] and their significant effect on structures and properties of various materials have been revealed.[6–13] As the great potential application of high entropy alloys and bulk metallic glasses, liquid phase separation is attracting more and more attention.[14,15] It is interesting that some peritectic and eutectic alloys characterized by a large positive enthalpy of mixing and a nearly flat liquidus curve show a miscibility gap in the metastable undercooled liquid, such as Co–Cu[16] and Fe–Cu.[17] The metastable liquid phase separation behaviors of the Co–Cu and Fe–Cu systems have been intensively investigated. It is found that the critical temperature of the miscibility gap of the Fe–Cu system is 120 K higher than that of the Co–Cu system.[18,19] When a third component is added into the above binary alloys characterized by liquid phase separation in the undercooled state, the resulting ternary alloy system also shows a metastable miscibility gap over a certain composition range. Much work has been done on the phase diagram and solidification behaviors of the Cu–Co–Fe ternary alloy system. Jellingahus[20] and Maddocks and Claussen[21] first investigated vertical sections of the phase diagram of Cu–Co–Fe ternary alloy system using thermal analysis and metallography, but they did not observe immiscibility behavior in this ternary system. Isothermal sections of the phase diagram at several temperatures have been obtained.[22] Munitz et al.[23] observed liquid phase separation in Cu–Co–Fe alloys containing 52–79 at.% Cu at high cooling rates. Kim et al.[24] measured the liquidus and demixing temperatures of some Cu–Co–Fe alloys by means of a pyrometer in the electromagnetic levitation state. Bamberger et al.[25] evaluated the stable and metastable Cu–Co–Fe phase diagrams. Wang et al.[26] calculated the phase equilibria in Fe–Cu–X (X: Co, Cr, Si, V) ternary systems and obtained the miscibility gaps theoretically. Applying high temperature differential thermal analysis technique, Cao and Görler[27] precisely determined the liquidus and the miscibility gap over a wide composition range for this system. The boundary lines of the miscibility gap, which are determined for the three quasi-binary cross-sections of the Cu–(Fe,Co) alloy system, show remarkably flat domes. It is found that the directly determined miscibility gap boundary is quasi-binary at a given Cu concentration, which is located between the corresponding binodals of the boundary systems Cu–Co and Cu–Fe. The liquid phase separation temperature determined directly and reproducibly from the onset temperature of the differential thermal analysis (DTA) traces, decreases monotonically with the increase of the Co content. Munitz et al.[28] used electromagnetic levitation to undercool the alloys and determined the metastable phase separation temperatures by analyzing the compositions of the two separated liquids by EDS after solidification. Curiotto et al.[29] measured the demixing temperatures of several selected Cu–Co–Fe alloys with different compositions by differential scanning calorimetry. Zhao et al.[30] investigated the solidification behavior of gas-atomized powders of a Cu–Co–Fe alloy.
Although the immiscibility of Cu–Co–Fe ternary system can be approximately treated as a Cu–(Fe, Co) quasi-binary system according to previous studies, the liquid phase separation and solidification behaviors are significantly dependent on the Cu–Co and Cu–Fe binary systems for the ternary alloys with high content of (Fe,Co). The phase separation product compositions and microstructures under different conditions are more complicated. It is very important to explore the formation of the minor liquid phase at the initial stage of separation and subsequent coalescence and migration processes. Is there any nucleation or is the process just a spinodal decomposition? How can the two phases exchange the three chemical elements so rapidly? These processes involve complex thermodynamics, kinetics, atomic diffusion, fluid migration, and convection. So far, however, little quantitative information has been available on the metastable liquid phase separation and rapid solidification of Fe–Co–Cu alloys with high (Fe,Co) concentration under different conditions, such as cooling rate, undercooling level, and container. Especially, no work has reported quantitative measurement of (Fe,Co) dendrite growth velocity at various undercooling levels.
In the present work, we employed differential thermal analysis method in combination with glass fluxing technique to characterize the metastable phase transformation of Co40Fe40Cu20 ternary alloy. The dendritic growth velocity of (Fe,Co) phase was measured as a function of undercooling by the electromagnetic levitation technique. The effect of undercooling level, cooling rate, convection, surface tension difference, and container state on the microstructure formation of Co40Fe40Cu20 alloy has been demonstrated.
The alloy samples were prepared by alloying highly pure Cu (99.999%), Co (99.998%), and Fe (99.99%) in an arc-melting furnace. They were remelted several times to achieve sufficient homogeneity. The samples were within 0.25–0.35 g in mass for DTA measurement and 1–1.2 g for electromagnetic levitation. For the DTA measurement, each sample was contained in an alumina crucible covered with crushed Duran glass as a denucleant and fluxing agent and was then placed in a differential thermal analyzer (Netzsch DTA 404 S). The sample was heated up to a temperature between 1700 K and 1800 K. After an isothermal period of 10 min, the sample was cooled at a constant rate down to 600 K. 2–4 heating and cooling cycles were performed for each sample at rates of +10 K/min and −20 K/min, respectively. Soak periods of 10 min at the maximum temperature were inserted to recover homogeneity of the melt after liquid demixing and macroscopic phase separation. The melting points of pure Ag, pure Cu, and pure Ni were used to calibrate the temperature scale of the facility to an accuracy of better than ±1.5 K.
The rapid solidification and dendritic growth velocity measurement at various undercooling levels were accomplished by using the electromagnetic levitation technique. The chamber of the EML facility was evacuated to better than 5.0 × 10−6 Pa and then backfilled with a He–8 vol.% H2 mixture. The specimen was heated above the liquidus temperature and subsequently cooled by a He–8 vol.% H2 gas flow. A two-color pyrometer with a time resolution of 10 μs was employed to measure the temperature of the sample from the top throughout the experiment with a relative accuracy of ±3 K at a sampling rate of 100 Hz, where the emissivity was calibrated to reproduce the liquidus temperature. At the desired undercooling, solidification was started by triggering the lower end of the levitated droplet with the boron nitride sample holder. The recalescence time during solidification was measured by a specially designed fast responding photodiode infrared device, where the two photodiodes were put to be parallel to the vertical axis of the sample. Both the pyrometer and photodiode signals were simultaneously recorded by a transient-signal memory recorder. The sampling rate used for the transient recorder was 1 MHz. The dendritic growth velocity can be obtained on the basis of the photodiode signals.[31] The phase constitutions of the solidified samples were examined by an X’pert Philips x-ray diffractometer using a Cu Kα radiation. The structures and concentration profiles were analyzed by means of a ZEISS SUPRA55 field emission scanning electron microscope (SEM) with an INCA E350 energy dispersive spectrometer under accelerating voltage of 15 kV.
Figure
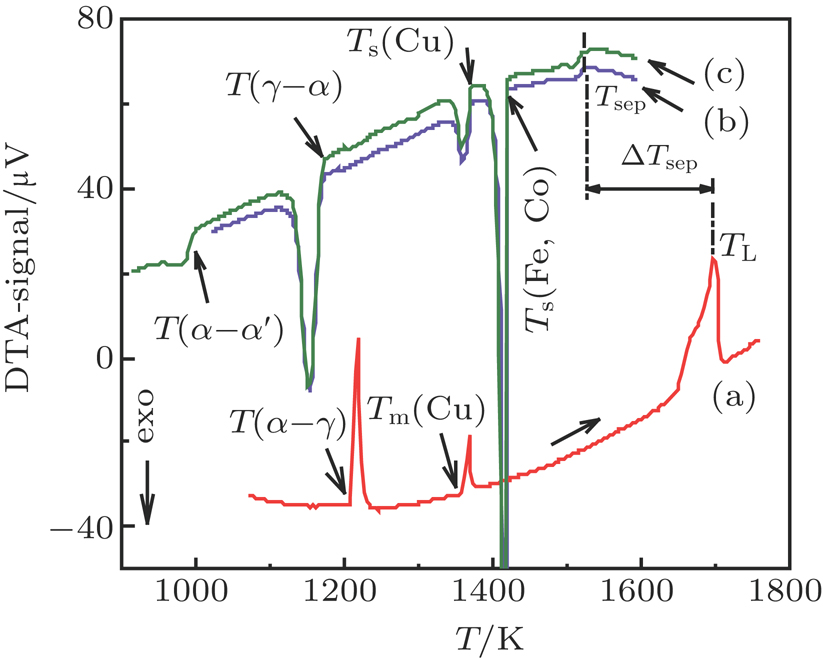 | Fig. 1. (color online) DTA traces of heating and cooling for a Co40Fe40Cu20 sample: (a) one heating segment; (b) and (c) two cooling segments. |
Figure
By using EML technique, undercooling up to 345 K has been achieved for Co40Fe40Cu20 alloy. Figure
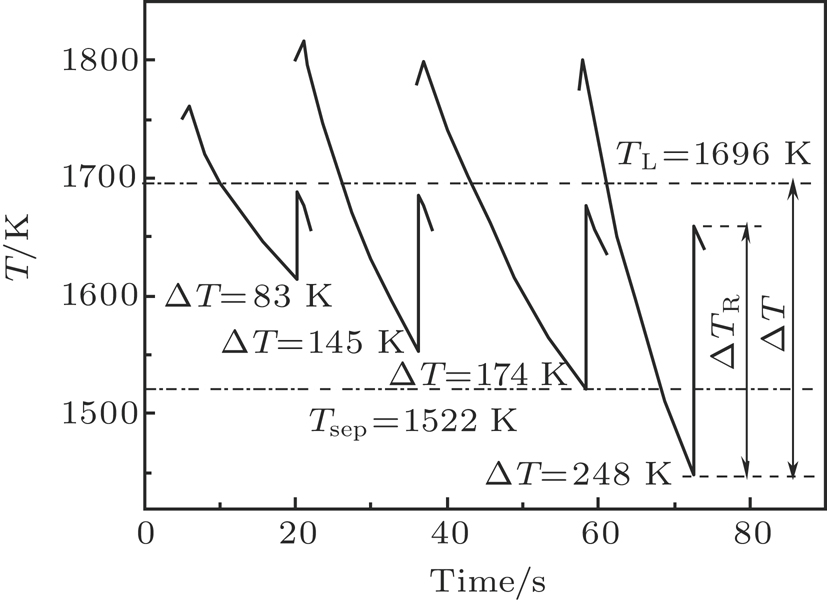 | Fig. 3. Illustration of recorded recalescence events of Co40Fe40Cu20 alloy from the pyrometer signals during electromagnetic levitation processing. |
The x-ray diffraction patterns of Co40Fe40Cu20 samples solidified during DTA measurement and EML processing are illustrated in Fig.
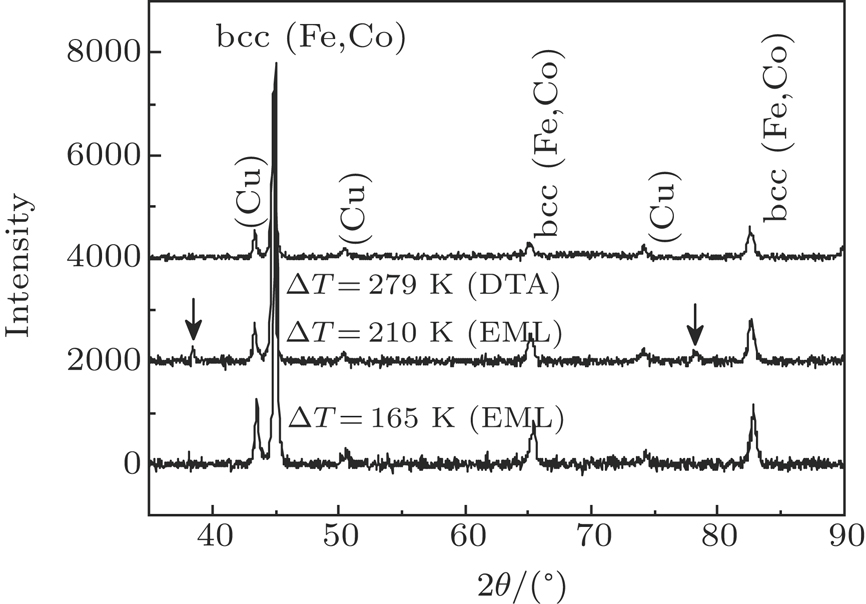 | Fig. 5. X-ray diffraction patterns of Co40Fe40Cu20 samples solidified during DTA measurement and EML processing. |
Figure
Experimental measurement of dendritic growth velocity of (Fe,Co) phase in Co40Cu20Fe40 alloys has been carried out at different undercoolings. The dendritic growth velocity, V, as a function of undercooling is shown in Fig.
The metastable phase separation and rapid solidification of undercooled Co40Fe40Cu20 alloy have been studied by differential thermal analysis and electromagnetic levitation containerless processing. Only bcc α−(Fe,Co) and fcc (Cu) phases are formed. It is found that the undercooling level, cooling rate, convection, and surface tension difference between the two separated phases play a dominant role in the coalescence and segregation of the separated phases. Enhanced undercooling, low cooling rate, and forced convection have the effect of facilitating the metastable phase separation and coalescence process, and the difference in surface tension of the two separated liquids leads to surface segregation of Cu-rich phase in DTA measurements. The dendritic growth velocity of (Fe,Co) phase has been measured as a function of undercooling up to 275 K. The temperature rise resulting from recalescence increases linearly with the increase of undercooling because of the enhancement of recalescence.
| [1] | |
| [2] | |
| [3] | |
| [4] | |
| [5] | |
| [6] | |
| [7] | |
| [8] | |
| [9] | |
| [10] | |
| [11] | |
| [12] | |
| [13] | |
| [14] | |
| [15] | |
| [16] | |
| [17] | |
| [18] | |
| [19] | |
| [20] | |
| [21] | |
| [22] | |
| [23] | |
| [24] | |
| [25] | |
| [26] | |
| [27] | |
| [28] | |
| [29] | |
| [30] | |
| [31] | |
| [32] |