After the pioneering work of Ikezi,^[1] the studies on the propagation characteristics of dust acoustic (DA) waves in strongly coupled dusty plasmas have received significant attention due to their fascinating aspects from practical points of view.^[1–5] Depending on the coupling strength of dust grains, dusty plasma may be identified in strongly (Γ ⩾ 1)^[1–16] or weakly (Γ ≪ 1)^[17–31] coupled regimes (with Γ being the coupling parameter defined by , where q_d = eZ_d is the dust grain charge, a_d is the inter-particle distance, T_d is the dust kinetic temperature, and λ_d is the Debye length). The existence of such weakly and strongly coupled regime in dusty plasma has been theoretically predicted by Ikezi.^[1] Furthermore, the strong interaction among highly charged massive micron sized dust grains in a classical Coulomb plasma which was predicted by Ikezi^[1] has been verified experimentally^[2,3] and with numerical simulation.^[5]

The physics of strongly coupled plasma is of considerable interest both from practical and theoretical points of views due to its applications in different physical situations, i.e., in the interior of heavy planets, e.g., the neutron stars, the white dwarf matter, in nuclear explosions, in laser plasma, in the semiconductor heterojunctions, and in non-ideal plasmas.^[9] It has been shown by a number of authors that strongly coupled dusty plasmas support many important physical phenomena. For instance, the phase transition, the crystallization, the noise induced tunneling between different dust-cluster configurations, etc.^[32] Also, strongly coupled dusty plasmas may be found in any of crystalline, fluid, and/or colloidal plasma states.^[1]

Though the viscosity and elasticity have no significant effects on weakly coupled plasma (Γ ≪ 1), however, in the strongly coupled regime (1 ⩽ Γ), the strong effects of viscosity appear by increasing the coupling parameter Γ. When the phase transition of plasmas from liquid to solid phase occurs,the viscosity property would be replaced by the elastic property. In the strongly coupled plasma, the strong correlation among the dust grains modifies the dust associated waves in dusty plasma systems.^[9,33,34] Kaw and Sen^[9] showed that the elastic property induced by the dust grains can lead to the formation of a new transverse shear-like mode.

It must be added that the physical properties of DA waves in a weakly coupled dusty plasma is typically different from those in presence of strong correlation among dust grains. In the weakly coupled regime, the restoring force comes from the thermal pressures of electrons and ions, while in a strongly coupled regime, it appears to be from the shielded Coulomb force between the nearest neighboring dust grains. Melandsø^[7] has theoretically predicted the occurrence of the DA waves in a strongly coupled dusty plasma system. As Γ increases in the vicinity of Γ_c, another group of the DA waves can propagate.^[11,15] The formation of DA waves in the presence of strong correlation among the dust grains has been examined experimentally by Pieper and Goree.^[35] They observed that the DAlike structures have shown no resemblance to the dust lattice waves.^[35] On the other hand, some previous investigations in a magnetized strongly coupled dusty plasma reported that the magnetic field significantly affects the basic features of those plasmas, i.e., in the magnetized target fusion scenarios,^[36,37] in the neutron star crusts, and in the white dwarf stars.^[38,39] The transition from weak to strong coupling regimes may significantly affect not only the dusty plasma configuration, but also the collective features. The previous investigations^[15,40,41] in strongly coupled dusty plasmas have indicated how the strong correlations as well as the magnetic field significantly modify the basic features of collective modes. Thus, it seems to be interesting to investigate the influence of strong correlations and external magnetic field on the dielectric response function as well as on the collective modes in dusty plasmas. Xie and Chen^[32] generalized the work of Kaw and Sen^[9] to the case of magnetized dusty plasma. Employing the fluid model,^[39] we^[15] have studied the linear and nonlinear propagation of dust acoustic waves in a magnetized strongly coupled dusty plasma. We found that the strong correlation of charged dust grains modifies both the existing dust acoustic and dust-cyclotron modes in magnetized dusty plasmas.^[15]

There are several models^{[9–11,15,32]} to study the dynamics of a strongly correlated plasma, i.e., the generalized hydrodynamic model,^[9,10,18] the quasilocalized charge approximation,^[42–44] the local field corrections method,^[45] the thermodynamic,^[46] and the fluid^[11,15] models. However, we employ the generalized hydrodynamic (GH) model that includes the effects of strong correlation via the visco-elastic coefficients. The GH model is valid over a broad range of the coupling parameter Γ, from the weakly coupled (Γ ≪ 1) to the strongly coupled (1 < Γ < Γ_c, where Γ_c is the critical coupling parameter at which the crystallization phase occurs).^[9] In this work, the GH model is employed to investigate the influence of strong interparticle interaction, dust kinematic viscosity, obliqueness, and external magnetic field on the dielectric response function as well as the wave propagation in magnetized strongly coupled dusty plasmas.

The manuscript is organized as follows. After the introduction, the governing equations are described and the dielectric response function is delivered in Section 2. The propagation properties of longitudinal waves are studied and numerically analyzed in Section 3. The transverse shear mode is also obtained in Section 4. A brief discussion is provided in Section 5.

We first obtain an expression for the dielectric permeability in the strongly coupled dusty magnetoplasma (SCDMP) composed of Boltzmann distributed ions and electrons n_e(i) = n_e(i)0 exp (±eϕ/T_e(i)), where n_e(i)0, T_e(i), and ϕ are the equilibrium electron (ion) number density, the electron (ion) temperature (in energy unit), and the electrostatic wave potential, respectively. Then we employ the GH model to describe the dynamical behaviors of dust grains as follows:

The aforementioned equation is the original result of the present work, and exhibits the longitudinal part of the dielectric response function, including the effects of the external magnetic field as well as the obliqueness of the wave in strongly coupled magnetized dusty plasma. This expression shows how the external magnetic field and the direction of the wave propagation affect the dielectric response function. Equation (5) recovers the previous results in the limiting cases. In the absence of the external magnetic field, equation (5) produces the result of Kaw and Sen.^[9] In the weakly coupled regime, our result is in good agreement with that reported by Nambu and Nitta^[46] for higher frequency waves. In order to check our results numerically, we examine the dispersion characteristics of longitudinal and transverse waves in the SCDMP model under consideration in Section 3.

In order to obtain the dispersion relation of dust associated electrostatic waves in the SCDMP system, we set ε(ω,k) = 0. Note that different values of τ_m can refer to different configurations, as τ_m → 0 is in accordance with a usual fluid with η showing its viscosity characteristics, while τ_m → ∞ is in relevance with a solid-like configuration. Here, we consider a wide range of τ_m as 0 < τ_m < ∞, which shows a transition from usual fluid toward a viscous configuration.^[9] To do so, we restrict our attention to the dispersion relation in the limits of ωτ_m ≪ 1 (hydrodynamic regime) and ωτ_m ≫ 1 (kinetic regime).

Popel et al.^[47] discussed the differences of the kinetic and hydrodynamic regimes, and classified the dissipative processes to determine the ranges of plasma parameters in which some particular processes dominate. The basic requirements for distinguishing the viscous configuration under the laboratory conditions were discussed in Ref. [48]. Obviously, there exist many possible parameters on which, in particular, the viscous configuration depends, such as the grain size, the kinetic temperature, the inter-grain distance, etc.

3.1. Hydrodynamics regime

In this limit, we assume ωτ_m ≪ 1, and the reduced form of the dispersion relation can be obtained from Eq. (5) as follows:

The above dispersion relation includes the combined effects of external magnetic field, obliqueness, and strong correlation between dust grains. It extends the previous results^[9,18] to the case of magnetized strongly coupled dusty plasma situations. Now let ω → ω + iδ in the dispersion relation and assume δ ≪ ω, we obtain the real and imaginary parts of the wave frequency as

Equations (7a) and (7b) describe the real and imaginary parts of the oblique DA wave frequency in SCDMP system. To check the behavior of the wave frequency in extent conditions, we see that by ignoring η in Eq. (7a), it reduces to

Equation (8) describes the coupling of obliquely propagating magnetized DA and obliquely propagating dust-cyclotron (DC) waves, which is similar to that obtained by Ref. [26]. In order to decouple these two modes, one can consider the propagation in parallel and perpendicular directions separately, as and describe the DA and DC modes, respectively. The former equation is in good accordance with that obtained by Piel and Goree^[32] and the latter one is similar to the result of Shukla and Rahman.^[30] On the other hand, in the unmagnetized limit, Eqs. (7a) and (7b) reveal the results of Kaw and Sen^[9] as δ → −ηk²/2Mn₀ and . After some straightforward manipulation, the dispersion relation in Eq. (7a) takes the following form:

where and . For the case of η → 0, the wave damping disappears, and thus equation (9) describes two DA and DC branches in a usual magnetized dusty plasma. The typical and approximate values of the transport coefficients are given in Ref. [49], in which the normalized shear viscosity η* = η/Mn₀ω_pda², where a = (4πn₀/3)^−1/3 is the Wigner–Seitz radius, has typical values η* ≈ 1.04 for Γ = 1, η* ≈ 0.08 for Γ = 10, and η* ≈ 0.3 for Γ = 160. Moreover, similar approximate estimations may be derived from the MD simulations. For instance, Xie and Chen^[42] have shown that one can obtain from Table V and Fig. 33 of Ref. [49] the functional relation of for the range 10 ⩽ Γ ⩽ 200. The excess internal energy of the system has also been estimated for 10 ⩽ Γ ⩽ 200 as u(Γ) ≅ −0.90Γ + 0.95Γ^1/4 + 0.19Γ^−1/4 − 0.81^[50,51] and for Γ < 1 as .^[52] Therefore, the above expressions can be used to estimate the relaxation time and the compressibility μ_d = 1 + u(Γ)/3 + Γ∂_#x0393;u(Γ)/9 in different coupling regimes. Considering such dependence on the coupling parameter Γ, it is easy to find that in Eq. (7) the coupling parameter Γ has appeared with a negative sign. It turns out that the strong correlation leads to wave frequency reduction, and the increasing effect of strong correlation on the viscosity term η makes the wave frequency purely damped at higher values of Γ. It may even lead to the change of sign of the group velocity, ∂ω/∂k, in strongly coupled regime. This shows that by increasing the coupling parameter, the hydrodynamic mode stops propagating because of the change in sign of μ_d at high values of Γ.^[9]

3.2. Kinetic regime

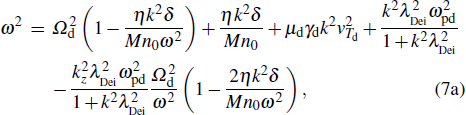
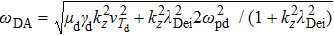
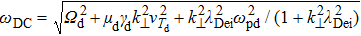
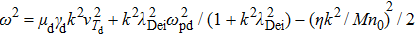
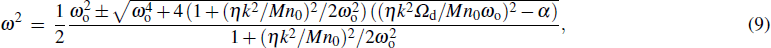
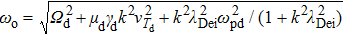
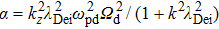
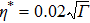
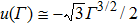
The kinetic regime refers to where the dusty plasma exhibits viscous liquid/solid like behaviors. However, this regime is valid only in the very strong coupling regime or in the weak coupling regime where the condition ωτ_m ≫ 1 can be fulfilled. Equation (5) in the limit of ωτ_m ≫ 1 may be written as

The above dispersion relation can be analyzed to derive the appropriate expression for the two existing branches as

In the absence of η, equations (9) and (11) have exactly a similar form. The typical and approximate values of the normalized relaxation time may take the approximate values for Γ = 1, for Γ = 10, and for Γ = 160. For the range 10 ⩽ Γ ⩽ 200, one can obtain an estimation for as .^[32]

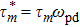
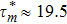
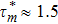
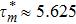
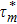
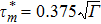
In the laboratory strongly coupled dusty plasmas,^[53,54] the typical parameters of dust densities and temperatures are respectively given by ⋅10⁵ cm⁻³ and ∼3–5 eV, which yield the coupling parameter Γ in magnitude from 1 up to 50, for which then typical values of the external magnetic field provide the large dust Larmor radii, therefore the dust grains almost remain unmagnetized. On the other hand, for the high-density astrophysical plasma medium with a wide range of the magnetic field strengths, such as those occurring in white dwarfs and neutron stars, the dust grains can be magnetized and the generalized hydrodynamic approach incorporating the contributions of the viscosity effects and magnetic fields can be employed as an appropriate theoretical model for investigation of relevant modes in an SCDPM system. Merlino et al.^[31] showed that strong magnetic fields are required for having a reasonable allowed range for dust cyclotron radii. Such physical situation may occur in some regime of astrophysics where the dust charge is high and the dust size is small. It seems also appropriate here to add that the presence of an external magnetic field in a dusty plasma system can alter the charging currents reaching on the dust grain surface, which changes the dust charge dynamics. Theoretical estimations^[55] showed that the dust charge profile in the presence of an external magnetic field depends on the dust grain size (in comparison with the cyclotron radii of ion and electron). For strong magnetic fields, the dust charge number can become larger than that in the absence of the external magnetic fields. But there is a serious problem as any dependence of dust grain charge on the magnitude of the external magnetic field depends on the coupling parameter Γ for which no explicit relationship has yet been known.

Kaw and Sen^[9] showed that the strong correlation among the dust grains may lead to a novel transverse shear mode in strongly coupled dusty plasma. Here, we are interested to investigate how the uniform external magnetic field affects such transverse mode. To obtain the dispersion relation of transverse shear modes in SCDMP system, we take the curl of the momentum equation for dust grains, ignoring the contribution of ∇ × E, to obtain

In the limit of ωτ_m ≪ 1, equation (14) reduces to

On the other hand, in the limit of ωτ_m ≫ 1, we obtain

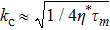
The present article advances some previous studies^[9,18,32,33] with the inclusion of an external magnetic field and the effect of the obliqueness of propagation in strongly coupled dusty plasma. It is shown that the external magnetic field as well as the obliqueness parameter can significantly modify the basic features of the wave propagation in an SCDMP system. The results of our investigation can be pinpointed as follows:

(i) We derived an analytical expression for the longitudinal part of the dielectric response function, including the effects of the external magnetic field as well as the obliqueness of the wave in strongly coupled magnetized dusty plasma. This expression shows how the external magnetic field and the direction of the wave propagation affect the dielectric response function.

(ii) It is shown that for the laboratory strongly coupled dusty plasmas,^[53,54] the dust grains almost remain unmagnetized, while for the high density astrophysical plasmas where a wide range of the magnetic field strengths (such as those occurring in white dwarfs and neutron stars, in which the magnetic field strength in magnetic white dwarfs may be strong, varying in the range of 10³ Gs–10⁹ Gs (1 Gs = 10⁻⁴ T)^[56,57]) exist, the dust grains can be magnetized and the generalized hydrodynamic approach incorporating the contributions of the viscosity effects and magnetic fields can be employed as an appropriate theoretical model.

(iii) It is shown that the longitudinal wave consists of two branches, corresponding to the oblique DA and oblique DC modes, both of which are affected by the strong correlation between the dust grains. It is found that both the real and imaginary parts of the oblique longitudinal wave frequency are modified by the magnitude of the external magnetic field and the direction of the wave propagation.

(iv) The damping rate of such modes has also been obtained and analyzed. On the other hand, the peculiar transverse mode (which is found to be a special mode in strongly coupled systems^[9]) has also been analyzed in an SCDMP system. It is found that in the hydrodynamic limit (ωτ_m≪ 1), the external magnetic field changes the nature of the transverse shear mode drastically. The external magnetic field alters the transverse shear mode from a purely damped mode^[33] to an oscillative one. In the latter, the direction of the wave propagation and the magnitude of the external magnetic field play an important role.

(v) It is also found that the combination effects of the obliqueness parameter and the external magnetic field in the kinetic regime play a crucial role in the propagation of the transverse shear mode. Both the real and imaginary parts of the transverse wave frequency get modified. The cutoff of the transverse mode turns to zero value due to the presence of the external magnetic field and the obliqueness of propagation. The damping rate of the transverse mode is also modified by the influence of the obliqueness parameter. It means that the transverse shear mode is very sensitive to the effect of obliqueness and the external magnetic field.

Also, it must be added that, the present results are more general than that of Xie and Chen^[32] which were restricted to the parallel case of propagation for the transverse shear mode and perpendicular propagation for the longitudinal mode. It must be emphasized that the present results in the limiting cases recover the results of previous studies of Kaw and Sen,^[9] Kaw,^[33] and Shahamsnouri et al.^[18] in the absence of magnetic field, and Nambu and Nita^[49] in the weakly coupled regime and high frequencies.