Potentials of classical force fields for interactions between Na<sup>+</sup> and carbon nanotubes

Cite this Article

Li De-Yuan, Shi Guo-Sheng, Hong Feng, Fang Hai-Ping. Potentials of classical force fields for interactions between Na⁺ and carbon nanotubes**

Project supported by the National Science Fund for Outstanding Young Scholars of China (Grant No. 11722548) and the National Natural Science Foundation of China (Grant Nos. 11574339 and 11404361).

. Chinese Physics B, 2018, 27(9): 098801 Copy to clipboard

Permissions

Potentials of classical force fields for interactions between Na⁺ and carbon nanotubes**

Project supported by the National Science Fund for Outstanding Young Scholars of China (Grant No. 11722548) and the National Natural Science Foundation of China (Grant Nos. 11574339 and 11404361).

Li De-Yuan^{1, 2}, Shi Guo-Sheng^{1, 2, †}, Hong Feng^{1, ‡}, Fang Hai-Ping^{1, 2}

1Department of Physics and Shanghai Applied Radiation Institute, Shanghai University, Shanghai 200444, China

2Division of Interfacial Water and Key Laboratory of Interfacial Physics and Technology, Shanghai Institute of Applied Physics, Chinese Academy of Sciences, Shanghai 201800, China

† Corresponding author. E-mail: gsshi@shu.edu.cn

fenghong@shu.edu.cn

Project supported by the National Science Fund for Outstanding Young Scholars of China (Grant No. 11722548) and the National Natural Science Foundation of China (Grant Nos. 11574339 and 11404361).

Abstract

Carbon nanotubes (CNTs) have long been expected to be excellent nanochannels for use in desalination membranes and other bio-inspired human-made channels owing to their experimentally confirmed ultrafast water flow and theoretically predicted ion rejection. The correct classical force field potential for the interactions between cations and CNTs plays a crucial role in understanding the transport behaviors of ions near and inside the CNT, which is key to these expectations. Here, using density functional theory calculations, we provide classical force field potentials for the interactions of Na⁺/hydrated Na⁺ with (7,7), (8,8), (9,9), and (10,10)-type CNTs. These potentials can be directly used in current popular classical software such as nanoscale molecular dynamics (NAMD) by employing the tclBC interface. By incorporating the potential of hydrated cation-π interactions to classical all-atom force fields, we show that the ions will move inside the CNT and accumulate, which will block the water flow in wide CNTs. This blockage of water flow in wide CNTs is consistent with recent experimental observations. These results will be helpful for the understanding and design of desalination membranes, new types of nanofluidic channels, nanosensors, and nanoreactors based on CNT platforms.

PACS: 88.30.rh;31.15.E;87.10.Tf

Keyword:carbon nanotube;density functional theory;force field;molecular dynamics simulation

Show Figures

1. Introduction

A nanotube that enables fast water flow while rejecting ions is of great importance in water desalination and purification,^[1–6] nanofluidics manipulation,^[7–12] ion detection,^[13] drug delivery,^[14,15] nanodevice fabrication,^[16–21] and biomimetic membrane pore design.^[22,23] It has been found that carbon nanotubes (CNTs) have an extra high water flow rate from theoretical simulations^[24–28] and experimental observations.^[29,30] In 2001, Hummer et al.^[24] theoretically predicted the potential effectiveness of water flow in nanotubes using a molecular dynamics (MD) simulation, and in 2006, Holt et al.^[30] experimentally demonstrated that the water permeability of CNT-based membranes is several orders of magnitude higher than that expected from conventional hydrodynamic theories. Using classical MD simulations based on traditional force fields, it has been theoretically shown that narrower CNTs, with diameters smaller than the size of hydrated ions, would reject the passage of ions while still maintaining ultrahigh permeability for the water flow; an example is a (6,6)-type CNT with a diameter of ∼ 0.5 nm.^[25,31]

With the benefit of this unique property of fast water flow combined with ion rejection, narrower CNTs have been expected to be good candidates for water desalination and purification channels for a decade.^[31,32] However, to date, there has been insufficient experimental demonstration of salt rejection adequate for desalination using pristine CNTs.^[2] Moreover, for wide CNTs (inner diameter above 1 nm), several experiments^[33–35] have shown that there is only very, and even negligibly, small water flow and ion permeation across the CNTs without the addition of pressure or electric field above a threshold. In contrast, results based on popular classical MD simulations showed that there should be fast water permeation across wider tubes.^[31] One of the main reasons is the lack of a correct classical force field potential for the interactions between cations and different CNTs, which results in an incomplete understanding of the transport behaviors of ions near and inside the CNT.

Recently, by incorporating the cation-π interaction potential^[3] to classical all-atom force fields, we found that cations block water flow through narrow (6,6)-type CNTs due to interactions between cations and aromatic rings in CNTs. In wide CNTs (8,8), these interactions trap the cations in the interior of the CNT, inducing unexpected open or closed state switching of ion transfer under a strong electric field, which is consistent with experiments. More appropriate classical force field potentials for the interactions between cations and other CNTs are expected to enable better understanding of the solution transport behaviors of ions near and inside CNTs. Here, we provide classical force field potentials for the interactions of Na⁺/hydrated Na⁺ with (7,7), (8,8), (9,9), and (10,10)-type CNTs using density functional theory (DFT) calculations, which can be directly used in current popular classical software such as nanoscale molecular dynamics (NAMD) by employing the tclBC interface. For an (8,8)-type CNT, compared to our previous potential,^[3] we provide a more accurate classical force field potential for the interactions of Na⁺/hydrated Na⁺ adsorbing inside an (8,8)-type CNT. This will facilitate further CNT-based research and applications.

2. Methods

We built four armchair CNT clusters with different curvatures: (7,7), (8,8), (9,9), and (10,10), corresponding to molecular formulas C₁₆₈H₂₈, C₁₉₂H₃₂, C₂₁₆H₃₆, and C₂₄₀H₄₀, respectively. All their entrances were passivated by H atoms. The lengths of the (7,7), (8,8), (9,9), and (10,10)-type CNTs (along the tube axis, defined as the Z axis) were approximately 15.4 Å. They contained five aromatic rings, and both ends had H atoms attached. Their diameters were 9.6 Å, 10.9 Å, 12.3 Å, and 13.6 Å after DFT optimization.

The original configurations of the (7,7), (8,8), (9,9), and (10,10)-type CNTs were derived from the visual package visual molecular dynamics (VMD).^[36] First, the atoms of the four CNT models were freely optimized to obtain the most stable CNT structures, shown in Fig. 1. These stable CNTs were further used to optimize the most stable geometric structures for Na⁺/hydrated Na⁺ ions in the center of the CNT and at the entrance of these CNTs. We defined the corresponding adsorption energies of Na⁺ and hydrated Na⁺ in the center of CNTs as ΔE_g and ΔE_l, and those at the entrance of the CNTs as and , respectively. Next, we scanned the adsorption energy curves from the center to the outside of the CNTs along the Z axis with steps of 0.6 Å using the Gaussian 09 program.^[37] In hydrated systems, an Na⁺ ion combines with six water molecules.^[3,38] All calculations on the optimized geometric structures were performed with the B3LYP/6-31G(d) model, which was widely employed in studies of the interactions of carbon-based materials with ions.^[3,39–46]

	Figure Option View Download New Window
	Fig. 1. (color online) The side views of carbon nanotubes (CNTs) with different curvatures: (7,7), (8,8), (9,9), and (10,10).

3. Results and discussion

3.1. Adsorption of Na⁺ in the center and at the entrance of (7,7), (8,8), (9,9), and (10,10)-type CNTs

In our previous work,^[3] we provided the classical force field potentials for the interactions of Na⁺/hydrated Na⁺ with (6,6) and (8,8)-type CNTs by simply scanning the interaction energies along the central axis of the tubes using DFT calculations. This method is relatively rough for wide tubes such as (8,8)-type CNTs since the most stable cation adsorption sites are not always in the central axis of the tube. In this work, we provide more accurate potentials for Na⁺/hydrated Na⁺ inside wide tubes. First, we optimized the geometric structures for Na⁺ ions in the center (the point along the Z axis defined as the original point, Z = 0 Å) and at the entrance (Z = 7.8 Å) of (7,7), (8,8), (9,9), and (10,10)-type CNTs. We note that there are three different types of site inside CNTs. We investigated the most stable Na⁺ structure in a (7,7)-type CNT in PS1 of the ESI. We obtained results similar to our previous study,^[40] which are that the H site is the most stable, the B sites are sub-stable, and the T site is unstable, where the Na⁺ ion moves to the H site during geometry optimization. The most stable structures of the system using (7,7)-type CNTs as examples are shown in Figs. 2(a)–2(c), and the other systems are shown in PS2 of the ESI. To study the stability of Na⁺ with different CNTs, we calculated the adsorption energies (ΔE_g) of Na⁺ ions with CNTs, which are defined as

where E_Na⁺−CNT, E_CNT, and E_Na⁺ are the total energies of the Na⁺ ion with the CNT, the CNT clusters, and the Na⁺ ion alone, respectively.

Figure Option
View Download New Window

Fig. 2. (color online) (a), (d) Top views and (b), (e) side views of Na⁺/hydrated Na⁺, respectively, in the most stable geometric structures in the center of a (7,7)-type CNT. (c) and (f) Side views of the most stable geometric structures of the Na⁺ and hydrated Na⁺, respectively, at the entrance of a (7,7)-type CNT. In panel (b), the different adsorption sites are shown, i.e., above the hollow (H), C–C bond (B), and carbon atom top (T) sites of the hexagonal ring.

These adsorption energies are shown in Fig. 3. We note that the adsorption energies of Na⁺ in the center of the CNTs are over 42 kcal/mol, which is about seven times that of the hydrogen binding energy of two water molecules at the same temperature, indicating that the adsorption of Na⁺ in the center of the CNTs is quite stable at room temperature. The adsorption energies will be largely reduced when the Na⁺ ion moves from the center of the CNTs to the entrance of these CNTs. This indicates that Na⁺ prefers to be inside these tubes. The adsorption energy of Na⁺ in the center of a (9,9)-type CNT is 1.0 kcal/mol, 0.3 kcal/mol, and 0.6 kcal/mol larger than that of Na⁺ in the center of (7,7), (8,8), and (10,10)-type CNTs, respectively. Adsorption energy of Na⁺ at the entrance of a (7,7)-type CNT is 0.3 kcal/mol, 0.3 kcal/mol, and 1.0 kcal/mol larger than that of Na⁺ at the entrance of (8,8), (9,9), and (10,10)-type CNTs, respectively. We have performed a residuary charges analysis to illustrate the physical nature of the cation-π interaction between an Na⁺ ion and a single-walled CNT. We find the Hirshfeld charge transfer is consistent with the adsorption energies of Na⁺ at the entrances of different CNTs, as shown in PS3 of the ESI.

	Figure Option View Download New Window
	Fig. 3. (color online) Adsorption energies of Na⁺ (ΔE_g) and hydrated Na⁺ (ΔE_l) in the center (a) and at the entrance (b) of (7,7), (8,8), (9,9), and (10,10)-type CNTs.

3.2. Adsorption of hydrated Na⁺ in the center and at the entrance of (7,7), (8,8), (9,9), and (10,10)-type CNTs

Hydrated Na⁺ combined with six water molecules adsorbed in (7,7), (8,8), (9,9), and (10,10)-type CNTs was investigated, with the corresponding adsorption sites at Z = 0 Å and Z = 7.8 Å. When comparing with Na⁺ at the H site, B site, and T site, we found the H site is also the most stable for hydrated Na⁺ in (7,7)-type CNTs. The geometric structures and the adsorption energies are shown in PS4 of the ESI. We also investigated the different hydrated Na⁺ conformers to find the most stable conformer at the H site in PS5 of the ESI. Examples of the most stable structures of the system for (7,7)-type CNTs are shown in Figs. 2(d)–2(e). According to previous work, the adsorption energies (ΔE_l) of the hydrated Na⁺ with the CNT were calculated as follows:^[3]

where ΔE_{CNT − Na⁺·(H₂O)₆} is the total interaction energy of the hydrated Na⁺ with the CNT, and ΔE_{CNT − (H₂O)₆} is the interaction energy of the water cluster with the CNT.

where E_{CNT − Na⁺·(H₂O)₆} and E_{Na⁺·(H₂O)₆} are the optimized energy of hydrated Na⁺ with CNT and the total energy of six waters with Na⁺, while E_{CNT − (H₂O)₆} and E_(H₂O)₆ are the energies of corresponding geometries taken from the optimized structure of CNT-Na⁺ · (H₂O)₆.

From Fig. 3, we find the adsorption energies of hydrated Na⁺ at Z = 0 Å and Z = 7.8 Å are around 20 kcal/mol and 10 kcal/mol, respectively, which indicates that hydrated Na⁺ in the center of a CNT is more stable than at the entrance. Even though the adsorption energies of hydrated Na⁺ are about half of the energies of Na⁺ with CNTs, they still are over three times the hydrogen binding energy in the center of CNTs. In addition, the adsorption energies of hydrated Na⁺ have similar change tendencies to Na⁺.

3.3. The classical force field potentials for the interactions between Na⁺/hydrated Na⁺ and (7,7), (8,8), (9,9), and (10,10)-type CNTs

Moving the Na⁺ ion along the inner wall with fixed Z coordinate while freely varying the X and Y coordinates can reveal the most stable points of adsorption energies between Na⁺ and CNTs with Z ranging from Z = 0 Å to Z = ± 10.2 Å. Fitting these points as curves can reveal the classical force field potential for the interactions between Na⁺ and CNTs. The curve fits, shown in Fig. 4(a), exhibit two distinct shapes inside the CNT (−5 Å < Z < 5 Å) and outside the CNT (Z < −5 Å or Z > 5 Å). Therefore, the potential energy curve is treated as a piecewise function with a symmetric cosine function inside the CNT and an arctan function outside the CNT

In Eq. (4), Z is the distance between Na⁺ and the center (origin point) of the CNT along the tube’s axis, ε is the depth of the potential well, which is derived from the adsorption energies of Na⁺ in center (Z = 0 Å) of CNTs calculated by DFT, and the parameters α, β, and λ are the nondimensional fit coefficients adjusting the potential curve for the corresponding adsorption energies. λ_out, λ_in, and Z_m are parameters corresponding to the characteristics of the CNTs.

	Figure Option View Download New Window
	Fig. 4. (color online) (a)–(d) Adsorption energies and curve fits for Na⁺ with (7,7), (8,8), (9,9), and (10,10)-type CNTs, respectively.

The seven parameters of the classical force field potential curves for the interactions between Na⁺ and (7,7), (8,8), (9,9), and (10,10)-type CNTs are shown in Table 1. According to these parameters, we can obtain the classical force field potential energy curves, as shown in Fig. 4. For hydrated Na⁺, the adsorption energies and curve fits are shown in PS6 of the ESI. We find that the function is consistent with Eq. (3) for Na⁺, though the depth of the potential well is less than that of Na⁺. From Table 1, we can see that the parameter λ_out increases from 0.7 Å⁻¹ to 2.6 Å⁻¹, which means the steepness becomes sharp at the entrance from (7,7) to (10,10) CNT. For interactions between hydrated Na⁺ and CNTs, the parameter ε is 18.7 kcal/mol, 19.6 kcal/mol, 21.3 kcal/mol, and 20.4 kcal/mol for (7,7), (8,8), (9,9), and (10,10)-type CNTs, respectively, and the other six parameters of the potential curves of classical force fields remain unchanged.

Table 1.

Seven parameters from fitting the potential energy curves of Na⁺ ion in CNT systems of different curvatures.

4. Classical simulations adding the cation-π interaction

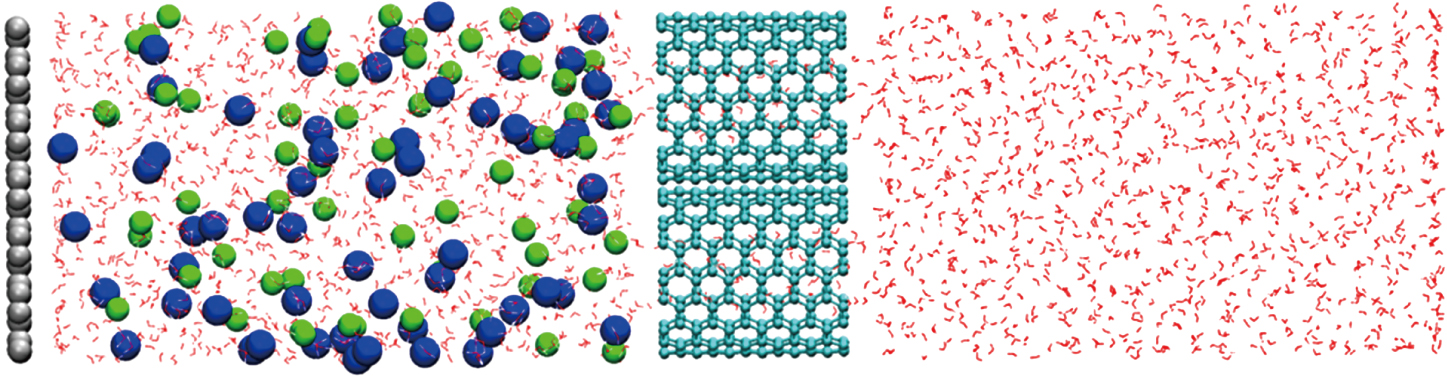We use a (9,9)-type CNT as an example. By incorporating the cation-π interaction potential in a classical all-atom force field using the tclBC code (the details of the tclBC code for (7,7), (8,8), (9,9), and (10,10)-type CNTs are shown in PS7 of the ESI), we perform a simulation using a filter membrane containing four (9,9)-type CNTs, as shown in Fig. 5. The filter membrane separates the NaCl aqueous solution from the pure water. There are 60 pairs of NaCl ions along with 3464 water molecules on the left side, and 3513 water molecules on the right side. To obtain water flux, a constant pressure of 20 MPa between the two entrances is applied on the impermeable wall from the NaCl aqueous solution to the pure water side. Additional parameters for classical MD are shown in PS8 of ESI.

	Figure Option View Download New Window
	Fig. 5. (color online) Initial carbon nanotube (CNT) filter membrane system containing four (9,9)-type CNTs segregating the NaCl solution and pure water.

Figure 6(a) shows that the ions will move inside the CNT and accumulate. This will block the water flow in wide CNTs (see Fig. 6(c)), which is consistent with recent experimental observation.^[34,35] For comparison, we also performed the MD simulation without the cation-π interactions. From Figs. 6(b) and 6(c), we can see that the Na⁺ ions are still dispersed in the solution after 10 ns.

	Figure Option View Download New Window
	Fig. 6. (color online) (a) Na⁺ ions move into the filter membrane consisting of four (9,9)-type CNTs and blocked the water flow at 10 ns with the cation-π interactions. (b) Na⁺ ions are still dispersed in the solution without the cation-π interactions at 10 ns. (c) Cumulative water flow with time through the CNT membrane with (red) and without (black) cation-π interactions.

5. Conclusions

We investigated the adsorption energies of Na⁺/hydrated Na⁺ with (7,7), (8,8), (9,9), and (10,10)-type CNTs in the center and at the entrance using DFT calculations. The results show the interaction between Na⁺ with CNTs is quite strong, over 42 kcal/mol in the center and over 27 kcal/mol at the entrance of the CNTs. Although the adsorption energies of hydrated Na⁺ with these CNTs are reduced to about half that of Na⁺, they remain approximately three times stronger than the hydrogen binding energy in two water molecules at the same temperature.

By scanning the adsorption energies from the inside to the outside of these CNTs and by utilizing the cosine function in conjunction with paired arctan functions, we found the potential curve can be described well by fitting Eq. (3) as a complement to the classical force fields. Based on the potential curves, along with the adsorption energy sharply receding at the entrances of these CNTs, we also found the adsorption energies at the entrance of these CNTs decrease quickly from (7,7) to (10,10)-type CNT.

By incorporating the hydrated cation-π interaction potential obtained here to classical all-atom force fields, we showed that the ions will move inside the CNT and accumulate there. This will block water flow through wide CNTs, which is consistent with recent experimental observations. Our results will help in water desalination and purification, nanofluidic manipulation applications, and other aspects based on the CNT platform.

Reference

[1]	Shannon M A Bohn P W Elimelech M Georgiadis J G Mariñas B J Mayes A M 2008 Nature 452 301
[2]	Elimelech M Phillip W A 2011 Science 333 712
[3]	Liu J Shi G S Guo P Yang J R Fang H P 2015 Phys. Rev. Lett. 115 164502
[4]	Whitby M Quirke N 2007 Nat. Nanotechnol. 2 87
[5]	Liu J Shi G S Fang H P 2017 Nanotechnology 28 084004
[6]	Moradi F Ganji M D Sarrafi Y 2017 Phys. Chem. Chem. Phys. 19 8388
[7]	Wan R Z Li J Y Lu H J Fang H P 2005 J. Am. Chem. Soc. 127 7166
[8]	Li J Y Gong X J Lu H J Li D Fang H P Zhou R H 2007 Proc. Natl. Acad. Sci. USA 104 3687
[9]	Vuković L Vokac E Král P 2014 J. Phys. Chem. Lett. 5 2131
[10]	Bocquet L Charlaix E 2010 Chem. Soc. Rev. 39 1073
[11]	Su J Y Zhao Y Z Fang C Ahmed S B Shi Y 2017 Phys. Chem. Chem. Phys. 19 22406
[12]	Qin X C Yuan Q Z Zhao Y P Xie S B Liu Z F 2011 Nano Lett. 11 2173
[13]	Howorka S Siwy Z 2009 Chem. Soc. Rev. 38 2360
[14]	Bianco A Kostarelos K Prato M 2005 Curr. Opin. Chem. Biol. 9 674
[15]	Zang J L Yuan Q Z Wang F C Zhao Y P 2009 Comput. Mater. Sci. 46 621
[16]	Hilder T A Hill J M 2009 Small 5 300
[17]	Tasis D Tagmatarchis N Bianco A Prato M 2006 Chem. Rev. 106 1105
[18]	Meng S Wang W L Maragakis P Kaxiras E 2007 Nano Lett. 7 2312
[19]	Zhao Y Truhlar D G 2007 J. Am. Chem. Soc. 129 8440
[20]	Yuan Q Z Zhao Y P 2009 Biomicrofluidics 3 6
[21]	Yuan Q Z Zhao Y P 2009 J. Am. Chem. Soc. 131 6374
[22]	Garcia-Fandiño R Sansom M S P 2012 Proc. Natl. Acad. Sci. USA 109 6939
[23]	Yang L H Gordon V D Trinkle D R Schmidt N W Davis M A DeVries C Som A Cronan J E Tew G N Wong G C L 2008 Proc. Natl. Acad. Sci. USA 105 20595
[24]	Hummer G Rasaiah J C Noworyta J P 2001 Nature 414 188
[25]	Kalra A Garde S Hummer G 2003 Proc. Natl. Acad. Sci. USA 100 10175
[26]	Striolo A 2006 Nano Lett. 6 633
[27]	Tu Y S Xiu P Wan R Z Hu J Zhou R H Fang H P 2009 Proc. Natl. Acad. Sci. USA 106 18120
[28]	Falk K Sedlmeier F Joly L Netz R R Bocquet L 2010 Nano Lett. 10 4067
[29]	Majumder M Chopra N Andrews R Hinds B J 2005 Nature 438 44
[30]	Holt J K Park H G Wang Y M Stadermann M Artyukhin A B Grigoropoulos C P Noy A Bakajin O 2006 Science 312 1034
[31]	Corry B 2008 J. Phys. Chem. B 112 1427
[32]	Jia Y X Li H L Wang M Wu L Y Hu Y D 2010 Sep. Purif. Technol. 75 55
[33]	Secchi E Marbach S Nigues A Stein D Siria A Bocquet L 2016 Nature 537 210
[34]	Lee C Y Choi W Han J Strano M S 2010 Science 329 1320
[35]	Choi W Lee C Y Ham M Shimizu S Strano M S 2011 J. Am. Chem. Soc. 133 203
[36]	Humphrey W Dalke A Schulten K 1996 J. Mol. Graphics 14 33
[37]	Frisch G W T M J Schlegel H B Scuseria G E et al. 2009 Gaussian 09 Revision A. 01 Wallingford CT Gaussian Inc
[38]	Shi G S Ding Y H Fang H P 2012 J. Comput. Chem. 33 1328
[39]	Shi G S Chen L Yang Y Z Li D Y Qian Z Liang S S Yan L Li L H Wu M H Fang H P 2018 Nat. Chem. 10 776
[40]	Gao S H Shi G S Fang H P 2016 Nanoscale 8 1451
[41]	Chen L Shi G S Shen J Peng B Zhang B W Wang Y Z Bian F G Wang J J Li D Y Qian Z Xu G Liu G P Zeng J R Zhang L J Yang Y Z Zhou G Q Wu M H Jin W Q Li J Y Fang H P 2017 Nature 550 380
[42]	Shi G S Dang Y R Pan T T Liu X Liu H Li S X Zhang L J Zhao H W Li S P Han J G Tai R Z Zhu Y M Li J C Ji Q Mole R A Yu D H Fang H P 2016 Phys. Rev. Lett. 117 238102
[43]	Lyu G X Shi G S Tang L Fang H P Wu M H 2017 Phys. Chem. Chem. Phys. 19 9354
[44]	Shi G S Yang J R Ding Y H Fang H P 2014 Chem Phys Chem. 15 2588
[45]	Yang J R Shi G S Tu Y S Fang H P 2014 Angew. Chem. Int. Ed. 53 10190
[46]	Shi G S Liu J Wang C L Song B Tu Y S Hu J Fang H P 2013 Sci. Rep. 3 3436