Cite this Article
Guo Shengli, Ning Fanlong. Progress of novel diluted ferromagnetic semiconductors with decoupled spin and charge doping: Counterparts of Fe-based superconductors. Chinese Physics B, 2018, 27(9): 097502  
Permissions
Progress of novel diluted ferromagnetic semiconductors with decoupled spin and charge doping: Counterparts of Fe-based superconductors
Guo Shengli, Ning Fanlong †
Department of Physics, Zhejiang University, Hangzhou 310027, China

 

† Corresponding author. E-mail: ningfl@zju.edu.cn

Project supported by the Chinese Ministry of Science and Technology (Grant No. 2016YFA0300402), the National Natural Science Foundation of China (Grant No. 11574265), the Natural Science Foundation of Zhejiang Province, China (Grant Nos. LR15A040001 and LY14A040007), and the Fundamental Research Funds for the Central Universities, China.

Abstract

Diluted ferromagnetic semiconductors (DMSs) that combine the properties of semiconductors with ferromagnetism have potential application in spin-sensitive electronic (spintronic) devices. The search for DMS materials exploded after the observation of ferromagnetic ordering in III–V (Ga,Mn)As films. Recently, a series of DMS compounds isostructural to iron-based superconductors have been reported. Among them, the highest Curie temperature TC of 230 K has been achieved in (Ba,K)(Zn,Mn)2As2. However, most DMSs, including (Ga,Mn)As, are p-type, i.e., the carriers that mediate the ferromagnetism are holes. For practical applications, DMSs with n-type carriers are also advantageous. Very recently, a new DMS Ba(Zn,Co)2As2 with n-type carriers has been synthesized. Here we summarize the recent progress on this research stream. We will show that the homogeneous ferromagnetism in these bulk form DMSs has been confirmed by microscopic techniques, i.e., nuclear magnetic resonance (NMR) and muon spin rotation (μSR).

PACS: 75.50.Pp;76.75.+i;75.50.Lk;77.80.B-
Keyword:diluted ferromagnetic semiconductors;homogenous ferromagnetism;muon spin rotation (μSR);nuclear magnetic resonance (NMR)
1. Introduction

After fast development of nearly a hundred years, the traditional electronics have met several bottlenecks to improve the performance.[1] Developing spin electronic (spintronic) devices that are based on electron spin rather than (or in addition to) charge is one of the alternative solutions. Ferromagnetic semiconductors are types of materials that combine the properties of semiconductor with ferromagnetism and have potential applications in spintronics. In the past, one of the main aims of the ferromagnetic semiconductor research is to fabricate all-semiconductor spintronics devices.[2] The core issue is to realize spin injection, spin manipulation, and spin detection as firstly introduced by Datta and Das in a spin field effect transistor design.[3] Nowadays, the focus of ferromagnetic semiconductor research has developed from concentrated magnetic semiconductors to diluted magnetic semiconductors (DMSs). Concentrated magnetic semiconductors have the same magnetic atoms in every unit cell. Lanthanide compounds, EuX (X = O, S, Se, and Te)[4] are one type of representative concentrated magnetic semiconductors. The Curie temperature (TC) of ∼ 170 K has been reported in Gd-doped EuO films,[5] which is still far below room temperature and is hard to be improved.[3,6]

DMSs are produced by doping magnetic transition metal elements into nonmagnetic parent semiconductors. Mn doped II–VI DMSs are widely investigated decades ago, as represented by (Cd,Mn)Te, (Zn,Mn)Se, (Zn,Mn)Te, etc.[7] Although the doping level can be as high as 80%, carriers are difficult to be introduced in these compounds. TC does not exceed a few Kelvins.[8,9] More details about group II–VI DMSs can be found in a comprehensive review by Furdyna.[7,10] In the 1990’s, the successful doping of Mn into III–V semiconductor GaAs was achieved by low-temperature molecular-beam-epitaxy (LT-MBE) method, and the research on the ferromagnetism in (Ga,Mn)As has exploded.[11–14] The highest TC in (Ga,Mn)As, as of today, has been reported to be ∼ 190–200 K.[15–17] In addition, various functionalities, like the tunneling anisotropic magnetoresistance,[18,19] current control of magnetization by spin orbit torque,[20,21] and gate control of ferromagnetism,[22,23] based on (Ga,Mn)As DMSs have been reported. The difficulty encountered in (Ga,Mn)As is that the covalent Mn substitution for trivalent Ga introduces spins and holes simultaneously, which makes it hard to separately investigate the individual influence of holes and spins on the ferromagnetism. More progresses about (Ga,Mn)As can be found in a recent review article by Dietl and Ohno.[24] The research of DMS has certainly not been satisfactorily concluded. We list three but not all key issues here. (A) Improving TC to (or above) room temperature; (B) synthesizing an intrinsic DMS with n-type carriers; (C) understanding the mechanism responsible for the ferromagnetic long range ordering.

Recently, several series of novel DMSs that are counterparts of Fe-based superconductors have been synthesized. They include but not limited to “I–II–V” type Li(Zn,Mn)As,[25] “122” type (Ba,K)(Zn,Mn)2As2,[26] and “1111” type (La,Ba)(Zn,Mn)AsO.[27] All these materials have the advantages of iso-valent substitution of Mn for Zn and share two important characteristics. Firstly, the spins and carriers can be separately doped and controlled. Secondly, these material have the possibility to make hetero-structure with Fe-based superconductors, since they all share the same crystal structure and the lattice matching is within 5% with their counterparts. For convenience, we named these DMSs as Zn-based DMSs in the following.

2. Material synthesis and crystal structure

The bulk form Zn-based DMSs are synthesized by solid state reaction from high purity elements. Initially, Masek et al. proposed that Li(Zn,Mn)As is a potential candidate of ferromagnetic DMSs with n-type carriers.[28] The idea is that the isovalent substitution of Mn for Zn introduces spins, and off-stoichiometrical Li can adjust the carrier’s type and concentration, for instance, excess Li for n-type and deficient Li for p-type, respectively. Experimentally, Deng et al. successfully synthesized the bulk form DMS Li(Zn,Mn)As with the highest TC ∼ 50 K,[25] and then Li(Zn,Mn)P with highest TC ∼ 34 K.[29] However, the excess Li doping results in p-type carriers rather than n-type carriers as theoretically expected. A DFT calculation shows that the excess Li prefers to occupy the Zn sites and therefore introduces holes.[29] In Fig. 1, we show the crystal structures of “II–VI” DMS (Zn,Mn)Se, “III–V” DMS (Ga,Mn)As, and “I–II–V” DMS Li(Zn,Mn)As. They all share the same cubic F-43m crystal structure.

Fig. 1. (color online) The crystal structures of (a) ZnSe, (b) GaAs, and (c) LiZnAs.

Later on, some other bulk form Zn-based DMSs isostructural to Fe-based superconductors have been successfully synthesized, which can be roughly categorized into “122”, “1111”, and “32522” type DMSs, according to their chemical formulas. Here we list some of them. “122”-type: (Ba,K)(Zn,Mn)2As2,[26] (Sr,Na)(Zn,Mn)2As2,[30] (Sr,K)(Zn,Mn)2As2,[31] and (Ca,Na)(Zn,Mn)2As2;[32] “1111”-type: (La,Ba)(Zn,Mn)AsO,[27] (La,Sr)(Zn,Mn)AsO,[33] (La,Ca)(Zn,Mn)AsO,[34] (La,Ca)(Zn,Mn)SbO,[35] and (Ba,K)F(Zn,Mn)As;[36] “32522”-type: SrLaO(Zn,Mn)As.[37] All these compounds have the advantages that spins and carriers are doped at different sites. It is worth noting that if the Zn atoms are fully substituted by Mn, the end products are all antiferromagnetic insulators.

In these crystal structures, DMSs can also be obtained by doping carriers and spins at the same ionic sites. Man et al. firstly synthesized a type of Mn and Cu codoped DMS, Ba(Zn,Mn,Cu)2As2, with TC ∼ 70 K where the monovalent Cu1+ is expected to introduce the p-type carriers.[38] Several Mn and Cu codoped DMSs including Li(Zn,Mn,Cu)As, La(Zn,Mn,Cu)AsO, and La(Zn,Mn,Cu)SbO have been reported as well.[39–41] In Table 1, we compare the crystal structure and the Curie temperature among superconductors, the derived DMSs, and their antiferromagnetic counterparts. TN is the Néel temperature. Additionally, several DMSs without Zn constituent have been reported as well. They are Cu-based DMSs (La,Sr)(Cu,Mn)SO,[52] (Ba,K)(Cu,Mn)2Se2,[53] and Cd-based DMSs (Ba,K)(Cd,Mn)2As2,[54] (Ba,Na)(Cd,Mn)2As2.[55]

Table 1.

The selected properties of superconductor (SC), DMS, DMS (Cu), and antiferromagnet (AFM).

.

All aforementioned DMSs are p-type. The ferromagnetic n-type DMSs are rarely reported both in II–VI and III–V DMSs. However, for practical applications, the n-type DMSs are also required. Gu et al. theoretically proposed that an n-type DMS may be realized in a narrow band gap semiconductor.[56] Since the band gap of BaZn2As2 is only ∼ 0.2 eV,[57] which is therefore regarded as a potential parent semiconductor for n-type DMS. Experimentally, Guo et al. reported the successful synthesis of n-type Ba(Zn,Co)2As2 DMS with TC of ∼ 45 K.[58] In Ba(Zn,Co)2As2, Co substitution for Zn introduces spins and electrons simultaneously. More interestingly, it shares the same crystal structure with Fe-based superconductor Ba(Fe,Co)2As2,[59] p-type DMS (Ba,K)(Zn,Mn)2As2,[26] antiferromagnetic insulator BaMn2As2,[48] and paramagnetic metal BaCo2As2.[60] It is conceivable that various junctions and devices can be fabricated among different ordering states.

3. Electrical and magnetic properties
3.1. Electrical properties

The separated doping of spins and carriers provides opportunities to investigate the individual effect of spins and carriers on the electrical and magnetic properties of DMSs. The trend found in these bulk form DMSs is that carriers doping usually reduces the resistivity of DMSs by several orders of magnitude, while Mn doping usually increases the resistivity instead. As an example, we show the resistivity of Li(Zn,Mn,Cu)As with doping dependence in Fig. 2 (adopted from Ref. [39]). We can see that when only doping Cu into the parent compound LiZnAs, the resistivity of Li(Zn,Mn,Cu)As decreases drastically and eventually becomes a metal at the doping level of 15%. This is a common phenomenon for general semiconductors. However, Mn doping introduces spins, and electrons are scattered by spin fluctuations, which increases the electrical resistivity. These two effects jointly affect the resistivity behavior in bulk form DMSs. Wang et al. elucidated that Li(Zn,Mn)As is half metallic from ab initio calculation.[61] On the other hand, once a magnetic field is applied, a negative magneto-resistance is always observed, which can be understood as the suppression of the magnetic scattering by the magnetic field. The magneto-resistance of p-type DMS Ba(Zn,Mn,Cu)2As2 is as large as ∼ −53%,[38] and the n-type DMS Ba(Zn,Co)2As2 is ∼ −17%.[58] In Fig. 2(b), we show the magneto-resistivity of Li(Zn0.70Mn0.15Cu0.15)As. The suppression of resistivity by the magnetic field can be clearly seen.

Fig. 2. (color online) (a) The resistivity of Li(Zn,Mn,Cu)As with different doping levels. (b) The magneto-resistivity of Li(Zn0.70Mn0.15Cu0.15)As. Adopted from Ref. [39].
3.2. Magnetic properties

Although the mechanism responsible for the long range ferromagnetic ordering is still in debate, it is widely accepted that the carrier-mediated ferromagnetism and the antiferromagnetism arising from Mn at nearest-neighbour sites coexist in any Mn doped DMSs. Taking advantage of the decoupled charge and spin doping, we are able to see the critical roles played by carriers in DMSs clearly. For example, doping Mn alone into the parent compounds BaZn2As2[38] and LaZnAsO[27] up to 10% will not induce any type of magnetic ordering. A paramagnetic state is always observed.[27,38] On the other hand, with Mn doped, as small as 2% Ba substitution for La in La(Zn,Mn)AsO immediately induces a clear ferromagnetic ordering.[62] In early days, Dietl et al. predicted that if the carrier and Mn concentrations are high enough, TC of (Ga,Mn)As will increase above room temperature.[63] As of today, the record TC of (Ga,Mn)As is 190–200 K.[15–17] This value is comparable to that of (Ba,K)(Zn,Mn)2As2, which has been reported to be ∼ 230 K.[47]

With a fixed spin density, i.e., for a certain amount of Mn concentration in Li(Zn,Mn)P, it does not mean that the more carriers are doped, the higher TC would be achieved.[33, 64] In Fig. 3, we show the magnetic properties measured for different Li doping levels with a fixed Mn concentration of 0.07 (adopted from Ref. [64]). As shown in Fig. 3(c), the Curie temperature TC reaches to the maximum of 25 K for Li doping level of 7%. While with more carriers doped (overdoped regime), TC, Weiss temperature θ, saturation moment Msat, and effective moment Meff decrease simultaneously. Apparently, doping more Li modifies the density of states and subsequently the shape of Fermi surface, and therefore the ferromagnetic ordering. In a recent work, Dobrowolska et al. reported that TC in (Ga,Mn)As is controlled by the location of the Fermi level within the impurity band, not by the carrier concentration directly.[65] In Li(Zn,Mn)P, the field cooling (FC) and zero field cooling (ZFC) curves show clear bifurcation at some temperature Tf below TC. Tf is the spin freezing temperature that represents the freezing of domain walls. This phenomenon has also been observed in other bulk form DMSs.

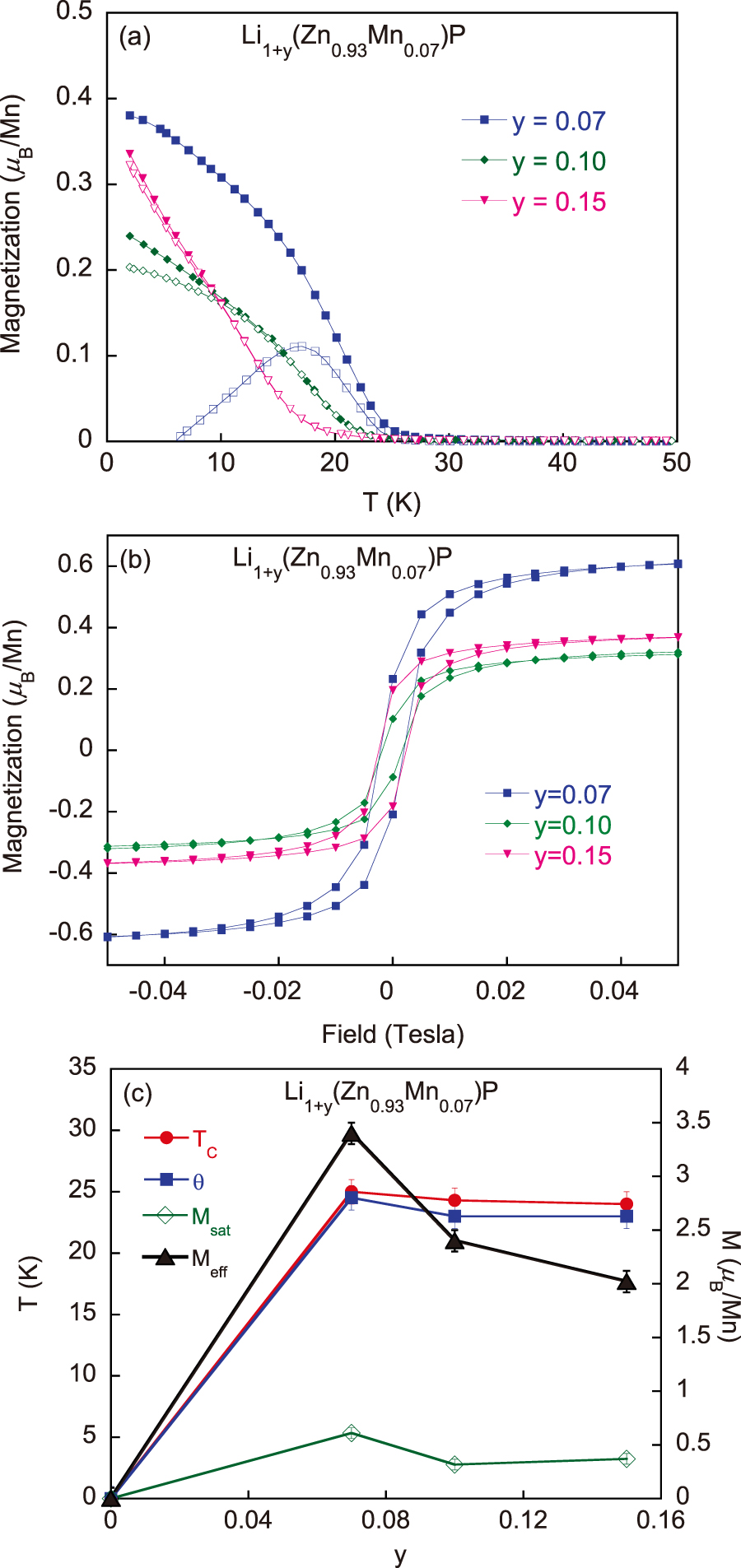Fig. 3. (color online) The Curie temperature (TC), Weiss temperature (θ), saturation moment (Msat), and effective moment (Meff) of Li(Zn,Mn)P with different Li concentrations after fixing the Mn concentration. Adopted from Ref. [64].

The spin state of Mn in (Ga,Mn)As has been determined to be S = 5/2.[11] In general, the spin state of Mn in DMSs can be deduced from Curie–Weiss fitting from temperature dependent magnetization and the saturation moment. Basing on the Curie–Weiss fitting, the effective moment of Mn substituted in Zn-based DMSs is estimated to be ∼ 5 μB/Mn.[25–27] However, it is difficult to accurately determine the value of S by the same method due to the influence of antiferromagnetism and the intrinsic inhomogeneous distribution of carriers and Mn atoms. Suzuki et al. applied x-ray absorption spectroscopy and confirmed that the spin state of Mn in (Ba,K)(Zn,Mn)2As2 is 5/2, as that in (Ga,Mn)As and (Ga,Mn)N.[66]

4. NMR, neutron scattering, μSR, and optical methods

The availability of bulk form DMSs enables them to be measured by NMR, neutron scattering, and μSR techniques which are usually based on bulk form specimens in addition to the optical methods. In the following, we introduce the microscopic properties obtained by these techniques.

4.1. NMR

Ding et al. firstly performed NMR measurements on Li(Zn,Mn)P.[67] In addition to Li(0) (here zero means no Mn at nearest neighbour Zn sites), they observed a broad Li(Mn) peak arising from Mn doping (here Mn in parentheses means Mn at nearest neighbor Zn sites). The broadening of Li(0) site displays the same temperature dependance as the Knight shifts of Li(Mn) sites all the way down to the base temperature of 4.2 K. This indicates that Li(0) and Li(Mn) are electronically coupled, and Mn–Mn interaction extends over many unit cells.[67] The spin dynamics for Li(0) and Li(Mn) sites could be measured, respectively. In Fig. 4, we show the spin-lattice relaxation rate, 1/T1, for Li(0) and Li(Mn) of LiZn0.9Mn0.1P (adopted from Ref. [67]). 1/T1 at Li(Mn) sites is ∼ 50 times larger than that of Li(0). That means, the relaxation time at Li(Mn) sites are ∼ 50 times shorter than that of Li(0) sites. Moreover, 1/T1 at Li(Mn) sites is constant above TC, while 1/T1 at Li(0) sites is linearly proportional to temperature. Constant 1/T1 indicates that the spin fluctuations are locked between TC and 300 K, that is, the averaged Mn–Mn spin interaction energy scale |J| is in the order of ∼ 100 K.[67] On the other hand, 1/T1 is proportional to T for Li(0) site, which follows the Korringa relation arising from the Fermi surface excitations of a small number of conduction carriers. Apparently, these carriers facilitate the interactions between distant Mn sites. Combining every piece of information, a conclusion of carrier mediated ferromagnetism is readily arrived.

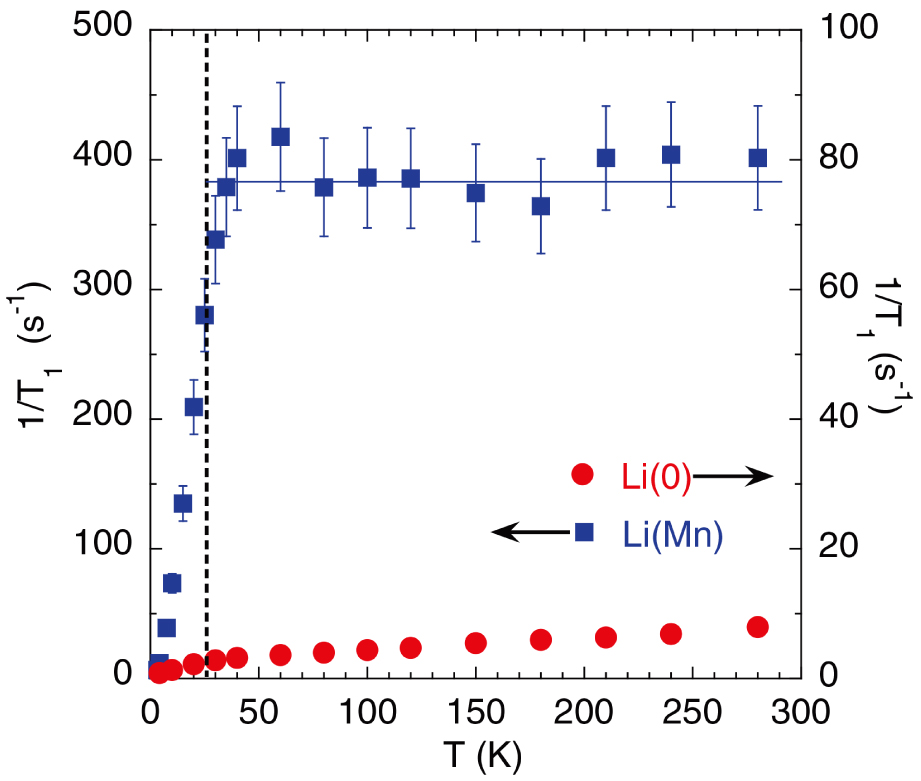Fig. 4. (color online) 1/T1 of Li(0) and Li(Mn) of LiZn0.9Mn0.1P. The dashed line marks TC. Adopted from Ref. [67].
4.2. Neutron scattering

Neutron scattering is a powerful method to map out the spin structure. In Fig. 5, we show the neutron scattering pattern of (La0.9Sr0.1)(Zn0.9Mn0.1)AsO at 4 K (adopted from Ref. [33]). No impurity phase is observed, and no structural phase transition is observed down to 4.2 K. Unfortunately, because the Mn spins are diluted and the averaged magnetic moment size is very small ∼ 0.1 μB/Mn, which is about the resolution limit of neutron scattering, our measurement could not separate the Bragg peaks between structural and ferromagnetic spins.[26,27] Recently, using neutron pair distribution function measurements, Frandsen et al. found that robust short-range ferromagnetic alignment of nearest-neighbour Mn spins along the c axis persists even well above TC and weaker ferromagnetic correlation among Mn spins with further distance increases below TC.[68] Using the inelastic neutron scattering method, Surmach et al. successfully distinguished the localized magnetic excitation and observed the destruction of nearest-neighbour Mn–Mn singlet ground state upon holes doping in (Ba,K)(Zn,Mn)2As2.[69]

Fig. 5. (color online) The neutron scattering pattern of (La0.9Sr0.1)(Zn0.9Mn0.1)AsO at 4 K. Adopted from Ref. [33].
4.3. μSR

μSR is the abbreviation for muon spin rotation/relaxation/resonance. A beam of ∼ 100% polarized muons hit into samples. Muons will precess in the magnetic field after stopping in the inter-sites of the crystal lattice. By recording the positrons muons emit, we can infer the internal field environment around muons. Due to its extreme high field sensitivity, ∼ 0.1 Oe, μSR method is quite suitable to investigate DMSs. μSR is a volume-sensitive method, and we can extract the volume fraction of magnetic ordered state using zero field (ZF) μSR and weak transverse field (wTF) μSR methods. In addition, ZF-μSR and longitudinal field (LF) μSR give us important information about static internal field and spin dynamics. These methods have been widely applied in strongly correlated materials, especially in high temperature superconductors.

Although the low energy μSR has been developed, the procedure to apply μSR for thin films is still sophisticated. Dunsiger et al. firstly confirmed the intrinsic ferromagnetism in (Ga,Mn)As by μSR method.[70] They deduced the temperature dependent magnetic volume fraction, and established the linear relation between the muon relaxation rate versus TC. In Fig. 6, we show the μSR results of Li(Zn,Mn)P (adopted from Ref. [64]). Below TC, ZF-μSR spectra show fast relaxation and wTF-μSR spectra show reduction of oscillation amplitude. The deduced magnetic volume fraction reaches nearly 100% at base temperature, indicating that the ferromagnetism is homogeneous and intrinsic. When we plot the relation of static local field parameter as and TC for (Ga,Mn)As and other Zn-base DMSs, we find that all points fall on the same line. This indicates that the bulk form Zn-based DMSs share the same ferromagnetic mechanism as that of (Ga,Mn)As films. Guo et al. also conducted μSR measurements on the n-type DMS Ba(Zn,Co)2As2.[58] The point for Ba(Zn,Co)2As2 lies a very different location from other p-type DMSs, indicating that either a very strong ferromagnetic interaction exists in Ba(Zn,Co)2As2, or a completely different ferromagnetism in this n-type DMS.[58]

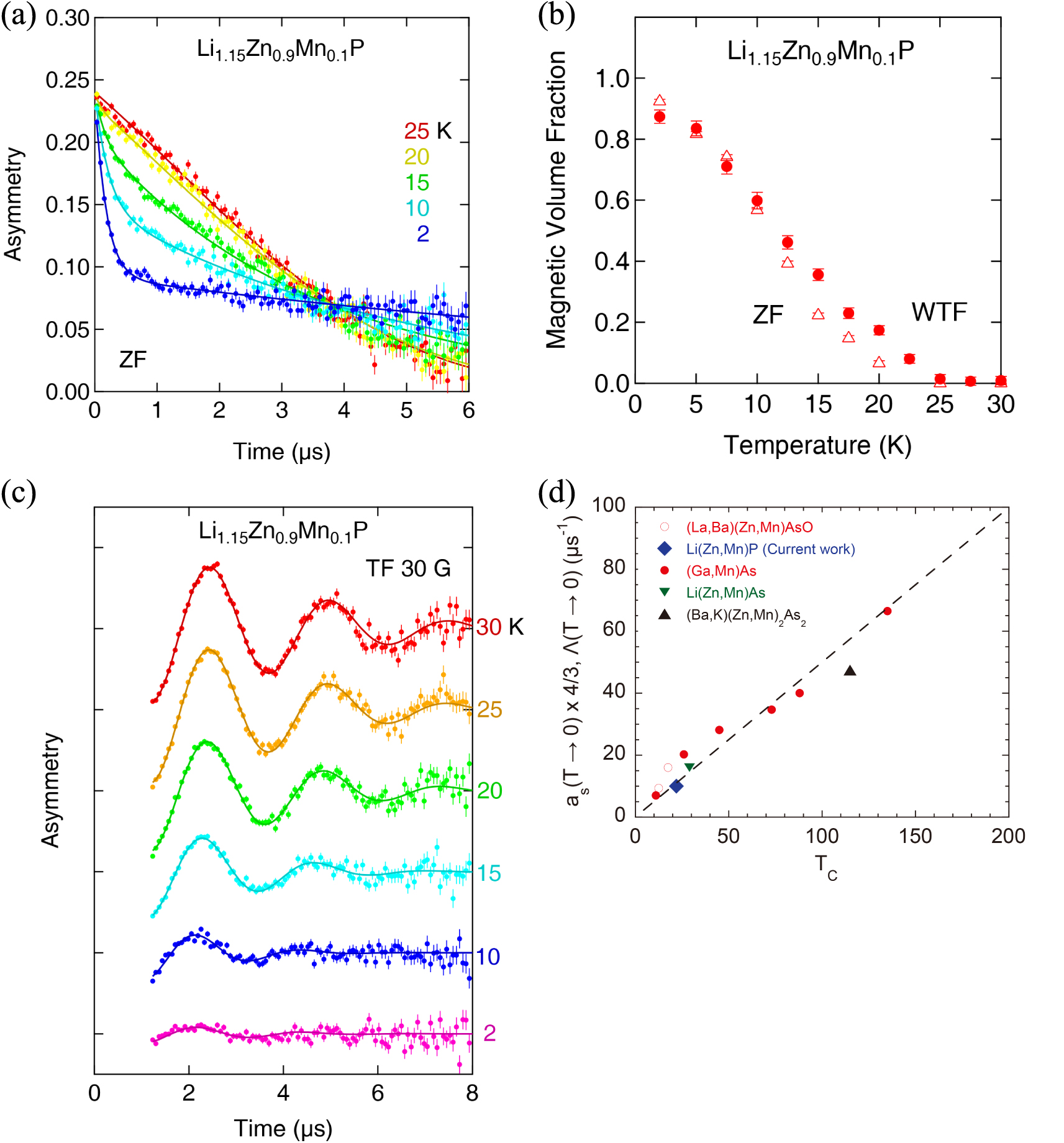Fig. 6. (color online) (a) The zero field μSR time spectra of Li1.15(Zn0.9Mn0.1)P. (b) The magnetic volume fraction of Li1.15(Zn0.9Mn0.1)P deduced from zero field and weak transverse field μSR. (c) The weak transverse field μSR time spectra of Li1.15(Zn0.9Mn0.1)P. (d) The relation of static internal field parameter as obtained form zero field μSR and TC for different DMSs. Adopted from Ref. [64].
4.4. Optical methods

Many efforts have been made to understand the ferromagnetic mechanism in Zn-based DMSs by optical methods, like x-ray absorption spectroscopy (XAS), x-ray emission spectroscopy (XES), resonance photoemission spectroscopy (RPES), angle-resolved photoemission spectroscopy (ARPES), and x-ray magnetic circular dichroism (XMCD) measurements. Taking the example of (Ba,K)(Zn,Mn)2As2, Mn L2,3-edge XAS and RPES measurements showed that doped holes go into the top of the As 4p-derived valence band and weakly bound to the Mn local spins.[71] Resonance soft x-ray ARPES measurements revealed that the Mn 3d impurity band is below the valence band maximum, in contrast to the position of impurity band in (Ga,Mn)As that is above the valence band maximum.[72] XMCD measurements under ambient and high pressure confirmed that the As 4p carriers mediate the ferromagnetic interaction between Mn spins.[73] More recent Mn Kβ XES and As K-edge XMCD measurements focusing on the effect of holes doping under pressure showed that holes doping increased the p–d hybridization strength at the cost of the reduction in Mn local spin density, resulting in an enhanced exchange interaction between Mn dopants.[74]

5. Summary

In summary, a series of bulk form DMSs that are derivatives of iron-based superconductors have been successfully synthesized. The features of Zn-based DMSs are summarized as follows. (1) They have the advantage of decoupled spins and carriers doping. (2) They share the same ferromagnetic mechanism as that of (Ga,Mn)As thin films, as indicated by the μSR results. (3) Carriers play a critical role to form a long range ferromagnetic ordering state. (4) The over-doping of carriers is not always a benefit to improve TC. (5) The availability of bulk form enables it to be further investigated by microscopic experimental technique, such as NMR, neutron scattering, and μSR. (6) The highest TC of (Ba,K)(Zn,Mn)2As2 has reached to 230 K, which is comparable to the highest record TC of (Ga,Mn)As. Its TC has potential to be increased to room temperature.[75] (7) An n-type DMS Ba(Zn,Co)2As2 with TC ∼ 45 K has been synthesized.[58]

One main object of DMS research is to increase TC to (or above) room temperature. However, because of the lattice distortion and solid solubility, it is hard to further increase the carrier concentrations. Since it has been reported that the ferromagnetism in (Ti,Co)O2 could been induced by electric field,[76] carrier accumulation by electrical method is a path to try in future. The other research effort we could expect is the design of functional heterostructure on the basis of Zn-based DMSs. Zhao et al. have reported the successful fabrication of prototypical Andreev reflection junction from single crystal of (Ba,K)(Zn,Mn)2As2 and achieved 66% spin polarization.[Zhao] Since the lattice matching is within 5%, it is conceivable that various junctions and devices can be fabricated to combine n-type DMS Ba(Zn,Co)2As2,[58] p-type DMS (Ba,K)(Zn,Mn)2As2,[26] the antiferromagnetic insulator BaMn2As2 (TN = 625 K),[48] the paramagnetic metal BaCo2As2,[60] and the superconductor Ba(Fe,Co)2As2[59] through the As layers. Recently, superconducting films of Ba(Fe1−xCox)2As2 fabricated with pulsed laser deposition methods have been reported by many groups.[78] More recently, Xiao et al. have successfully grown high-quality epitaxial films of the tetragonal β-BaZn2As2,[57] and Cao et al. are working on the growth of Ba(Zn,Co)2As2 films.[79] With the progress of thin film growth, we expect a better understanding of interplay between p–d Zener ferromagnetism, antiferromagnetic superexchange, and superconductivity.

Reference
[1] Datta S Das B 1990 Appl. Phys. Lett. 56 665
[2] Molnar S V Read D 2003 Proc. IEEE 91 715
[3] Mack C A 2011 IEEE Trans. Semicond. Manuf. 24 202
[4] McGuire T T Argyle B E Shafer M W Smart J S 1963 J. Appl. Phys. 34 1345
[5] Ott H Heise S J Sutarto R Hu Z Chang C F Hsieh H H Lin H J Chen C T Tjeng L H 2006 Phys. Rev. B 73 094407
[6] Zhao J H Deng J J Zheng H Z 2007 Progress In Physics 27 109 in Chinese
[7] Furdyna J K 1988 J. Appl. Phys. 64 R29
[8] Haury A Wasiela A Arnoult A Cibert J Tatarenko S Dietl T d’Aubigne Y M 1997 Phys. Rev. Lett. 97 511
[9] Ferrand D Cibert J Wasiela A Bourgognon C Tatarenko S Fishman G Andrearczyk T Jaroszynski J Kolesnik S Dietl T Barbara B Dufeu D 2001 Phys. Rev. B 63 085201
[10] Samarth N Furdyna J K 1990 Proc. IEEE 78 990
[11] Ohno H 1998 Science 281 951
[12] Jungwirth T Sinova J Masek J Kucera J MacDonald A H 2006 Rev. Mod. Phys. 78 809
[13] Ditel T 2010 Nat. Mater. 9 965
[14] Zutic I Fabian J Sarma S D 2004 Rev. Mod. Phys. 76 323
[15] Wang M Campion R P Rushforth A W Edmonds K W Foxon C T Gallagher B L 2008 Appl. Phys. Lett. 93 132103
[16] Chen L Yan S Xu P F Lu J Wang W Z Deng J J Qian X Ji Y Zhao J H 2009 Appl. Phys. Lett. 95 182505
[17] Chen L Yang X Yang F H Zhao J H Misuraca J Xiong P Molnar S V 2011 Nano Lett. 11 2584
[18] Gould C Rüster C Jungwirth T Girgis E Schott G M Giraud R Brunner K Schmidt G Molenkamp L W 2004 Phys. Rev. Lett. 93 117203
[19] Wunderlich J Jungwirth T Kaestner B Irvine A C Shick A B Stone N Wang K Y Rana U Giddings A D Foxon C T Campion R P Williams D A Gallagher B L 2006 Phys. Rev. Lett. 97 077201
[20] Chernyshov A Overby M Liu X Furdyna J K Lyanda-Geller Y Rokhinson L P 2009 Nat. Phys. 5 656
[21] Li Y Cao Y F Wei G N Li Y Ji Y Wang K Y Edmonds K W Campion R P Rushforth A W Foxon C T Gallagher B L 2013 Appl. Phys. Lett. 103 022401
[22] Chiba D Yamanouchi M Matsukura F Ohno H 2003 Science 301 943
[23] Sawicki M Chiba D Korbecka A Nishitani Y Majewski J A Matsukura F Dietl T Ohno H 2010 Nat. Phys. 6 22
[24] Dietl T Ohno H 2014 Rev. Mod. Phys. 86 187
[25] Deng Z Jin C Q Liu Q Q Wang X C Zhu J L Feng S M Chen L C Yu R C Arguello C Goko T Ning F L Zhang J S Wang Y Y Aczel A A Munsie T Williams T J Luke G M Kakeshita T Uchida S Higemoto W Ito T U Gu B Maekawa S Morris G D Uemura Y J 2011 Nat. Commun. 2 422
[26] Zhao K Deng Z Wang X C Han W Zhu J L Li X Liu Q Q Yu R C Goko T Frandsen B Liu L Ning F L Uemura Y J Dabkowska H Luke G M Luetkens H Morenzoni E Dunsiger S R Senyshyn A Boni P Jin C Q 2013 Nat. Commun. 4 1442
[27] Ding C Man H Y Qin C Lu J C Sun Y L Wang Q Yu B Q Feng C M Goko T Arguello C J Liu L Frandsen B A Uemura Y J Wang H D Luetkens H Morenzoni E Han W Jin C Q Munsie T Williams T J D’Ortenzio R M Medina T Luke G M Imai T Ning F L 2013 Phys. Rev. B 88 041102(R)
[28] Masek J Kudrnovsky J Maca F Gallagher B L Campion R P Gregory D H Jungwirth T 2007 Phys. Rev. Lett. 98 067202
[29] Deng Z Zhao K Gu B Han W Zhu J L Wang X C Li X Liu Q Q Yu R C Goko T Frandsen B Liu L Zhang J S Wang Y Y Ning F L Maekawa S Uemura Y J Jin C Q 2013 Phys. Rev. B 88 081203(R)
[30] Chen B J Zhao K Deng Z Han W Zhu J L Wang X C Liu Q Q Frandsen B Liu L Cheung S Ning F L Munsie T J S Medina T Luke G M Carlo J P Munevar J Uemura Y J Jin C Q 2014 Phys. Rev. B 90 155202
[31] Yang X J Chen Q Li Y P Wang Z Bao J K Li Y K Tao Q Cao G H Xu Z A 2014 EPL 107 67007
[32] Zhao K Chen B J Deng Z Han W Zhao G Q Zhu J L Liu Q Q Wang X C Frandsen B Liu L Cheung S Ning F L Munsie T J S Medina T Luke G M Carlo J P Munevar J Zhang G M Uemura Y J Jin C Q 2014 J. Appl. Phys. 116 163906
[33] Ding C Gong X Man H Y Zhi G X Guo S L Zhao Y Wang H D Chen B Ning F L 2014 EPL 107 17004
[34] Ding C Guo S L Zhao Y Man H Y Fu L C Gu Y L Wang Z Y Liu L Frandsen B A Cheung S Uemura Y J Goko T Luetkens H Morenzoni E Zhao Y Ning F L 2016 J. Phys.: Condens. Matter 28 026003
[35] Han W Zhao K Wang X C Liu Q Q Ning F L Deng Z Liu Y Zhu J L Ding C Man H Y Jin C Q 2013 Sci. China Phys. Mech. 56 2026
[36] Chen B J Deng Z Li W M Gao M R Liu Q Q Gu C Z Hu F X Shen B G Frandsen B Cheung S Liu L Uemura Y J Ding C Guo S L Ning F L Munsie T J S Wilson M N Cai Y P Luke G Guguchia Z Yonezawa S Li Z Jin C Q 2016 Sci. Rep. 6 36578
[37] Man H Y Qin C Ding C Wang Q Gong X Guo S L Wang H D Chen B Ning F L 2014 EPL 105 67004
[38] Man H Y Guo S L Sui Y Guo Y Chen B Wang H D Ding C Ning F L 2015 Sci. Rep. 5 15507
[39] Guo S L Zhao Y Man H Y Ding C Gong X Zhi G X Fu L C Gu Y L Frandsen B A Liu L Cheung S C Munsie T J Wilson M N Cai Y P Luke G M Uemura Y J Ning F L 2016 J. Phys.: Condens. Matter 28 366001
[40] Guo S L Zhao Y Gong X Man H Y Ding C Zhi G X Fu L C Gu Y L Wang H D Chen B Guo Y Ning F L 2016 EPL 114 57008
[41] Zhao Y Wang K Guo S L Fu L C Gu Y L Zhi G X Xu L X Cui Q Cheng J G Wang H D Chen B Ning F L 2018 EPL 120 47005
[42] Hsu F C Luo J Y Yeh K W Chen T K Huang T W Wu P M Lee Y C Huang Y L Chu Y Y Yan D C Wu M K 2008 Proc. Natl. Acad. Sci. U.S.A. 105 14262
[43] Ito T Ito K Oka M 1977 Jpn. J. Appl. Phys. 17 371
[44] Wang X C Liu Q Q Lv Y X Gao W B Yang L X Yu R C Li F Y Jin C Q 2008 Solid State Commun. 148 538
[45] Bronger W Muller P Hoppner R Schuster H U 1986 Z. Anorg. Allg. Chem. 539 175
[46] Rotter M Tegel M Johrendt D 2008 Phys. Rev. Lett. 101 107006
[47] Zhao K Chen B J Zhao G Q Yuan Z Liu Q Q Deng Z Zhu J L Jin C Q 2014 Chin. Sci. Bull. 59 2524
[48] Singh Y Green M A Huang Q Kreyssig A McQueeney R J Johnston D C Goldman A I 2009 Phys. Rev. B 80 100403(R)
[49] Kamihara Y Watanabe T Hirano M Hosono H 2008 J. Am. Chem. Soc. 130 3296
[50] Emery N Wildman E J Skakle J M S Mclaughlin A C Smith R I Fitch A N 2011 Phys. Rev. B 83 144429
[51] Shirage P M Kihou K Lee C H Kito H Eisaki H Iyo A 2011 J. Am. Chem. Soc. 133 9630
[52] Yang X J Li Y K Shen C Y Si B Q Sun Y L Tao Q Cao G H Xu Z A Zhang F C 2013 Appl. Phys. Lett. 103 022410
[53] Guo S L Man H Y Gong X Ding C Zhao Y Chen B Guo Y Wang H D Ning F L 2016 J. Magn. Magn. Mater. 400 295
[54] Yang X J Li Y K Zhang P Jiang H Luo Y K Chen Q Feng C M Cao C Dai J H Tao Q Cao G H Xu Z A 2013 J. Appl. Phys. 114 223905
[55] Chen B J Deng Z Li W M Gao M R Li Z Zhao G Q Yu S Wang X C Liu Q Q Jin C Q 2016 J. Appl. Phys. 120 083902
[56] Gu B Maekawa S 2016 Phys. Rev. 94 155202
[57] Xiao Z W Ran F Y Hiramatsu H Matsuishi S Hosono H Kamiya T 2014 Thin Solid Films 559 100
[58] Guo S L Man H Y Ding C Zhao Y Fu L C Gu Y L Zhi G X Frandsen B A Cheung S C Guguchia Z Yamakawa K Chen B Wang H D Deng Z Jin C Q Uemura Y J Ning F L arXiv:1712.06764 [cond-mat.mtrl-sci]
[59] Sefat A S Jin R McGuire M A Sales B C Singh D J Mandrus D 2008 Phys. Rev. Lett. 101 117004
[60] Sefat A S Singh D J Jin R McGuire M A Sales B C Mandrus D 2009 Phys. Rev. 79 024512
[61] Wang A L Wu Z M Hu A Y Zhao R Y 2013 Acta Phys. Sin. 62 137101 in Chinese
[62] Ding C Guo S Zhi G Ning F L The investigation of ferromagnetism in an extreme diluted regime in (La1-xBax)(Zn1-xMnx)AsO diluted magnetic semiconductor to be submitted
[63] Dietl T Ohno H Matsukura F Cibert J Ferrand D 2010 Science 287 1019
[64] Ning F L Man H Y Gong X Zhi G X Guo S L Ding C Wang Q Goko T Liu L Frandsen B A Uemura Y J Luetkens H Morenzoni E Jin C Q Munsie T Luke G M Wang H D Chen B 2014 Phys. Rev. 90 085123
[65] Dobrowolska M Tivakornsasithorn K Liu X Furdyna J K Berciu M Yu K M Walukiewicz W 2012 Nat. Mater. 11 444
[66] Suzuki H Zhao K Shibata G Takahashi Y Sakamoto S Yoshimatsu K Chen B J Kumigashira H Chang F H Lin H J Huang D J Chen C T Gu B Maekawa S Uemura Y J Jin C Q Fujimori A 2015 Phys. Rev. 91 140401(R)
[67] Ding C Qin C Man H Y Imai T Ning F L 2013 Phys. Rev. 88 041108
[68] Frandsen B A Gong Z Terban M W Banerjee S Chen B Jin C Feygenson M Uemura Y J Billinge S J L 2016 Phys. Rev. 94 094102
[69] Surmach M A Chen B J Deng Z Jin C Q Glasbrenner J K Mazin I I Ivanov A Inosov D S 2018 Phys. Rev. 97 104418
[70] Dunsiger S R Carlo J P Goko T Nieuwenhuys G Prokscha T Suter A Morenzoni E Chiba D Nishitani Y Tanikawa T Matsukura F Ohno H Ohe J Maekawa S Uemura Y J 2010 Nat. Mater. 9 299
[71] Suzuki H Zhao K Shibata G Takahashi Y Sakamoto S Yoshimatsu K Chen B J Kumigashira H Chang F H Lin H J Huang D J Chen C T Gu B Maekawa S Uemura Y J Jin C Q Fujimori A 2015 Phys. Rev. 91 140401(R)
[72] Suzuki H Zhao G Q Zhao K Chen B J Horio M Koshiishi K Xu J Kobayashi M Minohara M Sakai E Horiba K Kumigashira H Gu B Maekawa S Uemura Y J Jin C Q Fujimori A 2015 Phys. Rev. 92 235120
[73] Sun F Li N N Chen B J Jia Y T Zhang L J Li W M Zhao G Q Xing L Y Fabbris G Wang Y G Deng Z Uemura Y J Mao H K Haskel D Yang W G Jin C Q 2016 Phys. Rev. 93 224403
[74] Sun F Zhao G Q Escanhoela C A Chen B J Kou R H Wang Y G Xiao Y M Chow P Mao H K Haskel D Yang W G Jin C Q 2017 Phys. Rev. 95 094412
[75] Hirohata A Sukegawa H Yanagihara H Zutic I Seki T Mizukami S Swaminathan R 2015 IEEE Trans. Magn. 51 1
[76] Yamada Y Ueno K Fukumura T Yuan H T Shimotani H Iwasa Y Gu L Tsukimoto S Ikuhara Y Kawasaki M 2011 Science 332 1065
[77] Zhao G Q Lin C J Deng Z Gu G X Yu S Wang X C Gong Z Z Uemera Y J Li Y Q Jin C Q 2018 Sci. Rep. 7 14473
[78] Haindl S Kidszun M Oswald S Hess C Buchner B Kolling S Wilde L Thersleff T Yurchenko V V Jourdan M Hiramatsu H Hosono H 2014 Rep. Prog. Phys. 77 046502
[79] Cao L X at Institute of Physics, Chinese Academy of Science. Private communications