† Corresponding author. E-mail:
Project supported by the National Natural Science Foundation of China (Grant Nos. 11635003, 11025524, and 11161130520), the National Basic Research Program of China (Grant No. 2010CB832903), and the European Commission’s 7th Framework Programme (Fp7-PEOPLE-2010-IRSES) (Grant Agreement Project No. 269131).
We present a systematic investigation of the impact of changing the geometry structure of the SPC/E water model by performing a series of molecular dynamic simulations at 1 bar (1 bar = 105 Pa) and 298.15 K. The geometric modification includes altering the H–O–H angle range from 90° to 115° and modifying the O–H length range from 0.90 Å to 1.10 Å in the SPC/E model. The former is achieved by keeping the dipole moment constant by modifying the O–H length, while in the latter only the O–H length is changed. With the larger bond length and angle, we find that the liquid shows a strong quadrupole interaction and high tetrahedral structure order parameter, resulting in the enhancement of the network structure of the liquid. When the bond length or angle is reduced, the hydrogen bond lifetime and self-diffusion constant decrease due to the weakening of the intermolecular interaction. We find that modifying the water molecular bond length leading to the variation of the intermolecular interaction strength is more intensive than changing the bond angle. Through calculating the average reduced density gradient and thermal fluctuation index, it is found that the scope of vdW interaction with neighbouring water molecules is inversely proportional to the change of the bond length and angle. The effect is mainly due to a significant change of the hydrogen bond network. To study the effect of water models as a solvent whose geometry has been modified, the solutions of ions in different solvent environments are examined by introducing NaCl. During the dissolving process, NaCl ions are ideally dissolved in SPC/E water and bond with natural water more easily than with other solvent models.
Water is the most important liquid for our existence. Despite the tiny size of the water molecule, a network structure can be made by strong intermolecular hydrogen bonds (H-bonds) in the liquid ambient condition.[1–4] This capacity to form H-bonds, except as the non-directional interactions seen in some simple liquids, results in many unusual properties. For instance, density exhibits as maximum at 4°C and viscosity shows decreases under high pressure.[5–8] These property changes are strongly related to the structure of the water liquid phase, which in turn depend on the intermolecular potential. Additionally, in aqueous solutions, water as a solvent also has a significant effect on the conformation of proteins and nucleic acids molecule, such as pleated sheets and helices.[9–12] Despite significant research into properties of water, its structural evolution is still somewhat unclear.[13–15] The problem is, how does a water molecule evolve to an optimal geometric structure? How does the intermolecular interaction change and is the solubility of water changed as the water molecule evolves to the optimal structure? Therefore, to explore the structural evolution of the water molecule is very important and intriguing. With the development of computer technology, molecular dynamics (MD) simulations become an important tool: they are not limited by the constraints of experiments, thus offering an ideal route for studying the individual contributions of different aspects.
Two types of modified water models used in such investigations include the alteration of either the geometric angle (Bent model) or the relative strength of electronic and dispersive forces alteration (Hybrid model). Lynden-Bell and colleagues performed a series of molecular dynamic simulations, which resulted in various interpretations regarding the properties of these modified liquids.[16–21] They did so by changing the SPC/E model’s H–O–H angles from 90° to 60° and adjusting the Lennard-Jones (LJ) parameters σ to produce approximately the same dipole moment parameters as the SPC/E water model. This resulted in the water’s network structure changing from a three-dimensional to a one-dimensional chain. The H-bonding strength was affected by altering the weight of the Lennard-Jones term relative to the electrostatic term. This leads to a change of the liquid structure from water-like to a new type of Lennard-Jones liquid without an evident local order structure.
Yet another study[22] focused on investigating the effects of altering the SPC/E model geometric angle as a function of temperature within the angle range from 120° to 60°. They found that the density anomalies appear as the bond angle is at least 100°. Increasing the bond angle leads to a shift of the density maximum anomaly. In addition, their study also showed that the first-neighbour shell for 60° is relatively changed over 200, 250, and 300 K; however, both 90° and 100° gradually acquire water-like structural features upon cooling.
It is inevitable that the change of a water molecule’s structure, and the hydrogen and oxygen atom charge lead to changing its properties. Nevertheless, the dissolving of charged ions strongly depends on the structure or property of the solvent around them. In our pervious research,[23] the relative weight of Lennard-Jones and electronic components was modified, based on the SPC water model, to investigate the relationship between the solution of NaCl and the H-bonding ability of water at room temperature and standard atmospheric pressure. The results indicated that NaCl is most ideally dissolved in normal water with the strongest hydration effects around both cations and anions.
The current study seeks to probe the property changes of water when the geometry structure of the water molecule is altered, and also tries to understand changes in the intermolecular interaction between water molecules during the geometric evolution of a water molecule. So we build a series of water models (not real water) based on the SPC/E water model. Similarly, the solvation behavior of the Na+ and Cl− ions in these water models is also investigated. The O–H lengths variation ranges from 0.90 Å to 1.10 Å (1 Å in SPC/E), while the change in H–O–H angles ranges from 90° to 115° (109.47° in SPC/E). The optimal distance between atoms is found to vary with the change in the SPC/E model geometric structure. The distance change, however, has a significant effect on the strength of H-bonding due to the variation of the inter-molecular interaction. The average reduced density gradient (aRDG) and thermal fluctuation index (TFI) methods are used further to study the change of intermolecular interaction in a dynamic environment and provide a good way to distinguish H-bond, vdW interaction, and steric effect. Finally, we consider the solvation of NaCl in these models. These studies can help us to further gain fundamental understanding of the microscopic origin of the water molecule during geometric evolution.
The remainder of this paper is organized as follows. In Section
The SPC/E water model[24] is used for the simulation in conjunction with classical molecular dynamics simulations. The force field parameters of the SPC/E model, Na+ and Cl− ion are presented in Table
| Table 1. Values of Lennard-Jones and electrostatic interaction potential parameters. e represents the magnitude of the electronic charge. . |
| Table 2. Modified water models. ∠HOH, rOH, and rHH are respectively the water molecule’s bond angle, bond length, and distance between two hydrogen atoms. Model (I) represents the change of the H–O–H angle. Model (II) represents the change of the O–H length. . |
The potential energy E between two molecules is written as a sum of pairwise intermolecular interactions and can be expressed as
 |
In our simulations, the following molecular dynamic simulation protocol is adopted. Every simulation cell contains 256 water molecules for pure solvent in a cubic box. The v-rescale thermostat[26] and Berendsen barostat[27] are used to regulate the temperature T at 298.15 K and pressure P at 1 atm (1 atm = 1.01325 × 105 Pa), with time constants of 0.2 ps and 0.5 ps, respectively. The leapfrog algorithm with a time step size 1 fs and the SHAKE algorithm[28] are used respectively to integrate the equation of motion and constrain the intramolecular geometry for the rigid models. The cutoff radius of the Lennard-Jones and Coulombic interactions is 9 Å. The Particle Mesh Ewald (PME) method is used to treat electrostatic interactions.[29,30] In the ion solution, the molarity of NaCl is 2.3 M. Every simulation cell contains 234 solvent molecules, 11 Na+, and 11 Cl− ions with cubic periodic boundary conditions. To simulate the variations introduced by ions, we use the same ensemble conditions as pure solvents. Finally, the resulting 70-ns NPT simulation is carried out for each case using GROMACS software.[31]
The reduced density gradient (RDG) and the thermal fluctuation index (TFI) are carried out by the Multiwfn program.[32] The RDG can be written as follows:
 |


Furthermore, the stability of weak interaction between molecules can be given by the TFI based on the aRDG and is defined as:
 |
 |
The H-bond lifetimes can be computed in different ways.[34,35] In the present study, we use a geometrical criterion to determine the existence of H-bonding as shown in Fig.
The value of 3.5 Å corresponds to the first minimum of the radial distribution functions (RDFs) of the SPC/E water model. The lifetime of the H-bonds is calculated from the average over all autocorrelation functions of the existence functions (either 0 or 1) of all H-bonds:
 |
 |
The local structure of liquid can be conveniently studied by the radial distribution functions (RDFs). In Fig.
 | Fig. 2. The RDFs of (a) oxygen–oxygen and (b) oxygen–hydrogen atom pairs for various bond length models. |
 | Fig. 3. The RDFs of (a) oxygen–oxygen and (b) oxygen–hydrogen atom pairs for various bond angle models. |
As shown in Fig.
Svishchev and Kusalik[36] used firstly the SDFs to study the local structure of water. Figure
As seen in Fig.
The parameters of orientational order ⟨q⟩ have often been used in recent years to investigate structural order in liquids and glasses.[37–40] The 
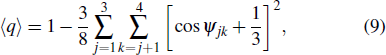 |
 |
As shown in Fig.
 | Fig. 5. Variation of the local order parameters ⟨q⟩ and quadrupolar interactions QT for various H–O–H angles, and O–H length models. |
The bond angle or length decrease, due to the weakening of the intermolecular attraction, resulting in the water molecule becoming more mobile. This indicates that the local tetrahedral order of water molecules is disrupted. Thus, ⟨q⟩ is reduced monotonically. In addition to the variation in gOO(r) [shown in Fig.
The self-diffusion constants of various models were calculated from their mean squared displacements
 |
The correlation of self-diffusion constants and H-bonding lifetime for various H–O–H angles and O–H length models are depicted in Fig.
 | Fig. 6. Variation of the self-diffusion constants and hydrogen bond lifetime for various H–O–H angles and O–H length models. |
To explore further the sensitivity to changes in bond length (elastic model) or bond angle (bent model), the data of the H-bonding lifetime in Fig.
From the mean squared displacement (MSD) as a function of time, Fig.
Weak interaction analysis between molecules or atoms, called non-covalent interaction analysis, has been recently developed by Contreras-Garcia et al.[44–47] It is based on an analysis of the electron density distribution in molecular systems in the regions of low electron density and low gradient. This approach is often referred to as reduced density gradient (RDG) analysis[37] and provides a good way to distinguish H-bond, vdW interaction, and steric effect.
Figure
Furthermore, the green isosurface around a water molecule exhibits in which direction this water tends to interact with other waters by vdW interaction, as shown in Figs.
In order to investigate the solution properties of water models with the geometry modified, ion solutions in different solvent environments are examined by adding NaCl ions. Use of the ion solution generally introduces two effects due to the ions on interactions: (i) it breaks the original solvent structures, making the solvent a little more mobile, and (ii) new hydration shells around ions reduce water’s diffusion constant.[49–52]
The RDFs of ion–ion and ion–water in the NaCl solutions of different solvents are given in Fig.
In this work, we have studied the liquid structure, dynamic, water molecule local weak interaction, and NaCl’s solution behavior of modified water models at 1 bar and 298.15 K. These models, based on alteration of the SPC/E model, have O–H lengths ranging from 0.90 Å to 1.10 Å (the H–O–H angles do not change) and H–O–H angles ranging from 90° to 115° (the O–H length changes from 0.82 Å to 1.08 Å). Both types of models have the same atomic charge and LJ parameters as the original SPC/E model.
To summarize, we find that the properties of the liquids undergo dramatic change caused by altering the water molecule geometry structure. Increasing the H–O–H angle and O–H length leads to: the structure of liquid becoming more highly-structured, raising the order parameter and QT, longer H-bond lifetime, and shorter atoms distance adjoining water molecules. In contrast, decreasing the H–O–H angle and O–H length results in a gradual changing of the liquid structure into being more like a Lennard-Jones liquid with spherical symmetrical local arrangement. The liquid exhibits a glassy-like state feature, when the SPC/E’s O–H length is increased by 0.1 Å. All these variations can be primarily attributed to the alteration of the intermolecular interaction strength between water molecules. However, the change in the intermolecular interaction strength is very sensitive to changes in the bond length.
Using the weak interaction and thermal fluctuation analysis methods, some interesting regions are obtained around a central water molecule. The scope of vdW (van der Waals) interaction with neighboring water molecules is reduced as the bond length and angle are decreased, and vice versa. A water molecule as a donor forms H-bonds with other water molecules leading to more stability, whereas the stability slightly reduces when the water acts as an H-bond acceptor. In addition, for the NaCl dissolving process, SPC/E water is an ideal solvent which dissolves ions and more easily couples with Na+ and Cl− ions than in other solvent environment models.
This study helps gain further fundamental understanding of the property changes of a water molecule by altering the water molecule geometry structure. Ongoing research will investigate the conformation transitions of large molecules such as DNA, protein, etc., in these modified models.
| [1] | |
| [2] | |
| [3] | |
| [4] | |
| [5] | |
| [6] | |
| [7] | |
| [8] | |
| [9] | |
| [10] | |
| [11] | |
| [12] | |
| [13] | |
| [14] | |
| [15] | |
| [16] | |
| [17] | |
| [18] | |
| [19] | |
| [20] | |
| [21] | |
| [22] | |
| [23] | |
| [24] | |
| [25] | |
| [26] | |
| [27] | |
| [28] | |
| [29] | |
| [30] | |
| [31] | |
| [32] | |
| [33] | |
| [34] | |
| [35] | |
| [36] | |
| [37] | |
| [38] | |
| [39] | |
| [40] | |
| [41] | |
| [42] | |
| [43] | |
| [44] | |
| [45] | |
| [46] | |
| [47] | |
| [48] | |
| [49] | |
| [50] | |
| [51] | |
| [52] |







