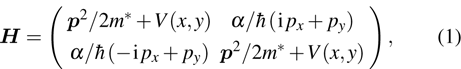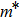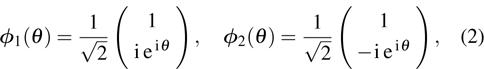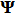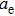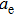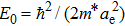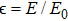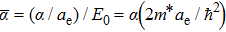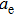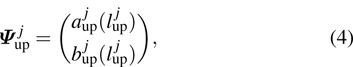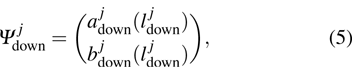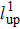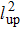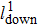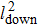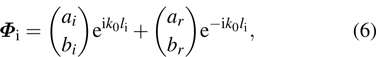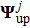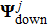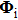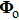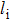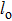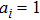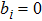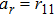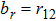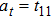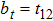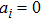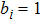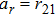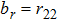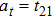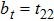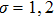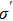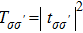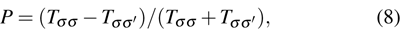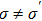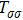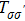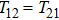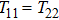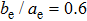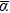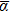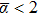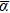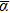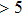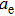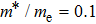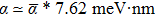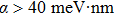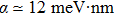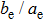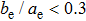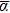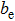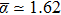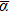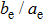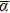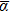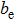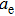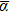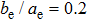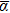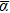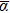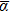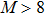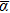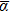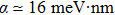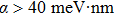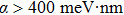1. IntroductionNowadays much of research in semiconductor spintronics has been shifting toward the field of the Rashba spin–orbit interaction (RSOI)[1] in low dimensional semiconductor structures.[2–9] Many low dimensional semiconductor structures with RSOI have been intensively studied,[10–13] and these structures are expected to be potential spin devices, such as spin-inverter or spin-polarizer. In these quantum structures, spin transport is a basic problem, so many investigations focus on ballistic macroscopic circular rings[14–20] as its one dimensional Hamiltonian.[21] In macroscopic circular rings, many intriguing spin interference phenomena[22,23] have been found. For instance, ballistic transport of Rashba electron through a chain of quantum circular rings has been investigated by Molnar et al.[16] They have shown that a periodic of spin transport is determined by the incident electronʼs energy E, the magnetic field B, and the strength of the RSOI α. Recently Naeimi et al. have shown that a double quantum rings in the presence of RSOI and a magnetic flux can work as a spin-inverter.[20]
In this paper, we study the spin transport of electrons in an elliptical ring with the RSOI, and the same case in an circular ring is studied for comparison. We focus on the spin flip in two kinds of rings and expect to find a suitable structure and conditions for a spin inverter.
2. Theoretical modelFor a ring in x–y plane, in the presence of Rashba spin–orbital interaction, the effective Hamiltonian of an electron in this two dimensional system could be written as
where
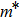
is the electron effective mass and
α is the Rashba coefficient. For a one-dimensional (1D) circular ring, the equation (
1) can be rewritten into a 1D form.
[21] With this 1D Hamiltonian, the eigenvalues, eigenstates, and spin transport problems could be easily solved. However, for other 1D rings, such as hexagonal or elliptical rings, we cannot find 1D Hamiltonian. To solve problems of spin transport in these structures, we adopted the method of dividing a curved line into
N segments.
[24] For a curved line, such as an elliptical ring or a circular ring,
N is large enough and every segment is very small, so each segment can be approximated to be a line segment along the tangential direction. For every linear segment, we could easily obtain its eigenstates
[25]where
θ is the azimuthal angle of the segment. Based on Eq. (
2), we can write the wavefunction in the curve as
where
l denotes the coordinates on the curved line and
θ is the azimuthal angle of the tangent line of the curve. By Adopting
to describe the wave function and by using the Griffithʼs boundary conditions
[24–27] in each vertex, we can relate the wave function at the two endpoints by a transfer matrix. For a polyline structure, such as a hexagonal ring, every lead is a natural line segment, so
N is the number of the leads. In circular ring this method gave results which are identical with those obtained from 1D Hamiltonian,
[24] so this method is reasonable.
In each arm of an Aharonov–Bohm (AB) ring, the transfer matrix connects the wave functions at a junction with the wave function at the other junction. Since the wave function must be continuous and the spin current must be conserved at the junctions, we can just determine all unknown coefficients when an electron beam is injected into the AB ring. In our method, rings with any shape could be easily dealt with. For instance, spin transport in a polyline structure is more convenient to be calculated in our method.
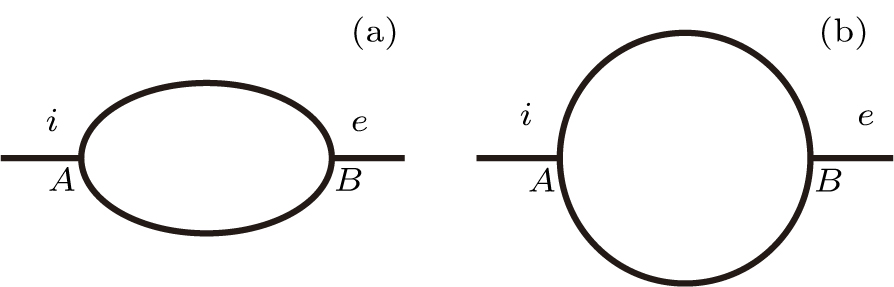
Consider an 1D AB ring, such as an elliptical ring as shown in Fig. 1(a), the electron current is injected from circuit i at point A, and output circuit e is at the right side with intersection point B. We assume that the semimajor axis and the semiminor axis of the ellipse are denoted by
 and
and
 respectively, and the strength of RSOI in the ring is denoted by α. The size of the AB ring can be denoted by the distance between point A and point B, so the characteristic length of the elliptical ring can be denoted by
respectively, and the strength of RSOI in the ring is denoted by α. The size of the AB ring can be denoted by the distance between point A and point B, so the characteristic length of the elliptical ring can be denoted by
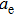 . In this paper, we adopt the dimensionless physical quantities. Taking the energy unit
. In this paper, we adopt the dimensionless physical quantities. Taking the energy unit
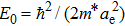 , the energy
, the energy
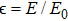 and the Rashba coefficient
and the Rashba coefficient
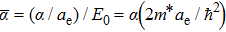 .For a circular ring as shown in Fig. 1(b), their dimensionless physical quantities are similar, just with
.For a circular ring as shown in Fig. 1(b), their dimensionless physical quantities are similar, just with
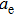 replaced by the radius of the circle R. For any 1D AB ring, the wave function in the upper and lower arms can be divided into two components: one in clockwise direction and another in counterclockwise direction, so they can be written as
replaced by the radius of the circle R. For any 1D AB ring, the wave function in the upper and lower arms can be divided into two components: one in clockwise direction and another in counterclockwise direction, so they can be written as
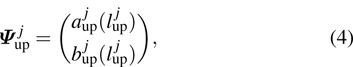 | |
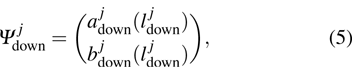 | |
where up and down denote the upper and lower arms of the AB ring and
j = 1,2 correspond to the clockwise and counterclockwise directions, respectively. In particular,
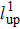
and
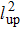
,or
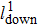
and
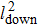
have opposite positive direction. The wavefunction of the electron in the input and output lead can be written as
According to the transfer matrix method, we can obtain
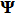 of each arm if we know its value at any one point, so there are 12 unknown coefficients in
of each arm if we know its value at any one point, so there are 12 unknown coefficients in
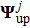 ,
,
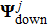 ,
,
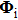 ,and
,and
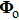 . Using Griffithʼs boundary conditions,[25–27] we can determine all unknown coefficients. We assume that the original point of
. Using Griffithʼs boundary conditions,[25–27] we can determine all unknown coefficients. We assume that the original point of
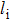 and
and
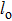 are point A and point B, respectively. When
are point A and point B, respectively. When
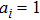 and
and
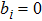 ,we have
,we have
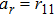 ,
,
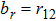 ,
,
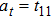 , and
, and
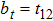 . Similarly, for
. Similarly, for
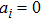 and
and
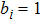 , we have
, we have
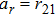 ,
,
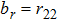 ,
,
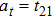 , and
, and
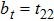 ,where
,where
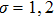 denote spin up and down states that are quantized in z direction. The spin dependent transmission coefficient of an electron with incoming spin σ and outgoing spin
denote spin up and down states that are quantized in z direction. The spin dependent transmission coefficient of an electron with incoming spin σ and outgoing spin
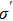 can be written as
can be written as
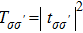 .
.
For a spin-inverter, we expect that T12 and T21 are as large as possible and T11 and T22 to be the opposite. Therefore the spin flip degree could be given as[20]
where
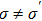
. For
P = 1, it means the conservation of the spin degree of freedom during the transition of the spin current.
P = −1 means the spin flip during the spin injection and transmission. Now there is a problem that for
σ = 1,2,
P not always has the same value, and for a spin-inverter,
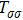
and
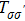
should be identical for spin up or spin down injection. We have calculated the spin transport in many configurations, and find that
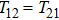
is satisfied in any case, and
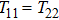
is satisfied in the absence of the perpendicular magnetic field. Considering this fact, we can expect an AB ring as a spin-inverter just in the presence of RSOI.
3. Results and discussionIn previous work, we found that P is associated with
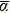 only in a certain AB ring, which possesses the same behavior as the Datta spin filed effect transistor.[28] In this paper, we are intent to compare spin flip in different AB rings, especially in the elliptical ring and in the circular ring.
only in a certain AB ring, which possesses the same behavior as the Datta spin filed effect transistor.[28] In this paper, we are intent to compare spin flip in different AB rings, especially in the elliptical ring and in the circular ring.
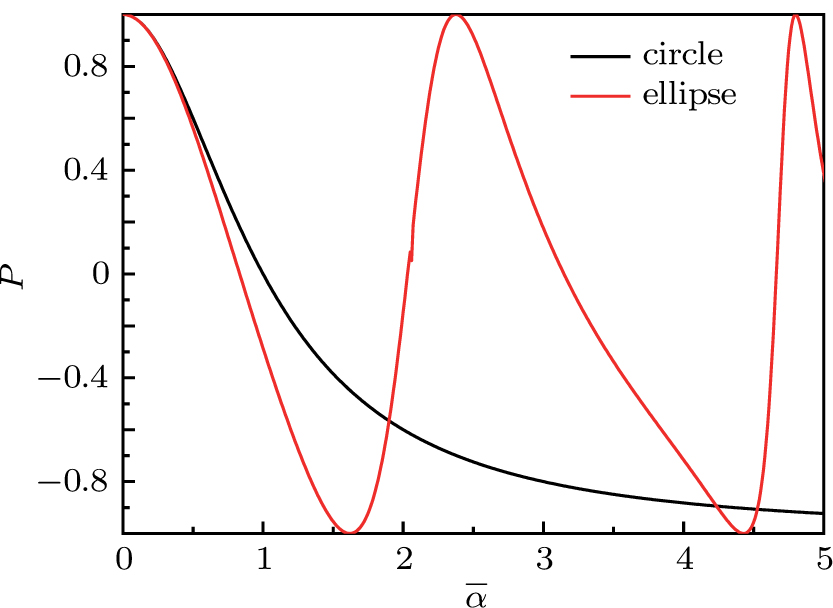
In Fig. 2, we show P as a function of the RSOI strength
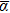 in the elliptical ring and in the circular ring. The relevant parameters in our calculation are ε = 5 and
in the elliptical ring and in the circular ring. The relevant parameters in our calculation are ε = 5 and
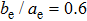 for the elliptical ring. We can see that P in the elliptical ring oscillates between −1 and 1 when
for the elliptical ring. We can see that P in the elliptical ring oscillates between −1 and 1 when
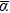 changes. This means that as a spin-inverter, the elliptical ring is sensitive and can work in a reasonable range of
changes. This means that as a spin-inverter, the elliptical ring is sensitive and can work in a reasonable range of
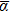 , i.e.
, i.e.
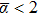 . P in the circular ring has a different expression because it decreases monotonously when
. P in the circular ring has a different expression because it decreases monotonously when
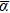 increases, and it has asymptotic value −1. Therefore, in a single circular ring it is unrealistic to modulate the spin of the emergent electron completely. Only when
increases, and it has asymptotic value −1. Therefore, in a single circular ring it is unrealistic to modulate the spin of the emergent electron completely. Only when
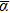 is very large (
is very large (
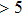 ), P can reach a value less than −0.9. For an AB ring with
), P can reach a value less than −0.9. For an AB ring with
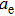 (/R) =50 nm,
(/R) =50 nm,
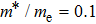 and
and
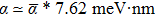 . So to make the AB ring work as a spin-inverter well, we need a RSOI strength
. So to make the AB ring work as a spin-inverter well, we need a RSOI strength
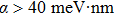 in a circular ring, but in a elliptical ring we just need
in a circular ring, but in a elliptical ring we just need
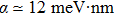 . On the other hand, if a large α can be reached, a spin-inverter based on a circular ring can work in a vast range of α, so it has a high stability.
. On the other hand, if a large α can be reached, a spin-inverter based on a circular ring can work in a vast range of α, so it has a high stability.
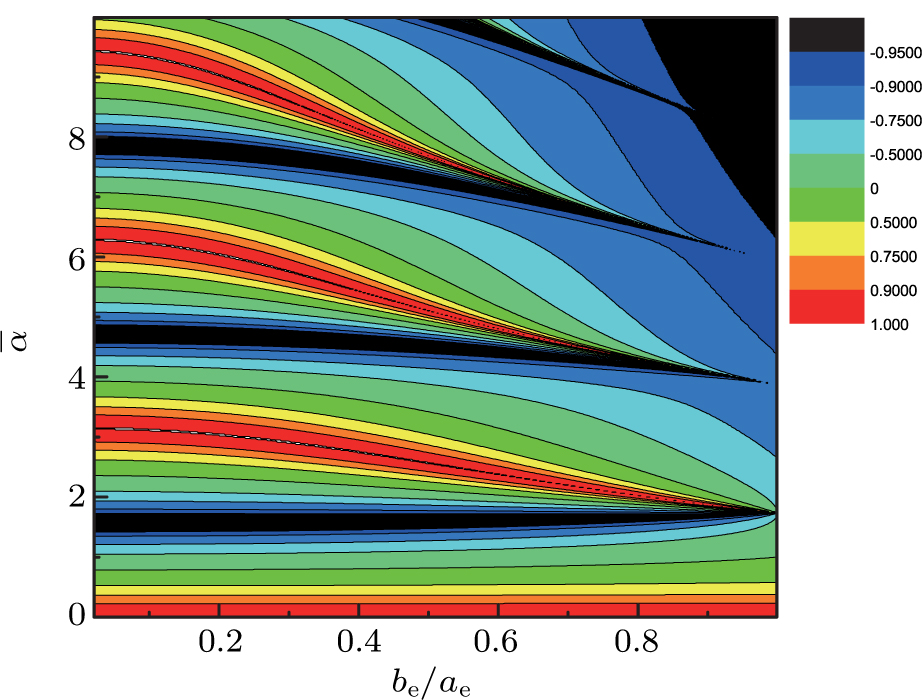
To study the influence of the geometry on the spin transport ulteriorly, we compare P in elliptical rings with different eccentricities. The contour maps of P as a function of the RSOI strength
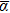 and the semiminor axis
and the semiminor axis
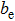 in a elliptical ring are shown in Fig. 3. Here the energy of the electron ε=5. We find that if
in a elliptical ring are shown in Fig. 3. Here the energy of the electron ε=5. We find that if
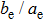 is small (as
is small (as
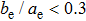 ), spin inversion can occur in some regions of
), spin inversion can occur in some regions of
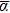 , and these regions are nearly the same for all
, and these regions are nearly the same for all
 , such as
, such as
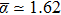 . For these cases, P oscillates between 1 and −1 when
. For these cases, P oscillates between 1 and −1 when
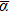 increases, but if
increases, but if
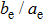 is close to 1, i.e. the elliptical ring is close to a circular one, P decreases monotonically and has a asymptotic value −1 when
is close to 1, i.e. the elliptical ring is close to a circular one, P decreases monotonically and has a asymptotic value −1 when
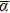 increases. As
increases. As
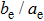 increases, P as a function of the RSOI strength
increases, P as a function of the RSOI strength
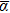 changes more and more gently. We infer that the increase of the transverse size of the ring weakens the quantum confinement effect, and then undermines the interference of two spin states in the longitudinal dimension.
changes more and more gently. We infer that the increase of the transverse size of the ring weakens the quantum confinement effect, and then undermines the interference of two spin states in the longitudinal dimension.
To produce ideal ellipses is virtually impossible, and to produce an inscribed polygon of the ellipse is a concise and effective method. To consider this possible geometry, we calculated P in the inscribed polygon of the elliptical ring and the circular ring. We assume that one focus of the elliptical ring and the centre of the circular ring are poles of polar coordinates in each system, respectively. Every edge of the inscribed polygon has the same polar angle. Because two arms of AB rings in this paper are symmetrical, we can assume that each arm of the ring is replaced by a M section polyline. Obviously the inscribed polygon of the circular ring will be a regular polygon of 2M sides.
The contour maps of P as a function of the RSOI strength
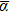 and half of the edges’ number of the inscribed polygon M in the elliptical ring and the circular ring are shown in Fig. 4(a) and Fig. 4(b), respectively. Here the energy of the electron ε = 5 and
and half of the edges’ number of the inscribed polygon M in the elliptical ring and the circular ring are shown in Fig. 4(a) and Fig. 4(b), respectively. Here the energy of the electron ε = 5 and
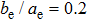 in the elliptical ring. In Fig. 4(a), we find that the influence of the edges’ number on P is very small in the inscribed polygon of the elliptical ring, and for every inscribed polygon, P has nearly the same curve followed the RSOI strength
in the elliptical ring. In Fig. 4(a), we find that the influence of the edges’ number on P is very small in the inscribed polygon of the elliptical ring, and for every inscribed polygon, P has nearly the same curve followed the RSOI strength
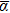 . This result shows that an AB ring similar to a elliptical ring in shape can replace the elliptical ring and work as a spin-inverter with a small α. The result is different in the circular ring. Figure 4(b) shows that the curve of P as
. This result shows that an AB ring similar to a elliptical ring in shape can replace the elliptical ring and work as a spin-inverter with a small α. The result is different in the circular ring. Figure 4(b) shows that the curve of P as
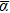 changes has changed greatly when M increases. If M is small, for example the inscribed polygon is square, hexagon, or octagon, P oscillates between 1 and −1. When
changes has changed greatly when M increases. If M is small, for example the inscribed polygon is square, hexagon, or octagon, P oscillates between 1 and −1. When
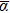 increases, P can reach −1 at a low value of
increases, P can reach −1 at a low value of
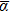 (about 2–3). If M is relatively large, such as
(about 2–3). If M is relatively large, such as
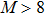 , P decreases monotonically and has a asymptotic value −1 when
, P decreases monotonically and has a asymptotic value −1 when
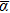 increases, and to reduce P to the same value, the required value of
increases, and to reduce P to the same value, the required value of
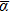 increases when M increases. This result is similar to the result in Fig. 3, and shows that as the limit case of elliptical rings and of inscribed polygons, the circular ring has much different spin transport character from elliptical rings and inscribed polygons. In addition, Figure 4 shows that there is a limit result of spin transport in the inscribed polygon of the elliptical ring and the circular ring when M becomes very large, so our method in which a curve is divided into many segments is self-consistent.
increases when M increases. This result is similar to the result in Fig. 3, and shows that as the limit case of elliptical rings and of inscribed polygons, the circular ring has much different spin transport character from elliptical rings and inscribed polygons. In addition, Figure 4 shows that there is a limit result of spin transport in the inscribed polygon of the elliptical ring and the circular ring when M becomes very large, so our method in which a curve is divided into many segments is self-consistent.
The elliptical ring or the regular polygon with a small number of edges (such as a regular hexagon) can work as a spin-inverter with the normalized Rashba constant about 2, which corresponds to RSOI strength
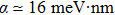 . This value is in the range of that obtained experimentally in InGaAs,[29,30] so these spin-inverters are realizable. A spin-inverter of the circular ring requires
. This value is in the range of that obtained experimentally in InGaAs,[29,30] so these spin-inverters are realizable. A spin-inverter of the circular ring requires
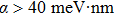 , which is hard to achieve in traditional III–V semiconductor. However, recently giant RSOI with
, which is hard to achieve in traditional III–V semiconductor. However, recently giant RSOI with
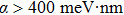 has been obtained in the bulk Rashba semiconductor BiTeI.[31] Although the requirement is harsh, the value of RSOI strength required in the circular ring will be possible to reach in more common situation in the near future.
has been obtained in the bulk Rashba semiconductor BiTeI.[31] Although the requirement is harsh, the value of RSOI strength required in the circular ring will be possible to reach in more common situation in the near future.
4. ConclusionWe have studied spin transport, especially spin flip in single quantum ring with RSOI to find suitable geometry and other conditions for a spin-inverter. The elliptical ring and the circular ring are studied as typical AB rings. We found that if these two kinds of ring work as spin-inverters, they have much different character. The elliptical ring can realize spin flip with a relatively small RSOI strength α, but the spin flip degree P will oscillate quickly and the spin-inverter can only work in a small range of α. The circular ring can not realize spin flip perfectly, but P in circular ring can be very close to −1 with a relatively large α, and it is insensitive to α at this time. In addition, we have investigated the influence of the defect of the AB ringʼs geometry on the spin transport. Results show that in an elliptical ring spin transport is insensitive to the defect of the ring, but in a circular ring, the defect should be small to give P closed to that of the ideal circular ring. These results show that if RSOI strength is relatively small and can be controlled well, the elliptical ring is a good choice for a spin-inverter, and we just need a approximate ellipse. If large RSOI strength is realized, a circular ring can work as a spin-inverter with a high stability.