Cite this Article
Liu Shuang, Ma Yan-Zhen, Yang Yun-Fan, Liu Song-Song, Li Yong-Qing, Song Yu-Zhi. Excited state intramolecular proton transfer mechanism of o-hydroxynaphthyl phenanthroimidazole. Chinese Physics B, 2018, 27(2): 023103  
Permissions
Excited state intramolecular proton transfer mechanism of o-hydroxynaphthyl phenanthroimidazole
Liu Shuang1, Ma Yan-Zhen2, Yang Yun-Fan2, Liu Song-Song1, Li Yong-Qing2, †, Song Yu-Zhi1, ‡
School of Physics and Electronics, Shandong Normal University, Jinan 250014, China
Department of Physics, Liaoning University, Shenyang 110036, China

 

† Corresponding author. E-mail: yqli@lnu.edu.cn yzsong@sdnu.edu.cn

Abstract

By utilizing the density functional theory (DFT) and the time-dependent density functional theory (TDDFT), the excited state intramolecular proton transfer (ESIPT) mechanism of o-hydroxynaphthyl phenanthroimidazole (HNPI) is studied in detail. Upon photo is excited, the intramolecular hydrogen bond is obviously enhanced in the S1 state, which thus promotes the ESIPT process. Hydrogen bond is shown to be strengthened via comparing the molecular structures and the infrared vibration spectra of the S0 and S1 states. Through analyzing the frontier molecular orbitals, we can conclude that the excitation is a type of the intramolecular charge transfer excitation, which also indicates the trend of proton transfer in S1 state. The vertical excitation based on TDDFT calculation can effectively repeat the absorption and fluorescence spectra of the experiment. However, the fluorescence spectrum of normal structure, which is similar to the spectrum of isomer structure is not detected in the experiment. It can be concluded that the fluorescence measured in the experiment is attributed to both structures. In addition, by analyzing the potential energy curves (PECs) calculated by the B3LYP functional method, it can be derived that since the molecule to cross the potential barrier in the S1 state is smaller than in the S0 state and the reverse proton transfer process in the S1 state is more difficult than in the S0 state, the ESIPT occurs in the S1 state.

PACS: 31.15.ae;31.15.A-;31.15.es
Keyword:hydrogen bond;ESIPT;RDG;PECs
1. Introduction

As one of the cases of most important weak interactions, hydrogen bond plays quite a vital role in the study of photochemistry, such as supramolecular photochemistry, material photochemistry, biochemistry, and photochemistry of solution, etc.[1–5] Since the pioneering work by Weller et al.,[6,7] the excited state intra- and inter-molecular proton transfer (ESIPT) have become a research focus in experiment and in theory.[1,2,8–11] For example, Zhao et al. have been deciphered the excited state behavior for 2- ( -N, N-dimethylaminophenyl) imidazo [4, 5-b] pyridine.[12] Specifically, Han et al.[13–18] first reported that the hydrogen bond was strengthened on a 200-fs time scale, which broke the commonly accepted hydrogen bond dissociation mechanism, and proposed the strengthening mechanism of hydrogen bonding by studying excited state proton transfer.[10,11] Once a molecule is excited to the lowest excited state (enol* form) from the ground state (enol form), the hydrogen bond is first enhanced, proton will transfer between proton acceptor and donor through intra- or inter-molecular hydrogen bond. Then, the keto* form becomes the ground-state keto structure by emitting a longer wavelength fluorescence.[19–21] Finally ground-state keto form transfers back to the ground-state enol species via a reverse ground-state proton transfer process, which constitutes a four-level reaction cycle. The ESIPT thus possesses different photophysical and photochemical properties providing various applications in some areas, such as in luminescent materials, metal ion sensors, fluorescent probes, photonabilium, laser fuels, UV filters, light-emitting diodes, molecular switches, photostabilization, radiation hard-scintillator counters, organic light emitting devices (OLEDs), etc.[22–28]

Since the phenanthroimidazole derivatives have good molecular structural characteristics, they have aroused the great research interest of researchers. By using the TiO2(R) nano-semiconductor as the catalyst under solventless condition, Jayabharathi et al.[29] synthesized five phenanthroimidazole derivatives, which were then characterized via nuclear magnetic resonance (NMR) and single crystal x-ray diffraction (XRD) techniques. Shahid and Misra[30] synthesized three molecular systems based phenanthroimidazole moiety and studied photo enolization via ESIPT mechanism. The results revealed that the o-hydroxynaphthyl phenanthroimidazole (HNPI) and o-hydroxyphenyl phenanthroimidazole (HPPI) show light triggered enolization. However, benzyl phenanthroimidazole (BPNI) could not show the phenomenon of proton transfer due to the absence of OH moiety. Specifically, fluorescence “turn-off” and “turn-on” behavior could occur when HNPI interacts with AcO−, which thus could be used as a probe of AcO−.

Although Shahid and Misra[30] experimentally studied in detail the photo enolization process of HNPI triggered by the ESIPT mechanism, they obtained only the geometry optimization and frontier molecular orbital by quantum chemical calculation, which cannot be quantitatively nor qualitatively explain the ESIPT mechanism. Thus we are motivated to carry out a clear and detailed theoretical investigation on ESIPT of HNPI by utilizing DFT and TDDFT methods.[31] We study the excited state dynamical process of HNPI by performing detailed calculation on the properties of both the ground and excited states, such as the geometry optimization, potential energy curves, the frontier molecular orbital, reduced density gradient (RDG), IR spectrum, absorption and emission spectra, etc. We investigate the ESIPT mechanism of HNPI in detail, which is not only to clarify excited state dynamical process of HNPI system, but also to facilitate the understanding of intramolecular hydrogen bond dynamical processes.

The rest of this paper is organized as follows. In Section 2 we describe the computational details used in the present work. In Section 3, we describe in detail the results and discussion. The final remarks of the current work are presented in Section 4.

2. Computational details

The S0 and S1 state optimizations of HNPI molecule are carried out by using the DFT and TDDFT methods, which are implemented in the Gaussian 09 program suite.[31] B3LYP[32] functional and 6-31+G (d) basis set[33] are employed in both the DFT and TDDFT calculations. In order to simulate the environment of the acetonitrile solvent, the polarizable continuum model (PCM) and integral equation formalism variant PCM (IEFPCM)[34–37] are employed in the present work, which can reproduce the experimental results well. All the bond lengths, bond angles and dihedral angles are relaxed in the optimization of HNPI molecule in S0 and S1 states. In order to verify the structures corresponding to local minima in S0 and S1 states, the vibrational frequencies are calculated, which give no imaginary frequency. By using the optimized geometry of ground-state HNPI molecule, the vertical excitations are obtained by TDDFT calculation considering ten low-lying electronic states.[1,16,18,38–42] The PECs of S0 and S1 states are scanned,[43] which follows a constrained optimization by varying O–H bond length in the enol form ranging from 0.9 Å to 1.9 Å in steps of 0.03 Å. The vibration frequencies are determined via the diagonalization of the Hessian matrix. The gradients of dipole moment are utilized to determine the infrared (IR) intensity. In order to distinguish among the different types of interactions in HNPI molecule, the reduced density gradient (RDG) is calculated using Multiwfn.[44] Such as RDG is then visualized, from which the hydrogen bond can be easily recognized.

3. Results and discussion
3.1. Optimization of HNPI

The enol and keto forms are optimized employing DFT and TDDFT methods in ground (S0) and excited (S1) states of HNPI, respectively, which is subsequently followed by vibrational frequency analysis in order to validate such stationary points. The optimized enol and keto structures in ground state are shown in Fig. 1. For illustrating the change of hydrogen bond more clearly and concisely, the atoms related to hydrogen bond are marked with serial numbers as shown in Fig. 1. The corresponding bond lengths and bond angles of HNPI are listed in Table 1. For the enol form, it can be seen from Table 1 that O1–H2 bond length is 0.998 Å in S0 state, which increases to 1.001 Å in the first excited state (S1). In the meanwhile, the H2–N3 bond length decreases from 1.727 Å to 1.692 Å, and the O1-H2 ⋯ N3 bond angle increases from 146.8° to 147.7°. The decrease of the H2–N3 bond length and the increase of the O1–H2 bond length as well as the increase of bond angle O1-H2 ⋯ N3 in the S1 state lead to the hydrogen bonding enhancement. For the keto form, the O1–H2 bond length increases by 0.117 Å from S0 state to S1 state. While the H2–N3 bond length and O1 ⋯ H2–N3 bond angle decrease by 0.01 Å and 4.3°, respectively. It can also be concluded that the hydrogen bond in the S0 state is stronger than in S1 state.

Fig. 1. (color online) Optimized structures of HNPI molecules of enol and keto forms in S0 state: blue: H, yellow: C, red: O, pink: N. The dash line refers to the intramolecular hydrogen bond.
Table 1.

Primary optimized bond lengths (in unit Å) and bond angles (°) of hydrogen bond groups for normal form (enol) and tautomeric forms (keto) of the HNPI in S0 and S1 states.

.

A clear-cut feature of hydrogen bond dynamics can be obtained by analyzing the vibrational frequency of O1–H2 moiety which is related to the O1–H2 ⋯ N3. It is well known that IR spectrum is an effective method to determine whether the proton transfer occurs, which is judged by the blue shift or red shift of the IR spectrum.[45] Thus, the IR spectra of HPNI in enol form are calculated both for the S0 state and for the S1 state, which is shown in Fig. 2. The O1–H2 stretching vibrational frequency in S0 state is calculated to be 3165 cm−1, which then becomes 3079 cm−1 when HPNI is excited to S1 state, showing a red shift of 86 cm−1. The red shift of O1–H2 stretching vibrational frequency also shows that the intramolecular hydrogen bond O1–H2 ⋯ N3 is reinforced in S1 state.

Fig. 2. (color online) IR spectra of enol-form HPNI at the spectral region of the O1–H2 stretching band in the S0 and S1 states.
3.2. Frontier molecular orbital (MO) analyses

The frontier molecular orbitals (mainly the highest occupied molecular orbital (HOMO) and the lowest unoccupied molecular orbital (LUMO)) are calculated and analyzed for better understanding the charge distribution and charge transfer in the HNPI molecule. In Fig. 3, we display the calculated HOMO and LUMO of the HPNI molecule in the enol form. The calculated electronic transition energies, oscillator strengths and compositions are listed in Table 2. The oscillator strength of the first excited state is calculate to be 0.6050 with the orbital contribution being 97.94%, which is much larger than those of the second and third excited states. It can be seen from Fig. 3 that the HOMO displays π feature, while the LUMO exhibits π* feature, thus the transition of two orbitals shows * character in the first excited state, it can also be observed that the electron distributions in HOMO and LUMO are different from each other. The electron distribution of O1–H2 bond is reduced, whereas the electron distribution of the N3 atom is increased, which strengthens the hydrogen bonding and promotes the ESIPT process.[46,47] In addition, the electron population of the HOMO is distributed on the whole molecule, while the electrons of LUMO are distributed mainly only on the right moiety, which exhibits a distinct intramolecular charge transfer (ICT). Thus, we can conclude that the excitation type belongs to intramolecular charge transfer excitation.

Fig. 3. (color online) Visual electron population of the frontier molecular orbitals HOMO and LUMO.
Table 2.

Excitation wavelengths, oscillator strengths and the corresponding orbital transition contributions of HNPI molecule.

.
3.3. Absorption and emission spectra

The absorption and fluorescence spectra of HNPI molecule are displayed in Fig. 4 with wavelengths ranging from 200 nm to 800 nm. The peaks of calculated absorption spectra are located at 378.8 nm, which is consistent with the experiment result[48] 370 nm, showing a minor difference of 8.8 nm. As for the emission spectra, the peak of keto* form is calculated to be 482.1 nm which is 35.9 nm smaller than the experimental result.[48] The fluorescence spectrum of enol* form at 476.2 nm which is similar to the keto* form is not detected in the experiment, thus the fluorescence measured in the experiment is attributed to both structures. The consistence between the absorption and emission peaks ensures the validity of the method employed in the present work. The abnormal change of photophysical property is very often along with tremendous change in molecular structure compared with in normal structure. Abnormal change in the optical characteristic is often accompanied by a huge change in the molecular structure compared with in the normal structure. Therefore, we can conclude that the change in Stokes shift reflects the change in photophysical property.

Fig. 4. (color online) Absorption (abs) and fluorescence (flu.) spectra of HNPI, obtained at the calculated level of B3LYP/6-31+G (d)/IEF-PCM (acetonitrile).
3.4. Potential energy curves

For a more comprehensive understanding of the process that the proton migrates from the hydroxyl group to nitrogen atom, we plot in Fig. 5 that the PECs as a function of O1–H2 bond ranging from 0.9 Å to 1.9 Å in steps of 0.03 Å. It can be derived from Fig. 5 that the proton transfer may overcome a potential barrier about 5.76 kcal/mol in the S0 state, which is slightly bigger than that in S1 state (5.12 kcal/mol). However, the reverse proton transfer immediately occurs after proton transfer. The potential barrier of reversed proton transfer is 2.2 kcal/mol in the S0 state, which is much smaller than that of S1 state (4.28 kcal/mol), thus it is unstable and returns to the enol form quickly. This demonstrates that the reversed proton transfer process is more difficult to occur in the S1 state than in the S0 state. Subsequently, keto* form returns to ground-state keto form via radiation fluorescence.

Fig. 5. (color online) Curves of potential energy versus O1–H2 bondlength in a frequency range from 0.9 Å to 1.9 Å of the HNPI molecule in S0 and S1 states.
3.5. Discriminating weak interaction types by filling color to RDG isosurfaces

By combining the RDG function and the function, we can distinguish between the type and intensity of weak interaction.[49] The equation can be expressed as

where RDG is the reduced density gradient and is the total electron density. According to atom in molecule (AIM) theory,[50] the relationship between the second largest eigenvalue λ2 and of the electron density Hessian matrix as shown in Eq. (2), is an important measure of weak interaction intensity, while the type of weak interaction is represented by the sign ( function. Therefore, the weak interactions of different types can be analyzed by drawing the scatter graph of function 1 (RDG) and function 2 ( ). The function obtained by multiplying the function of and sign ( and thus yielding the RDG isosurface is shown in Fig. 6(a) with a contour value of 0.5 and the RDG isosurface ranging from −0.04 to 0.02. The spike of the hydrogen bond is located at −0.036 a.u., which indicates that the hydrogen bond is extremely strong in the S1 state. Similarly, the weak interactions can be distinguished in Fig. 6(b). It can be explicitly observed from Fig. 6(b) that there is a strong hydrogen bond between .

Fig. 6. (color online) (a) Plot of reduced density gradient (RDG) versus for function value 1 and function value 2. (b) Different types of weak interactions represented by different color gradients as well as RDG isometric surfaces.
4. Conclusions and perspectives

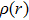
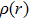
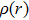
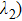
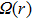
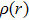
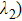
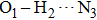
The DFT/TDDFT/B3LYP functional method is utilized to study the ESIPT mechanism of HNPI molecule. We can conclude that the proton transfer occurs in the S1 state via analyzing the calculated electron spectrum, infrared spectrum and potential energy curves. In addition, by analyzing the bond lengths, bond angles and the frontier molecular orbitals in the S0 state and the S1 state, respectively, we can prove that the hydrogen bond interaction can be strengthened, which plays a decisive role in proton transfer. In addition, we carry out a detailed study of the proton transfer process of HNPI molecule by calculating the PECs of S0 and S1 states. The obtained potential barriers of reversed proton transfer are 4.28 kcal/mol in the S1 state and 2.2 kcal/mol in the S0 state, respectively. Thus it can be concluded that the reverse proton transfer process in the S0 state is more difficult than in the S1 state. Therefore, we can derive from the above analysis that the proton transfer occurs in the S1 state, which is of great significance for doing molecular research.

Reference
[1] Zhao G J Han K L 2008 J. Comput. Chem. 29 2010
[2] Zhao G J Han K L Stang P J 2009 J. Chem. Theory. Comput 5 1955
[3] Zhao G J Han K L 2008 Biophys. J. 94 38
[4] Zhao G J Han K L 2010 Phys. Chem. Chem. Phys. 12 8914
[5] Zhou P Song P Liu J Han K He G 2009 Phys. Chem. Chem. Phys. 11 9440
[6] Weller A Elektrochem Z 1956 Phys. Chem. 60 1144
[7] Weller A 1955 Naturwissenschaften 42 175
[8] Han K L He G Z Lou N Q 1996 J. Chem. Phys. 105 8699
[9] Li D Huang X Han K Zhan C G 2011 J. Am. Chem. Soc. 133 7416
[10] Yang Y Zhao J Li Y 2016 Sci. Rep. UK 6 32152
[11] Zhang Y Sun M Li Y 2016 Sci. Rep. UK 6 25568
[12] Zhao J Liu X Zheng Y 2017 J. Lumin. 188 1
[13] Zhao G J Han K L 2007 J. Chem. Phys. 127 024306
[14] Chai S 2009 Phys. Chem. Chem. Phys. 11 4385
[15] Zhao G J Liu Y H Han K L Dou Y 2008 Chem. Phys. Lett. 453 29
[16] Zhao G J Han K L 2012 Acc. Chem. Res. 45 404
[17] Zhao G J Han K L 2007 J. Phys. Chem. 111 2469
[18] Zhao G J Han K L Lei Y B Dou Y S 2007 J. Chem. Phys. 127 415
[19] Song P Ma F C 2013 Int. Rev. Phys. Chem. 32 589
[20] Demchenko A P Tang K C Chou P T 2013 Chem. Soc. Rev. 42 1379
[21] Tseng H W 2015 J. Phys. Chem. Lett. 6 1477
[22] Chen J S Zhou P W Yang S Q Fu A P Chu T S 2013 Phys. Chem. Chem. Phys. 15 16183
[23] Yu F Li P Wang B Han K 2013 J. Am. Chem. Soc. 135 7674
[24] Ji E K Park S Y 2011 Adv. Mater. 23 3615
[25] Tang K C 2011 J. Am. Chem. Soc. 133 17738
[26] P S S J G Y P T E V 2009 J. Phys. Chem. 113 4953
[27] Li Y Xu B Song P Ma F C Sun M 2017 J. Phys. Chem. 121 12546
[28] Xu B Li Y Peng S Ma F Sun M 2017 Sci. Rep. UK 7 45688
[29] Jayabharathi J Ramanathan P Thanikachalam V 2014 New J. Chem. 39 142
[30] Shahid M Misra A 2016 J. Photoch. Photobio. 335 190
[31] Frisch M J 2009 Gaussian 09, Revision A.02 Wallingford CT Gaussian, Inc.
[32] Lee C Yang W Parr R G 1988 Phys. Rev. 37 785
[33] Rassolov V A Ratner M A Pople J A Redfern P C Curtiss L A 2001 J. Comput. Chem. 22 976
[34] Cammi R Tomasi J 1995 J. Comput. Chem. 16 1449
[35] Cancès E Mennucci B Tomasi J 1997 J. Chem. Phys. 107 3032
[36] Johnson E R 2010 J. Am. Chem. Soc. 132 6498
[37] Tang W Sanville E Henkelman G 2009 J. Phys. Condens. Matter 21 084204
[38] Hui L 2015 Spectrochim. Acta. 141 211
[39] Zhao X Chen M 2011 Chem. Phys. Lett. 512 35
[40] Zhao G J Northrop B H Han K L Stang P J 2010 J. Phys. Chem. 114 9007
[41] Liu Y H Lan S C Zhu C Lin S H 2015 J. Phys. Chem. 119 6269
[42] Chou P Mcmorrow D Aartsma T J Kasha M 1984 J. Phys. Chem. 88 370
[43] Liu Y H Mehata M S Lan S C 2014 Spectrochim. Acta 128 280
[44] Lu T Chen F 2012 J. Comput. Chem. 33 580
[45] Zhao J Ji S Chen Y Guo H Yang P 2012 Phys. Chem. Chem. Phys. 14 8803
[46] Grabowski Z R Rotkiewicz K Rettig W 2003 Chem. Rev. 103 3899
[47] Marcus R A 1968 Electrochim. Acta 13 995
[48] Shahid M Misra A 2017 J. Photoch. Photobio. 335 190
[49] Johnson E R 2010 J. Am. Chem. Soc. 132 6498
[50] Tang W Sanville E Henkelman G 2009 J. Phys.: Condens. Matter 21 084204