† Corresponding author. E-mail:
Nitrogen–vacancy (NV) center in diamond is one of the most promising candidates to implement room temperature quantum computing. In this review, we briefly discuss the working principles and recent experimental progresses of this spin qubit. These results focus on understanding and prolonging center spin coherence, steering and probing spin states with dedicated quantum control techniques, and exploiting the quantum nature of these multi-spin systems, such as superposition and entanglement, to demonstrate the superiority of quantum information processing. Those techniques also stimulate the fast development of NV-based quantum sensing, which is an interdisciplinary field with great potential applications.
Nitrogen–vacancy (NV) center is one of the hundreds color centers in diamond.[1] As shown in Fig.
There are a series of theoretical and experimental works toward the quantum information process with NV centers in diamond. These fundamental topics include understanding the spin dynamics[3–12] and charge dynamics,[13–16] preserving center spin coherence by employing isotopic purified diamond[17,18] or dedicate quantum control techniques such as dynamical decoupling[6,19] and dynamical nuclear spin polarization.[20,21] With nearby nuclear spins, quantum registers[22–24] and multiparticle entanglement[25] have been demonstrated. And high-fidelity projective (single-shot) readout of individual spins,[26–29] universal quantum gates[30–35] have developed, which leads to the recent experimental demonstration of quantum error correction,[36,37] entanglement distillation,[38] and quantum algorithms[31,39–42] in this solid-state system. Meanwhile, using NV electron spin as a quantum probe has been proposed and demonstrated,[43,44] which now is at the core of the fast-developing field of quantum sensing.[45]
There are several nice reviews about the basic of NV center[46–49] and the emergent field of diamond quantum sensing.[45,50–54] In this review, we focus on the working principles and recent experimental progresses of NV-based quantum computing, which includes a brief introduction to the ODMR techniques, followed by the understanding and prolonging of center spin coherence, then the physics and techniques to build universal quantum gates, and principal applications of those few spins. Several protocols of scaling up NV-based qubits are discussed at the end of the review.
We first introduce the basic spin and optical properties of NV centers in diamond. A six-electron model has been generally accepted to explain the energy levels of the negatively charged NV− center.[48] As shown in Fig. 



The polarization and readout of this qubit is realized by exploiting the spin-dependent optical transitions of NV centers. As shown in Fig. 



The spin-dependent optical transitions enable room temperature ODMR of an NV center. This technique can be understood in analogy with the electron paramagnetic resonance (EPR) or nuclear magnetic resonance (NMR), while the optical pulses instead of the huge magnetic fields are employed to polarize (and readout) the target spins. As discussed above, a very high spin polarization (

Figure 
The spin-dependent optical properties of NV center also enable coherent manipulation of single nuclear spins around an NV center. As shown in Figs.
In this section, we discuss the spin coherence of NV centers, which is of crucial importance for all the quantum information processing and quantum sensing applications. The spin state, for example, 
The process of losing phase information is known as decoherence (dephasing), which happens in a time scale of T2. For NV centers in diamond, the decoherence is dominated by the hyperfine coupling with its surrounding spins. In type Ib diamond, the large amount of substitutional nitrogen impurities (P1 centers, more than 100 ppm) contribute an electron spin bath and limit T2 to several microseconds.[7] In high-purity IIa diamond (
There is another characteristic time of decoherence named 






Besides the above mentioned thermal fluctuations, the local field also shows quantum fluctuations, which affects the coherence of the center electron spin. In general, the local field operator b does not commute with the total Hamiltonian of the spin bath HE, thus the eigenstates of the local field will evolve to a superposition states of different eigenstates in a later time, which leads to a random distribution of the following measurement on b and causes quantum fluctuations.[7,9] At room temperature, thermal noise is usually much stronger than the quantum fluctuation, so the depasing process of an NV electron spin is dominated by the thermal fluctuations, which has Gaussian distribution and the envelope of the coherence signal shows Gaussian decay. By tuning the strength of the external magnetic field, the effect of quantum fluctuation can be enhanced and became measurable in experiments.
In Fig. 

 |
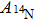
 | Fig. 4. (color online) Controllable effects of quantum fluctuations on the free-induction decay (FID) of an NV center spin: (a) the dephasing time 

|
Quantum coherence is fragile but at the core of quantum information processing. In this section, we will discuss two effective strategies, dynamical nuclear spin polarization (DNP) and dynamical decoupling (DD), to combat with the above mentioned noise effects thus protect the coherence of NV-based spin qubits. Furthermore, we will show that DD can be used to selectively address and manipulate nearby weakly coupled 13C nuclear spins around an NV center, which largely extends the physical resources of scalable quantum computing.
The thermal and quantum fluctuations of the unpolarized spin bath cause the decoherence of NV electron spins.[7–9,60] A straight-forward scheme of center spin coherence protection is to polarize the nuclear spin bath and suppress their inhomogeneous broadening, as shown in Fig.
 | Fig. 5. (color online) Protection of center spin coherence by dynamic nuclear spin polarization (DNP). (a) Illustration of the dynamically polarized nuclear spin bath (purple) around an NV center (red). The spin polarization is first built by the green laser pulse, then it can be transfer to the surrounding 13C nuclear spins under certain conditions. (b) At excited-state level anti-crossing (ESLAC) point, the energy mismatch between the electron spin and nearby nuclear spins is compensated by the external magnetic field (about 500 Gs). (c) The Hartman–Hahn condition. (d) Experimental measured FID signals without (black line) and after (red line) DNP, the prolongation of the dephasing time (from 


|
The three-order gyromagnetic ratios difference between the electron spin and the nearby nuclear spins blocks the direct polarization transfer between them. Fortunately, with the large zero-field splitting (2.87 GHz for the ground states and 1.4 GHz for the excited states), the energy gap of the electron spin states (between the 

Under other magnetic fields, the energy mismatch between the two types of spins can be compensated by driving the electron spin (Rabi frequency, in its rotating frame) to the Larmor frequency of the 13C nuclear spin. This special resonant requirement is known as Hartman–Hahn condition,[62] as shown in Fig.
Dynamical decoupling is a well-developed technique in NMR and ESR,[63,64] and recently it has been demonstrated as an efficient technique to protect the center spin coherence. For NV centers in diamond, there are several nice demonstrations of extending electron spin coherence by DD,[6,19,65] and later on detecting[66–71] and manipulation[29,32,35,37,68,72–74] remote nuclear spins with engineered DD sequence. In this section, we first introduce the working principles of DD-based spin coherence protection, and then discuss the core idea of coherence as a source of quantum sensing. Special attention is paid to the controllable quantum measurement implemented by DD on weakly coupled nuclear spins. A discussion of noise-resilient quantum evolution steered by DD can be found in the section of quantum gate.
In the realistic spin bath of an NV center in high-purity diamond, as show in Fig.
 | Fig. 6. (color online) Protection of center spin coherence by dynamical decoupling (DD). (a) An NV electron spin (red) and its 13C nuclear spin bath (purple). The spin state of a strongly coupled 13C nuclear spin can be mapped to the electron spin through selective MW pulses. For weakly coupled nuclear spins, DD is employed to address one of them while decoupling the others. (b) Spin coherence as function of the total evolution time, while CPMG of different pulse numbers is applied. (c) Coherence of the center electron spin under CPMG-12 (red line) and CPMG-1 (gray line), as a function of the pulse interval τ. Different nuclear spins can be selectively addressed by choosing the value of τ. (d) Under the protection of a CPMG sequence, the NV spin state only accumulates a controllable phase from the selected target nuclear spin. Reproduced from Refs. [19] and [29], APS. |
Center spin decoherence caused by those weakly coupled nuclear spins can be effectively suppressed with DD sequence. In general, a DD sequence contains many fast-flipping pulses acting on the center electron spin. The bath spin evolution is a quasi-static process compared to the fast flippings, thus the unwanted coupling between the center spin and bath spins can be averaged to zero, and the coherence (phase information of the quantum state) of the center spin can persist for a much longer time under DD sequence.[6,19,65] As shown in Fig.
DD can also be used to amplify the interaction from one selected 13C nuclear spin and implement controllable quantum measurement on it. This happens when the precession frequency of this nuclear spin matches the flip rate of the DD sequence, thus the phase from this nuclear spin will be added up and the coherence signal of the center spin shows abrupt dips, as shown by the red line in Fig.
After addressing one of the weakly coupled nuclear spins by tuning the pulse intervals, DD can act as a controllable quantum measurement on it. The accumulated phase of the center electron spin from the target nuclear spin is controlled by the pulse number (total interaction time) of the DD sequence (Fig. 
 | Fig. 7. (color online) Controlling the strength of quantum measurements by DD and single-shot readout of a weakly coupled nuclear spin. (a) The accumulated phase of the center electron spin (and thus the measurement strength of the weakly coupled target nuclear spin) is controlled by the pulse number of the applied CPMG sequence. (b) Pulse sequence. The weakly coupled nuclear spin is selectively addressed and its state is mapped to the center electron spin by the CPMG sequence, and then readout by the subsequent projective measurement on the NV electron spin. (c) Typical quantum jump signal of a weakly coupled nuclear spin under an external magnetic field of 691 Gs. Each data point is the sum of 
|
One application of these controllable quantum measurements is to implement single-shot readout of these weakly coupled 13C nuclear spins, which are good candidates for quantum memories due to their strength in number and good coherence. As discussed in Ref. [29], a set of nuclear spin basis states are stable under repetitively applied DD sequence. As the nuclear spin will be trapped to this state with the repetitively applied DD sequence, and the following projective measurements on the center electron spin give the same results and can be summed up together to extract the state of the target nuclear spin. As shown in Figs.
In quantum computation, a quantum gate is an operation on a few qubits, which manipulates the quantum states to implement elementary tasks. Quantum gates are the building blocks of quantum information processing. In this section, we briefly survey the quantum gates of NV-based spin qubits. The key factors which affect the gate fidelity in experimental implementation, including the pulse imperfections and spin dephasing during manipulation, are discussed.
Based on their protocols, these quantum gates can be categorized into four groups, as listed in Table 

 | Fig. 8. (color online) Quantum gate and its experimental implementation. (a) A single qubit quantum gate (Hadamard gate) can be understood as a rotation on the Bloch sphere. (b) The envelop of a plain pulse and (c) a shaped pulse (REBURP) in the time domain. The carrier frequency is ignored in this schematic plot. Panels (d) and (e) are the same pulses as shown in panels (b) and (c), but in frequency domain. The shaped pulse has almost equal excitation in a sharply edged region, makes it particularly suite for the selective excitations. Reproduced from Ref. [76], AIP. |
| Table 1.
Quantum gates for NV-based spin qubits. . |
In realistic experimental implementation, the shape of an MW pulse is important to combat the noise effects. Figure
To achieve fault-tolerant quantum control, one needs to analysis the qubit system as well as the applied driving pulses.[84,85] As demonstrated in reference,[33] the gate errors may come from the Overhauser field of an NV center, the magnetic field fluctuation, the instability of the microwave frequency, and pulse distortions due to the above mentioned finite bandwidth, or the instability of the microwave source. These imperfections can be eliminated with dedicated control techniques. For example, the phase and amplitude distortions of an MW pulse can be corrected by the pulse-fixing technique. Furthermore, with the knowledge of the noise sources, one can design composite pulse to combat them. In Ref. [33], a composite pulses named BB1inC has achieved single-qubit gate fidelity of 0.999952(6). Meanwhile, a two-qubit gate with fidelity of 0.992 is implemented by an optimized GRAPE pulses, as shown in Fig.
 | Fig. 9. (color online) High fidelity universal quantum gate implemented by optimal composite pulses. (a) and (b) Gate fidelity of an original gradient ascent pulse engineering (GRAPE) (a) and a modified one (b). The upper figures are the contour maps of the gate fidelity. The modified GRAPE is more robust to the noise effects and pulse errors. A CNOT gate with fidelity of 0.992 is achieved with this modified GRAPE. (c) The used pulse parameters of the optimal control experiment. The left (right) one is the amplitude (phase) sequence. Reproduced from Ref. [33], NPG. |
The above discussion focuses on either the task of coherence protection or the implementation of quantum gates. It is nature to ask whether there is a scheme to achieve both goals simultaneously. As DD is an effective technique to protect the center spin coherence, a direct solution to this problem is applying both the DD pulses and gate pulses simultaneous. However, this is a non-trivial mission since the two control pulses may distort the effect of each other. Nevertheless, several groups have demonstrated that it is possible to implement the quantum gates while using a DD sequence to protect the center spin coherence.[31,79] These schemes can be classified into the gate while DD (see Table
We now introduce another scheme, named as gate by DD, which uses only the DD pulses to achieve both the gate operation and the coherence protection. The essential idea of DD-based nuclear spins manipulation is to fully exploit their electron spin state dependent evolution. Taking a nearby 13C nuclear spin for example, as shown in Fig.
As shown in Fig.
 | Fig. 10. (color online) Noise-resilient quantum gates implemented by DD. (a) Propagator evolution in the conventional settings of FID or periodic CPMG sequence in the operator space. (b) In contrast to the conventional schemes, one can relax the timing constraint in applying the DD pulses and thereby steer the system propagator to the desired points in the operator space. For instance, the C-NOT and the nuclear qubit Pauli-X (Xn) gates can be realized by the purple and the yellow paths, respectively. The gate is characterised by performing state tomography of the two-qubit system before and after its implementation. (c) State tomography for the initial state 



|
To demonstrate the DD-steered quantum gate, the coupling strength between a nearby 13C nuclear spin and the center electron spin is measured in experiment, and then it is used in the numerical simulations of optimizing the effect of the DD sequence, with the aims of preserving the electron spin coherence as well as steering the quantum evolution of the target nuclear spin. Figures
There are other schemes to implement quantum gate on these spin qubits (Table
Although the one or two well-controlled spin qubits are far away from a realistic quantum computer, they provide a good platform to exploit and verify fundamental issues in quantum information processing, such as demonstration of quantum cloning machine,[40,41] quantum error correction,[36,37] quantum Zeno effect,[72,86] and quantum algorithm.[31,34,39,42] In this section, as examples, we discuss the demonstration of entanglement enhanced phase estimation[87] and loophole-free Bell inequality violation.[88]
Quantum parameter estimation is the emerging field of yielding higher statistical precision of unknown parameters by harnessing entanglement and other quantum resources. Precise parameter estimation is of crucial importance in science and technology. Repeating measurement is the straight-forwards protocol to decease the statistical error in estimation. However, it is not the most efficient strategy. In the case of using N particles to estimate a parameter φ, if these N particles are independent, the standard quantum limit (SQL) gives the scaling law of the uncertainty on the averaging numbers 

A well controlled two-spin system (an NV electron spin and its nearby 13C nuclear spin) is employed to demonstrate the entanglement-enhanced phase estimation protocol. The phase of the spin qubits is the parameter of interested, which can be prepared and measured with the techniques mentioned in Ref. [87]. The two spin qubits can be prepared and measured independently, as shown in the upper pane of Fig. 

 | Fig. 11. (color online) Entanglement-enhanced phase estimation. (a) Phase estimation schemes of the independent states and the electron-nuclear entangled state. By harnessing entanglement, quantum metrology yields higher statistical precision than classical approaches. (b) Phase relation of an independent states and entangled states. (c) The phase uncertainty is fitted by function 

|
For different repeat number and input phases, the uncertainties of phase estimation with independent and entangled states are compared in Figs. 
Bell’s inequality[93] provides an experimental measurable scheme to verify the difference between quantum mechanics and local hidden variables theories. It is of crucial importance in understanding the fundamental property of nature and it is the guarantee of the device-independent quantum cryptography.[94] In this section, we introduce the recent loophole-free Bell inequality violation experiment with NV centers in diamond.
Bell’s inequality can be verified with entangled particles, as proposed in reference.[95] There are two major loopholes in all the Bell test experiments: the locality loophole[96] and the detection loophole.[97] To exclude the locality loophole, the entangled particles can be separated far away from each other thus the communication between them during the measurement time is impossible (information can not be transferred faster than light). One candidate for such demonstration is entangled photon pairs.[98] However, these flying qubits always suffer from the photon loss during the spatial distribution of entanglement and also the imperfection in detection, which lead to the detection loophole.
In the NV-based Bell inequality experiment,[88] both of the loopholes are excluded. The locality loophole is excluded by establishing remote entanglement of two NV electron spins (1.3 km). As shown in Figs. 

 | Fig. 12. (color online) Elements of the loophole-free Bell inequality violation experiment.[88] (a) The lambda transition makes it is possible to entangle the NV electron spin state with the polarization of an emitted photon. For example, the detection of a 

|
The statistic of 245 trials of CHSH–Bell inequality give 


In summary, we discussed the basic properties and experimental techniques to manipulate the NV spin qubits. These qubits include the electron spin of an NV center and the nearby nuclear spins. The randomly distributed nuclear spins are the sources of the electron spin decoherence, but they also could be a good resources of multi-qubit system.
With dynamical nuclear spin polarization, the coherence of center electron spins can be prolonged, and this technique can be extended to other spins, either inside or outside the diamond lattice, to build a hyperpolarization of surrounding spins, which may benefit the field of NMR and MRI (magnetic resonance imaging). With dynamical decoupling, the nearby nuclear spin can be selectively addressed, manipulated and measured, which offers a great chance in building multi-qubits quantum node (see discussion below), as the elementary part of a quantum repeater and quantum computer. With those well-controlled few spins, we are able to demonstrate and verify fundamental issues in quantum information process, such as entanglement-enhanced phase estimation and loophole-free Bell inequality violation.
At the end of this review, we discuss the strategies of scaling up NV-based qubits for quantum computing and distributed quantum networks.
The straight-forward scheme of scaling up is to couple NV centers with their direct interactions. As demonstrated in reference Ref. [103], when two NV centers are close to each other, for example, with a distance below 10 nm, the coupling between them is strong enough to be observed directly in the CWODMR spectrum. And selective addressing and manipulation are available for those strong coupled NV electron spins. Furthermore, nearby nuclear spins around the NV centers can be harnessed to prolong the lifetime of the spin entanglement.[104] Unfortunately, the deterministic fabrication of directly coupled NV centers, which requires three-dimensional precision better than 10 nm, is still beyond the current techniques.[105–108]
A substantial effort has been geared towards coupling NV electron spin to other solid state quantum systems such as superconducting qubits[109–112] and mechanism resonator.[113–117] In these schemes, the merits of different physical system could be merged together. For example, the convenience in sample fabrication and fast manipulation of superconducting qubits, together with the long coherence time of NV spin ensemble, make such hybrid system as one of the most promising node for scalable quantum computing.[118]
There are also exciting progress in scaling up NV spin qubit with photons. The spin states of NV centers are initialized and readout with optical pulses, and both the excitation and emission photons can be exploited to establish entanglement with the NV electron spins.[24,91] By employing one of the optical lambda transition, Harvard group demonstrated that the polarization of the emission photons preserve good entanglement with the NV spin states.[91] Later, indistinguishable photon emission from two separate NV centers was demonstrated by Delft and Harvard groups, where the optical transition energies difference was compensated by the dc Stark effect.[99,100] Then Delft group demonstrated remote spin entanglement with a joint measurement on the NV emission photons,[119] which enabled the loophole-free Bell inequality verification[88] and entanglement distillation between solid-state quantum network nodes.[38]
To build a realistic quantum networks or scalable quantum computing with NV centers, quantum nodes with better optical coupling efficiency and longer build-in quantum memory are needed,[120–122] which could be achieved by fabricating photonic nanostructures[123–126] and exploiting the long coherence time of nearby nuclear spins.[24,72,74,127–130] Again, the development of NV-based quantum computing involves cross-disciplinary efforts, and will offer unprecedented rewards in a broad range of frontier studies, such as condensed matter physics and nanoscale magnetic resonance imaging.[45,50,52–54]
| [1] | |
| [2] | |
| [3] | |
| [4] | |
| [5] | |
| [6] | |
| [7] | |
| [8] | |
| [9] | |
| [10] | |
| [11] | |
| [12] | |
| [13] | |
| [14] | |
| [15] | |
| [16] | |
| [17] | |
| [18] | |
| [19] | |
| [20] | |
| [21] | |
| [22] | |
| [23] | |
| [24] | |
| [25] | |
| [26] | |
| [27] | |
| [28] | |
| [29] | |
| [30] | |
| [31] | |
| [32] | |
| [33] | |
| [34] | |
| [35] | |
| [36] | |
| [37] | |
| [38] | |
| [39] | |
| [40] | |
| [41] | |
| [42] | |
| [43] | |
| [44] | |
| [45] | |
| [46] | |
| [47] | |
| [48] | |
| [49] | |
| [50] | |
| [51] | |
| [52] | |
| [53] | |
| [54] | |
| [55] | |
| [56] | |
| [57] | |
| [58] | |
| [59] | |
| [60] | |
| [61] | |
| [62] | |
| [63] | |
| [64] | |
| [65] | |
| [66] | |
| [67] | |
| [68] | |
| [69] | |
| [70] | |
| [71] | |
| [72] | |
| [73] | |
| [74] | |
| [75] | |
| [76] | |
| [77] | |
| [78] | |
| [79] | |
| [80] | |
| [81] | |
| [82] | |
| [83] | |
| [84] | |
| [85] | |
| [86] | |
| [87] | |
| [88] | |
| [89] | |
| [90] | |
| [91] | |
| [92] | |
| [93] | |
| [94] | |
| [95] | |
| [96] | |
| [97] | |
| [98] | |
| [99] | |
| [100] | |
| [101] | |
| [102] | |
| [103] | |
| [104] | |
| [105] | |
| [106] | |
| [107] | |
| [108] | |
| [109] | |
| [110] | |
| [111] | |
| [112] | |
| [113] | |
| [114] | |
| [115] | |
| [116] | |
| [117] | |
| [118] | |
| [119] | |
| [120] | |
| [121] | |
| [122] | |
| [123] | |
| [124] | |
| [125] | |
| [126] | |
| [127] | |
| [128] | |
| [129] | |
| [130] |








