† Corresponding author. E-mail:
Project supported by the National Natural Science Foundation of China (Grant Nos. 11634008, 61227902, 11574187, and 11674203), the National Key Research and Development Program of China (Grant No. 2017YFA0304500), and the Fund of “1331 Project” Key Subjects Construction of Shanxi Province, China.
We report the experimental generation of a squeezed vacuum at frequencies ranging from 2.5 kHz to 200 kHz that is resonant on the cesium D2 line by using a below-threshold optical parametric oscillator (OPO). The OPO is based on a periodically-poled KTiOPO4 (PPKTP) crystal that is pumped using a bow-tie four-mirror ring frequency doubler. The phase of the squeezed light is controlled using a quantum noise locking technique. At a pump power of 115 mW, maximum quadrature phase squeezing of 3.5 dB and anti-squeezing of 7.5 dB are detected using a home-made balanced homodyne detector. This squeezed vacuum at an atomic transition in the kilohertz range is an ideal quantum source for quantum metrology of enhancing measurement precision, especially for ultra-sensitive measurement of weak magnetic fields when using a Cs atomic magnetometer in the audio frequency range.
Squeezed light is an important source in many areas of continuous-variable quantum information science, including quantum communication,[1] precision measurement,[2–4] quantum storage and studies of the interactions between light and atoms.[5,6] Parametric down-conversion process in a sub-threshold optical parametric oscillator (OPO) is often used to generate continuous wave (CW) squeezed light. As a result of the development of modern technologies such as high-quality nonlinear crystals, low-loss coatings and high-efficiency detectors, 15 dB of squeezing has been obtained with the ultimate goal of surpassing the standard quantum limit for high-sensitivity measurements at 1064 nm.[7] Unfortunately, the generation process and the measurement frequency range mainly focus on the radio-frequency range. Laser relaxation oscillation and other technical noise sources have typically limited squeezing to the megahertz range,[8] and thus CW squeezed sources at audio frequencies have rarely been reported to date. However, the development of various applications often requires signal measurements to be performed at low frequencies, which would require squeezed light to be produced in the low-frequency range. For example, stably controlled squeezed states with bandwidths ranging from kilohertz down to a few hertz or even lower will be required for gravitational wave detectors.[9] In the quantum communication field, squeezed light can be stored and released by the electromagnetically-induced transparency (EIT) of the atomic medium.[10] However, because of the narrow transparency windows that are available in EIT, squeezed light at low frequencies is again required.[11] In addition, high-sensitivity magnetic field measurements are an important requirement in the measurements of geomagnetic anomalies, spatial magnetic fields and biological magnetic fields, in which the sideband frequencies range from kilohertz to hertz levels or lower.[12–14] Therefore, shifting the spectrum of the squeezed vacuum states downwards into the acoustic band is highly important for use in quantum metrology and quantum optics experiments. In 2004, McKenzie et al. first realized a broadband continuous squeezed field in a frequency range of 280 Hz–100 kHz, and the phase of the squeezed vacuum was controlled using a quantum noise locking technique.[15] In 2007, Vahlbruch et al. observed squeezed states in a Fourier frequency band down to 1 Hz by using a frequency-shifted coherent light control method to lock the squeezed vacuum phase.[16] In 2012, Stefszky et al. successfully obtained more than 10 dB of squeezing at an analysis frequency of 10 Hz as a result of a series of technical improvements.[17] Unfortunately, these results were observed at 1064 nm and 1560 nm, which are in the wavelength ranges of gravitational wave detection and fiber telecommunication and far from those of the alkali metal atomic transitions. Nevertheless, squeezed light corresponding to the transitions of the alkali metal atoms, such as cesium (Cs) and rubidium (Rb), have great potential applications in fields including nonclassical spectroscopy, light–atom interactions, information storage and readout, quantum information networks, and ultra-precision measurements. In 2007, Takeno et al. observed 9 dB of vacuum squeezing at 860 nm, which was close to the Cs D2 line, at a measurement frequency of 1 MHz.[18] A major step forward was made by Burks et al., who focused on squeezed light generation at an atomic transition. They obtained 3 dB of squeezing at a frequency of 50 kHz at 852 nm.[19] In 2010, Wolfgramm et al. applied polarization-squeezed probe light to a hot unpolarized ensemble of Rb atoms, and improved the sensitivity of their magnetometer by 3.2 dB at a measurement frequency of 120 kHz.[20] In contrast, squeezed light on atomic lines is urgently needed of further developing the lower frequency direction. In this paper, we demonstrate a system that produces vacuum squeezed light on the Cs D2 line, in which a frequency doubler is used as a pump source of an OPO based on KTiOPO4 (PPKTP). Using a subthreshold parametric down-conversion process, the squeezed vacuum is obtained experimentally at frequencies ranging from 2.5 kHz to 200 kHz. The phase of the squeezed vacuum on the homodyne detector is controlled using a quantum noise locking technique that operates without a carrier.[21] The maximum squeezing level is 3.5 dB below the standard quantum level (SQL), while the corresponding anti-squeezing is 7.5 dB above the SQL.
A schematic diagram of the experimental setup is shown in the following Fig.
The system is mainly composed of (i) a second harmonic generator (SHG), (ii) an OPO, and (iii) a homodyne detection (HD). A CW Ti:sapphire laser is locked onto the D2 transition of the Cs atoms. The 852-nm laser beam is then divided into four parts. Approximately 400 mW of the fundamental wave light is used for SHG to produce 180 mW of the pump field at 426 nm that allows the OPO to generate a squeezed vacuum.[22] A small fraction of the beam is used as a local beam (LO) for the HD. The remaining two parts of the beam are used as a cavity length locking beam and the probe beam for the OPO cavity, respectively. To avoid the interference between the locking beam and the probe beam (see Fig.
In Fig.
Firstly, we study the classical performance of the OPO as a phase-sensitive amplifier. For that purpose, a weak probe beam is injected into the OPO through high-reflectivity mirror M2, and by slowly scanning the injection phase, the amplification G is measured through output coupler mirror M1. The measured parametric gain G versus pump power characteristic is shown in Fig.
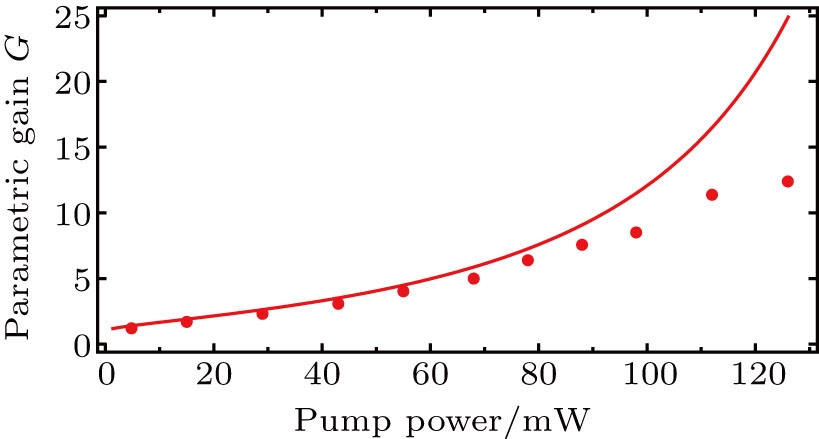 | Fig. 2. (color online) Parametric gain versus pump power, where solid line represents the theoretical simulation results and solid circles denote experimental results. |
In this experiment, the output coupler transmission is T = 10%. The single pass conversion coefficient is measured to be ENL = 2% W−1, and the intracavity loss is L = 2.56%. Using these experimental parameters, the threshold P2,t is inferred to be approximately 197 mW.
In the below-threshold OPO, the anticipated noise variances of the squeezed (Sq) and anti-squeezed (Asq) quadratures are given as follows:[25]
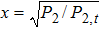
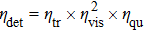
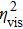
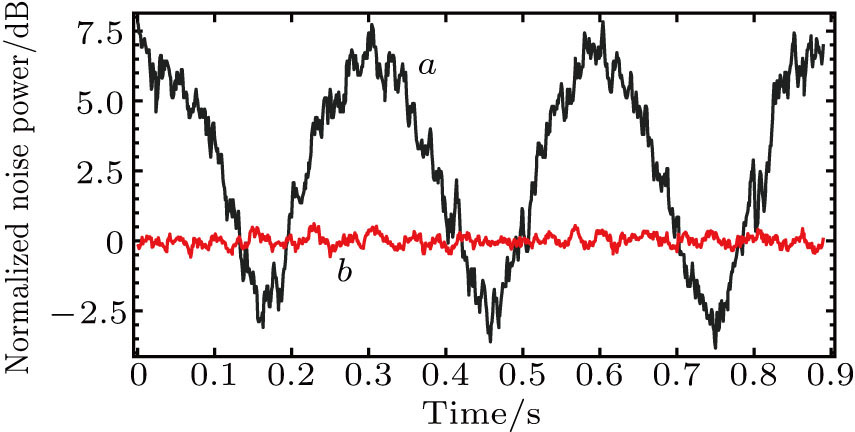
The normalized noise power spectrum in a range from 1 kHz–200 kHz is shown in Fig.
We perform quantum noise measurements of the SQL and the squeezed and antisqueezed noise powers while scanning the LO phase as a function of time at a frequency of 35 kHz with RBW = 1 kHz and VBW = 300 Hz. The results are shown in Fig.
| Table 1. Factors limiting squeezing from the OPO and the detection system. . |
In accordance with Eq. (
In this work, we demonstrate the squeezed light generation on the cesium D2 line by degenerate parametric down-conversion in a symmetrical bow-tie ring subthreshold OPO cavity. Adopting a quantum noise locking technique without a carrier, squeezing of 3.5 dB is observed directly and this squeezing is preserved for frequencies down to 2.5 kHz. Squeezing at such low frequencies is measured using a home-made balanced homodyne detection system. At present, the squeezing is limited by the intracavity losses of the OPO and the detection efficiency. The generation of this squeezed light at such a low frequency on the atomic transition is an important step towards applications of squeezed light such as ultra-sensitive measurements beyond the SQL, precision control of optical systems, quantum memories and further investigation of nonclassical light-matter interactions.
| [1] | |
| [2] | |
| [3] | |
| [4] | |
| [5] | |
| [6] | |
| [7] | |
| [8] | |
| [9] | |
| [10] | |
| [11] | |
| [12] | |
| [13] | |
| [14] | |
| [15] | |
| [16] | |
| [17] | |
| [18] | |
| [19] | |
| [20] | |
| [21] | |
| [22] | |
| [23] | |
| [24] | |
| [25] | |
| [26] | |
| [27] | |
| [28] |