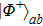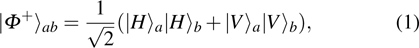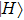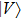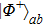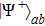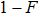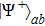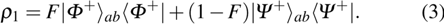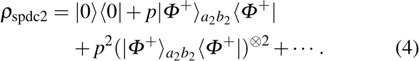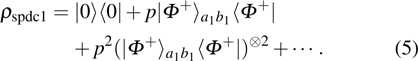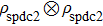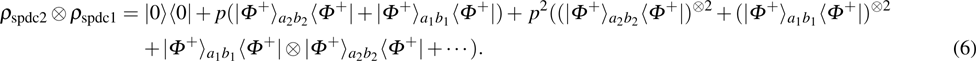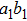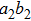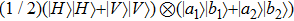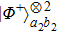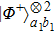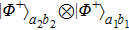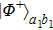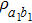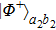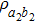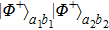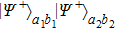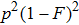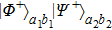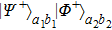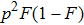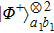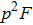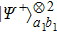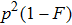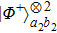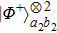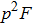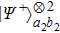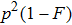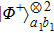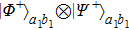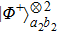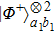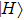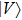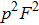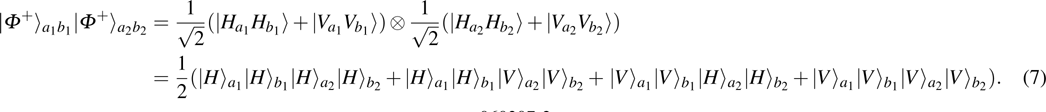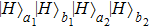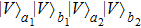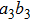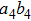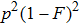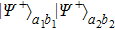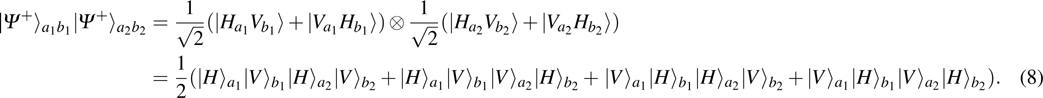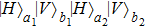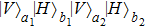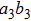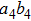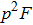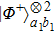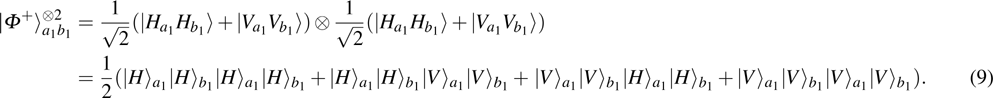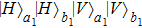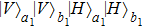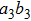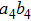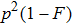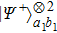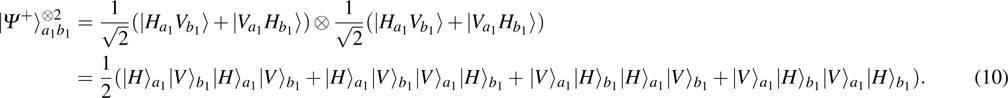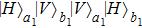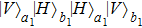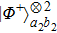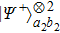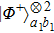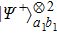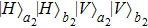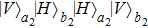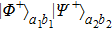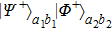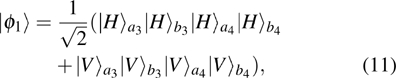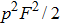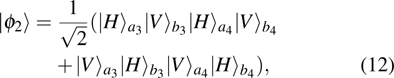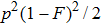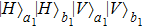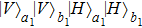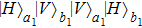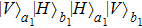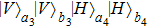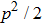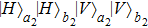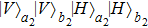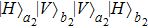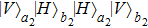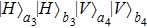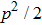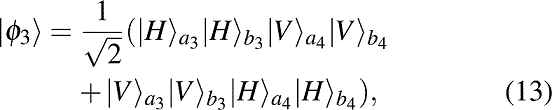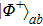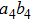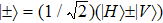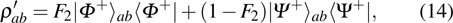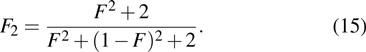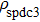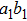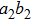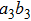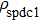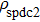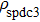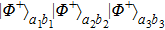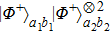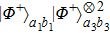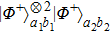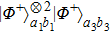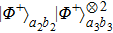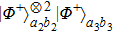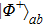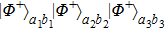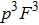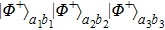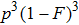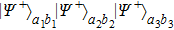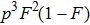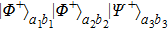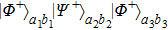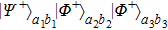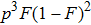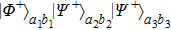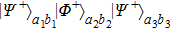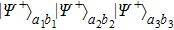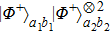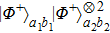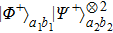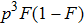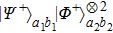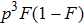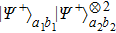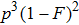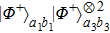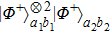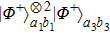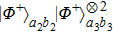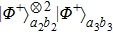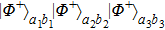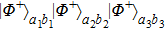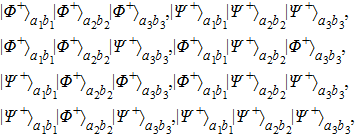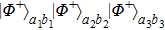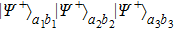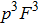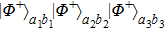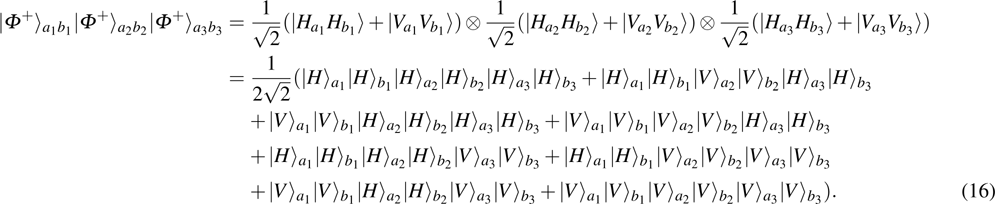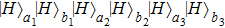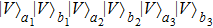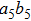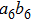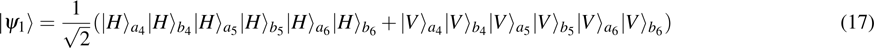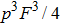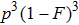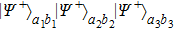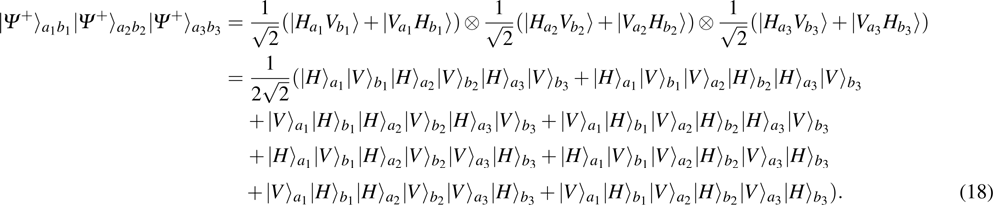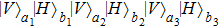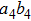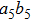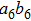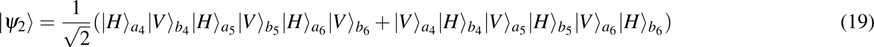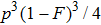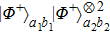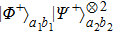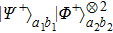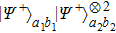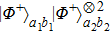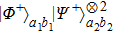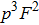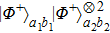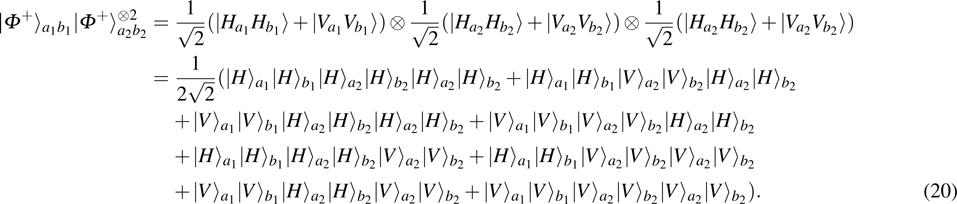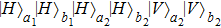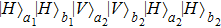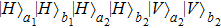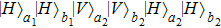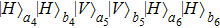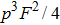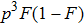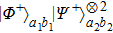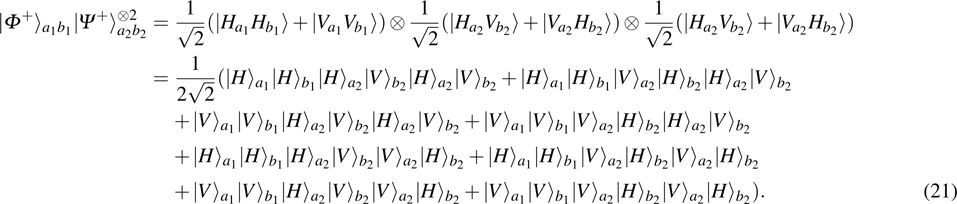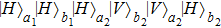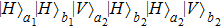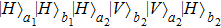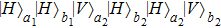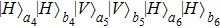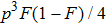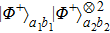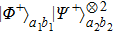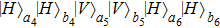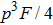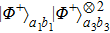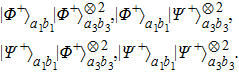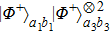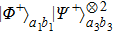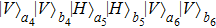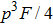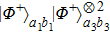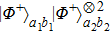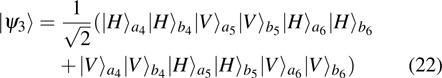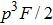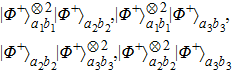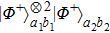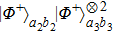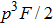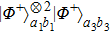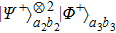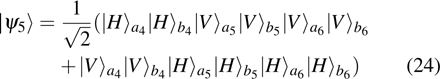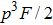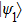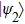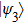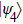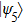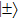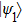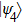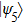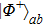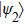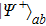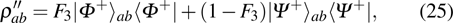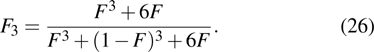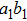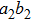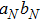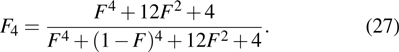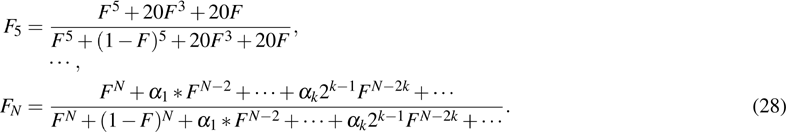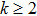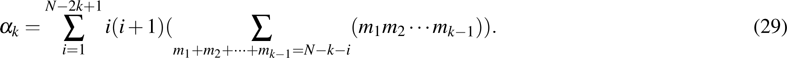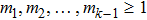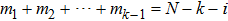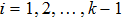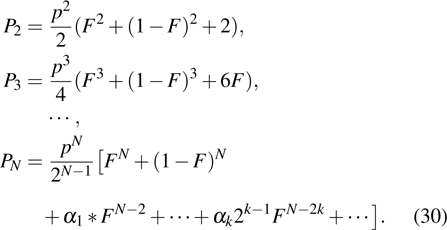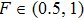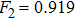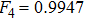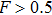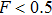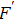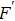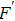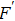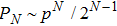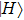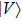Multi-copy entanglement purification with practical spontaneous parametric down conversion sources

Cite this Article

Zhang Shuai-Shuai, Shu Qi, Zhou Lan, Sheng Yu-Bo. Multi-copy entanglement purification with practical spontaneous parametric down conversion sources. Chinese Physics B, 2017, 26(6): 060307 Copy to clipboard

Permissions

Multi-copy entanglement purification with practical spontaneous parametric down conversion sources

Zhang Shuai-Shuai^{1, 3}, Shu Qi^{1, 3}, Zhou Lan², Sheng Yu-Bo^{1, †}

1 Key Laboratory of Broadband Wireless Communication and Sensor Network Technology of Ministry of Education, Nanjing University of Posts and Telecommunications, Nanjing 210003, China

2 College of Mathematics & Physics, Nanjing University of Posts and Telecommunications, Nanjing 210003, China

3 Bell Honors School, Nanjing University of Posts and Telecommunications, Nanjing 210003, China

† Corresponding author. E-mail: shengyb@njupt.edu.cn

Abstract

Entanglement purification is to distill the high quality entanglement from the low quality entanglement with local operations and classical communications. It is one of the key technologies in long-distance quantum communication. We discuss an entanglement purification protocol (EPP) with spontaneous parametric down conversion (SPDC) sources, in contrast to previous EPP with multi-copy mixed states, which requires ideal entanglement sources. We show that the SPDC source is not an obstacle for purification, but can benefit the fidelity of the purified mixed state. This EPP works for linear optics and is feasible in current experiment technology.

PACS: 03.67.Hk;03.65.Ud;03.67.Lx

Keyword:quantum communication;entanglement;entanglement purification

Show Figures

1. Introduction

The absolute security of quantum communication makes it have great value in future communication technologies. Many important protocols have been proposed, such as quantum teleportation,^[1] quantum secure direct communication (QSDC),^[2–5] quantum secret sharing (QSS),^[6] quantum key distribution (QKD),^[7–9] and some other interesting and important quantum communication and quantum information processing protocols.^[10–18] In order to realize the long-distance quantum communication, high quality entanglement is the indispensable resource. However, entanglement can only be prepared locally and then be distributed in the free space or in a fiber. In a practical transmission, the distributed photons will inevitably suffer from the noise in environment. If the entangled quantum system degrades seriously, the quantum communication tasks cannot be well achieved. Moreover, the quantum communication will become insecure.

Entanglement purification is an important quantum technique to obtain the high quality entanglement from the low quality entanglement.^[19] In 1996, Bennett et al. firstly proposed the concept of entanglement purification which attracted much attention.^[19] Generally, existing entanglement purification protocols (EPPs) can be divided into three groups. The first group is to use one copy of degraded mixed state.^[20–27] They are the deterministic EPPs which exploit the hyperentanglement or robust entanglement encoded in other degree of freedom. The second group which contains the most EPPs is to use two copies of degraded mixed states.^[19,28–44] The third group is to use the multi-copy degraded mixed states.^[45–50] In the third group, they usually exploit the controlled-not (CNOT) gates and controlled-controlled-not (CCNOT) gates to complete the purification. However, CNOT and CCNOT are not feasible in current experimental condition, which makes this kind of protocols be far away from current application. In 2015, Cai et al. described a fast multi-copy EPP.^[50] Their protocol can obtain a high fidelity in one purification step and it does not require the sophisticated CNOT gate or CCNOT gate. The protocol only exploits the feasible polarization beam splitters (PBSs) and single-photon detectors. Similar to Ref. [29], the protocol in Ref. [50] still needs the ideal entanglement source. It is known that the ideal entanglement source is not available. Such obstacle will make this protocol still be far away from practical application.

In this paper, we will investigate the multi-copy EPP with practical spontaneous parametric down conversion (SPDC) sources. Interestingly, the SPDC source is not an obstacle in realization purification, but will benefit the protocol. The SPDC source makes us obtain a higher fidelity than the original protocol.^[50] This EPP also requires the linear optics and is feasible in current experiment condition.

This paper is organized as follows. In Section 2, we will describe the basic model of this protocol and discuss the two-copy EPP with SPDC sources. In Section 3, we first discuss the three-copy EPP with SPDC sources and then extend this EPP to a more general case. In Section 4, we will provide a discussion and conclusion.

2. Two-copy EPP with SPDC sources

The basic model of the protocol is shown in Fig. 1, which is similar to Ref. [29]. Bob and Alice want to share the maximally entangled state 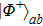of the form

where

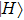
denotes the horizontally polarized photon and

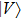
denotes the vertically polarized photon, respectively. The subscripts “a” and “b” are the spatial modes belonging to Alice and Bob, respectively. Because of the noise, the state

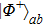
will become

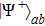
with the probability of

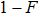
, which is called a bit-flip error. Here

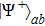
can be written as

Then Alice and Bob will obtain a mixed state of the form

In order to realize the EPP, some EPPs should exploit the ideal entanglement source.^[28,29,50] Unfortunately, such ideal entanglement source is unavailable. Here we use the practical SPDC source to perform the EPP. As shown in Fig. 1, the pump passes through the first beta barium borate (BBO) crystal, named BBO₂, and produces correlated pair of photons in spatial modes

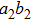
of the form^[20,32,51]

Then the pump pass through the second BBO (BBO₁) and produces a second correlated pair of photons in spatial modes

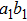
of the form

Therefore, state

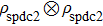
can be written as

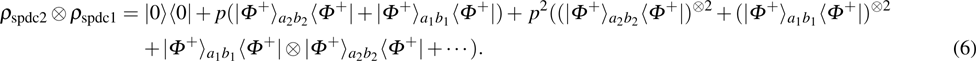	Figure Option View Download New Window
	Fig. 1. (color online) The entanglement purification protocol (EPP) with two copies of mixed states using two SPDC sources. PBSs are polarization beam splitters. PBSs can fully transmit the polarized photon and reflect the polarized photon, respectively.

From Eq. (6), the produced states in spatial modes 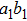and 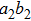can be described as follows. With the probability of p, it is in the state 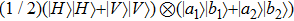. It is essentially the hyperentanglement in both polarization and spatial mode degrees of freedom.^{[20,22,23,52]} With the probability of p², they are in the states 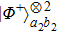, 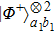or 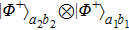. If we consider the errors, the state 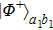will become 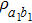and 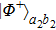will become 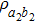. In this way, the original states will become four possible cases. 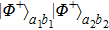States are with a probability of . States are 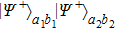with a probability of 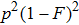. States are 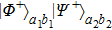and 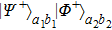with an equal probability of 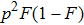. Then for , it becomes states 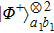with a probability of 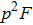and states 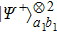with a probability of 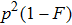. Similarly, for 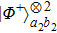, it becomes states 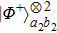with a probability of 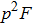and states 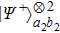with a probability of 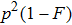. Here we should point out that 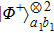cannot become 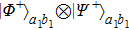, for the two pairs are emitted from the BBO simultaneously and they are always in the same position. The two pairs of photons always suffer from the same error. Therefore, they are always the same states. 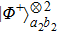is analogy with .

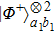As shown in Fig. 1, PBSs can fully transmit the 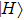polarization photon and reflect the 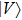polarization photon, respectively. The key step of purification is to select the case that there is only one photon in each output mode which we call it four-mode case.

For example, with the probability of 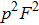, states can be written as

After passing through PBS₁ and PBS₂, only items

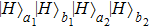
and

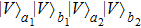
can make each of the output modes

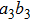
and

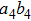
contain one photon. Similarly, with the probability of

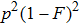
, states

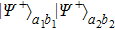
can be written as

After passing through PBS₁ and PBS₂, only items

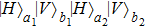
and

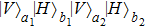
can make each of the output modes

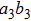
and

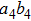
contain one photon. With the probability of

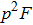
, states

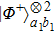
can be written as

After passing through PBS₁ and PBS₂, only items

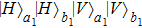
and

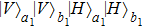
can make each of the output modes

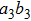
and

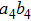
contain one photon. With the probability of

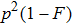
, states

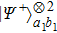
can be written as

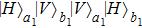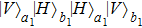After passing through PBS₁ and PBS₂, only items and can make each of the output modes 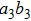and contain one photon. States 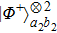and 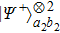can also have the probability to cause the four-mode cases, similar to states 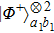and 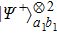, respectively, i.e., items 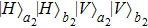, , and items , 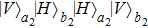can satisfy four-mode case.

On the other hand, states 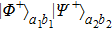and 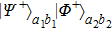cannot satisfy the four-mode case. By selecting the four-mode case, one can obtain

with the probability of

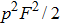
, and

with the probability of

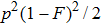
. For the rest of the states, which satisfy the four-mode case, the following states

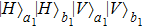
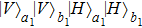
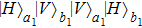
, and

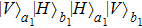
all become

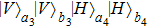
and the total probability is

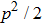
. Similarly

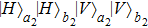
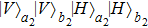
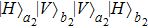
, and

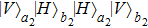
all become

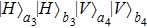
, and the probability is

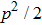
. Therefore, one can also obtain the state

with the probability of p². In order to get

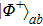
, one should measure the states above in spatial modes

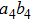
in the basis

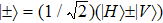
. Similar to Refs. [29],[30], and [49], one can ultimately obtain a new mixed state

and the fidelity is

Here the subscription “2” means that the EPP with two copies of the mixed states.

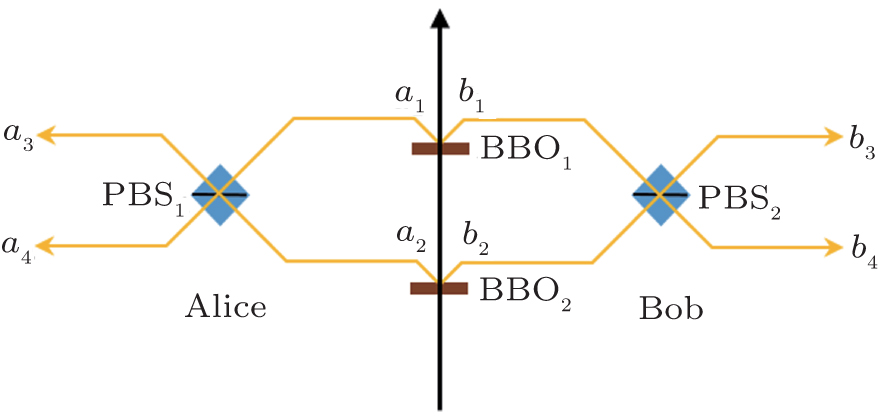
3. Multi-copy EPP with SPDC sources

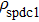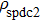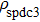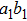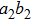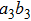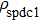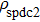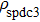In this section, we will discuss the EPP with multi-copy mixed states. As shown in Fig. 2, the pump passes through three BBOs, and produces the entangled photon pairs , , and in spatial modes , , and , respectively. Here , , and have the same form as shown in Eq. (4) and Eq. (5). In order to realize the EPP, we should select the six-mode case. That is each of the output modes a₄, b₄, a₅, b₅, a₆, and b₆ exactly registers one photon. 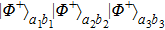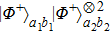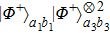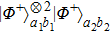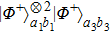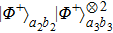Therefore, for the whole system , only items , , , , , , and 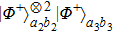can satisfy the six-mode cases.

	Figure Option View Download New Window
	Fig. 2. (color online) The EPP with three copies of mixed states using three SPDC sources.

If the state 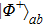suffers from the noise, it will become a mixed state ρ₁ as shown in Eq. (3). In this way, the original state 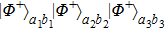will become a mixture of the following states as given below.

(i) With the probability of 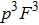, they are in the states .

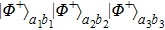(ii) With the probability of 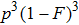, they are in the states .

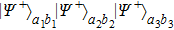(iii) With the equal probability of 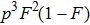, they are in the states 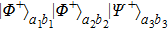, , 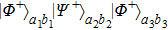and .

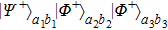(iv) With the equal probability of 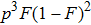, they are in the states 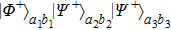, , 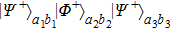and 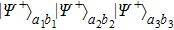, respectively.

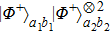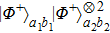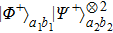For the states , it becomes states with the probability of , states with the probability of 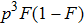, states 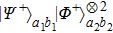with the probability of 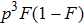, and 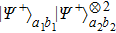with the probability of 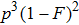, respectively. The other states 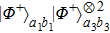, , 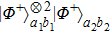, , 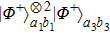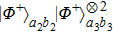and 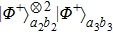can also be described in the same principle.

Similar to Section 2, the key step of this EPP is to select the case that there is only one photon in each output mode and we call it six-mode case. Firstly, we discuss the state .

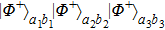Because of noise as described in the above discussion, the state 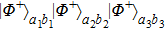will become eight possible different states as

respectively. Interestingly, as shown in Fig. 2, after passing through the four PBSs, only states

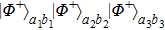
and

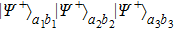
have the possibility to satisfy the six-mode case. For example, with the probability of

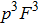
, states

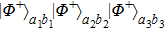
can be written as

After passing through PBS₁, PBS₂, PBS₃, and PBS₄, items

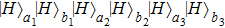
and

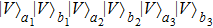
can make each of the output modes

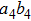
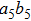
, and

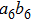
contain one photon. By selecting the six-mode case, they can obtain

with the probability of

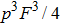
. On the other hand, with the probability of

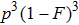
, states

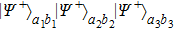
can be written as

After passing through PBS₁, PBS₂, PBS₃, and PBS₄, items

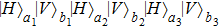
and

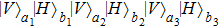
can make each of the output modes

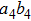
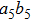
, and

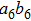
contain one photon. By selecting the six-mode case, they can obtain

with the probability

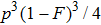
Next, we discuss states 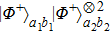. If errors occurs, states 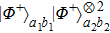will become states 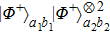, , 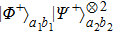, 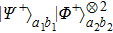and 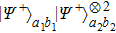which we have described before. Interestingly, only two states 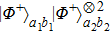and 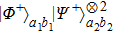have the possibility to satisfy the six-mode case. For example, with the probability of 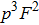, states 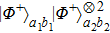can be written as

After passing through PBS₁, PBS₂, PBS₃, and PBS₄, only

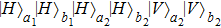
and

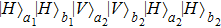
can satisfy six-mode case. By selecting six-mode case,

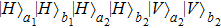
and

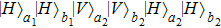
will both become the state

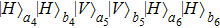
with the probability of

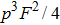
. With the probability of

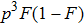
, states

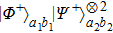
can be written as

After passing through PBS₁, PBS₂, PBS₃, and PBS₄, only items

and

can satisfy the six-mode case. Interestingly, by selecting the six-mode case,

and

both become

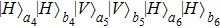
with the probability

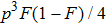
Therefore, from the states 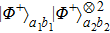and 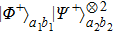, they can obtain the states

with the total probability

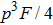
Similarly, the states 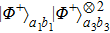can be described in the same way. Because of errors, it will become four different states as

Only the states

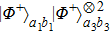
and

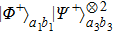
have the possibility to satisfy six-mode case. By selecting six-mode case, they can totally obtain

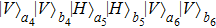
with the probability of

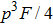
Moreover, if considering the states 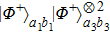and 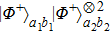together, they can obtain the state

with the probability of

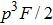
The other states

can be discussed with the same principle. If considering the states

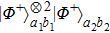
and

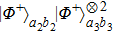
together, they can obtain the state

with the probability of

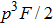
. If considering the states

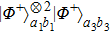
and

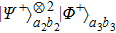
together, they can obtain the state

with the probability of

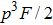
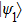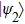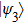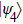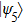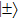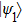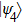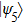From the states , , , , and , after measuring the photons in spatial modes a₅, b₅, a₆, and b₆ in the basis , states , , , and will become 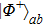and 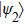will become .

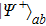Finally, they can ultimately obtain a new mixed state

and the fidelity is

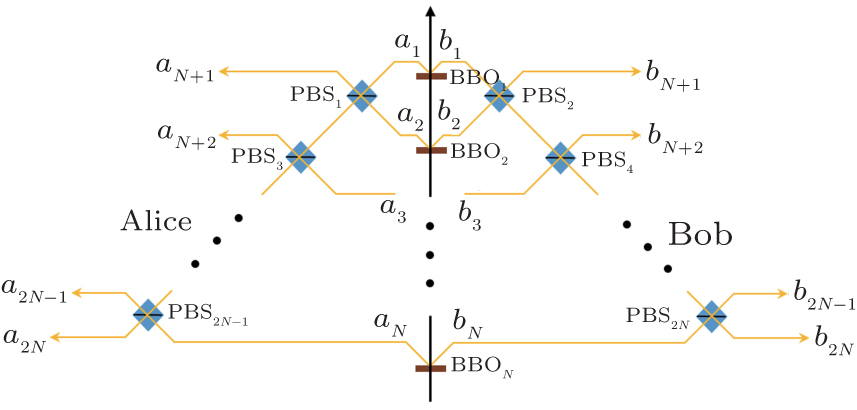
It is straightforward to extend this protocol to the EPP with N pairs of mixed states.

As shown in Fig. 3, the pump passes through N BBOs and creates the correlated photon pairs in spatial modes 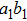, , 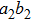…, , 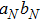respectively. Similar to the EPP with two pairs of mixed states and three pairs of mixed states described above, for the EPP with four pairs of mixed states, they select the eight-mode cases and obtain the new fidelity as

Generally, we can also obtain

Here

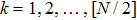
. If N is odd, [N/2] equals

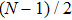
. Otherwise, it equals N/2. Here

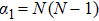
and α_k (

) is

	Figure Option View Download New Window
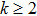
	Fig. 3. (color online) The EPP with N copies of mixed states using N SPDC sources.

Here 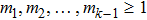and they are all different positive integers which satisfy the condition 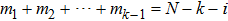. Here we should iterate over all possible m_i ( 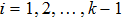) and sum over all possible cases.

We can also calculate the total success probability as

Here P₂, P₃, P_N are the success probabilities with two-copy, three-copy, and N-copy purification.

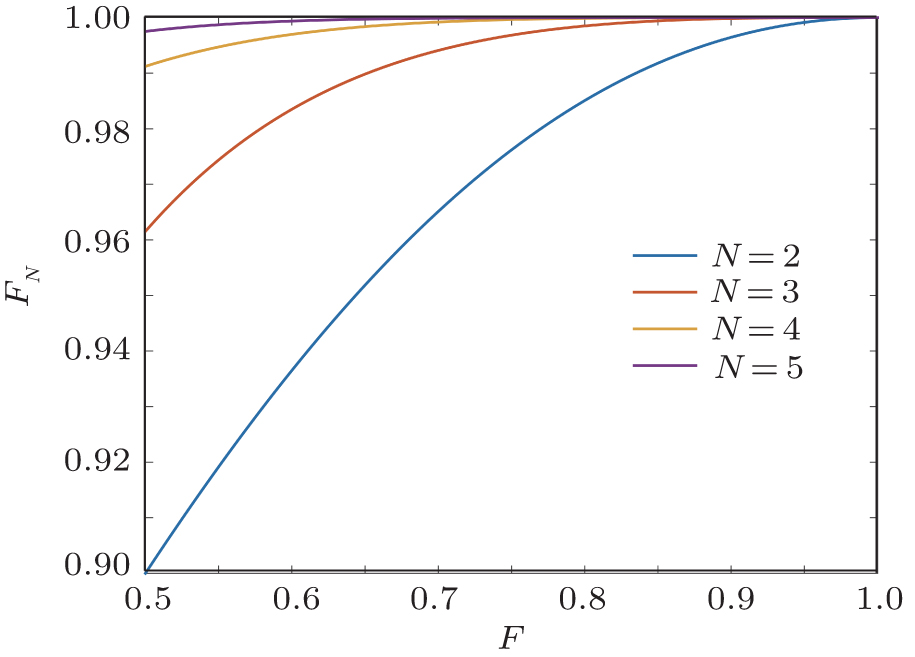
4. Discussion and conclusion

So far, we have completely discussed this EPP. We mainly discussed the EPP for bit-flip error. Certainly, similar to Refs. [29] and [50], the phase-flip error can also be purified with the same principle, by adding the Hadamard operations to convert the phase-flip error to bit-flip error. In linear optics, the Hadamard operation can be implemented by quarter wave plate (QWP). It is interesting to calculate the fidelity of this EPP. As shown in Fig. 4, we calculated the new fidelity F_N altered with the initial fidelity F. Here we let 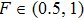and N = 2, 3, 4 and 5 respectively. For example, if F = 0.55, we can obtain that 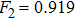and 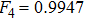. In conventional EPPs,^[29,50] we require the fidelity of initial mixed state to 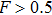. In this EPP, we show that if 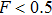, we can also obtain a higher fidelity of mixed state.

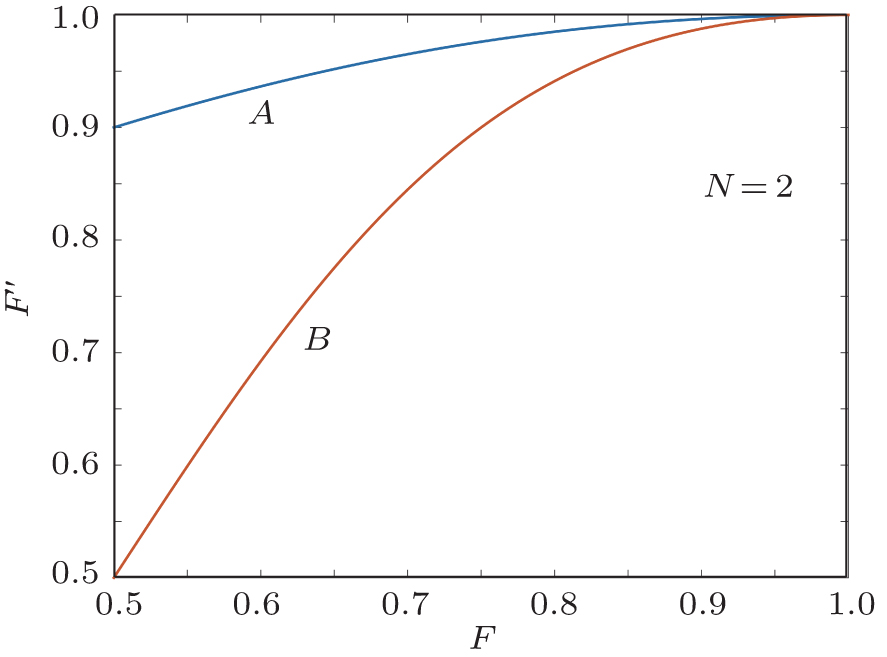
	Figure Option View Download New Window
	Fig. 4. (color online) The increased fidelity F_N altered with the initial fidelity F. N is the number of the degraded photon pairs.

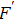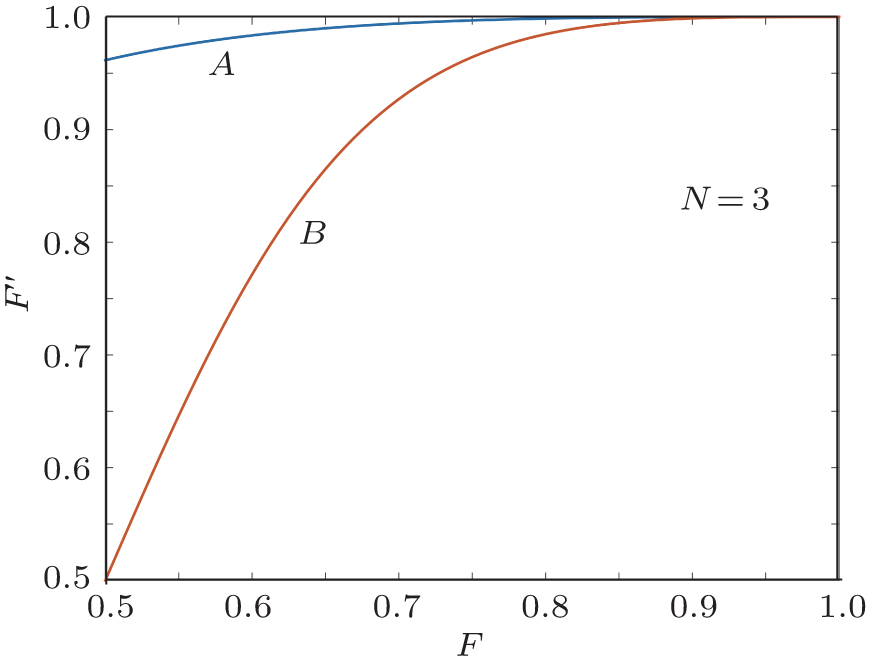
In Figs. 5 and 6, we calculated the purified fidelity in EPPs with two copies and three copies in Refs. [29] and [50], respectively. In Fig. 5, curve A represents the purified fidelity altered with the initial fidelity F in this EPP with two copies. Curve B represents the purified fidelity 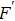altered with the initial fidelity F in Ref. [29]. In Fig. 6, curve A represents the purified fidelity 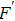altered with the initial fidelity F in this EPP with two copies. Curve B represents the purified fidelity 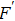altered with the initial fidelity F in Ref. [50]. From both Fig. 5 and Fig. 6, we show that the SPDC source is not the obstacle but the advantage. Actually, in Ref. [30], they have realized the EPP with SPDC sources. In their experiment, if the initial fidelity is 3/4, they can obtain a higher fidelity 13/14, which is also larger than the theory value 9/10 with ideal entanglement sources.

	Figure Option View Download New Window
	Fig. 5. (color online) The increased fidelity altered with the initial fidelity F. The curve A is this two-copy EPP with SPDC sources. Curve B is the EPP in Ref. [29].

	Figure Option View Download New Window
	Fig. 6. (color online) The increased fidelity altered with the initial fidelity F. The curve A is this three-copy EPP with SPDC sources. Curve B is the EPP in Ref. [50].

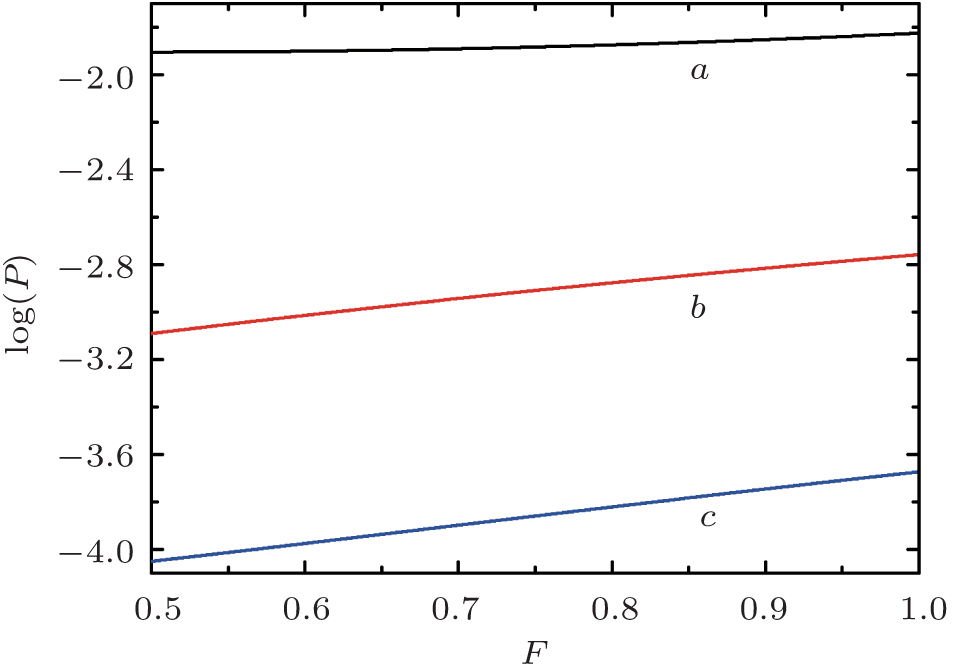
In Fig. 7, we calculated the total success probability altered with the initial fidelity F. We let p = 0.1. Curve a is the case of two-copy purification. Curve b is the case of three-copy purification, and curve c is the case of four-copy purification, respectively. We show that the success probability 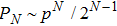and it decreases with N. In EPP with linear optics, they should exploit the post-selection principle. After the success purification, the purified mixed state should also be detected and they cannot improve the fidelity by repeating the EPP. With multi-copy purification, though the total success probability is low, we can obtain a higher fidelity for perform the EPP for one step. Therefore, it is a trade off between success probability and fidelity. In order to realize the multi-copy EPP, we should let the pump pass through N BBOs to generate N pairs of mixed states simultaneously. Such cascaded entanglement sources have been widely realized in current experiment, such as multi-photon entanglement generation,^[53] quantum teleportation of multiple degrees of freedom of a single photon,^[54] experimental Greenberger–Horne–Zeilinger-type six-photon quantum nonlocality,^[55] and so on.

	Figure Option View Download New Window
	Fig. 7. (color online) The success probability P altered with the initial fidelity F. The curve a is the success probability for two-copy EPP. Curve b is success probability for three-copy EPP, and curve c is success probability for four-copy EPP, respectively. Here p = 0.1.

In conclusion, we have discussed the multi-copy EPP with practical SPDC sources. This EPP requires linear optics to complete the task and it is feasible in current experiment condition. Moreover, compared with the EPP with ideal entanglement sources, we show that the SPDC source is not an obstacle but an advantage and can benefit the purification. As the ideal entanglement sources are not available, this EPP may be useful in long-distance quantum communication.

Reference

[1]	Bennett C H Brassard G Crepeau C Jozsa R Peres A Wootters W K 1993 Phys. Rev. Lett. 70 1895
[2]	Long G L Liu X S 2002 Phys. Rev. 65 032302
[3]	Deng F G Long G L Liu X S 2003 Phys. Rev. 68 042317
[4]	Wang C Deng F G Li Y S Liu X S Long G L 2005 Phys. Rev. 71 042305
[5]	Hu J Y Yu B Jing M Y Xiao L T Jia S T Qin G Q Long G L 2016 Light Sci. Appl. 5 e16144
[6]	Hillery M Bužek V Berthiaume A 1999 Phys. Rev. 59 1829
[7]	Ekert A K 1991 Phys. Rev. Lett. 67 661
[8]	Wang Q Zhou X Y Guo G C 2016 Sci. Rep. 6 35394
[9]	Wang Q Zhang C H Wang X B 2016 Phys. Rev. 93 032312
[10]	Xu J S Li C F 2015 Sci. Bull. 60 141
[11]	Zhang C Li C F Guo G C 2015 Sci. Bull. 60 249
[12]	Ma H X Bao W S Li H W Chou C 2016 Chin. Phys. 25 080309
[13]	Tan Y G Liu Q 2016 Chin. Phys. Lett. 33 090303
[14]	Chang Y Zhang S B Yan L L Han G H 2016 Chin. Phys. 24 050307
[15]	Wang M Y Yan F L Gao T 2016 Sci. Rep. 6 29853
[16]	Ding D He Y Q Yan F L Gao T 2015 Acta Phys. Sin. 64 160301
[17]	Ye T Y 2015 Sci. China-Phys. Mech. Astron. 58 040301
[18]	Lu X M Zhang L J Wang Y G Chen W Huang D J Li D Wang S He D Y Yin Z Q Zhou Y Hui C Han Z F 2016 Sci. China-Phys. Mech. Astron. 58 120301
[19]	Bennett C H Brassard G Popescu S Schumacher B Smolin J A Wootters W K 1996 Phys. Rev. Lett. 76 722
[20]	Simon C Pan J W 2002 Phys. Rev. Lett. 89 257901
[21]	Sheng Y B Deng F G 2010 Phys. Rev. 81 032307
[22]	Sheng Y B Deng F G 2010 Phys. Rev. 82 044305
[23]	Li X H 2010 Phys. Rev. 82 044304
[24]	Deng F G 2011 Phys. Rev. 83 062316
[25]	Deng F G 2011 Phys. Rev. 84 052312
[26]	Sheng Y B Zhou L 2014 Laser Phys. Lett. 11 085203
[27]	Sheng Y B Zhou L 2015 Sci. Rep. 5 7815
[28]	Deutsch D Ekert A Jozsa R Macchiavello C Popescu S Sanpera A 1996 Phys. Rev. Lett. 77 2818
[29]	Pan J W Simon C Zeilinger A 2001 Nature 410 1067
[30]	Pan J W Gasparonl S Ursin R Weihs G Zeilinger A 2003 Nature 423 417
[31]	Sangouard N Simon C Coudreau T Gisin N 2008 Phys. Rev. 78 050301
[32]	Sheng Y B Deng F G Zhou H Y 2008 Phys. Rev. 77 042308
[33]	Wang C Zhang Y Jin G S 2011 Phys. Rev. 84 032307
[34]	Xiao L Wang C Zhang W Huang Y D Peng J D Long G L 2008 Phys. Rev. 77 042315
[35]	Wang C Zhang Y Jin G S 2011 Quantum Inform. Comput. 11 988
[36]	Gonta D van Loock P 2011 Phys. Rev. 84 042303
[37]	Sheng Y B Zhou L Long G L 2013 Phys. Rev. 88 022302
[38]	Zwerger M Briegel H J Dür W 2013 Phys. Rev. Lett. 110 260503
[39]	Zwerger M Briegel H J Dür W 2014 Phys. Rev. 90 012314
[40]	Ren B C Du F F Deng F G 2014 Phys. Rev. 90 052309
[41]	Wang G Y Liu Q Deng F G 2016 Phys. Rev. 94 032319
[42]	He Y Q Ding D Yan F L Gao T 2015 J. Phys. B: At. Mol. Opt. Phys. 48 055501
[43]	Zhou L Sheng Y B 2016 Sci. Rep. 6 28813
[44]	Dong D Zhang Y L Zou C L Zou X B Guo G C 2015 Chin. Phys. 24 100306
[45]	Feng X L Gong S Q Xu Z Z 2000 Phys. Lett. 271 44
[46]	Metwally N Obada A S 2006 Phys. Lett. 352 45
[47]	Chi D P Kim T Lee S 2012 Phys. Lett. 376 143
[48]	Xu Y Y Feng X L Zhang Z M 2012 Chin. Opt. Lett. 10 042701
[49]	Jafarpour M Ashrafpouri F 2015 Quantum Inform. Process. 14 607
[50]	Cai C Zhou L Sheng Y B 2015 Chin. Phys. 24 120306
[51]	Deng F G Ren B C Li X H 2017 Sci. Bull. 62 46
[52]	Yamamoto T Koashi M Imoto N 2001 Phys. Rev. 64 012304
[53]	Wang X L Chen L K Li W Huang H L Liu C Chen C Luo Y H Su Z E Wu D Li Z D Lu H Hu Y Jiang X Peng C Z Li L Liu N L Chen Y A Lu C Y Pan J W 2016 Phys. Rev. Lett. 117 210502
[54]	Wang X L Cai X D Su Z E Chen M C Wu D Li L Liu N L Lu C Y Pan J W 2015 Nature 518 516
[55]	Zhang C Huang Y F Wang Zhao Liu B H Li C F Guo G C 2016 Phys. Rev. Lett. 115 260402