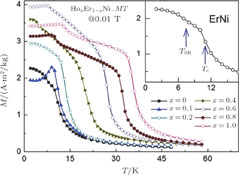
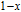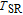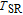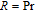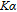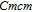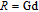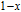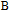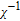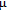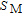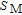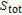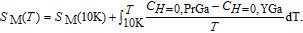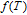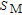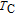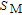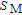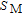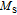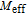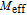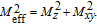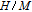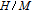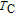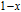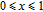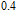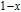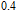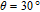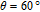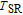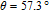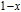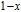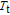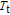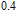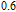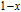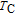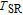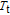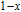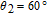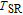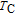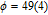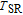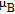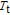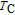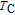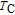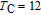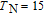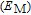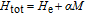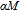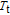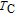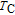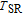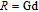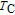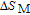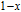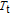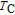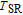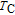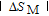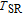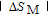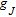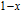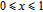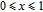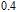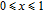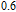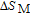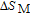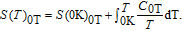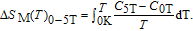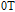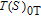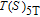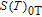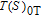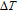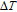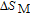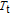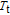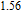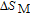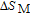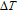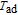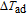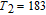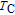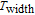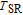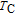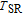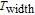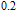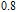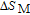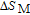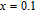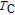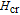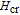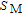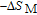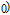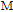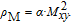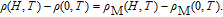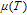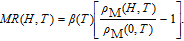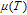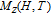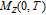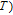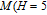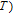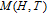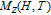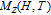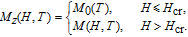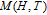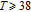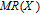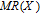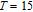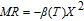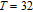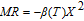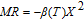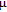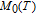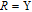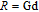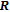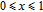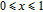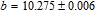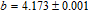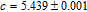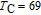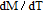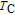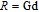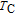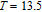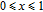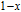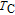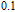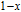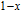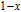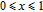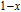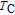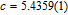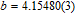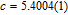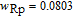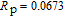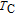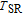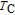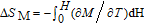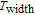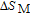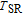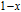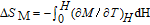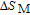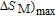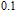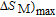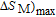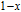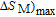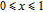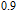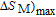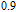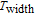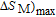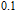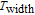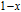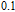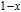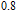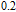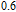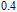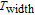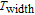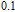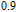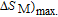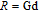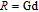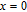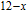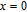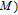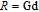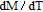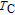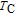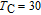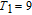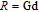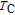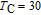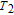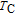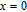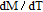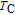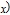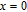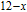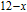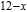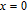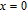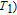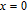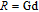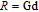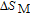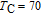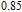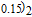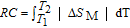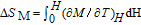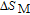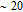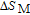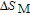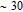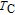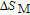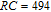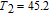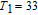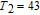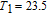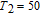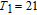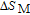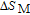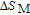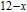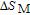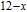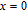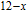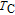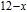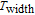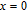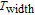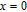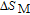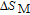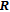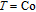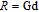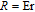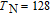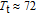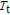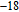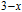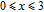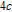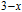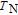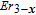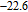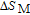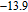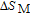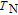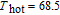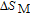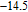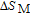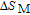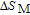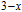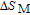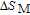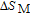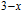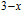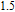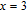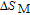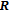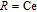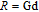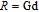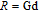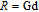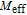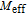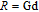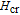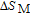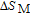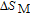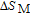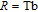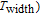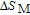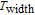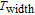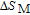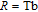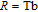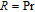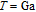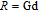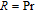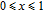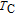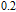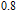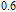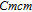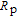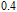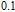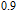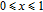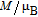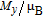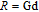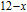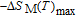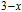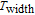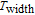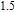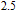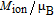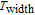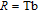2.
 Ga compounds
Ga compoundsThe crystal structure of RGa compounds have been investigated before. The RGa binary compounds crystallize in the orthorhombic CrB-type structure (space group cmcm #64). The structure was described as the stacking of the slabs of trigonal prisms with R atoms at the corners of the prism and the Ga atoms nearly at the corner.[20,21] Both R and Ga atoms are in the same crystallographic 4(c) sites with the C
2v point symmetry described as (0 0 0; 0.5 0.5 0.5) + (0 y0.25; 0
 0.75).[22,23] The axes of the prisms are parallel to the crystallographic b axis, displaced by (0.5, 0.5, 0), so that each Ga atom has seven neareat R neighbors.[20] The basic magnetic properties of RGa compounds have also been studied. Magnetization and susceptibility measurements have been done on the RGa (
0.75).[22,23] The axes of the prisms are parallel to the crystallographic b axis, displaced by (0.5, 0.5, 0), so that each Ga atom has seven neareat R neighbors.[20] The basic magnetic properties of RGa compounds have also been studied. Magnetization and susceptibility measurements have been done on the RGa (
 , Nd, Sm, Gd, Tb, Dy, Ho, Er) compounds. The Curie temperatures are determined to be 32 K, 44 K, 108 K, 183 K, 158 K, 116 K, 63 K, and 32 K, respectively. And the calculation of effective paramagnetic moments indicates that only R atoms contribute to the magnetic moment in RGa compounds.[24] According to the magnetization measurements on polycrystalline and single crystal RGa compounds, these compounds are ferromagnetic with large magneto-crystalline anisotropy at low temperatures.[22,24–26] A non-collinear ferrimagnetic structure is observed for ErGa compound by neutron diffraction experiments.[27] According to the researches on the single crystal RGa compounds, the easy direction of magnetization for PrGa compound is found to be c axis.[22] The spin reorientation (SR) of RGa compounds have been studied by Mössbauer spectrum experiments.[20,28] SR in NdGa, SmGa, HoGa, and ErGa compounds is observed obviously. The magnetic moments of GdGa show a direction with
, Nd, Sm, Gd, Tb, Dy, Ho, Er) compounds. The Curie temperatures are determined to be 32 K, 44 K, 108 K, 183 K, 158 K, 116 K, 63 K, and 32 K, respectively. And the calculation of effective paramagnetic moments indicates that only R atoms contribute to the magnetic moment in RGa compounds.[24] According to the magnetization measurements on polycrystalline and single crystal RGa compounds, these compounds are ferromagnetic with large magneto-crystalline anisotropy at low temperatures.[22,24–26] A non-collinear ferrimagnetic structure is observed for ErGa compound by neutron diffraction experiments.[27] According to the researches on the single crystal RGa compounds, the easy direction of magnetization for PrGa compound is found to be c axis.[22] The spin reorientation (SR) of RGa compounds have been studied by Mössbauer spectrum experiments.[20,28] SR in NdGa, SmGa, HoGa, and ErGa compounds is observed obviously. The magnetic moments of GdGa show a direction with
 or a direction with
or a direction with
 below
below
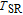 , but they point to an identify direction with
, but they point to an identify direction with
 above
above
 . In other words, the moments rotate towards the b axis in the ab plane with temperature increasing. The direction of the moments for ErGa below
. In other words, the moments rotate towards the b axis in the ab plane with temperature increasing. The direction of the moments for ErGa below
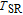 is complex. But it is proved that the moments also rotate toward b axis with temperature increasing.[20] The Curie temperature of PrGa compound is determined to be 36 K with a large derivation with previous results.[20,22,24] The SR transition of HoGa and GdGa was studied in detail by Susilo et al.[29,30] The MCE of GdGa compound is also studied, but the temperature range is between 100 K and 265 K, which is not large enough to study the influence of SR on MCE.[31]
is complex. But it is proved that the moments also rotate toward b axis with temperature increasing.[20] The Curie temperature of PrGa compound is determined to be 36 K with a large derivation with previous results.[20,22,24] The SR transition of HoGa and GdGa was studied in detail by Susilo et al.[29,30] The MCE of GdGa compound is also studied, but the temperature range is between 100 K and 265 K, which is not large enough to study the influence of SR on MCE.[31]
In the following sections, the magnetic properties, magnetic transitions and MCEs of RGa compounds will be studied and discussed in detail.
2.1. The crystal structure and magnetic structure of RGa compoundsThe polycrystalline RGa (
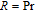 , Gd, Tb, Dy, Ho, Er, Tm) compounds were prepared by arc melting R and Ga in argon atmosphere. And the purity of the starting elements is more than 99.9%. In order to make the ingots homogenous, they were turned over and melted several times. Then the samples were wrapped in molybdenum foils, sealed in a high-vacuum quartz tube and annealed at 800
, Gd, Tb, Dy, Ho, Er, Tm) compounds were prepared by arc melting R and Ga in argon atmosphere. And the purity of the starting elements is more than 99.9%. In order to make the ingots homogenous, they were turned over and melted several times. Then the samples were wrapped in molybdenum foils, sealed in a high-vacuum quartz tube and annealed at 800
 C for 7 days. After annealing they were quenched into liquid Nitrogen. To confirm purity and crystal structure of the samples, we carried out powder x-ray diffraction (XRD) experiments with Cu
C for 7 days. After annealing they were quenched into liquid Nitrogen. To confirm purity and crystal structure of the samples, we carried out powder x-ray diffraction (XRD) experiments with Cu
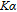 radiation. The XRD patterns of RGa (
radiation. The XRD patterns of RGa (
 , Tb, Dy, Er, (Gd, Er)) compounds are shown in Fig. 1.[32,34] And the inset of Fig. 1 is the rietveld refined powder XRD patterns of TbGa compound. Results show that all of the peaks can correspond to the Bragg positions of the orthorhombic CrB-type structure (space group
, Tb, Dy, Er, (Gd, Er)) compounds are shown in Fig. 1.[32,34] And the inset of Fig. 1 is the rietveld refined powder XRD patterns of TbGa compound. Results show that all of the peaks can correspond to the Bragg positions of the orthorhombic CrB-type structure (space group
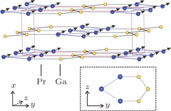
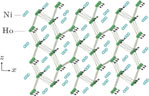
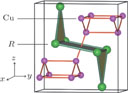
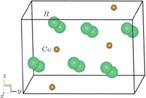
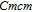 ), which indicates that the prepared compounds are of single phase. The crystal structure of RGa compounds has been described before,[20,22] but we can also describe it from another view. The detailed crystal structure of PrGa compound is shown in Fig. 2. All of the atoms distribute in a form of layers perpendicular to a-axis, and Pr and Ga atoms are connected in a form of hexagon rings within each layer. Other RGa compound has the same crystal structure.
), which indicates that the prepared compounds are of single phase. The crystal structure of RGa compounds has been described before,[20,22] but we can also describe it from another view. The detailed crystal structure of PrGa compound is shown in Fig. 2. All of the atoms distribute in a form of layers perpendicular to a-axis, and Pr and Ga atoms are connected in a form of hexagon rings within each layer. Other RGa compound has the same crystal structure.
High resolution neutron powder diffraction (NDP) experiment was then employed to study the magnetic structure of PrGa compound. The NPD experiments were performed at 295 K and 4.5 K, respectively. Fitting and calculations were carried out by Rietveld refinement method afterwards. The observed and the calculated neutron powder diffraction patterns at 295 K and 4.5 K are shown in Figs. 3(a) and 3(b). NPD results at 295 K show that PrGa compound has a pure phase and it crystalizes in a CrB-type orthorhombic structure (space group #63 cmcm). Both the nuclear and the magnetic structure models are involved in the calculation at 4.5 K. The magnetic moments are FM-ordered along crystallographic c axis and the ordered magnetic moment is determined to be 2.6

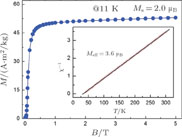
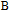 at 4.5 K.[33] Figure 4 shows the isothermal magnetization curve at 11K and the inset of Fig. 4 shows the temperature dependence of
at 4.5 K.[33] Figure 4 shows the isothermal magnetization curve at 11K and the inset of Fig. 4 shows the temperature dependence of
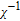 up to 300 K, where χ is the magnetic susceptibility. The linear relationship of
up to 300 K, where χ is the magnetic susceptibility. The linear relationship of
 and temperature in the high temperature range indicates that the Curie–Weiss law is appropriate here. According to the Curie–Weiss law and the definition of χ,
and temperature in the high temperature range indicates that the Curie–Weiss law is appropriate here. According to the Curie–Weiss law and the definition of χ,
 is calculated to be 3.6
is calculated to be 3.6

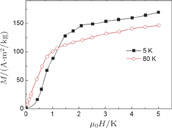
 from the slope of the linear fitting curve.[33] The MH curve at 5 K (not shown in Fig. 1) has also been measured and it is almost coincided with the MH curve at 11 K in high field range. For the isothermal magnetization curve at 5 K and 11 K, the magnetization increases with field increasing and reaches its saturation value rapidly, indicating that it is reasonable to calculate saturated magnetization
from the slope of the linear fitting curve.[33] The MH curve at 5 K (not shown in Fig. 1) has also been measured and it is almost coincided with the MH curve at 11 K in high field range. For the isothermal magnetization curve at 5 K and 11 K, the magnetization increases with field increasing and reaches its saturation value rapidly, indicating that it is reasonable to calculate saturated magnetization
 from the MH curve at 5 K or 11 K.
from the MH curve at 5 K or 11 K.
 ʼs at these two temperature are calculated to be the same value of 2.0
ʼs at these two temperature are calculated to be the same value of 2.0
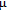
 , which is the average value per Pr atom.[33] The value of
, which is the average value per Pr atom.[33] The value of
 is similar to the ordered magnetic moment obtained from NPD experiments at 4.5 K but considerably smaller than that of
is similar to the ordered magnetic moment obtained from NPD experiments at 4.5 K but considerably smaller than that of
 .
.
In order to study the magnetic ground state of PrGa compound at low temperature, we investigated the degree of magnetic order, which is known as magnetic entropy (
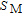 ). The total entropy can be calculated from the integration of the heat capacity data:
). The total entropy can be calculated from the integration of the heat capacity data:
 | (1) |
where

is the reference temperature,
C
H
is the heat capacity under a field of
H. The heat capacity data at zero field for PrGa and YGa was measured. We first took

K as reference temperature and calculated the total entropy of PrGa and YGa compound, respectively. Then we can obtain
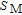
of PrGa by subtracting
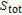
of YGa from

of PrGa, and the above method has been used before.
[35,36]
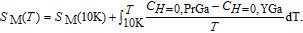 | (2) |
Now we introduce a new function
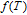
.
 | (3) |
The right-hand side of Eq. (
3) can be calculated and the curve was plotted in Fig.
5.
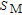
increases as temperature goes up. When the temperature exceeds
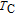
,
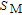
changes slowly and reaches its maximum value gradually.
 | (4) |
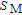
in PM zone can be calculated from the following equation
[37]
 | (5) |
where
N is the number of magnetic moments,

is the Boltzmann constant, and
J is the total angular quantum number. Therefore we can obtain

(10 K) = 29.65 J/kg

K.
[33] PrGa compound is in stable FM ordered state below 11 K. So the large value of
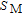
at 10 K is unusual. This indicates that the magnetic moments of PrGa are not parallel completely at 10 K, and more complex magnetic structure may exist. This conclusion is in accord with the results of NPD experiments and the analysis of
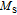
and
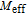
.
Considering that
 is much smaller than
is much smaller than
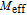 and
and
 at 10 K is considerably larger than zero, we can assume that the magnetic moments point to different directions but with a fixed deviation angle from c axis and the value of 2.5
at 10 K is considerably larger than zero, we can assume that the magnetic moments point to different directions but with a fixed deviation angle from c axis and the value of 2.5
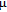
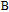 is the projection of the magnetic moments on c axis.[33] If we draw all of the magnetic moments at the same atom site, we can see that they are distributed randomly within a conical surface. The sketch of the magnetic structure of PrGa is shown in Fig. 6. The real magnetic moment is
is the projection of the magnetic moments on c axis.[33] If we draw all of the magnetic moments at the same atom site, we can see that they are distributed randomly within a conical surface. The sketch of the magnetic structure of PrGa is shown in Fig. 6. The real magnetic moment is
 and every magnetic moment can be resolved into two components. M
z
and M
xy
are the components along the c axis and within the ab crystal plane, respectively.[33] Furthermore, M
z
is long-range FM-ordered but M
xy
is distributed randomly without any long-range order.
and every magnetic moment can be resolved into two components. M
z
and M
xy
are the components along the c axis and within the ab crystal plane, respectively.[33] Furthermore, M
z
is long-range FM-ordered but M
xy
is distributed randomly without any long-range order.
 , M
z
, and M
xy
have the following relation:
, M
z
, and M
xy
have the following relation:
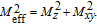 | (6) |
2.2. The magnetic properties and magnetic transitions of
 Ga compounds
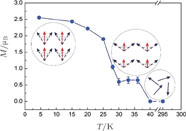
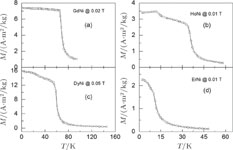
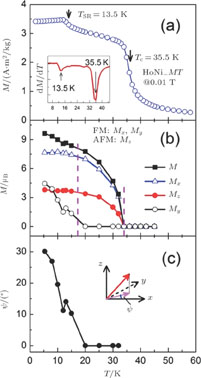
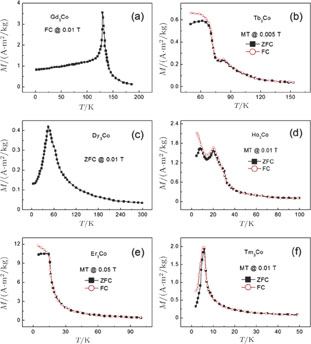
Ga compoundsSpin reorientation has been observed in some of the RGa compounds, such as PrGa, SmGa, HoGa, and ErGa according to the results of Mössbauer spectrum experiments.[20] Here we studied the magnetic properties of RGa compounds in detail including PrGa, GdGa, TbGa, DyGa, HoGa, ErGa, and TmGa compounds. First of all, we analyze the magnetic properties and magnetic transitions of heavy-rare-earth RGa compounds. Figure 7 is the thermal magnetization curves of GdGa, TbGa, DyGa, HoGa, and ErGa compounds under a field of 0.01 T. And the inset of Fig. 7 shows the zero-field-cooled (ZFC) thermal magnetization and field-cooled (FC) thermal magnetization curves of TmGa compound under a field of 0.04 T. All of the M–T curves show hight-variability twice as temperature increases. Besides of the drastic change around Curie temperature (
 , another obvious change of magnetization is observed in low temperature ranges, which is described as SR transition and the transition temperature is marked as
, another obvious change of magnetization is observed in low temperature ranges, which is described as SR transition and the transition temperature is marked as
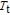 .[19,32,34,38] The transition temperatures for SR are determined to be 66 K, 31 K, 25 K, 20 K, and 15 K for GdGa, TbGa, DyGa, HoGa, and ErGa compounds, respectively. The Curie temperatures are determined to be 181.9 K, 154 K, 113 K, 69 K, and 30 K for GdGa, TbGa, DyGa, HoGa, and ErGa compounds, respectively. It is clearly that
.[19,32,34,38] The transition temperatures for SR are determined to be 66 K, 31 K, 25 K, 20 K, and 15 K for GdGa, TbGa, DyGa, HoGa, and ErGa compounds, respectively. The Curie temperatures are determined to be 181.9 K, 154 K, 113 K, 69 K, and 30 K for GdGa, TbGa, DyGa, HoGa, and ErGa compounds, respectively. It is clearly that
 decreases steadily as the atomic number of R atoms increases, but the alteration of
decreases steadily as the atomic number of R atoms increases, but the alteration of
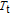 is not as obvious as R atom changes. The value of spin quantum number (S) decreases with the atomic number of R atoms increases, and
is not as obvious as R atom changes. The value of spin quantum number (S) decreases with the atomic number of R atoms increases, and
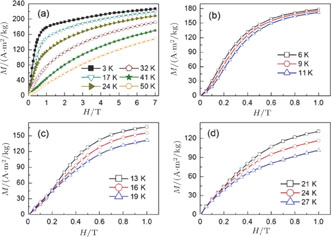
 is positively correlated with S. Figure 8(a) shows the isothermal magnetization curves at different temperatures for ErGa compound. All of the M–H curves at the temperature range above
is positively correlated with S. Figure 8(a) shows the isothermal magnetization curves at different temperatures for ErGa compound. All of the M–H curves at the temperature range above
 show typical FM characteristic. That is, magnetization increases as magnetic field goes up and reaches its saturation value quickly. Therefore, the SR magnetic transition is also ferromagnetic (FM) to FM transition. TmGa compound exhibits two successive magnetic transitions: ferromagnetic (FM) to antiferromagnetic (AFM) transition at
show typical FM characteristic. That is, magnetization increases as magnetic field goes up and reaches its saturation value quickly. Therefore, the SR magnetic transition is also ferromagnetic (FM) to FM transition. TmGa compound exhibits two successive magnetic transitions: ferromagnetic (FM) to antiferromagnetic (AFM) transition at
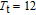 K and AFM to paramagnetic (PM) transition at
K and AFM to paramagnetic (PM) transition at
 K.[39] The ZFC and FC curves are well overlapped above
K.[39] The ZFC and FC curves are well overlapped above
 , indicating that thermal hysteresis is very small as observed in other MCE materials with a second-order magnetic transition.[16] However, there is an obvious bifurcation at temperatures below
, indicating that thermal hysteresis is very small as observed in other MCE materials with a second-order magnetic transition.[16] However, there is an obvious bifurcation at temperatures below
 and it is likely due to a domain wall pinning effect as described in literature.[40] All of the transition temperatures are listed in Table 1.
and it is likely due to a domain wall pinning effect as described in literature.[40] All of the transition temperatures are listed in Table 1.
Figure 8(b) shows that the M–H curves measured in field increasing and decreasing mode almost coincide, which means good magnetic reversibility. The ZFC and FC of ErGa compound also show no thermal hysteresis around
 . According to the Banerjee criterion,[42] a magnetic transition is expected to be of the first order when the slope of M
2 versus
. According to the Banerjee criterion,[42] a magnetic transition is expected to be of the first order when the slope of M
2 versus
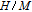 plot is negative, whereas it will be of the second order when the slope is positive. The relationship between
plot is negative, whereas it will be of the second order when the slope is positive. The relationship between
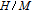 and M
2 has been calculated for ErGa compound and the arrott plot is not shown here. The positive slopes of plot around
and M
2 has been calculated for ErGa compound and the arrott plot is not shown here. The positive slopes of plot around
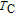 confirm that the FM–PM transition is of second order,[42] which accords well with the case where both thermal and magnetic hysteresis are absent. Further results show that all of the RGa (
confirm that the FM–PM transition is of second order,[42] which accords well with the case where both thermal and magnetic hysteresis are absent. Further results show that all of the RGa (
 , Gd, Tb, Dy, Ho, Er, Tm) compounds are of second order transition materials.
, Gd, Tb, Dy, Ho, Er, Tm) compounds are of second order transition materials.
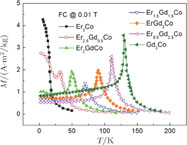
The thermal magnetization curves of Gd
x
Er
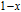 Ga (
Ga (
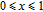 ) compounds are measured at a field of 0.1 T and the temperature dependence of the magnetization is shown in Fig. 9. Sharp decrease is observed on each M–T curve around
) compounds are measured at a field of 0.1 T and the temperature dependence of the magnetization is shown in Fig. 9. Sharp decrease is observed on each M–T curve around
 , and another anomaly is observed in low temperature range for all the samples. In the compounds with a low content of Gd, the magnetic properties are similar to ErGa compound. And when the content of Gd is large, such as Gd
, and another anomaly is observed in low temperature range for all the samples. In the compounds with a low content of Gd, the magnetic properties are similar to ErGa compound. And when the content of Gd is large, such as Gd
 Er
Er
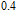 Ga and Gd
Ga and Gd
 Er
Er
 Ga, the magnetic properties show similar feature to GdGa compound.[34] According to previous analysis, the transition in low temperature range is SR transition for most Gd
x
Er
Ga, the magnetic properties show similar feature to GdGa compound.[34] According to previous analysis, the transition in low temperature range is SR transition for most Gd
x
Er
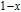 Ga (
Ga (
 ) compounds and we marked that transition temperature as
) compounds and we marked that transition temperature as
 . For Gd
. For Gd
 Er
Er
 Ga and Gd
Ga and Gd
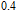 Er
Er
 Ga compounds, the transition in low temperature range is an AFM to FM transition, which will be discussed later. And we mark that transition temperature as
Ga compounds, the transition in low temperature range is an AFM to FM transition, which will be discussed later. And we mark that transition temperature as
 in this case. In fact, the SR transition of ErGa and GdGa has been studied carefully by Mössbauer spectrum.[20] The magnetic moment points to different directions at different temperatures and it results from the competition between exchange interaction and crystal field interaction. The magnetic moments of GdGa show a direction with
in this case. In fact, the SR transition of ErGa and GdGa has been studied carefully by Mössbauer spectrum.[20] The magnetic moment points to different directions at different temperatures and it results from the competition between exchange interaction and crystal field interaction. The magnetic moments of GdGa show a direction with
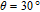 or a direction with
or a direction with
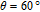 below
below
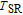 , but they point to an identify direction with
, but they point to an identify direction with
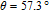 above
above
 .[20] In other words, the moments rotate towards the b axis in the ab plane with temperature increasing. The direction of the moments for ErGa below
.[20] In other words, the moments rotate towards the b axis in the ab plane with temperature increasing. The direction of the moments for ErGa below
 is complex. But it is proved that the moments also rotate toward b axis with temperature increasing.
is complex. But it is proved that the moments also rotate toward b axis with temperature increasing.
Isothermal magnetization curves up to 5 T at 5 K for Gd
x
Er
 Ga (
Ga (
 ) compounds are shown in Fig. 10. The magnetization goes up and comes to its saturation value quickly with magnetic field increasing for Gd
x
Er
) compounds are shown in Fig. 10. The magnetization goes up and comes to its saturation value quickly with magnetic field increasing for Gd
x
Er
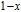 Ga (
Ga (
 , 0.1, 0.2, 0.6, 0.8, and 1.0) compounds, and it is the typical characteristic of the FM ground state. For Gd
, 0.1, 0.2, 0.6, 0.8, and 1.0) compounds, and it is the typical characteristic of the FM ground state. For Gd
 Er
Er
 Ga and Gd
Ga and Gd
 Er
Er
 Ga compounds, the magnetic exchange interaction is not large enough to counterbalance the crystal field interaction below
Ga compounds, the magnetic exchange interaction is not large enough to counterbalance the crystal field interaction below
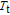 , and Er and Gd magnetic moment each points to the favorite directions on their own.[34] That is to say, the ground state is AFM below
, and Er and Gd magnetic moment each points to the favorite directions on their own.[34] That is to say, the ground state is AFM below
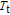 for Gd
for Gd
 Er
Er
 Ga and Gd
Ga and Gd
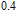 Er
Er
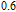 Ga compounds. As a result, the magnetization at 5 K increases linearly with increasing magnetic field in low field ranges (see Fig. 10). Besides, it is found that the magnetization exhibits a sharp increase when the applied field exceeds a certain value, indicating that the field-induced metamagnetic transition from AFM to FM state occurs. The critical field required for metamagnetic transition for Gd
Ga compounds. As a result, the magnetization at 5 K increases linearly with increasing magnetic field in low field ranges (see Fig. 10). Besides, it is found that the magnetization exhibits a sharp increase when the applied field exceeds a certain value, indicating that the field-induced metamagnetic transition from AFM to FM state occurs. The critical field required for metamagnetic transition for Gd
 Er
Er
 Ga and Gd
Ga and Gd
 Er
Er
 Ga, which is determined from the maximum of
Ga, which is determined from the maximum of
 , is found to be 0.98 T and 1.03 T, respectively.[34] The transition temperatures of Gd
x
Er
, is found to be 0.98 T and 1.03 T, respectively.[34] The transition temperatures of Gd
x
Er
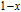 Ga (
Ga (
 ) compounds, which are determined from derivative of M–T curves, are all listed in Table 1. We can see that
) compounds, which are determined from derivative of M–T curves, are all listed in Table 1. We can see that
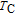 increases from 30 K to 181.9 K as the content of Gd increases from 0 to 1. The reason is that the average value of spin quantum number (S) increases with the content of Gd, and
increases from 30 K to 181.9 K as the content of Gd increases from 0 to 1. The reason is that the average value of spin quantum number (S) increases with the content of Gd, and
 increases with S increasing. The
increases with S increasing. The
 and
and
 are listed in the same column, and results show that the change of
are listed in the same column, and results show that the change of
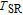 or
or
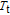 is not as large as
is not as large as
 . The lowest and highest values of
. The lowest and highest values of
 are 15 K and 66 K respectively for the Gd
x
Er
are 15 K and 66 K respectively for the Gd
x
Er
 Ga compounds.
Ga compounds.
The SR transition due to the competition between the magnetic exchange interaction and the crystal field interaction has been observed in NdGa, SmGa, HoGa, ErGa, and GdGa compounds by Mössbauer spectrum experiments.[20] With decreasing temperature, the magnetic moments rotate gradually from the bc plane toward the crystallographic a axis. For HoGa compound, the azimuth angle ϕ does not change with temperature and the rotation of the magnetic moments occurs in the ac plane when only the angle θ is changed. For GdGa compound, in the temperature range 5 K To 100 K, the ferromagnetic structure of the GdGa compound is noncollinear. At 5 K the magnetic moments of the Gd
 ions point in two distinct directions with respect to the crystallographic a axis (
ions point in two distinct directions with respect to the crystallographic a axis (
 and
and
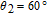 ).[20] High-resolution neutron powder diffraction was also employed to study the SR transition of HoGa compound.[29] In the temperature range between
).[20] High-resolution neutron powder diffraction was also employed to study the SR transition of HoGa compound.[29] In the temperature range between
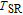 and
and
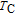 , the magnetic structure comprises ferromagnetic order of the Ho sublattice along the c axis. Upon cooling below
, the magnetic structure comprises ferromagnetic order of the Ho sublattice along the c axis. Upon cooling below
 , the Ho magnetic moments cant away from the c axis towards the ab plane. At 3 K, the Ho moment is 8.8(2)
, the Ho magnetic moments cant away from the c axis towards the ab plane. At 3 K, the Ho moment is 8.8(2)
 and the Ho magnetic moments point in the direction
and the Ho magnetic moments point in the direction
 and
and
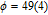 with respect to the crystallographic c axis. The observation of an ab plane component at around 50 from the a axis is in contrast with the suggested magnetic structure reported on the basis of a 119Sn Mössbauer spectroscopy study of an Sn-doped HoGa sample.[20,29] The SR transition GdGa compound is also studied by 155Gd Mössbauer spectroscopy and neutron powder diffraction.[30] Between
with respect to the crystallographic c axis. The observation of an ab plane component at around 50 from the a axis is in contrast with the suggested magnetic structure reported on the basis of a 119Sn Mössbauer spectroscopy study of an Sn-doped HoGa sample.[20,29] The SR transition GdGa compound is also studied by 155Gd Mössbauer spectroscopy and neutron powder diffraction.[30] Between
 and
and
 , the magnetic structure is characterized by ferromagnetic order of the Gd moments along the b axis. On cooling below
, the magnetic structure is characterized by ferromagnetic order of the Gd moments along the b axis. On cooling below
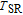 , the Gd 4c magnetic moments split into two groups (2:2). At 3.6 K, the Gd moment is 6.7(4)
, the Gd 4c magnetic moments split into two groups (2:2). At 3.6 K, the Gd moment is 6.7(4)
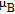 , and the Gd magnetic moments are in the bc plane, canted by 84(3) and 46(4) with respect to the crystallographic b axis. This splitting into two magnetically inequivalent sites is confirmed by 5 K 155Gd Mössbauer results.[30]
, and the Gd magnetic moments are in the bc plane, canted by 84(3) and 46(4) with respect to the crystallographic b axis. This splitting into two magnetically inequivalent sites is confirmed by 5 K 155Gd Mössbauer results.[30]
The thermal magnetization curves at different magnetic fields and isothermal magnetization curves are measured for PrGa compound, which are shown in Fig. 11. From M–T curves at 0.05 T, we can see that the magnetization curve experiences two drops at 28 K and 38 K respectively. The sharp changes are corresponding to two magnetic transition temperatures marked as
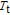 and
and
 . The transition at lower temperature is similar to the FM to AFM transition and it seems to be supported by isothermal magnetization results shown in Fig. 11(b). In the temperature range between
. The transition at lower temperature is similar to the FM to AFM transition and it seems to be supported by isothermal magnetization results shown in Fig. 11(b). In the temperature range between
 and
and
 , the magnetization curve shows a linear increase and then a sudden jump with magnetic field increasing, which appears to indicate the ground state is AFM and there is a metamagnetic transition from AFM to FM phase.[41,43] As the applied field is added,
, the magnetization curve shows a linear increase and then a sudden jump with magnetic field increasing, which appears to indicate the ground state is AFM and there is a metamagnetic transition from AFM to FM phase.[41,43] As the applied field is added,
 moves to higher temperature zone. It indicates that the temperature region of metamagnetic transition gets larger when the applied field increases. To make it clear, partially enlarged view is shown in the inset of Fig. 11(a). When the applied field is high enough, metamagnetic transition occurs in the whole temperature region between
moves to higher temperature zone. It indicates that the temperature region of metamagnetic transition gets larger when the applied field increases. To make it clear, partially enlarged view is shown in the inset of Fig. 11(a). When the applied field is high enough, metamagnetic transition occurs in the whole temperature region between
 and
and
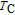 , thus the two drops of magnetization almost become one. The M–T curves of PrGa are very similar to those of LaFe
, thus the two drops of magnetization almost become one. The M–T curves of PrGa are very similar to those of LaFe
 Al
Al
 .[44] The critical field of metamagnetic transition determined by the maximum value of is also calculated. The result indicates that the critical field increases with temperature. In other words, the temperature zone where metamagnetic transition happens extends with applied field increasing. It is consistent with the conclusion obtained from M–T curve.
.[44] The critical field of metamagnetic transition determined by the maximum value of is also calculated. The result indicates that the critical field increases with temperature. In other words, the temperature zone where metamagnetic transition happens extends with applied field increasing. It is consistent with the conclusion obtained from M–T curve.
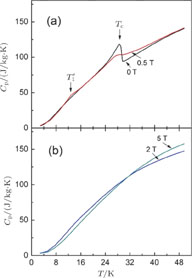
The temperature dependence of heat capacity at different magnetic field for YGa, PrGa, ErGa, and TmGa compounds are measured and the C
H
–T curves are shown in Fig. 12. The YGa compound is non-magnetic and has the same crystal structure with other RGa compounds. The C
H
–T curve of the YGa compound obeys the typical heat capacity change rule as temperature increases and there is no peak observed on the curve (see Fig. 12(a)). Unlike the YGa compound, peaks are observed on the C
H
–T curves of magnetic PrGa, ErGa, and TmGa compounds. The heat capacity results show similar change rules with M–T curves. Two peaks are clearly shown on the C
H
–T curve when the applied field is zero for the PrGa compound (Fig. 12(b)). And the two peaks are corresponding to
 and
and
 respectively. The peak at lower temperature moves toward higher zone with applied field increasing. When the applied field exceeds a certain value, the two peaks turn into a large one, such as those in the case of 1, 2, and 5 T.[41] The C
H
–T curves of the ErGa compound for 0 T, 1 T, 2 T, and 5 T are shown in Fig. 12(c). Two sharp peaks are observed at 15 K and 30 K under zero-field, corresponding to the SR and FM to PM transitions, respectively. With the application of magnetic field, the intensity of the peak at
respectively. The peak at lower temperature moves toward higher zone with applied field increasing. When the applied field exceeds a certain value, the two peaks turn into a large one, such as those in the case of 1, 2, and 5 T.[41] The C
H
–T curves of the ErGa compound for 0 T, 1 T, 2 T, and 5 T are shown in Fig. 12(c). Two sharp peaks are observed at 15 K and 30 K under zero-field, corresponding to the SR and FM to PM transitions, respectively. With the application of magnetic field, the intensity of the peak at
 is reduced greatly, and the peak shifts toward higher temperatures with field increasing. On the other hand, the peak at
is reduced greatly, and the peak shifts toward higher temperatures with field increasing. On the other hand, the peak at
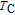 spreads to a broad anomaly with a much lower magnitude when a field larger than 1 T is applied, and it almost disappears under a field of 5 T. It may also be noted from Fig. 12(c) that the values of heat capacity in applied fields above
spreads to a broad anomaly with a much lower magnitude when a field larger than 1 T is applied, and it almost disappears under a field of 5 T. It may also be noted from Fig. 12(c) that the values of heat capacity in applied fields above
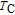 are much larger than those at zero-field in a wide temperature range. The abnormal heat capacity of ErGa may be related to the existence of short-range FM correlations in the PM state
are much larger than those at zero-field in a wide temperature range. The abnormal heat capacity of ErGa may be related to the existence of short-range FM correlations in the PM state
 .[19] Figure 12(d) displays the heat capacity curves for TmGa under the fields of 0, 1, 2, and 5 T, respectively. It clearly shows two successive magnetic transitions at
.[19] Figure 12(d) displays the heat capacity curves for TmGa under the fields of 0, 1, 2, and 5 T, respectively. It clearly shows two successive magnetic transitions at
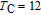 K and at
K and at
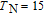 K, which is fully consistent with the magnetic measurements.[39] With the increase of magnetic field, the two peaks are not only turn a broader and lower, but also shifts toward higher temperature, which is the typical characteristic of FM material.[2] It is known that the heat capacity peak is caused by the absorption of heat which is utilized in randomization of magnetic moments around transition temperature.[39] With the application of field, the randomization of moments would spread out over a wide temperature region, and the maximum peak moves towards higher temperature.[5] In fact, the performance of C
H
–T curve is the inevitable result of thermal magnetization data. Now we discuss the form of the magnetic contribution to heat capacity approximately. The magnetic component of internal energy
K, which is fully consistent with the magnetic measurements.[39] With the increase of magnetic field, the two peaks are not only turn a broader and lower, but also shifts toward higher temperature, which is the typical characteristic of FM material.[2] It is known that the heat capacity peak is caused by the absorption of heat which is utilized in randomization of magnetic moments around transition temperature.[39] With the application of field, the randomization of moments would spread out over a wide temperature region, and the maximum peak moves towards higher temperature.[5] In fact, the performance of C
H
–T curve is the inevitable result of thermal magnetization data. Now we discuss the form of the magnetic contribution to heat capacity approximately. The magnetic component of internal energy
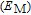 can be expressed as
can be expressed as
 , where M is the magnetic moment of the compound and
, where M is the magnetic moment of the compound and
 is the total magnetic field in the compound. According to the theory of mean field, the total magnetic field can be expressed as
is the total magnetic field in the compound. According to the theory of mean field, the total magnetic field can be expressed as
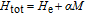 , where
, where
 is the external field, α is the mean field constant, and
is the external field, α is the mean field constant, and
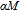 represents the internal field.[31,45] The magnetic contribution to heat capacity can be obtained by
represents the internal field.[31,45] The magnetic contribution to heat capacity can be obtained by
 , thus we can see that
, thus we can see that
 . According to this formula, a sudden drop of magnetic moment on the M–T curve can definitely cause a sharp peak on the C
H
–T curve. Every magnetic transition is accompanied by a sudden drop of magnetic moment in magnetic RGa compound. As a result, obvious peaks are observed on C
H
–T curves near transition temperatures.
. According to this formula, a sudden drop of magnetic moment on the M–T curve can definitely cause a sharp peak on the C
H
–T curve. Every magnetic transition is accompanied by a sudden drop of magnetic moment in magnetic RGa compound. As a result, obvious peaks are observed on C
H
–T curves near transition temperatures.
According to the magnetic and heat capacity measurements, ErGa compound undergoes an SR transition and an FM to PM transition as temperature increases.[19] And the SR transition has been confirmed by 119Sn Mössbauer spectroscopy study.[20] Two magnetic transitions are also observed according to magnetic and heat capacity measurements for PrGa compound.[41] However, the no SR transition is observed according to 119Sn Mössbauer spectroscopy experiment.[20] So it is still not clear that what kind of magnetic transition it is at
 and whether the ground state at the temperature range between
and whether the ground state at the temperature range between
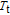 and
and
 is AFM. High resolution neutron powder diffraction (NPD) is a good way to study the magnetic properties. The magnetic structure of PrGa comound at 4.5 K has been determined in the above section. Then the change of magnetic structure as temperature increasing and magnetic transition of PrGa compound can also be studied by NPD method. All the magnetic structures at different temperatures were solved out. The detailed results including crystal lattice parameters, ordered magnetic moments and errors of refinement are listed in Table 2. The temperature dependence of the ordered magnetic moment and the sketch of the magnetic structures are shown in Fig. 13. As temperature increases, the magnetic moment shows a sudden decrease around
is AFM. High resolution neutron powder diffraction (NPD) is a good way to study the magnetic properties. The magnetic structure of PrGa comound at 4.5 K has been determined in the above section. Then the change of magnetic structure as temperature increasing and magnetic transition of PrGa compound can also be studied by NPD method. All the magnetic structures at different temperatures were solved out. The detailed results including crystal lattice parameters, ordered magnetic moments and errors of refinement are listed in Table 2. The temperature dependence of the ordered magnetic moment and the sketch of the magnetic structures are shown in Fig. 13. As temperature increases, the magnetic moment shows a sudden decrease around
 K, whereas the direction of the ordered magnetic moment remains the same. That is to say, the only change of magnetic structure at
K, whereas the direction of the ordered magnetic moment remains the same. That is to say, the only change of magnetic structure at
 is the variation of angle of cone. At
is the variation of angle of cone. At
 K the ordered magnetic moment decreases to zero, which indicates an FM to PM transition. Therefore we can conclude that PrGa compound undergoes an FM to FM magnetic transition at
K the ordered magnetic moment decreases to zero, which indicates an FM to PM transition. Therefore we can conclude that PrGa compound undergoes an FM to FM magnetic transition at
 and an FM to PM transition at
and an FM to PM transition at
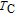 as temperature increases. According to the results of Mössbauer spectroscopy experiments, a magnetic spin reorientation transition from bc plane toward the a axis with decreasing temperature was observed for NdGa, SmGa, HoGa, and ErGa compounds.[18] But for PrGa compound, there is no such reorientation of the ordered magnetic moments around
as temperature increases. According to the results of Mössbauer spectroscopy experiments, a magnetic spin reorientation transition from bc plane toward the a axis with decreasing temperature was observed for NdGa, SmGa, HoGa, and ErGa compounds.[18] But for PrGa compound, there is no such reorientation of the ordered magnetic moments around
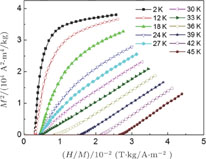
 , though the crystal structure of PrGa compound is almost the same as other RGa compounds. Furthermore, lattice parameters a, b, and c change as temperature increases, but only c shows an obvious response to both the FM to FM transition and the FM to PM transition, because the change of magnetic structure at transition temperatures occurs mainly along the c axis.
, though the crystal structure of PrGa compound is almost the same as other RGa compounds. Furthermore, lattice parameters a, b, and c change as temperature increases, but only c shows an obvious response to both the FM to FM transition and the FM to PM transition, because the change of magnetic structure at transition temperatures occurs mainly along the c axis.
Table 2.
Table 2.
 Table 2.
Refined structural parameters of PrGa compound. Space group
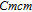 , Atomic positions: Pr (0, y, 0.25); Ga (0, y, 0.25). a, b, c, and V are lattice constant and volume of unit cell. M, M
x
, M
y
, and M
z
are the total ordered magnetic moment and the individual component of moment along a, b, and c axes, respectively. ϕ and θ are the angle between the ordered magnetic moment and x, z axes, respectively. [34]
, Atomic positions: Pr (0, y, 0.25); Ga (0, y, 0.25). a, b, c, and V are lattice constant and volume of unit cell. M, M
x
, M
y
, and M
z
are the total ordered magnetic moment and the individual component of moment along a, b, and c axes, respectively. ϕ and θ are the angle between the ordered magnetic moment and x, z axes, respectively. [34]
.
| Atoms |
Parameters |
4.5 K |
15 K |
20 K |
25 K |
28 K |
30 K |
33 K |
36 K |
40 K |
295 K |
|
|
a/Å |
4.4545(3) |
4.4545(3) |
4.4545(3) |
4.4538(3) |
4.4533(3) |
4.4522(3) |
4.4515(4) |
4.4506(3) |
4.4504(4) |
4.4494(4) |
|
|
b/Å |
11.2533(9) |
11.2530(9) |
11.2527(9) |
11.2547(9) |
11.259(1) |
11.261(1) |
11.264(1) |
11.265(1) |
11.267(1) |
11.312(1) |
|
|
c/Å |
4.1878(3) |
4.1880(3) |
4.1877(3) |
4.1875(3) |
4.1865(3) |
4.1860(3) |
4.1861(3) |
4.1862(3) |
4.1857(3) |
4.1953(4) |
|
|
V/Å3
|
209.93(4) |
209.93(4) |
209.91(4) |
209.90(4) |
209.91(4) |
209.87(4) |
209.90(5) |
209.88(4) |
209.89(5) |
211.15(5) |
| Pr |
y
|
0.1416(2) |
0.1419(2) |
0.1418(2) |
0.1421(2) |
0.1420(2) |
0.1425(3) |
0.1424(3) |
0.1426(3) |
0.1429(3) |
0.1441(3) |
|
|

|
0 |
0 |
0 |
0 |
0 |
0 |
0 |
0 |
0 |
0 |
|
|

|
2.56(4) |
2.43(4) |
2.22(4) |
1.90(4) |
1.05(6) |
0.6(1) |
0.6(1) |
0.65(9) |
0 |
0 |
|
|
 /(
/(
 )
) |
0 |
0 |
0 |
0 |
0 |
0 |
0 |
0 |
0 |
0 |
| Ga |
y
|
0.4301(2) |
0.4301(2) |
0.4305(2) |
0.4302(2) |
0.4303(2) |
0.4304(2) |
0.4306(2) |
0.4304(2) |
0.4306(2) |
0.4304(2) |
|
|
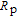 6.01
6.01 |
6.07 |
6.12 |
5.85 |
|
|
 7.39
7.39 |
7.29 |
7.29 |
7.08 |
|
|
χ
2
|
1.363 |
1.274 |
1.300 |
1.281 |
1.292 |
1.276 |
1.313 |
1.224 |
1.246 |
1.458 |
| Table 2.
Refined structural parameters of PrGa compound. Space group
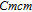 , Atomic positions: Pr (0, y, 0.25); Ga (0, y, 0.25). a, b, c, and V are lattice constant and volume of unit cell. M, M
x
, M
y
, and M
z
are the total ordered magnetic moment and the individual component of moment along a, b, and c axes, respectively. ϕ and θ are the angle between the ordered magnetic moment and x, z axes, respectively. [34]
, Atomic positions: Pr (0, y, 0.25); Ga (0, y, 0.25). a, b, c, and V are lattice constant and volume of unit cell. M, M
x
, M
y
, and M
z
are the total ordered magnetic moment and the individual component of moment along a, b, and c axes, respectively. ϕ and θ are the angle between the ordered magnetic moment and x, z axes, respectively. [34]
. |
2.3. The magnetocaloric effects of
 Ga compounds
Ga compoundsThe magnetic entropy change of RGa (
 , Tb, Dy, Ho, Er, Tm) compounds was calculated from magnetization isotherms by using the Maxwell relation
, Tb, Dy, Ho, Er, Tm) compounds was calculated from magnetization isotherms by using the Maxwell relation
 . The
. The
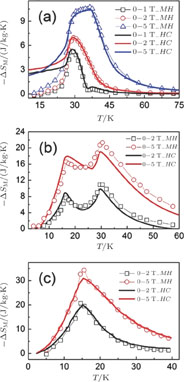
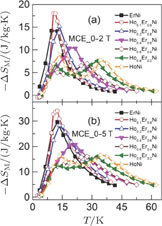
 curves calculated from isothermal magnetizations for a field change of 0 T–2 T and 0 T–5 T are presented in Figs. 14(a) and 14(b), respectively. The insets of Fig. 14 are the enlarged view of the curves. Two peaks are found to be centered at
curves calculated from isothermal magnetizations for a field change of 0 T–2 T and 0 T–5 T are presented in Figs. 14(a) and 14(b), respectively. The insets of Fig. 14 are the enlarged view of the curves. Two peaks are found to be centered at
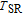 and
and
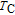 for the RGa (
for the RGa (
 , Tb, Dy, Ho, Er) compounds. The two peaks are corresponding to the SR transition and PM to FM transition. The peaks around
, Tb, Dy, Ho, Er) compounds. The two peaks are corresponding to the SR transition and PM to FM transition. The peaks around
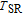 for TbGa, DyGa, HoGa, and ErGa compounds can be detected clearly in magnetic measurements. Although there are also two magnetic transitions as temperature increases for TmGa compound, only one peak can be observed on the
for TbGa, DyGa, HoGa, and ErGa compounds can be detected clearly in magnetic measurements. Although there are also two magnetic transitions as temperature increases for TmGa compound, only one peak can be observed on the
 curve. The maximal values of
curve. The maximal values of
 with a field change of 0 T–5 T for RGa (
with a field change of 0 T–5 T for RGa (
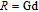 , Tb, Dy, Ho, Er, Tm) compounds are calculated to be 5.3 J/kg
, Tb, Dy, Ho, Er, Tm) compounds are calculated to be 5.3 J/kg
 K, 8.3 J/kg
K, 8.3 J/kg
 K, 5.8 J/kg
K, 5.8 J/kg
 K, 17.1 J/kg
K, 17.1 J/kg
 K, 21.3 J/kg
K, 21.3 J/kg
 K, and 34.3 J/kg
K, and 34.3 J/kg
 K, respectively. The refrigerant temperature width is calculated to be 159.4 K, 155.5 K, 112.7 K, 83 K, 30.9 K, and 14.2 K, respectively. The RC is 616.1 J/kg, 620.6 J/kg, 381.9 J/kg, 455J/kg, 494 J/kg, and 359.7 J/kg, respectively. All of the MCE parameters with a field change of 0 T–2 T and 0 T–5 T are listed in Table 1. It can be seen that for some compounds the two peaks are far from each other, and for some compounds the two peaks are close to each other. The two magnetic transitions are so near that only one peak can be seen for TmGa compound. Also it is found that the peak value around
K, respectively. The refrigerant temperature width is calculated to be 159.4 K, 155.5 K, 112.7 K, 83 K, 30.9 K, and 14.2 K, respectively. The RC is 616.1 J/kg, 620.6 J/kg, 381.9 J/kg, 455J/kg, 494 J/kg, and 359.7 J/kg, respectively. All of the MCE parameters with a field change of 0 T–2 T and 0 T–5 T are listed in Table 1. It can be seen that for some compounds the two peaks are far from each other, and for some compounds the two peaks are close to each other. The two magnetic transitions are so near that only one peak can be seen for TmGa compound. Also it is found that the peak value around
 is comparable to that around
is comparable to that around
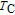 for ErGa compound. Figure 15 shows the
for ErGa compound. Figure 15 shows the
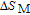 curves of all the Gd
x
Er
curves of all the Gd
x
Er
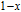 Ga (
Ga (
 ) compounds for a field change of 0 T–2 T and 0 T–5 T, respectively. Each curve has two peaks, though the peak near
) compounds for a field change of 0 T–2 T and 0 T–5 T, respectively. Each curve has two peaks, though the peak near
 is not obvious enough for GdGa. The location of the two peaks is corresponding to
is not obvious enough for GdGa. The location of the two peaks is corresponding to
 or
or
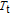 and
and
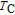 , respectively. It indicates that both SR transition and PM to FM transition contribute to MCE. As the content of Gd increases, the position of the peak near
, respectively. It indicates that both SR transition and PM to FM transition contribute to MCE. As the content of Gd increases, the position of the peak near
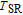 or
or
 changes not much, but the peak near
changes not much, but the peak near
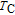 moves to higher temperature obviously. As the content of Gd increases, the value of
moves to higher temperature obviously. As the content of Gd increases, the value of
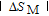 near
near
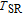 or
or
 decreases from 16.5 J/kg
decreases from 16.5 J/kg
 K to 3.0 J/kg
K to 3.0 J/kg
 K and the value of
K and the value of
 near
near
 decreases from 21.3 J/kg
decreases from 21.3 J/kg
 K to 5.3 J/kg
K to 5.3 J/kg
 K. Oesterreicher and Parker[47] have proposed the relationship between
K. Oesterreicher and Parker[47] have proposed the relationship between
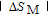 near
near
 and other magnetic parameters as follows:
and other magnetic parameters as follows:
 . Where R is universal gas constant,
. Where R is universal gas constant,
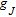 is Lande factor,
is Lande factor,

 is Bohr magneton, J is the total angular quantum number, H is external magnetic field,
is Bohr magneton, J is the total angular quantum number, H is external magnetic field,
 is Boltzmann constant, and
is Boltzmann constant, and
 is Curie temperature. For Gd
x
Er
is Curie temperature. For Gd
x
Er
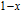 Ga (
Ga (
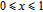 ) compounds, the value of J decreases and the value of
) compounds, the value of J decreases and the value of
 increases with the content of Gd increasing, so the
increases with the content of Gd increasing, so the
 near
near
 shows downtrend. For Gd
shows downtrend. For Gd
 Er
Er
 Ga and Gd
Ga and Gd
 Er
Er
 Ga, the value of
Ga, the value of
 is positive below
is positive below
 (inset of Fig. 15), and that is because the magnetic ground state in low temperature zone is AFM. And positive
(inset of Fig. 15), and that is because the magnetic ground state in low temperature zone is AFM. And positive
 is always observed in that case, which is due to the disordered magnetic sub-lattice antiparallel to the applied magnetic field.[48,49]
is always observed in that case, which is due to the disordered magnetic sub-lattice antiparallel to the applied magnetic field.[48,49]
The value of
 can be calculated from magnetization data according to the Maxwell relation and from heat capacity data. The
can be calculated from magnetization data according to the Maxwell relation and from heat capacity data. The
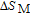 –T curves for PrGa, ErGa, and TmGa compounds obtained from the two methods are shown in Fig. 16(a), Fig. 16(b), and Fig. 16(c), respectively. Before we give the details of calculating the
–T curves for PrGa, ErGa, and TmGa compounds obtained from the two methods are shown in Fig. 16(a), Fig. 16(b), and Fig. 16(c), respectively. Before we give the details of calculating the
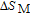 of PrGa compound from heat capacity data, we need to review the standard methods to extract
of PrGa compound from heat capacity data, we need to review the standard methods to extract
 from C
H
data. Take the
from C
H
data. Take the
 for example, we need three steps to achieve the result:[48]
for example, we need three steps to achieve the result:[48]
The first step is to obtain the total entropy at zero-field
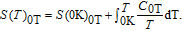 | (7) |
Second step is to have the total entropy at

T
 | (8) |
The last step is to make the difference, that is,

on the assumption that
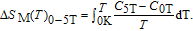 | (9) |
However, heat capacity needs be measured at extremely low temperature, so now the question is whether we may use the above standard method. Considering the fact that the starting temperature of heat capacity measurement for PrGa compound is 10 K and the magnetic structure is complex in low temperature range, the standard method to calculate
 from C
H
data will not hold true in this case anymore. In fact, the problem can be solved as long as we make some modifications to this method. The key point of modification is to replace the reference temperature of 0 K with 72.5 K.[41] In this case, the value of is not zero, but we can acquire its value from the calculations of M–H data. The reason why we choose 72.5 K as the reference temperature is that the magnetic ground state and magnetic transition are clearly known here far from
from C
H
data will not hold true in this case anymore. In fact, the problem can be solved as long as we make some modifications to this method. The key point of modification is to replace the reference temperature of 0 K with 72.5 K.[41] In this case, the value of is not zero, but we can acquire its value from the calculations of M–H data. The reason why we choose 72.5 K as the reference temperature is that the magnetic ground state and magnetic transition are clearly known here far from
 . And we can see that the curves obtained from the two methods are consistent well with each other above 25 K. Large deviation below 25 K may result from the complex magnetic structure and magnetic transition in PrGa compound.[41] The temperature dependences of
. And we can see that the curves obtained from the two methods are consistent well with each other above 25 K. Large deviation below 25 K may result from the complex magnetic structure and magnetic transition in PrGa compound.[41] The temperature dependences of
 for ErGa and TmGa compounds calculated from magnetizations and heat capacity data for field changes of 0–2 and 0 T–5 T are shown in Fig. 16(b) and Fig. 16(c), respectively. The results obtained by using the two techniques are in good agreement with each other.
for ErGa and TmGa compounds calculated from magnetizations and heat capacity data for field changes of 0–2 and 0 T–5 T are shown in Fig. 16(b) and Fig. 16(c), respectively. The results obtained by using the two techniques are in good agreement with each other.
As another important parameter to evaluate the MCE of magnetocaloric materials, the adiabatic temperature change
 was calculated from the C
P
versus T curves by using the method suggested by Pecharsky and Gschneidner,[50] and it is summarized below. Firstly,
was calculated from the C
P
versus T curves by using the method suggested by Pecharsky and Gschneidner,[50] and it is summarized below. Firstly,
 is obtained according to formula (7) and S(72.5 K)
is obtained according to formula (7) and S(72.5 K)
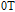 is set to be zero. Secondly,
is set to be zero. Secondly,
 is obtained from
is obtained from
 and
and
 , which is calculated from M–H data. Thirdly, we can obtain the curves of
, which is calculated from M–H data. Thirdly, we can obtain the curves of
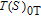 and
and
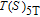 , which are the inverse functions of
, which are the inverse functions of
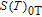 and
and
 respectively. Finally, we make a difference according to the formula
respectively. Finally, we make a difference according to the formula
 –
–
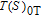 and then the
and then the
 –T curves are obtained.
–T curves are obtained.
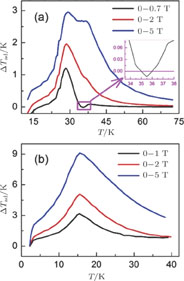
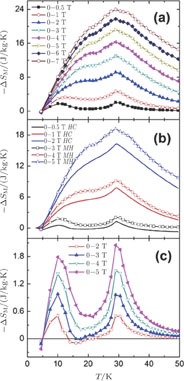
The
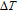 curves of PrGa compound for a field change of 0 T–0.7 T, 0 T–2 T, and 0 T–5 T are shown in Fig. 17(a) and the inset is the enlarge view of the curves. As two most important parameters to characterize MCE,
curves of PrGa compound for a field change of 0 T–0.7 T, 0 T–2 T, and 0 T–5 T are shown in Fig. 17(a) and the inset is the enlarge view of the curves. As two most important parameters to characterize MCE,
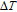 and
and
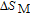 curves show similar change rules. When the magnetic field change is small such as 0.7 T, two peaks are observed on the curves near
curves show similar change rules. When the magnetic field change is small such as 0.7 T, two peaks are observed on the curves near
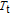 and
and
 respectively. That is to say, both the FM to FM transition and the PM to FM transition contribute to MCE. We can also see that the peak near
respectively. That is to say, both the FM to FM transition and the PM to FM transition contribute to MCE. We can also see that the peak near
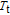 is much higher than that near
is much higher than that near
 . That is because the FM to FM transition is of first order and accompanied by volume transition, which always plays an important role in enhancing MCE.[51,52] With the field change increasing, the peak in lower temperature zone shifts towards higher temperature region. That is because the temperature zone where the FM to FM transition occurs extends. When the field change is high enough such as 5 T, the two peaks are merged together and a wide platform is observed on the curve from 29.5 K to 37.5 K. This kind of material with nearly constant
. That is because the FM to FM transition is of first order and accompanied by volume transition, which always plays an important role in enhancing MCE.[51,52] With the field change increasing, the peak in lower temperature zone shifts towards higher temperature region. That is because the temperature zone where the FM to FM transition occurs extends. When the field change is high enough such as 5 T, the two peaks are merged together and a wide platform is observed on the curve from 29.5 K to 37.5 K. This kind of material with nearly constant
 and
and
 in a wide temperature range has many practical applications, because people can use as few materials as possible in the limited space of magnetic refrigerator to realize refrigeration. The magnetic property and MCE of PrGa are very similar to those of LaFe
in a wide temperature range has many practical applications, because people can use as few materials as possible in the limited space of magnetic refrigerator to realize refrigeration. The magnetic property and MCE of PrGa are very similar to those of LaFe
 Al
Al
 .[44] Some other RGa compounds have the same crystal structures as PrGa compounds, but no platforms are found on their
.[44] Some other RGa compounds have the same crystal structures as PrGa compounds, but no platforms are found on their
 –T curves.[19,29,32] The main difference is that the transition temperature does not shift toward higher zone obviously with applied field increasing for other RGa compounds. Though the maximum value of
–T curves.[19,29,32] The main difference is that the transition temperature does not shift toward higher zone obviously with applied field increasing for other RGa compounds. Though the maximum value of
 and
and
 for PrGa is not large enough ((
for PrGa is not large enough ((
 is 10.7 J/kg
is 10.7 J/kg
 K and (
K and (
 is 2.9 K for a field change of 5 T), the feature of nearly constant
is 2.9 K for a field change of 5 T), the feature of nearly constant
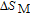 and
and
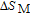 is valuable for researches compared with other RGa compounds.[41] Anyway, the future work is still necessary to improve the maximum value of
is valuable for researches compared with other RGa compounds.[41] Anyway, the future work is still necessary to improve the maximum value of
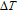 and keep the platform on the curves at the same time. Positive
and keep the platform on the curves at the same time. Positive
 value and negative
value and negative
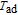 value are observed around 35.5 K for the field changes of 0.7 T, and similar results have been reported more than once.[48,49] The maximum values of
value are observed around 35.5 K for the field changes of 0.7 T, and similar results have been reported more than once.[48,49] The maximum values of
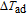 for TmGa compound are 3.2, 5, and 9.1 K for the field change of 1, 2, and 5 T, respectively (Fig. 17(b)). The MCE of TmGa, especially under the low magnetic field change, is comparable with or even larger than those of other magnetocaloric materials around the liquid hydrogen temperature. Therefore, TmGa compound appears to be a very attractive candidate material for use in a magnetic refrigerator working in low temperature.
for TmGa compound are 3.2, 5, and 9.1 K for the field change of 1, 2, and 5 T, respectively (Fig. 17(b)). The MCE of TmGa, especially under the low magnetic field change, is comparable with or even larger than those of other magnetocaloric materials around the liquid hydrogen temperature. Therefore, TmGa compound appears to be a very attractive candidate material for use in a magnetic refrigerator working in low temperature.
To evaluate the applicability of RGa compounds as magnetic refrigerant materials in a reasonable way, we have estimated the RC by using the approach suggested by Gschneidner et al.[53] The RC is defined as
 , where T
1 and T
2 are the temperatures corresponding to both sides of the half maximum value of
, where T
1 and T
2 are the temperatures corresponding to both sides of the half maximum value of
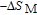 peak, respectively. Calculations show that the maximal value of RC is 158.7, 616.1, 620.6, 381.9, 455, 494, 359.7 J/kg with a magnetic field change of 0 T–5 T for RGa (
peak, respectively. Calculations show that the maximal value of RC is 158.7, 616.1, 620.6, 381.9, 455, 494, 359.7 J/kg with a magnetic field change of 0 T–5 T for RGa (
 , Gd, Tb, Dy, Ho, Er, Tm) compounds, respectively. Especially, the value of RC for TbGa is found to be 900 J/kg with
, Gd, Tb, Dy, Ho, Er, Tm) compounds, respectively. Especially, the value of RC for TbGa is found to be 900 J/kg with
 K (temperature of the cold end) and
K (temperature of the cold end) and
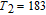 K (temperature of the hot end) for a field change of 0 T–7 T.[32] Although the TbGa compound has a moderate
K (temperature of the hot end) for a field change of 0 T–7 T.[32] Although the TbGa compound has a moderate
 , its RC value is much larger than those of other magnetic refrigerant materials in a wide temperature range. The large RC of TbGa originates from the combined contribution from SR and FM–PM transitions, which enlarges the temperature span of the MCE. It is noted that the value of
, its RC value is much larger than those of other magnetic refrigerant materials in a wide temperature range. The large RC of TbGa originates from the combined contribution from SR and FM–PM transitions, which enlarges the temperature span of the MCE. It is noted that the value of
 for RGa increases monotonically with the decrease of
for RGa increases monotonically with the decrease of
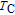 as the R atomic number increases. The results indicate that the working temperature and temperature span of the MCE could be tuned by the substitution between different R elements. All the magnetic parameters and MCE results of Gd
x
Er
as the R atomic number increases. The results indicate that the working temperature and temperature span of the MCE could be tuned by the substitution between different R elements. All the magnetic parameters and MCE results of Gd
x
Er
 Ga (
Ga (
 ) compounds are also listed in Table 1. We can see that though the maximum value of
) compounds are also listed in Table 1. We can see that though the maximum value of
 decreases with the content of Gd increasing, the value of RC is greatly improved compared with ErGa. Also we find that the
decreases with the content of Gd increasing, the value of RC is greatly improved compared with ErGa. Also we find that the
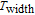 greatly extends as the content of Gd increases. Since both transitions (PM to FM transition and SR transition) contribute to MCE, there is a positive correlation between
greatly extends as the content of Gd increases. Since both transitions (PM to FM transition and SR transition) contribute to MCE, there is a positive correlation between
 –
–
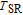 and T
2–T
1. According to the discussions on transition temperatures,
and T
2–T
1. According to the discussions on transition temperatures,
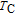 increases much faster than
increases much faster than
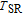 with Gd-content increasing; therefore, the increasing of Gd-content enhances
with Gd-content increasing; therefore, the increasing of Gd-content enhances
 . And the extending of
. And the extending of
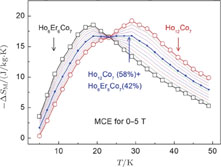
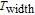 plays an important role in improving the value of RC according to the calculation method. The exact value of RC fluctuates with the content of Gd increasing, but Gd
plays an important role in improving the value of RC according to the calculation method. The exact value of RC fluctuates with the content of Gd increasing, but Gd
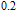 Er
Er
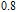 Ga and Gd
Ga and Gd
 Er
Er
 Ga compounds show better performances than others in this series by considering both
Ga compounds show better performances than others in this series by considering both
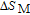 and RC. Another important result is that table-like
and RC. Another important result is that table-like
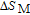 curves are observed for certain compounds, such as those in the case of
curves are observed for certain compounds, such as those in the case of
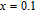 , 0.2, 0.3, and 0.4. This kind of
, 0.2, 0.3, and 0.4. This kind of
 curves has been observed several times and such a feature of
curves has been observed several times and such a feature of
 curves is very useful for practical applications of refrigeration.[54,55]
curves is very useful for practical applications of refrigeration.[54,55]
2.4. The physical mechanism of magnetic field controlled magnetocaloric effect and magnetoresistance in PrGa compoundAs we mentioned before, the isothermal magnetization curves showed a typical AFM-like characteristics in the temperature range between
 and
and
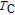 . However, neutron diffraction results show that PrGa compound is an FM-ordered state in this temperature zone. We take the representative isothermal magnetization curve at 32 K to reinterpret the magnetizing process in PrGa compound. The isothermal magnetization curve at 32 K is quoted and the magnetic structure sketches in different field zones have been plotted in Fig. 18.
. However, neutron diffraction results show that PrGa compound is an FM-ordered state in this temperature zone. We take the representative isothermal magnetization curve at 32 K to reinterpret the magnetizing process in PrGa compound. The isothermal magnetization curve at 32 K is quoted and the magnetic structure sketches in different field zones have been plotted in Fig. 18.
The magnetizing process can be divided into two stages by the critical field (
 , which is determined by the maximum of
, which is determined by the maximum of
 .[33] For PrGa compound,
.[33] For PrGa compound,
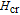 is the critical field where the metamagnetic transition begins and
is the critical field where the metamagnetic transition begins and
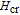 is calculated to be 0.6 T at 32 K. In the range of
is calculated to be 0.6 T at 32 K. In the range of
 , there are many magnetic domains with different FM-ordered directions, so the macroscopic magnetization is zero at the beginning. The magnetization increases gradually with increasing magnetic field. In this stage, magnetizing process is mainly achieved by means of domain-wall displacement. Inside of every magnetic domain, the projection of every magnetic moment on z axis does not really change. Since the ordered magnetic moment is rather small at 32 K (see Fig. 13), the magnetization changes slowly in this stage. In the range of
, there are many magnetic domains with different FM-ordered directions, so the macroscopic magnetization is zero at the beginning. The magnetization increases gradually with increasing magnetic field. In this stage, magnetizing process is mainly achieved by means of domain-wall displacement. Inside of every magnetic domain, the projection of every magnetic moment on z axis does not really change. Since the ordered magnetic moment is rather small at 32 K (see Fig. 13), the magnetization changes slowly in this stage. In the range of
 , the domain-wall displacement reaches its limits. As magnetic field continues to increase, the magnetizing process is achieved by means of magnetic moment rotation. That is, the direction of every magnetic moment tends to align with z axis gradually. The most remarkable difference between the above two stages is that the magnetizing process is irrelevant to the degree of magnetic order in the first stage, but it is closely related in the second stage.
, the domain-wall displacement reaches its limits. As magnetic field continues to increase, the magnetizing process is achieved by means of magnetic moment rotation. That is, the direction of every magnetic moment tends to align with z axis gradually. The most remarkable difference between the above two stages is that the magnetizing process is irrelevant to the degree of magnetic order in the first stage, but it is closely related in the second stage.
The
 curves calculated from the Maxwell relation of PrGa compound with a field change of 0 T–0.3 T and 0 T–5 T are shown Fig. 19, respectively. Figure 19 also shows that only for a large field change can we observe the plateau. When
curves calculated from the Maxwell relation of PrGa compound with a field change of 0 T–0.3 T and 0 T–5 T are shown Fig. 19, respectively. Figure 19 also shows that only for a large field change can we observe the plateau. When
 or
or
 , the value of
, the value of
 is relatively small no matter the field change is large or small. The temperature range between
is relatively small no matter the field change is large or small. The temperature range between
 and
and
 is the most important zone for the formation of the plateau. Again we take 32 K data to interpret the cause of the plateau.[33] When the field change is 0 T–0.3 T, the magnetizing process is in the first stage. As we have mentioned before, magnetizing process is achieved by domain-wall displacement, and
is the most important zone for the formation of the plateau. Again we take 32 K data to interpret the cause of the plateau.[33] When the field change is 0 T–0.3 T, the magnetizing process is in the first stage. As we have mentioned before, magnetizing process is achieved by domain-wall displacement, and
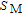 hardly changes in this stage. The value of
hardly changes in this stage. The value of
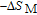 is almost zero and no plateau is observed for a field change of 0 T–0.3 T. When the magnetic field exceeds
is almost zero and no plateau is observed for a field change of 0 T–0.3 T. When the magnetic field exceeds
 , the magnetizing process steps into the second stage and all the magnetic moments begin to rotate toward z axis.
, the magnetizing process steps into the second stage and all the magnetic moments begin to rotate toward z axis.
 changes significantly and thus a large
changes significantly and thus a large
 value is observed for a field change of 0 T–5 T. It can be seen that the formation of a plateau is closely related to the magnetic structure, magnetic transition, and magnetizing process in PrGa compound.
value is observed for a field change of 0 T–5 T. It can be seen that the formation of a plateau is closely related to the magnetic structure, magnetic transition, and magnetizing process in PrGa compound.
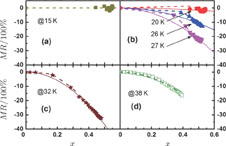
The magnetic field dependence of MR at various temperatures has been measured and calculated (shown in Fig. 20). It is observed that the MR drops significantly with the increase of field when the magnetic field smaller than
 T around
T around
 , then retains a large value with the further increase of field. It is worth noting that the MR value is nearly as large as 30% at 28 K when a relatively small field (1 T) is applied, which is beneficial for the potential technology applications of the present sample. For the PrGa compound, the values of MR are found to be
, then retains a large value with the further increase of field. It is worth noting that the MR value is nearly as large as 30% at 28 K when a relatively small field (1 T) is applied, which is beneficial for the potential technology applications of the present sample. For the PrGa compound, the values of MR are found to be
 % and
% and
 % at 28 K and 30 K in a magnetic field of 5 T, respectively. The maximal MR in PrGa is comparable to or much larger than those of some best known R-based intermetallic compounds, such as SmMn2Ge2 (8%),[56] Ce(FeRu)2 (20%),[57] Gd2In (29%),[58] Gd5(Si2Ge
% at 28 K and 30 K in a magnetic field of 5 T, respectively. The maximal MR in PrGa is comparable to or much larger than those of some best known R-based intermetallic compounds, such as SmMn2Ge2 (8%),[56] Ce(FeRu)2 (20%),[57] Gd2In (29%),[58] Gd5(Si2Ge
 (26%),[59] and Gd5(Si
(26%),[59] and Gd5(Si
 Ge
Ge
 (20%).[60] Theoretical study based on the independent scattering mechanism shows that in metallic systems, the difference in resistivity owing to the magnetic structure modification. The electrical resistivity in magnetic materials includes residual resistivity (ρ
(20%).[60] Theoretical study based on the independent scattering mechanism shows that in metallic systems, the difference in resistivity owing to the magnetic structure modification. The electrical resistivity in magnetic materials includes residual resistivity (ρ
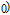 , resistivity due to electron–electron scattering (ρ
, resistivity due to electron–electron scattering (ρ
 , resistivity due to electron–phonon scattering (ρ
, resistivity due to electron–phonon scattering (ρ
 and resistivity due to the electron–magnon interactions (ρ
and resistivity due to the electron–magnon interactions (ρ
 .[59] Among them, magnetic field mainly affects the ρ
.[59] Among them, magnetic field mainly affects the ρ
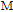 term. ρ
term. ρ
 increases with increasing
increases with increasing
 and reaches to its maximum value when the temperature exceeds
and reaches to its maximum value when the temperature exceeds
 .[61] Since the FM-ordered magnetic component is along z axis, spin polarization of electrons exists. The component in the xy crystal plane is in the disordered state, therefore the electron motion within the xy plane is almost forbidden. The only motion path of electrons is thus along z axis, and the obstacle of the movement mainly comes from the disordered M
xy
. Therefore, we assume that the resistance caused by the magnetic moments is proportional to the projected area of the circular cone in the xy crystal plane. The above assumption can be expressed in the following formula:[33]
.[61] Since the FM-ordered magnetic component is along z axis, spin polarization of electrons exists. The component in the xy crystal plane is in the disordered state, therefore the electron motion within the xy plane is almost forbidden. The only motion path of electrons is thus along z axis, and the obstacle of the movement mainly comes from the disordered M
xy
. Therefore, we assume that the resistance caused by the magnetic moments is proportional to the projected area of the circular cone in the xy crystal plane. The above assumption can be expressed in the following formula:[33]
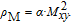 | (10) |
Coefficient
α is a constant.
Since MR mainly comes from magnetic contribution in PrGa compound, at the fixed temperature of T, the change of electrical resistivity with different magnetic fields can be written as follows:
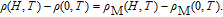 | (11) |
At zero-field, the non-magnetic part of resistivity can be written as the multiple of the magnetic part, and then the total resistivity at zero-field can be written as follows:
 | (12) |
where
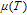
is a constant related to temperature.
MR is usually written in the following formula:
[61,62]
 | (13) |
Put Eq. (
11) and (Eq. (
12) into Eq. (
13), we can get a new form of MR:
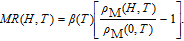 | (14) |
It should be noted that
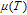
has been replaced by

on the basis of

. Considering Eq. (
6) and Eq. (
9), we can get the following expression:
[33]
 | (15) |
Then it is the key question to determine
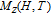 and
and
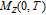 .
.
 is the FM-ordered component of magnetic moments at zero magnetic field and it cannot be detected from the macroscopic magnetic measurement because of the existence of magnetic domains. While
is the FM-ordered component of magnetic moments at zero magnetic field and it cannot be detected from the macroscopic magnetic measurement because of the existence of magnetic domains. While
 can be determined accurately by NPD results at zero field. The deviation of
can be determined accurately by NPD results at zero field. The deviation of
 obtained from bulk compound and powder sample has been discussed. In order to study MR in bulk PrGa compound, we revised the
obtained from bulk compound and powder sample has been discussed. In order to study MR in bulk PrGa compound, we revised the
 obtained from NPD experiment by taking M
z
(0, 5 K) as a reference. The expression of
obtained from NPD experiment by taking M
z
(0, 5 K) as a reference. The expression of
 is very complex, because it is not only temperature-dependent but also field-dependent. The whole temperature range is divided into three parts by
is very complex, because it is not only temperature-dependent but also field-dependent. The whole temperature range is divided into three parts by
 K and T= 38 K:[33]
K and T= 38 K:[33]
Case 1
 K
K
The magnetizing process is generally realized by domain-wall displacement and magnetic moment rotation sequentially as magnetic field increases.
 T,
T,
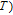 is a macroscopic physical quantity, and if
is a macroscopic physical quantity, and if
 T,
T,
 is no more than
is no more than
 , we can conclude that the magnetizing process is only in the first stage and M
z
does not really change even though the magnetic field is as high as 5 T. There is almost no difference between
, we can conclude that the magnetizing process is only in the first stage and M
z
does not really change even though the magnetic field is as high as 5 T. There is almost no difference between
 and
and
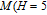 T,
T,
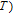 in this temperature range. So, we have the following expression:
in this temperature range. So, we have the following expression:
 | (16) |
Cases 2 17 K
 K
K
In this temperature range,
 T,
T,
 is considerably larger than
is considerably larger than
 , which indicates that the magnetizing process experiences both stages as magnetic field increases from 0 T to 5 T. Also it should be noted that
, which indicates that the magnetizing process experiences both stages as magnetic field increases from 0 T to 5 T. Also it should be noted that
 is obtained in this temperature range. As we discussed on the magnetizing process at 32 K,
is obtained in this temperature range. As we discussed on the magnetizing process at 32 K,
 is the field separatrix of the two magnetizing stages. And
is the field separatrix of the two magnetizing stages. And
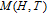 can be considered as the measure of
can be considered as the measure of
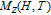 , when the magnetic field exceeds
, when the magnetic field exceeds
 . So
. So
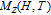 can be expressed in the following piecewise function:
can be expressed in the following piecewise function:
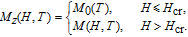 | (17) |
Case 3
 K
K
In this temperature range, PrGa compound is in the PM state and every magnetic moment distributes randomly at zero field. When the magnetic field is applied, the macroscopic magnetization
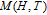 is the ordered component of the magnetic moment along z axis. So, we have the following expression:
is the ordered component of the magnetic moment along z axis. So, we have the following expression:
 | (18) |
Putting Eqs. (16)–(18) into Eq. (15), we can obtain
 . In order to simplify the expression of MR, we make the following variable transformation:
. In order to simplify the expression of MR, we make the following variable transformation:
 | (19) |
 | (20) |
 | (21) |
Then,
MR can be expressed below:
Case 1)
 K
K
 | (22) |
Case 2) 17 K
 K
K
 | (23) |
Case 3)
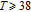 K
K
 | (24) |
On one hand,
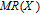 can be calculated according to Eq. (22)–(24). On the other hand, the experimental results of
can be calculated according to Eq. (22)–(24). On the other hand, the experimental results of
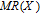 can be calculated from
can be calculated from
 and
and
 . The curves for X dependence of MR at
. The curves for X dependence of MR at
 K, 20 K, 26 K, 27 K, 32 K, and 38 K were plotted in Fig. 21. The dotted lines are the calculated results and the scattered symbols are the experimental results. The experimental results are in good accordance with the calculated results in all three temperature zones. This indicates that the assumption about
K, 20 K, 26 K, 27 K, 32 K, and 38 K were plotted in Fig. 21. The dotted lines are the calculated results and the scattered symbols are the experimental results. The experimental results are in good accordance with the calculated results in all three temperature zones. This indicates that the assumption about
 expressed in Eq. (10) and the calculation of MR are reasonable. And also we can see that MR is indeed closely related magnetic structure, magnetic transition and magnetizing process in PrGa compound.
expressed in Eq. (10) and the calculation of MR are reasonable. And also we can see that MR is indeed closely related magnetic structure, magnetic transition and magnetizing process in PrGa compound.
Many works on MR in granular system have been reported, and it indicates that MR and X have the quadratic relationship
 in granular materials.[63–65] So it is necessary to discuss whether the quadratic relationship is still appropriate in bulk PrGa compound. From Eqs. (22)–(24) and Fig. 21, it is clear that the MR for
in granular materials.[63–65] So it is necessary to discuss whether the quadratic relationship is still appropriate in bulk PrGa compound. From Eqs. (22)–(24) and Fig. 21, it is clear that the MR for
 K does not obey the quadratic relationship and the result for
K does not obey the quadratic relationship and the result for
 K matches the quadratic relationship strictly. The relationship of MR and X has more complex form in the temperature range between 17 K and 38 K according to Eq. (23). The MR results at
K matches the quadratic relationship strictly. The relationship of MR and X has more complex form in the temperature range between 17 K and 38 K according to Eq. (23). The MR results at
 K, 26 K, 27 K, and 32 K shown in Fig. 21(b) and Fig. 21(c) are in this temperature range. To make it easy to compare, the function of
K, 26 K, 27 K, and 32 K shown in Fig. 21(b) and Fig. 21(c) are in this temperature range. To make it easy to compare, the function of
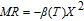 is also plotted in Fig. 21(b) and Fig. 21(c) in solid line. It shows that the experimental result for
is also plotted in Fig. 21(b) and Fig. 21(c) in solid line. It shows that the experimental result for
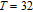 K can be considered to obey the quadratic relationship approximately. However, the experimental results for
K can be considered to obey the quadratic relationship approximately. However, the experimental results for
 K, 26 K, and 27 K show considerable deviations from the
K, 26 K, and 27 K show considerable deviations from the
 relation. In order to find out why the approximation is effective in Fig. 21(c) and why the approximation is ineffective in Fig. 21(b), we need to review the derivation formula of MR in the temperature range between 17 K and 38 K.[33] According to the second part of Eq. (23), if
relation. In order to find out why the approximation is effective in Fig. 21(c) and why the approximation is ineffective in Fig. 21(b), we need to review the derivation formula of MR in the temperature range between 17 K and 38 K.[33] According to the second part of Eq. (23), if
 can be approximated as zero, MR can be expressed as
can be approximated as zero, MR can be expressed as
 . It shows that M
0(20 K), M
0(26 K), and M
0(27 K) are 1.7
. It shows that M
0(20 K), M
0(26 K), and M
0(27 K) are 1.7
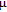
 , 1.3
, 1.3
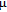
 , and 1.0
, and 1.0

 , respectively, while M
0(32 K) is only 0.5
, respectively, while M
0(32 K) is only 0.5

 which is relatively smaller than each of the above three. As an approximation, we treat M
0(32 K) as zero, and then obtain the quadratic relationship in the second part of Eq. (23). Further, if the
which is relatively smaller than each of the above three. As an approximation, we treat M
0(32 K) as zero, and then obtain the quadratic relationship in the second part of Eq. (23). Further, if the
 at 32 K is neglected, MR(32 K) can be expressed as
at 32 K is neglected, MR(32 K) can be expressed as
 approximately. However,
approximately. However,
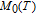 at 20 K, 26 K, and 27 K is relatively large and it cannot be approximated as zero. Therefore, MR curves deviate noticeably from the quadratic relationship shown in Fig. 21(b).
at 20 K, 26 K, and 27 K is relatively large and it cannot be approximated as zero. Therefore, MR curves deviate noticeably from the quadratic relationship shown in Fig. 21(b).
3.
 Ni compounds
Ni compoundsThe binary RNi (
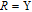 , Ce, Pr, Nd, Sm, Gd, Tb, Dy, Ho, Er, Tm) compounds have been fabricated and studied.[66] Results show that DyNi, HoNi, ErNi, TmNi, and YNi compounds crystallize in the FeB structure. But CeNi, PrNi, NdNi, SmNi, GdNi, and TbNi form in the CrB structure. YNi and CeNi compounds exhibit only Pauli paramagnetism according to magnetic measurement above 4.2 K. Other RNi compounds are all magnetic ordered. The Curie temperature is determined to be 22 K, 28 K, 45 K, 71 K, 52 K, 62 K, 37 K, 13 K, and 8 K for PrNi, NdNi, SmNi, GdNi, TbNi, DyNi, HoNi, ErNi, and TmNi, respectively. Nickel appears to be nonmagnetic in the RNi compounds and Curie–Weiss behavior is obeyed in the paramagnetic region for the RNi compounds except TmNi. The saturation moments obtained by extrapolating the measured magnetization to infinite field are less than that of free R ion moment for the RNi compounds except GdNi. This disagreement may be attributed to partial quenching of the orbital angular momentum of the R ion by the crystalline field generated by the surrounding ions although the possibility cannot be excluded that the low moments are merely due to strong magnetic anisotropy. A secondary magnetic transition is observed below Curie temperature for NdNi and HoNi compounds. And little short range exists past the magnetic transition for the RNi compounds.[66]
, Ce, Pr, Nd, Sm, Gd, Tb, Dy, Ho, Er, Tm) compounds have been fabricated and studied.[66] Results show that DyNi, HoNi, ErNi, TmNi, and YNi compounds crystallize in the FeB structure. But CeNi, PrNi, NdNi, SmNi, GdNi, and TbNi form in the CrB structure. YNi and CeNi compounds exhibit only Pauli paramagnetism according to magnetic measurement above 4.2 K. Other RNi compounds are all magnetic ordered. The Curie temperature is determined to be 22 K, 28 K, 45 K, 71 K, 52 K, 62 K, 37 K, 13 K, and 8 K for PrNi, NdNi, SmNi, GdNi, TbNi, DyNi, HoNi, ErNi, and TmNi, respectively. Nickel appears to be nonmagnetic in the RNi compounds and Curie–Weiss behavior is obeyed in the paramagnetic region for the RNi compounds except TmNi. The saturation moments obtained by extrapolating the measured magnetization to infinite field are less than that of free R ion moment for the RNi compounds except GdNi. This disagreement may be attributed to partial quenching of the orbital angular momentum of the R ion by the crystalline field generated by the surrounding ions although the possibility cannot be excluded that the low moments are merely due to strong magnetic anisotropy. A secondary magnetic transition is observed below Curie temperature for NdNi and HoNi compounds. And little short range exists past the magnetic transition for the RNi compounds.[66]
Neutron diffraction experiment was employed to study the magnetic structure and magnetic transition of single crystal HoNi compound.[67] The magnetic (102) and (201) peaks increase rapidly with decreasing temperature below 35 K, which indicates that there is a magnetic-disorder to magnetic-order transition around 35 K. The intensity of the (100) line is zero at 78 K and very strong at 20 and 4.2 K, indicating that there is a AFM z component exists at the temperature range. The intensity of (200) line increases rapidly below 15 K with decreasing temperature, indicating that there is a formation of y component around 15 K. The results of neutron diffraction confirmed the two magnetic transitions around 15 K and 35 K of HoNi compound. The magnetocaloric effect of PrNi compound has also been studied.[68] Powder x-ray diffraction shows that PrNi compound crystallizes in CrB-type orthorhombic structure. The heat capacity of PrNi was measured at the field of 0 T, 2 T, 5 T, 7.5 T, and 10 T, respectively. PrNi undergoes a second-order phase transition from an FM to PM state as temperature increases. The Curie temperature is determined to be 19 K according to the zero-field heat capacity data. The maximum MCE for PrNi is 4.2 K, which occurs at 19 K for a field change from 0 T to 10 T.[68] The magnetic structure of DyNi compound has also been studied by neutron diffraction technique.[69] From the magnetization measurements at 1.6 K, DyNi compound exhibits narrow domain walls frozen by the anisotropy.
The Pt–Ni substitution on the crystal structure and magnetic behavior of the PrNi
 Pt
x
pseudo-binary compounds has also been studied.[70] The orthorhombic CrB-type structure possessed by PrNi was found to remain conserved for Pt doped compounds. The unit cell volume (and consequently the interatomic distances) linearly increases with increasing Pt concentration. The MCE was determined consistently from the specific heat and magnetization data. It has the same value in the boundary compounds PrNi and PrPt.[70] The effect of hydrogenation on the structure and magnetic properties of ErNi has also been investigated.[71] Results show that ErNi compound can form crystalline hydrides which remain in the structure of the original compound. There is little change in lattice parameter and magnetic properties of the crystalline hydrides to compare with original compound.[71] The drastic change of the magnetization due to the spin flopping was observed for both single crystal ErNi and DyNi compounds.[72] It has been confirmed that Pr–Pr and Gd–Gd exchange interactions are ferromagnetic, but Pr–Gd exchange interactions are antiferromagnetic in single crystal Pr
Pt
x
pseudo-binary compounds has also been studied.[70] The orthorhombic CrB-type structure possessed by PrNi was found to remain conserved for Pt doped compounds. The unit cell volume (and consequently the interatomic distances) linearly increases with increasing Pt concentration. The MCE was determined consistently from the specific heat and magnetization data. It has the same value in the boundary compounds PrNi and PrPt.[70] The effect of hydrogenation on the structure and magnetic properties of ErNi has also been investigated.[71] Results show that ErNi compound can form crystalline hydrides which remain in the structure of the original compound. There is little change in lattice parameter and magnetic properties of the crystalline hydrides to compare with original compound.[71] The drastic change of the magnetization due to the spin flopping was observed for both single crystal ErNi and DyNi compounds.[72] It has been confirmed that Pr–Pr and Gd–Gd exchange interactions are ferromagnetic, but Pr–Gd exchange interactions are antiferromagnetic in single crystal Pr
 Gd
x
Ni compounds.[73] And the case is similar to the Nd
Gd
x
Ni compounds.[73] And the case is similar to the Nd
 Gd
x
Ni and Nd
Gd
x
Ni and Nd
 Tb
x
Ni system.[74,75]
Tb
x
Ni system.[74,75]
In this section, we will review the magnetic properties and magnetocaloric effect of binary RNi (
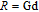 , Dy, Ho, Er) compounds and pseudo-binary (Ho,Er)Ni compounds. The data for GdNi and DyNi is cited from the work of Suresh et al.[77,78] The data for HoNi and ErNi is newly obtained in this work and at the same time we noted that some magnetic measurement has been carried out by Suresh et al.[77,78] The data for (Ho,Er)Ni compounds are all newly obtained.[76] The main research content is magnetic transition, magnetic structure, magnetocaloric effect and the main research method is magnetic measurement and powder neutron diffraction experiment.
, Dy, Ho, Er) compounds and pseudo-binary (Ho,Er)Ni compounds. The data for GdNi and DyNi is cited from the work of Suresh et al.[77,78] The data for HoNi and ErNi is newly obtained in this work and at the same time we noted that some magnetic measurement has been carried out by Suresh et al.[77,78] The data for (Ho,Er)Ni compounds are all newly obtained.[76] The main research content is magnetic transition, magnetic structure, magnetocaloric effect and the main research method is magnetic measurement and powder neutron diffraction experiment.
3.1. The crystal structure and basic magnetic properties of
 Ni (
Ni (
 , Dy, Ho, Er) compounds and Ho
, Dy, Ho, Er) compounds and Ho
 Er
Er
 Ni compounds
Ni compoundsBinary RNi (
 , Dy, Ho, Er) compounds and pseudo-binary Ho
x
Er
, Dy, Ho, Er) compounds and pseudo-binary Ho
x
Er
 Ni (
Ni (
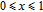 ) compounds were fabricated and powder x-ray diffraction was carried out to confirm the phase purity. The XRD patterns of Ho
x
Er
) compounds were fabricated and powder x-ray diffraction was carried out to confirm the phase purity. The XRD patterns of Ho
x
Er
 Ni (
Ni (
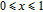 ) compounds are shown in Fig. 22. The patterns of GdNi and DyNi are not shown literature.[77,78] All of the XRD patterns have been fitted and refined. All of the RNi compounds is single phase without any impurity phases. The vertical bars on the bottom of Fig. 22 are Bragg positions. It can be seen that almost all of the peaks can be corresponding to the Bragg positions. Actually, results of refinement show that GdNi compound crystallize in the orthorhombic CrB-type structure (space group
) compounds are shown in Fig. 22. The patterns of GdNi and DyNi are not shown literature.[77,78] All of the XRD patterns have been fitted and refined. All of the RNi compounds is single phase without any impurity phases. The vertical bars on the bottom of Fig. 22 are Bragg positions. It can be seen that almost all of the peaks can be corresponding to the Bragg positions. Actually, results of refinement show that GdNi compound crystallize in the orthorhombic CrB-type structure (space group
 , #63), which is the same with RGa compounds. Both Gd and Ni atoms are in the same crystallographic
, #63), which is the same with RGa compounds. Both Gd and Ni atoms are in the same crystallographic
 sites with the C
2v point symmetry.[22,23] While other RNi compounds crystallize in the orthorhombic FeB type structure (space group Pnma, #62).[69,77,78] The unit cell of FeB-type crystal structure is shown in Fig. 23. Both R and Ni atoms occupy the
sites with the C
2v point symmetry.[22,23] While other RNi compounds crystallize in the orthorhombic FeB type structure (space group Pnma, #62).[69,77,78] The unit cell of FeB-type crystal structure is shown in Fig. 23. Both R and Ni atoms occupy the
 site of the unit cell. The structure is made up of trigonal prisms with R at the corners and Ni at the center.[77] The lattice parameters of GdNi are determined to be
site of the unit cell. The structure is made up of trigonal prisms with R at the corners and Ni at the center.[77] The lattice parameters of GdNi are determined to be
 Å,
Å,
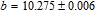 Å,
Å,
 Å. The lattice parameters of DyNi, HoNi, ErNi are
Å. The lattice parameters of DyNi, HoNi, ErNi are
 Å, 7.018±0.001 Å, 6.992±0.001 Å,
Å, 7.018±0.001 Å, 6.992±0.001 Å,
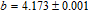 Å, 4.137±0.001 Å, 4.115±0.001 Å,
Å, 4.137±0.001 Å, 4.115±0.001 Å,
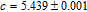 Å, 5.435±0.001 Å, 5.418±0.001 Å, respectively.[77,78] As the R atom number increases, the radius of R atom decreases. And as a result, the crystal parameters also show a decreasing tendency.
Å, 5.435±0.001 Å, 5.418±0.001 Å, respectively.[77,78] As the R atom number increases, the radius of R atom decreases. And as a result, the crystal parameters also show a decreasing tendency.
The thermal magnetization curves of RNi (
 , Dy, Ho, Er) compounds were measured and the field-cooling curves of GdNi, HoNi, DyNi, and ErNi are shown in Fig. 24(a), Fig. 24(b), Fig. 24(c), and Fig. 24(d), respectively.[77,78] As the temperature increases, the magnetization of GdNi compound shows a sudden decrease at
, Dy, Ho, Er) compounds were measured and the field-cooling curves of GdNi, HoNi, DyNi, and ErNi are shown in Fig. 24(a), Fig. 24(b), Fig. 24(c), and Fig. 24(d), respectively.[77,78] As the temperature increases, the magnetization of GdNi compound shows a sudden decrease at
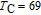 K, which is determined by the maximum of
K, which is determined by the maximum of
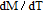 . In the low temperature range, the magnetization of GdNi compound almost keeps a stable value. The above result is in accordance with previous work.[66] From the M–T curve, it can be concluded that GdNi is a simple FM material, and it undergoes an FM to PM transition as temperature increases around
. In the low temperature range, the magnetization of GdNi compound almost keeps a stable value. The above result is in accordance with previous work.[66] From the M–T curve, it can be concluded that GdNi is a simple FM material, and it undergoes an FM to PM transition as temperature increases around
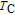 . HoNi compound has been known as a multi-phase-transition material, and the magnetic transitions have been observed by magnetic measurements and neutron diffraction technique.[66,67] From Fig. 24(b), it can be seen that as temperature increases the magnetization undergoes drastic change twice at 13.5 K and 35.5 K, respectively. The two temperatures are corresponding to the SR transition and FM to PM transition respectively. The magnetic properties of DyNi and ErNi compounds have been studied before, and drastic change of the magnetization due to the spin flopping was observed for both ErNi and DyNi compounds.[72] The magnetic structure of DyNi compound is non-collinear, and the magnetization of DyNi compound shows several increase due to spin flopping with external field increase.[77] DyNi and ErNi compounds have been treated as simple FM materials in previous work, and only one FM to PM transition has been discussed for DyNi and ErNi compounds.[78] However, from Fig. 24(c) and Fig. 24(d), it is clearly shown that there are two drastic change of magnetization below
. HoNi compound has been known as a multi-phase-transition material, and the magnetic transitions have been observed by magnetic measurements and neutron diffraction technique.[66,67] From Fig. 24(b), it can be seen that as temperature increases the magnetization undergoes drastic change twice at 13.5 K and 35.5 K, respectively. The two temperatures are corresponding to the SR transition and FM to PM transition respectively. The magnetic properties of DyNi and ErNi compounds have been studied before, and drastic change of the magnetization due to the spin flopping was observed for both ErNi and DyNi compounds.[72] The magnetic structure of DyNi compound is non-collinear, and the magnetization of DyNi compound shows several increase due to spin flopping with external field increase.[77] DyNi and ErNi compounds have been treated as simple FM materials in previous work, and only one FM to PM transition has been discussed for DyNi and ErNi compounds.[78] However, from Fig. 24(c) and Fig. 24(d), it is clearly shown that there are two drastic change of magnetization below
 as temperature increases for DyNi and ErNi compounds, which indicates that SR transition may exist both for DyNi and ErNi compounds. The value of
as temperature increases for DyNi and ErNi compounds, which indicates that SR transition may exist both for DyNi and ErNi compounds. The value of
 for DyNi and ErNi is determined to be 20 K and 6.5 K, respectively. The
for DyNi and ErNi is determined to be 20 K and 6.5 K, respectively. The
 for DyNi and ErNi is determined to be 59 K and 11 K, respectively.[77] All of the transition temperatures of RNi compounds (
for DyNi and ErNi is determined to be 59 K and 11 K, respectively.[77] All of the transition temperatures of RNi compounds (
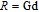 , Dy, Ho, Er) are shown in Table 3. As the atom number increases, Curie temperature shows a decrease tendency. That is because the spin angular momentum quantum number (S) increases with atom number for heavy rare earth elements. And S is positively correlated with Curie temperatures of R-based compounds.
, Dy, Ho, Er) are shown in Table 3. As the atom number increases, Curie temperature shows a decrease tendency. That is because the spin angular momentum quantum number (S) increases with atom number for heavy rare earth elements. And S is positively correlated with Curie temperatures of R-based compounds.
The isothermal magnetization curves of HoNi compound were measured up to 5 T and the curves at 2 K, 12 K, 18 K, 24 K, 30 K, 36 K, 42 K, 48 K, and 55 K are shown in Fig. 25, respectively. For the M–H curve obtained below
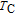 , the magnetization increases as magnetic field increases, and reaches its saturation values quickly. That is the typical feature of FM materials. The two magnetic transitions HoNi compound undergoes as temperature increases have been confirmed by magnetic measurement and neutron diffraction technique. Then it can be concluded that the transition around
, the magnetization increases as magnetic field increases, and reaches its saturation values quickly. That is the typical feature of FM materials. The two magnetic transitions HoNi compound undergoes as temperature increases have been confirmed by magnetic measurement and neutron diffraction technique. Then it can be concluded that the transition around
 is FM to FM transition, because the M–H curves obtained below and above
is FM to FM transition, because the M–H curves obtained below and above
 are both FM type. The M–H curve at 36 K shows a nearly linear change feature, which is the characteristic of PM state. So it can be concluded that there is little short range FM order above
are both FM type. The M–H curve at 36 K shows a nearly linear change feature, which is the characteristic of PM state. So it can be concluded that there is little short range FM order above
 for HoNi compound.
for HoNi compound.
Arrott plot was obtained from the isothermal magnetization curves for HoNi compound and it is shown in Fig. 26. According to the Banerjee criterion a magnetic transition is expected to be of the first-order when the slope of Arrott plot is negative, whereas it will be of the second-order when the slope is positive.[42,79] From the Arrott plots in the temperature range from 2 K to 45 K, it can be seen that none of them show a negative slope. The positive slope of Arrott around
 and
and
 indicates that the SR transition and FM to PM transition are both of second order. Generally to say, the magnetocaloric effect materials of second order are more valuable for actually use, because there are little magnetic hysteresis and heat hysteresis in the process of magnetic transition.[19]
indicates that the SR transition and FM to PM transition are both of second order. Generally to say, the magnetocaloric effect materials of second order are more valuable for actually use, because there are little magnetic hysteresis and heat hysteresis in the process of magnetic transition.[19]
Figure 27 shows the ZFC and FC curves of HoNi compound at a field of 0.02 T. It is clearly that HoNi compound show good thermal reversibility both around
 and
and
 . The results of ZFC and FC are in accordance with the results of Arrott plots. In addition, the ZFC and FC curves also clearly show that HoNi compound undergoes two magnetic transitions as temperature increases at
. The results of ZFC and FC are in accordance with the results of Arrott plots. In addition, the ZFC and FC curves also clearly show that HoNi compound undergoes two magnetic transitions as temperature increases at
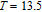 K and
K and
 K, respectively. The magnetic transition of HoNi will be discussed in detail in the following section.
K, respectively. The magnetic transition of HoNi will be discussed in detail in the following section.
In order to study the influence of Ho atom on the magnetic property of ErNi compound, Ho
x
Er
 Ni (
Ni (
 ) compounds were fabricated and the thermal magnetization curves at a field of 0.01 T were measured.[76] The M–T curves of Ho
x
Er
) compounds were fabricated and the thermal magnetization curves at a field of 0.01 T were measured.[76] The M–T curves of Ho
x
Er
 Ni (
Ni (
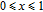 ) compounds are shown in Fig. 28. The inset of Fig. 28 is the enlarge view of M–T curve of ErNi compound. The magnetization undergoes a sharp decrease around
) compounds are shown in Fig. 28. The inset of Fig. 28 is the enlarge view of M–T curve of ErNi compound. The magnetization undergoes a sharp decrease around
 for all of the Ho
x
Er
for all of the Ho
x
Er
 Ni (
Ni (
 ) compounds. And it is clear that there is also another significant decrease in low temperature range for Ho
x
Er
) compounds. And it is clear that there is also another significant decrease in low temperature range for Ho
x
Er
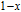 Ni (
Ni (
 ) compounds, which indicates that these compounds undergo another magnetic transition in low temperature zone. Considering of the SR transition of HoNi, the above transitions are probably SR transition as well. Spin slopping was observed with field increasing for ErNi compound, but ErNi compound has been treated as a simple FM material in previous researches.[72,78] But from the enlarge view of M–T curve of ErNi compound, it can be seen that there is also another decrease of magnetization around
) compounds, which indicates that these compounds undergo another magnetic transition in low temperature zone. Considering of the SR transition of HoNi, the above transitions are probably SR transition as well. Spin slopping was observed with field increasing for ErNi compound, but ErNi compound has been treated as a simple FM material in previous researches.[72,78] But from the enlarge view of M–T curve of ErNi compound, it can be seen that there is also another decrease of magnetization around
 K, although the decrease is not so obvious. Actually, the decrease has been observed in work given by Kumar et al.[78] So it can be concluded that ErNi compound also under goes an SR transition and an FM to PM transition as temperature increases, which is the same case as HoNi compound. After the detailed study of the ZFC and FC, it found that Ho
K, although the decrease is not so obvious. Actually, the decrease has been observed in work given by Kumar et al.[78] So it can be concluded that ErNi compound also under goes an SR transition and an FM to PM transition as temperature increases, which is the same case as HoNi compound. After the detailed study of the ZFC and FC, it found that Ho
 Er
Er
 Ni compound also undergoes two magnetic transitions. But for Ho
Ni compound also undergoes two magnetic transitions. But for Ho
 Er
Er
 Ni compound, no drastic change of magnetization can be observed obviously except for the one at
Ni compound, no drastic change of magnetization can be observed obviously except for the one at
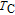 . AC susceptibility measurements show that Ho
. AC susceptibility measurements show that Ho
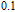 Er
Er
 Ni compound also undergoes an SR transition around 9 K.[76] All of the magnetic transition temperatures have been determined by the position of the peak on the
Ni compound also undergoes an SR transition around 9 K.[76] All of the magnetic transition temperatures have been determined by the position of the peak on the
 curves. The transition temperatures are shown in Table 3. It can be seen that the Curie temperature increases from 11 K to 35.5 K as the content of Ho atoms increases, showing a steadily increasing tendency. But the SR transition temperature only changes between 4 K and 13.5 K, which is relatively stable compared with
curves. The transition temperatures are shown in Table 3. It can be seen that the Curie temperature increases from 11 K to 35.5 K as the content of Ho atoms increases, showing a steadily increasing tendency. But the SR transition temperature only changes between 4 K and 13.5 K, which is relatively stable compared with
 . That is because the Curie temperature is positively correlated with S, and the average S of Ho
x
Er
. That is because the Curie temperature is positively correlated with S, and the average S of Ho
x
Er
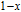 Ni compound increases as the content of Ho increases. But for
Ni compound increases as the content of Ho increases. But for
 , it is affected by crystal field and crystal structure. Considering that the Ho
x
Er
, it is affected by crystal field and crystal structure. Considering that the Ho
x
Er
 Ni compounds crystallize in the identify crystal structure,
Ni compounds crystallize in the identify crystal structure,
 thus changes not so much for different content of Ho atoms. It can also be seen that 10% of Ho-substitution for Er atoms obviously shortens the temperature interval between SR transition and FM to PM transition. The isothermal magnetization curves up to 5 T at 2 K for Ho
x
Er
thus changes not so much for different content of Ho atoms. It can also be seen that 10% of Ho-substitution for Er atoms obviously shortens the temperature interval between SR transition and FM to PM transition. The isothermal magnetization curves up to 5 T at 2 K for Ho
x
Er
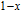 Ni (
Ni (
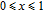 ) compounds were also measured and have been shown in Fig. 29. All of the M–H curves show that the magnetization increases as magnetic field adding up and arrives at its stable value quickly, which is the typical feature of FM ground state. It indicates that the ground state of Ho
x
Er
) compounds were also measured and have been shown in Fig. 29. All of the M–H curves show that the magnetization increases as magnetic field adding up and arrives at its stable value quickly, which is the typical feature of FM ground state. It indicates that the ground state of Ho
x
Er
 Ni (
Ni (
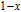 ) compounds in low temperature range is FM and it can be speculated that the SR transitions are FM to FM transition for Ho
x
Er
) compounds in low temperature range is FM and it can be speculated that the SR transitions are FM to FM transition for Ho
x
Er
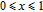 Ni (
Ni (
 ) compounds. The saturation magnetization for HoNi compound shows the largest value, because the total angular quantum number of HoNi compound is the largest. The saturation magnetizations for other Ho
x
Er
) compounds. The saturation magnetization for HoNi compound shows the largest value, because the total angular quantum number of HoNi compound is the largest. The saturation magnetizations for other Ho
x
Er
 Ni compounds show a confused order in value, which indicates the magnetizing process is affected by other factors, such as crystal field.
Ni compounds show a confused order in value, which indicates the magnetizing process is affected by other factors, such as crystal field.
3.2. The magnetic structure and magnetic transitions of HoNi compound studied by neutron diffraction experimentThe magnetic transition of HoNi compound has been studied according to many kinds of experiments such as magnetic measurements and neutron diffraction technique.[66,67,78,80–82] It indicates that HoNi compound undergoes an SR transition and an FM to PM transition as temperature increases. The neutron diffraction studies show that the magnetic moments in HoNi have ferromagnetic components along the a axis and antiferromagnetic components along the c axis in the temperature range between
 and
and
 .[67,78] Below
.[67,78] Below
 , a change in symmetry has been observed due to the development of the ferromagnetic component along the b axis which grows with decreasing temperature.[78] From the behavior of the M–Tcurve of HoNi, the presence of a canted structure below
, a change in symmetry has been observed due to the development of the ferromagnetic component along the b axis which grows with decreasing temperature.[78] From the behavior of the M–Tcurve of HoNi, the presence of a canted structure below
 can be inferred.[78] But the magnetic and magnetic transition of HoNi compound still needs to be studied further. In the following section the magnetic structure and magnetic transition of HoNi compound will be studied systemically by employing powder neutron diffraction experiments.[76]
can be inferred.[78] But the magnetic and magnetic transition of HoNi compound still needs to be studied further. In the following section the magnetic structure and magnetic transition of HoNi compound will be studied systemically by employing powder neutron diffraction experiments.[76]
Firstly, the magnetic structure of HoNi compound at low temperature needs to be determined. And before determining the magnetic structure, the crystal structure was analyzed and determined. The powder neutron diffraction pattern of HoNi compound at 295K is shown in Fig. 30(a). The fitted lines, differences and Bragg positions are also shown there. Since the Curie temperature is around 35 K, HoNi compound is in PM state at 295 K. Thus only nuclear model is considered in the process of refining and fitting. All of the peaks can be indexed to the FeB-type orthorhombic crystal structure (space group pnam, #62). The crystal structure has been shown in Fig. 23 and the related parameters are as follows:
 Å,
Å,
 Å,
Å,
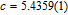 Å,
Å,
 ,
,
 ,
,
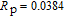 . The powder neutron diffraction pattern at 5.3 K is shown in Fig. 30(b). The fitted lines, differences and Bragg positions are also shown in the figure. At 5.3 K, HoNi compound is in FM-ordered magnetic state. Thus nuclear and magnetic structures are both considered. The Bragg positions for nuclear structure and magnetic structure are marked in Fig. 30(b), respectively. The related parameters are as follows:
. The powder neutron diffraction pattern at 5.3 K is shown in Fig. 30(b). The fitted lines, differences and Bragg positions are also shown in the figure. At 5.3 K, HoNi compound is in FM-ordered magnetic state. Thus nuclear and magnetic structures are both considered. The Bragg positions for nuclear structure and magnetic structure are marked in Fig. 30(b), respectively. The related parameters are as follows:
 Å,
Å,
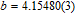 Å,
Å,
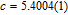 Å,
Å,
 ,
,
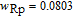 ,
,
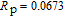 . Results indicate that the only Ho atoms are magnetic ordered, and the Ni atoms contribute nothing to the magnetic moment. According to the refined magnetic structure at 5.3 K, Ho atoms are FM-ordered along a axis and b axis, and they are AFM-ordered along c axis. The component of ordered magnetic moment for x, y, z is determined to be 7.66(7)
. Results indicate that the only Ho atoms are magnetic ordered, and the Ni atoms contribute nothing to the magnetic moment. According to the refined magnetic structure at 5.3 K, Ho atoms are FM-ordered along a axis and b axis, and they are AFM-ordered along c axis. The component of ordered magnetic moment for x, y, z is determined to be 7.66(7)
 , 4.4(1)
, 4.4(1)
 , and 3.80(5)
, and 3.80(5)
 , respectively. And the total ordered magnetic moment is calculated to be 9.63(8)
, respectively. And the total ordered magnetic moment is calculated to be 9.63(8)
 , which is close to the effective moment of Ho
, which is close to the effective moment of Ho
 ion. It indicates that almost no magnetic component is quenched for Ho ion in HoNi compound. The magnetic structure of HoNi compound is presented in Fig. 31, where it is viewed from b axis. Since it is viewed from b axis, the y component of ordered magnetic moment cannot be plotted.
ion. It indicates that almost no magnetic component is quenched for Ho ion in HoNi compound. The magnetic structure of HoNi compound is presented in Fig. 31, where it is viewed from b axis. Since it is viewed from b axis, the y component of ordered magnetic moment cannot be plotted.
From Fig. 31 it can be seen that the Ho atoms are connected in the form of six-member ring and the Ni atoms are in the center of the six-member ring. Half of the Ho atoms point to one direction with a positive x component and a positive z component, and the other half of Ho atoms point to another direction with a positive x component and negative z component. The x component is the same for all the Ho atoms, which indicates that Ho atoms are FM ordered in HoNi compound. The absolute value of z component of all the Ho atoms is also uniform, which means that AFM order of Ho atoms exists in HoNi compound. Just as what has been mentioned before, there is also an FM order of Ho atoms along b axis in HoNi compound, which cannot be presented in Fig. 31.
Secondly, the neutron powder diffraction patterns at different temperatures were also obtained and the magnetic structures at different temperatures were determined according to the refinement and fitting. The specific parameters of crystal structure, ordered magnetic moments, and error information are all listed in Table 4.
Table 4.
Table 4.
 Table 4.
(a) Refined structural parameters of HoNi compound. Space group
 , Atomic positions: Ho (x, 0.25, z); Ni (x, 0.25, z). a, b, c, and V are lattice constant and volume of unit cell. M, M
x
, M
y
, and M
z
are the total ordered magnetic moment and the individual component of moment along a, b, and c axes, respectively. ϕ and θ are the angle between the ordered magnetic moment and x, z axes, respectively.
, Atomic positions: Ho (x, 0.25, z); Ni (x, 0.25, z). a, b, c, and V are lattice constant and volume of unit cell. M, M
x
, M
y
, and M
z
are the total ordered magnetic moment and the individual component of moment along a, b, and c axes, respectively. ϕ and θ are the angle between the ordered magnetic moment and x, z axes, respectively.
.
| Atoms |
Parameters |
5.3 K |
8 K |
10 K |
12 K |
13 K |
15 K |
20 K |
25 K |
|
| a/Å |
6.9765(1) |
6.9763(2) |
6.9765(2) |
6.9768(2) |
6.9765(2) |
6.9769(2) |
6.9764(1) |
6.9766(2) |
|
|
b/Å |
4.15480(3) |
4.15477(4) |
4.15481(3) |
4.15486(4) |
4.15482(4) |
4.15490(4) |
4.15478(3) |
4.15482(4) |
|
|
c/Å |
5.4004(1) |
5.4008(1) |
5.4007(1) |
5.4010(2) |
5.4010(2) |
5.4015(2) |
5.4016(1) |
5.4019(2) |
|
|
V/Å3
|
156.534(5) |
156.542(6) |
156.545(5) |
156.562(6) |
156.556(6) |
156.582(6) |
156.566(5) |
156.582(6) |
| Ho |
x
|
0.1820(3) |
0.1816(3) |
0.1817(3) |
0.1813(4) |
0.1818(3) |
0.1812(3) |
0.1814(3) |
0.1808(4) |
|
|
z
|
0.1313(6) |
0.1327(7) |
0.1327(6) |
0.1332(7) |
0.1318(7) |
0.1307(8) |
0.1332(6) |
0.1336(7) |
|
|

|
7.66(7) |
7.63(8) |
7.73(6) |
7.58(7) |
7.57(8) |
7.42(7) |
6.98(5) |
5.93(7) |
|
|

|
4.4(1) |
3.9(1) |
2.7(1) |
1.5(3) |
1.9(2) |
1.3(3) |
0 |
0 |
|
|

|
–3.80(5) |
–3.74(6) |
–3.69(5) |
–3.70(6) |
–3.73(6) |
–3.65(6) |
–3.39(4) |
–3.06(6) |
|
|
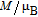
|
9.63(8) |
9.34(9) |
8.99(7) |
8.57(9) |
8.65(9) |
8.38(8) |
7.76(6) |
6.67(7) |
|
|

|
113.2(2) |
113.6(3) |
114.2(3) |
115.6(3) |
115.6(3) |
115.8(3) |
115.9(3) |
117.3(5) |
|
|

|
30.1(6) |
27.0(8) |
19.6(9) |
11(2) |
14(2) |
10(2) |
0 |
0 |
| Ni |
x
|
0.0358(3) |
0.0354(3) |
0.0355(3) |
0.0357(3) |
0.0364(3) |
0.0857(3) |
0.0362(2) |
0.0362(3) |
|
|
z
|
0.6282(5) |
0.6268(5) |
0.6261(5) |
0.6257(5) |
0.6271(6) |
0.6287(6) |
0.6269(4) |
0.6261(5) |
|
|
 /%
/% |
0.0673 |
0.0816 |
0.0705 |
0.0854 |
0.0838 |
0.0793 |
0.0609 |
0.0796 |
|
|
 /%
/% |
0.0803 |
0.0982 |
0.0837 |
0.1016 |
0.0996 |
0.0957 |
0.0736 |
0.0967 |
|
|
χ
2
|
2.795 |
1.346 |
1.984 |
1.396 |
1.364 |
1.335 |
2.271 |
1.262 |
| Table 4.
(a) Refined structural parameters of HoNi compound. Space group
 , Atomic positions: Ho (x, 0.25, z); Ni (x, 0.25, z). a, b, c, and V are lattice constant and volume of unit cell. M, M
x
, M
y
, and M
z
are the total ordered magnetic moment and the individual component of moment along a, b, and c axes, respectively. ϕ and θ are the angle between the ordered magnetic moment and x, z axes, respectively.
, Atomic positions: Ho (x, 0.25, z); Ni (x, 0.25, z). a, b, c, and V are lattice constant and volume of unit cell. M, M
x
, M
y
, and M
z
are the total ordered magnetic moment and the individual component of moment along a, b, and c axes, respectively. ϕ and θ are the angle between the ordered magnetic moment and x, z axes, respectively.
. |
Table 4.
Table 4.
 Table 4.
(b) Refined structural parameters of HoNi compound. Space group Pnma, Atomic positions: Ho (x, 0.25, z); Ni (x, 0.25, z). a, b, c, and V are lattice constant and volume of unit cell. M, Mx, My, and Mz are the total ordered magnetic moment and the individual component of moment along a, b, and c axes, respectively. ϕ and θ are the angle between the ordered magnetic moment and x, z axes, respectively.
.
| Atoms |
Parameters |
30 K |
32 K |
34 K |
36 K |
38 K |
40 K |
42 K |
45 K |
295 K |
|
|
a/Å |
6.9769(2) |
6.9774(2) |
6.9760(2) |
6.9758(2) |
6.9767(2) |
6.9768(2) |
6.9779(2) |
6.9784(2) |
7.0162(2) |
|
|
b/Å |
4.15490(4) |
4.15500(4) |
4.1559(1) |
4.1557(1) |
4.1555(1) |
4.1552(1) |
4.1546(1) |
4.1536(1) |
4.1405(1) |
|
|
c/Å |
5.4022(2) |
5.4011(2) |
5.3999(2) |
5.4003(2) |
5.4003(2) |
5.4008(2) |
5.4011(2) |
5.4019(2) |
5.4359(1) |
|
|
V/Å3
|
156.601(6) |
156.584(6) |
156.552(6) |
156.553(6) |
156.565(6) |
156.570(6) |
156.580(6) |
156.578(6) |
157.916(5) |
| Ho |
x
|
0.1804(3) |
0.1797(3) |
0.1802(3) |
0.1797(3) |
0.1797(3) |
0.1797(3) |
0.1798(3) |
0.1803(3) |
0.1800(2) |
|
|
z
|
0.1315(8) |
0.1316(7) |
0.1316(7) |
0.1332(7) |
0.1340(7) |
0.1318(7) |
0.1329(7) |
0.1334(6) |
0.1313(5) |
|
|

|
4.27(6) |
3.08(7) |
0 |
0 |
0 |
0 |
0 |
0 |
0 |
|
|
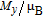
|
0 |
0 |
0 |
0 |
0 |
0 |
0 |
0 |
0 |
|
|

|
–2.00(7) |
–1.33(9) |
0 |
0 |
0 |
0 |
0 |
0 |
0 |
|
|

|
4.71(7) |
3.36(7) |
0 |
0 |
0 |
0 |
0 |
0 |
0 |
|
|

|
115.1(8) |
113(1) |
0 |
0 |
0 |
0 |
0 |
0 |
0 |
|
|

|
0 |
0 |
0 |
0 |
0 |
0 |
0 |
0 |
0 |
| Ni |
x
|
0.0367(3) |
0.0360(3) |
0.0367(3) |
0.0365(3) |
0.0364(3) |
0.0364(3) |
0.0364(3) |
0.0363(3) |
0.0372(2) |
|
|
z
|
0.6277(6) |
0.6285(6) |
0.6291(5) |
0.6286(5) |
0.6276(5) |
0.6282(5) |
0.6271(5) |
0.6275(5) |
0.6285(4) |
|
|
 /%
/% |
0.0667 |
0.0671 |
0.0642 |
0.0677 |
0.0639 |
0.0632 |
0.0609 |
0.0660 |
0.0384 |
|
|
 /%
/% |
0.0816 |
0.0811 |
0.0797 |
0.0832 |
0.0787 |
0.0783 |
0.0755 |
0.0804 |
0.0475 |
|
|
χ
2
|
1.087 |
0.9613 |
0.9177 |
0.8818 |
0.9514 |
0.8915 |
0.8803 |
0.9041 |
0.9758 |
| Table 4.
(b) Refined structural parameters of HoNi compound. Space group Pnma, Atomic positions: Ho (x, 0.25, z); Ni (x, 0.25, z). a, b, c, and V are lattice constant and volume of unit cell. M, Mx, My, and Mz are the total ordered magnetic moment and the individual component of moment along a, b, and c axes, respectively. ϕ and θ are the angle between the ordered magnetic moment and x, z axes, respectively.
. |
Results show that the x component of ordered magnetic moment exists in the temperature range from 5.3 K to 32 K, and it changes from 7.66(7)
 to 3.08(7)
to 3.08(7)
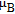 . The z component shows the similar case to the x component and it changes from 3.80(5)
. The z component shows the similar case to the x component and it changes from 3.80(5)
 to 1.33(9)
to 1.33(9)
 as temperature increases from 5.3 K to 32 K. So the temperature around 32 K is corresponding to magnetic order to disorder temperature point, which is known as Curie temperature. Also it is found that the y component of ordered magnetic moment changes from 4.4(1)
as temperature increases from 5.3 K to 32 K. So the temperature around 32 K is corresponding to magnetic order to disorder temperature point, which is known as Curie temperature. Also it is found that the y component of ordered magnetic moment changes from 4.4(1)
 to 1.3(3)
to 1.3(3)
 as temperature increases and it disappears from
as temperature increases and it disappears from
 K. It indicates that the there is a magnetic transition and the transition temperature is around 15 K. As a matter of fact, the magnetic transition of HoNi compound in low temperature range has been observed according to magnetic measurements.[66,78]
K. It indicates that the there is a magnetic transition and the transition temperature is around 15 K. As a matter of fact, the magnetic transition of HoNi compound in low temperature range has been observed according to magnetic measurements.[66,78]
Figure 32(a) shows the thermal magnetization curve of HoNi compound at a field of 0.01 T. As temperature increases, the magnetization shows drastic decrease at
 and
and
 , respectively. The value of
, respectively. The value of
 and
and
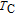 is determined to be 13.5 K and 35.5 K, respectively, according to the maximum of
is determined to be 13.5 K and 35.5 K, respectively, according to the maximum of
 (see the inset of Fig. 32(a)). The results of ordered magnetic moments and the components along different axis according to NPD fitting are also shown in Fig. 32(b). It is clear that the FM x component and AFM z component both undergo an order to disorder transition around 32 K, and the FM y component decreases as temperature increases and falls down to zero at 20 K. As the total ordered magnetic moment, M shows sudden decrease both at
(see the inset of Fig. 32(a)). The results of ordered magnetic moments and the components along different axis according to NPD fitting are also shown in Fig. 32(b). It is clear that the FM x component and AFM z component both undergo an order to disorder transition around 32 K, and the FM y component decreases as temperature increases and falls down to zero at 20 K. As the total ordered magnetic moment, M shows sudden decrease both at
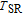 and
and
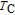 .
.
The angle between the x axis and the xy component of the ordered magnetic moment was also calculated for every temperature and it was marked as ψ. The temperature dependence of the ψ curve is shown in Fig. 32(c). As temperature increases, ψ decreases from 30
 at 5.3 K to 10
at 5.3 K to 10
 at 15 K, and it falls down to zero at 20 K, which means that the y component disappears. From the change tendency of the three components of ordered magnetic moment and the value of ψ as temperature increases, it can be seen that the HoNi compound undergoes a spin reorientation process at
at 15 K, and it falls down to zero at 20 K, which means that the y component disappears. From the change tendency of the three components of ordered magnetic moment and the value of ψ as temperature increases, it can be seen that the HoNi compound undergoes a spin reorientation process at
 except for the ferromagnetic to paramagnetic transition at
except for the ferromagnetic to paramagnetic transition at
 as temperature increases. Besides, an obvious change of crystal lattice parameters was also observed according to the neutron powder diffraction experiments. The temperature dependences of parameters a, b, c, and V are shown in Fig. 33. Parameters a, b, c, and V all show a change corresponding to the spin reorientation transition around 15 K, which means that the disappearing of the y component affects the crystal structure from three directions. As temperature increases, all of the above parameters show sharp variation at the temperature
as temperature increases. Besides, an obvious change of crystal lattice parameters was also observed according to the neutron powder diffraction experiments. The temperature dependences of parameters a, b, c, and V are shown in Fig. 33. Parameters a, b, c, and V all show a change corresponding to the spin reorientation transition around 15 K, which means that the disappearing of the y component affects the crystal structure from three directions. As temperature increases, all of the above parameters show sharp variation at the temperature
 , which indicates that the ferromagnetic to paramagnetic transition affects the crystal structure more than the spin reorientation transition does. Although the spin reorientation transition has been observed and studied before, the present work shows a more complete sketch of that magnetic transition for the HoNi compound.
, which indicates that the ferromagnetic to paramagnetic transition affects the crystal structure more than the spin reorientation transition does. Although the spin reorientation transition has been observed and studied before, the present work shows a more complete sketch of that magnetic transition for the HoNi compound.
3.3. The magnetocaloric effect of
 Ni compounds
Ni compoundsThe
 of RNi (
of RNi (
 , Dy, Ho, and Er) compounds was calculated based on the isothermal magnetization data (not shown here) by using the Maxwell relation
, Dy, Ho, and Er) compounds was calculated based on the isothermal magnetization data (not shown here) by using the Maxwell relation
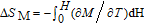 . The
. The
 curves as a function of temperature for RNi (
curves as a function of temperature for RNi (
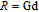 , Dy, Ho, Er) compounds with a field change of 0 T–2 T and 0 T–5 T are shown in Fig. 34(a) and Fig. 34(b), respectively. The data for GdNi and DyNi compounds are cited from literatures.[77,78] and the data for HoNi and ErNi compounds are newly obtained in this work. The MCE curve obtained for GdNi shows a steady increase in the
, Dy, Ho, Er) compounds with a field change of 0 T–2 T and 0 T–5 T are shown in Fig. 34(a) and Fig. 34(b), respectively. The data for GdNi and DyNi compounds are cited from literatures.[77,78] and the data for HoNi and ErNi compounds are newly obtained in this work. The MCE curve obtained for GdNi shows a steady increase in the
 value with temperature and attains maximum value around its
value with temperature and attains maximum value around its
 .[78] The maximum value of
.[78] The maximum value of
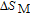 ,
,
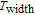 , and RC for a field change of 0 T–5 T for DyNi compound are estimated to be 18 J/kg
, and RC for a field change of 0 T–5 T for DyNi compound are estimated to be 18 J/kg
 K, 31 K, and 429.8 J/kg, respectively. The large MCE values in DyNi can be attributed to its metamagnetic nature.[77] It is possible that the gradual flopping of moments towards the direction of the applied field is responsible for the reduction in magnetic entropy, which results in MCE.[77] Spin-flop process has indeed been found to be responsible for the anomalous magnetoresistance observed along the c axis in single crystals of DyNi compound.[77,83] The curve for ErNi shows a sharp increase in the
K, 31 K, and 429.8 J/kg, respectively. The large MCE values in DyNi can be attributed to its metamagnetic nature.[77] It is possible that the gradual flopping of moments towards the direction of the applied field is responsible for the reduction in magnetic entropy, which results in MCE.[77] Spin-flop process has indeed been found to be responsible for the anomalous magnetoresistance observed along the c axis in single crystals of DyNi compound.[77,83] The curve for ErNi shows a sharp increase in the
 value near its
value near its
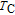 exhibiting the character of a typical ferromagnet whereas there is another peak around 15 K in the case of HoNi. The difference in the MCE behavior of ErNi and HoNi implies that though these compounds are non-collinear, they have different arrangements of moments in the magnetic sub-lattice.[78] The peak in low temperature range in HoNi compound is corresponding to the SR transition. It also can be seen that the maximum value at
exhibiting the character of a typical ferromagnet whereas there is another peak around 15 K in the case of HoNi. The difference in the MCE behavior of ErNi and HoNi implies that though these compounds are non-collinear, they have different arrangements of moments in the magnetic sub-lattice.[78] The peak in low temperature range in HoNi compound is corresponding to the SR transition. It also can be seen that the maximum value at
 is comparable to that at
is comparable to that at
 , which indicates that there is a very large change of magnetic entropy in the process of spin reorientation for HoNi compound. The two peaks on the
, which indicates that there is a very large change of magnetic entropy in the process of spin reorientation for HoNi compound. The two peaks on the
 curve for HoNi compound are corresponding to the two transition temperatures. As is discussed based on the thermal magnetization curves, there are also two magnetic transitions for DyNi and ErNi compounds. But no obvious magnetic entropy change is observed on the
curve for HoNi compound are corresponding to the two transition temperatures. As is discussed based on the thermal magnetization curves, there are also two magnetic transitions for DyNi and ErNi compounds. But no obvious magnetic entropy change is observed on the
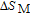 curve around
curve around
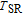 for DyNi and ErNi compounds, which indicates that the SR transition is very weak for the DyNi and ErNi compounds. All of the MCE parameters are listed in Table 3. It is also clear that the maximum
for DyNi and ErNi compounds, which indicates that the SR transition is very weak for the DyNi and ErNi compounds. All of the MCE parameters are listed in Table 3. It is also clear that the maximum
 for ErNi compound shows the largest value among the RNi compounds.
for ErNi compound shows the largest value among the RNi compounds.
The isothermal magnetization curves at different temperatures were measured and the
 was calculated for all of the Ho
x
Er
was calculated for all of the Ho
x
Er
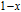 Ni (
Ni (
 ) compounds by using Maxwell relation
) compounds by using Maxwell relation
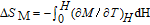 .[84] The temperature dependence of
.[84] The temperature dependence of
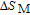 is shown in Fig. 35. It is found that the (
is shown in Fig. 35. It is found that the (
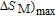 of Ho
of Ho
 Er
Er
 Ni is the largest among Ho
x
Er
Ni is the largest among Ho
x
Er
 Ni (
Ni (
 ) compounds. The value of (
) compounds. The value of (
 for ErNi and Ho
for ErNi and Ho
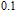 Er
Er
 Ni compound is 29.6 J/kg
Ni compound is 29.6 J/kg
 K and 34 J/kg
K and 34 J/kg
 K, respectively, i.e., 10% of Ho-substitution brings as high as
K, respectively, i.e., 10% of Ho-substitution brings as high as
 % ((34–29.6)/29.6 = 0.149) enhancement on (
% ((34–29.6)/29.6 = 0.149) enhancement on (
 . The refrigerant capacity (RC) of Ho
x
Er
. The refrigerant capacity (RC) of Ho
x
Er
 Ni (
Ni (
 ) compounds were also calculated by using the approach suggested by Gschneidner et al.[53] The RC is defined as
) compounds were also calculated by using the approach suggested by Gschneidner et al.[53] The RC is defined as
 , where T
1 and T
2 are the temperatures corresponding to both sides of the half-maximum value of (
, where T
1 and T
2 are the temperatures corresponding to both sides of the half-maximum value of (
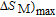 , respectively. The refrigerant temperature width (
, respectively. The refrigerant temperature width (
 is defined as the temperature span of the full width at half maximum value of (
is defined as the temperature span of the full width at half maximum value of (
 . Then
. Then
 can be obtained by T
2–T
1.
can be obtained by T
2–T
1.
All the MCE parameters including (
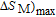 ,
,
 , and RC for Ho
x
Er
, and RC for Ho
x
Er
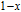 Ni (
Ni (
 ) compounds are listed in Table 3. As the content of Ho increases, the (
) compounds are listed in Table 3. As the content of Ho increases, the (
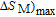 first increases and then decreases. Among all the Ho
x
Er
first increases and then decreases. Among all the Ho
x
Er
 Ni (
Ni (
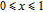 ) compounds, Ho
) compounds, Ho
 Er
Er
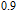 Ni compound shows the largest (
Ni compound shows the largest (
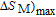 . Ho
. Ho
 Er
Er
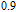 Ni compound has proved to be a second-order-transition material according to Arrott plots, but the (
Ni compound has proved to be a second-order-transition material according to Arrott plots, but the (
 (34 J/kg
(34 J/kg
 K for 0 T–5 T) is comparable to the representative low temperature first-order-transition MCE material ErCo2 compound (36.8 J/kg
K for 0 T–5 T) is comparable to the representative low temperature first-order-transition MCE material ErCo2 compound (36.8 J/kg
 K for 0 T–5 T).[85] The changing trend of
K for 0 T–5 T).[85] The changing trend of
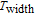 is opposite to (
is opposite to (
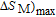 . Among all the Ho
x
Er
. Among all the Ho
x
Er
 Ni (
Ni (
 ) compounds, Ho
) compounds, Ho
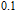 Er
Er
 Ni compound shows the smallest
Ni compound shows the smallest
 . As for RC, it increases monotonously from 350 J/kg to 556.7 J/kg as the content of Ho changes from 0 to 1. For Ho–Er–Ni compounds, Ho
. As for RC, it increases monotonously from 350 J/kg to 556.7 J/kg as the content of Ho changes from 0 to 1. For Ho–Er–Ni compounds, Ho
 Er
Er
 Ni exerts ideal (
Ni exerts ideal (
 but with ordinary
but with ordinary
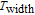 and RC. But it is valuable to discuss the MCE enhancement (peak value) with 10% of Ho-substitution compared with ErNi.
and RC. But it is valuable to discuss the MCE enhancement (peak value) with 10% of Ho-substitution compared with ErNi.
In fact, the MCE enhancement in Ho–Er–Ni compounds is closely related to the temperature interval between SR transition and FM to PM transition. Magnetic materials are in the non-steady magnetic state around transition temperatures. When a field is applied around transition temperature, obvious MCE can be observed, because the magnetic entropy is easy to change in this state. As a result, more than one
 peaks can be observed in multi-phase-transition MCE materials, such as ErGa.[19] and Ho2In.[86] compounds. According to thermal magnetization and ac susceptibility measurements, all of the Ho
x
Er
peaks can be observed in multi-phase-transition MCE materials, such as ErGa.[19] and Ho2In.[86] compounds. According to thermal magnetization and ac susceptibility measurements, all of the Ho
x
Er
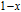 Ni (
Ni (
 ) compounds undergo two magnetic transitions as temperature increases: SR transition and FM to PM transition. More specially, the temperature interval between the two transitions is only 2 K for Ho
) compounds undergo two magnetic transitions as temperature increases: SR transition and FM to PM transition. More specially, the temperature interval between the two transitions is only 2 K for Ho
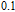 Er
Er
 Ni, which is the smallest value among the Ho
x
Er
Ni, which is the smallest value among the Ho
x
Er
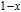 Ni (
Ni (
 ) compounds. When the temperature interval between the magnetic transitions is large, the corresponding two
) compounds. When the temperature interval between the magnetic transitions is large, the corresponding two
 peaks are separate, such as HoNi, Ho
peaks are separate, such as HoNi, Ho
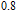 Er
Er
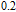 Ni and Ho
Ni and Ho
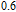 Er
Er
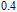 Ni compounds (see Fig. 35). But for other samples, the two peaks are hard to distinguish, because they overlap and merge together, which is similar to Ho12Co7 compound with large field change.[18] In this case,
Ni compounds (see Fig. 35). But for other samples, the two peaks are hard to distinguish, because they overlap and merge together, which is similar to Ho12Co7 compound with large field change.[18] In this case,
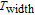 can also be used to evaluate the level of overlap. It is conspicuous that the
can also be used to evaluate the level of overlap. It is conspicuous that the
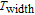 is the smallest for Ho
is the smallest for Ho
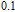 Er
Er
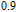 Ni compound, indicating the two
Ni compound, indicating the two
 peaks are almost entire-overlapping. Therefore, Ho
peaks are almost entire-overlapping. Therefore, Ho
 Er
Er
 Ni compound shows the largest (
Ni compound shows the largest (
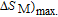
4.
 12Co7 compounds
12Co7 compoundsIt was reported that there are eight intermetallic compounds existing in the Co–R system, and R
12Co7 is among them.[87] Although R
12Co7 (
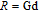 , Tb, Dy, Ho, Er) compounds have identical monoclinic structures, they exhibit different magnetic properties.[88] R
12Co7 (
, Tb, Dy, Ho, Er) compounds have identical monoclinic structures, they exhibit different magnetic properties.[88] R
12Co7 (
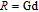 –Er) compounds crystallize in a monoclinic Ho12Co7-type structure (
–Er) compounds crystallize in a monoclinic Ho12Co7-type structure (
 ).[88] There are four different types of Co-centered R polyhedral in the unit cell: trigonal prism, cube, Archimedian antiprism, and truncated Archimedian antiprism.[88] The crystal structure of R
12Co7 compound is shown in Fig. 36. If the crystal structure is viewed from (100) direction, there are three types of Co-centered rare-earth polyhedral. Co(l) is at the center of a trigonal prism, Co(2) is surrounded by an Archimedian antiprism and Co(4) is at the center of a cube.[88]
).[88] There are four different types of Co-centered R polyhedral in the unit cell: trigonal prism, cube, Archimedian antiprism, and truncated Archimedian antiprism.[88] The crystal structure of R
12Co7 compound is shown in Fig. 36. If the crystal structure is viewed from (100) direction, there are three types of Co-centered rare-earth polyhedral. Co(l) is at the center of a trigonal prism, Co(2) is surrounded by an Archimedian antiprism and Co(4) is at the center of a cube.[88]
It has been reported that Gd12Co7, Tb12Co7, Gd4Tb8Co7, Gd8Tb4Co7, and Dy12Co7 undergo only one ferromagnetic (FM) to paramagnetic (PM) transition as temperature increases.[89–92] But a spin reorientation transition was observed at 123 K in Gd12Co
 compound according to literature.[92] According to our previous work, Ho12Co7 compound undergoes an antiferromagnetic (AFM) to AFM transition, an AFM to FM transition and an FM to PM transition at 9 K, 17 K, and 30 K, respectively.[18] According to the calculation and analysis of effective magnetic moments of R ions, Co atoms are considered to contribute to the magnetic moments in Ho12Co7 and Dy12Co7 compounds.[18,91] The magnetocaloric effect of R
12Co7 (
compound according to literature.[92] According to our previous work, Ho12Co7 compound undergoes an antiferromagnetic (AFM) to AFM transition, an AFM to FM transition and an FM to PM transition at 9 K, 17 K, and 30 K, respectively.[18] According to the calculation and analysis of effective magnetic moments of R ions, Co atoms are considered to contribute to the magnetic moments in Ho12Co7 and Dy12Co7 compounds.[18,91] The magnetocaloric effect of R
12Co7 (
 , Tb, Dy, Ho) compounds are studied in detail, and the value of RC is 555 J/kg for a field change of 0 T–5 T for Ho12Co7 compound with two
, Tb, Dy, Ho) compounds are studied in detail, and the value of RC is 555 J/kg for a field change of 0 T–5 T for Ho12Co7 compound with two
 peaks close to each other on the
peaks close to each other on the
 curves.[18] Recently, the magnetic property and magnetocaloric effect of Ho
x
Er
curves.[18] Recently, the magnetic property and magnetocaloric effect of Ho
x
Er
 Co7 (
Co7 (
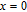 , 4, 6, 8, 10, 12) compounds are studied in detail.[93]
, 4, 6, 8, 10, 12) compounds are studied in detail.[93]
In the following section, we will review the property and magnetocaloric effect of binary R
12Co7 (
 , Tb, Dy, Ho) and pseudo-binary Ho
x
Er
, Tb, Dy, Ho) and pseudo-binary Ho
x
Er
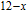 Co7 (
Co7 (
 , 4, 6, 8, 10, 12) and Gd
, 4, 6, 8, 10, 12) and Gd
 Tb
x
Co7 (
Tb
x
Co7 (
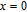 , 4, 8) compounds. The related data for Gd12Co7, Tb12Co7 and Gd
, 4, 8) compounds. The related data for Gd12Co7, Tb12Co7 and Gd
 Tb
x
Co7 (
Tb
x
Co7 (
 , 4, 8) compounds is cited from the literatures.[89,90,92]
, 4, 8) compounds is cited from the literatures.[89,90,92]
4.1. The magnetic transition of
 12Co7 compounds
12Co7 compoundsThe temperature (
 dependence of magnetization (
dependence of magnetization (
 was measured in both zero field-cooled (ZFC) and field-cooled (FC) processes in order to determine the thermal hysteresis and the magnetic transition temperature.
was measured in both zero field-cooled (ZFC) and field-cooled (FC) processes in order to determine the thermal hysteresis and the magnetic transition temperature.
Figure 37 shows the thermomagnetic M–T curves of R
12Co7 (
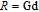 , Dy, Ho, Er) compounds measured under an external magnetic field of 0.1 T. The inset of the Fig. 37 is the ZFC and FC curves of Tb12Co7 compound under a field of 0.01 T. Apparently, the ZFC and FC magnetization curves are almost same for Gd12Co7 compound. The large drop of M–T curves corresponds to ferromagnetic to paramagnetic phase transition. The
, Dy, Ho, Er) compounds measured under an external magnetic field of 0.1 T. The inset of the Fig. 37 is the ZFC and FC curves of Tb12Co7 compound under a field of 0.01 T. Apparently, the ZFC and FC magnetization curves are almost same for Gd12Co7 compound. The large drop of M–T curves corresponds to ferromagnetic to paramagnetic phase transition. The
 of the compound is defined as the temperature where
of the compound is defined as the temperature where
 reached the peak point. In this work,
reached the peak point. In this work,
 of Gd12Co7 is about 163 K, the compound shows soft-ferromagnetic behavior below 163 K.[90] The ZFC and FC magnetization curves of Tb12Co7 compound are separated from each other below 100 K and there is a cusp in the ZFC curve, which is due to the temperature-dependent coercivity.[89] The compound shows ferromagnetic behavior. The Curie temperature
of Gd12Co7 is about 163 K, the compound shows soft-ferromagnetic behavior below 163 K.[90] The ZFC and FC magnetization curves of Tb12Co7 compound are separated from each other below 100 K and there is a cusp in the ZFC curve, which is due to the temperature-dependent coercivity.[89] The compound shows ferromagnetic behavior. The Curie temperature
 of Tb12Co7 is about 100 K, as obtained from the minimum of the first derivative of the FC M–T curve in a magnetic field of 0.01 T.[89] One can find that Dy12Co7 experiences an FM–PM transition around the Curie temperature
of Tb12Co7 is about 100 K, as obtained from the minimum of the first derivative of the FC M–T curve in a magnetic field of 0.01 T.[89] One can find that Dy12Co7 experiences an FM–PM transition around the Curie temperature
 K, defined as the minimum value of
K, defined as the minimum value of
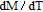 curve.[91] It can also be seen from Fig. 37 that the ZFC and FC curves are completely reversible in the vicinity of
curve.[91] It can also be seen from Fig. 37 that the ZFC and FC curves are completely reversible in the vicinity of
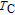 as shown usually in magnetic materials with a second-order magnetic transition. Meanwhile, a significant thermal irreversibility between the ZFC and FC branches is clearly observed below
as shown usually in magnetic materials with a second-order magnetic transition. Meanwhile, a significant thermal irreversibility between the ZFC and FC branches is clearly observed below
 under 0.1 T. Like many rare earth-metal compounds,[94] Dy12Co7 may have strong anisotropy due to its large Dy content. It has been reported that in materials with high anisotropy energy and low exchange energy, the domain wall width could be comparable to that of lattice spacing, thus leading to a large pinning effect.[94] Considering the magnetic anisotropy and low
under 0.1 T. Like many rare earth-metal compounds,[94] Dy12Co7 may have strong anisotropy due to its large Dy content. It has been reported that in materials with high anisotropy energy and low exchange energy, the domain wall width could be comparable to that of lattice spacing, thus leading to a large pinning effect.[94] Considering the magnetic anisotropy and low
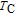 for Dy12Co7, the thermomagnetic irreversibility is likely attributed to the narrow domain wall pinning effect.[91] When the sample is cooled under a zero field, it freezes in an irregular domain structure where the direction of the spontaneous magnetization is only determined locally by the magnetic anisotropy.[91] When a small field is switched on and the sample is warmed up, the walls begin to move and in every domain the direction of magnetization turns into the energetically most favorable direction which now also depends on the direction of the applied field.[95] So we can see that the ZFC magnetization is low below
for Dy12Co7, the thermomagnetic irreversibility is likely attributed to the narrow domain wall pinning effect.[91] When the sample is cooled under a zero field, it freezes in an irregular domain structure where the direction of the spontaneous magnetization is only determined locally by the magnetic anisotropy.[91] When a small field is switched on and the sample is warmed up, the walls begin to move and in every domain the direction of magnetization turns into the energetically most favorable direction which now also depends on the direction of the applied field.[95] So we can see that the ZFC magnetization is low below
 and reaches a maximum at low temperature. Whereas in FC mode, the magnetic field during the cooling prevents the pinning effect, and therefore the magnetization at low temperature is higher than that in ZFC mode and gradually reaches saturation.[91] According to the M versus T characteristics, it is suggested that the Ho12Co7 compound undergoes three magnetic transitions in sequence with decreasing temperature. The transition at the highest temperature corresponds to a change from paramagnetic state to ferromagnetic state at
and reaches a maximum at low temperature. Whereas in FC mode, the magnetic field during the cooling prevents the pinning effect, and therefore the magnetization at low temperature is higher than that in ZFC mode and gradually reaches saturation.[91] According to the M versus T characteristics, it is suggested that the Ho12Co7 compound undergoes three magnetic transitions in sequence with decreasing temperature. The transition at the highest temperature corresponds to a change from paramagnetic state to ferromagnetic state at
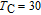 K. The rest two phase transitions are ferromagnetic to antiferromagnetic transition at
K. The rest two phase transitions are ferromagnetic to antiferromagnetic transition at
 K and antiferromagnetic to antiferromagnetic transition at
K and antiferromagnetic to antiferromagnetic transition at
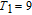 K, which will be confirmed by the isothermal magnetization curves.[18] The transition temperatures for Er12Co7 compound is determined to be 6.5 K and 13.5 K, respectively. All of the transition temperatures of R
12Co7 compounds are listed in Table 5.
K, which will be confirmed by the isothermal magnetization curves.[18] The transition temperatures for Er12Co7 compound is determined to be 6.5 K and 13.5 K, respectively. All of the transition temperatures of R
12Co7 compounds are listed in Table 5.
Table 5.
Table 5.
 Table 5.
The transition temperatures and magnetocaloric parameters of R
12Co7 (
 , Tb, Dy, Ho) and Ho
x
Er
, Tb, Dy, Ho) and Ho
x
Er
 Co7 (
Co7 (
 , 4, 6, 8, 10, 12) and Gd
, 4, 6, 8, 10, 12) and Gd
 Tb
x
Co7 (
Tb
x
Co7 (
 , 4, 8) compounds.
, 4, 8) compounds.
.
| Materials |
 /K
/K |
 /K
/K |
MCE_0–2 T |
MCE_0–5 T |
Ref. |
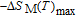 /(J/kg
/(J/kg
 K)
K) |
 /K
/K |
RC/(J/kg) |
 /(J/kg
/(J/kg
 K)
K) |
 /K
/K |
RC/(J/kg) |
| Gd12Co7
|
123 |
163/160.8 |
4.6 |
37.4 |
123.3 |
8.8 |
71.7 |
462.8 |
[90,92] |
| Gd8Tb4Co7
|
– |
140.8 |
– |
– |
– |
8.2 |
51.8 |
316 |
[92] |
| Gd4Tb8Co7
|
– |
118.9 |
– |
– |
– |
7.1 |
38.5 |
202.2 |
[92] |
| Tb12Co7
|
– |
100 |
3.1 |
8.7 |
20.5 |
– |
– |
– |
[89] |
| Dy12Co7
|
– |
64 |
5 |
21.7 |
81.5 |
10 |
42.1 |
303.7 |
[91] |
| Ho12Co7
|
9/17 |
30 |
9.2 |
29.4 |
206.2 |
19.2 |
37.4 |
555 |
[18] |
| Ho10Er2Co7
|
9/19 |
26.3 |
6.2 |
20.9 |
94.8 |
16.1 |
32.5 |
396 |
[93] |
| Ho8Er4Co7
|
11 |
22 |
7.8 |
22.8 |
137.8 |
17.0 |
31.8 |
422 |
[93] |
| Ho6Er6Co7
|
12 |
18 |
9.7 |
19.7 |
150.5 |
18.6 |
28.5 |
411 |
[93] |
| Ho4Er8Co7
|
8.6 |
15 |
9.6 |
16.5 |
122.6 |
18.0 |
24.7 |
342 |
[93] |
| Er12Co7
|
6.5 |
13.5 |
10.2 |
14.5 |
103.6 |
18.3 |
22.8 |
317 |
[93] |
| Ho6Er6Co7 42% |
| Ho12Co7 58% |
– |
– |
– |
– |
– |
16.7 |
38.0 |
553 |
[93] |
| Table 5.
The transition temperatures and magnetocaloric parameters of R
12Co7 (
 , Tb, Dy, Ho) and Ho
x
Er
, Tb, Dy, Ho) and Ho
x
Er
 Co7 (
Co7 (
 , 4, 6, 8, 10, 12) and Gd
, 4, 6, 8, 10, 12) and Gd
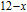 Tb
x
Co7 (
Tb
x
Co7 (
 , 4, 8) compounds.
, 4, 8) compounds.
. |
The M–T curves of Ho12Co7 compound under different external magnetic field are shown in Fig. 38. One can find that T
1 increases monotonically with magnetic field increasing from 0.01 T to 0.3 T. However, almost no change is observed for T
2 and
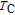 with magnetic field change. It indicates that a field-induced metamagnetic transition from AFM to FM state occurs below T
2, and T
1 is pushed towards higher temperature with the applied magnetic field increasing. Similar phenomenon has been observed in PrGa compound.[43] Figure 39(a) shows the isothermal magnetization curves of Ho12Co7 in a temperature range of 3 K–50 K under the magnetic fields up to 7 T. The isothermal magnetization curves in some selected temperature ranges at low magnetic fields are shown in Figs. 39(b), 39(c), and 39(d), respectively. One can find that the isothermal magnetization curves between
with magnetic field change. It indicates that a field-induced metamagnetic transition from AFM to FM state occurs below T
2, and T
1 is pushed towards higher temperature with the applied magnetic field increasing. Similar phenomenon has been observed in PrGa compound.[43] Figure 39(a) shows the isothermal magnetization curves of Ho12Co7 in a temperature range of 3 K–50 K under the magnetic fields up to 7 T. The isothermal magnetization curves in some selected temperature ranges at low magnetic fields are shown in Figs. 39(b), 39(c), and 39(d), respectively. One can find that the isothermal magnetization curves between
 K and
K and
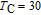 K, show FM characters (Fig. 39(d)). The magnetization remains a linear dependences of the magnetic field at low field range below
K, show FM characters (Fig. 39(d)). The magnetization remains a linear dependences of the magnetic field at low field range below
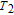 = 17 K (Figs. 39(b) and 39(c)), indicating the existence of AFM ground state.[18] Now we can confirm the transition around T
2 is corresponding to FM to AFM transition, and the one around T
1 is corresponding to AFM to AFM transition. The magnetization curves deviate from the linear relationship, when the applied field exceeds a certain value, showing a field-induced metamagnetic transition from AFM to FM state. The critical field determined from the maximum of
= 17 K (Figs. 39(b) and 39(c)), indicating the existence of AFM ground state.[18] Now we can confirm the transition around T
2 is corresponding to FM to AFM transition, and the one around T
1 is corresponding to AFM to AFM transition. The magnetization curves deviate from the linear relationship, when the applied field exceeds a certain value, showing a field-induced metamagnetic transition from AFM to FM state. The critical field determined from the maximum of
 for Ho12Co7 is found to be 0.12 T at 6 K and 0.28 T at 16 K.[18] The result indicates that the Ho12Co7 compound is a weak antiferromagnetic material below T
2, and a small magnetic field can destroy the AFM structure. One can also find that the isothermal magnetization curves obtained well above
for Ho12Co7 is found to be 0.12 T at 6 K and 0.28 T at 16 K.[18] The result indicates that the Ho12Co7 compound is a weak antiferromagnetic material below T
2, and a small magnetic field can destroy the AFM structure. One can also find that the isothermal magnetization curves obtained well above
 show strong curvatures at low fields from Fig. 39(a). Similar results have been observed in some other intermetallic compounds.[19,96,97] It may result from the existence of short-range ferromagnetic correlations in the PM state.
show strong curvatures at low fields from Fig. 39(a). Similar results have been observed in some other intermetallic compounds.[19,96,97] It may result from the existence of short-range ferromagnetic correlations in the PM state.
The S-shaped Arrott plots (not shown here) below T
2 confirm the existence of the field-induced first-order AFM–FM transition. The positive slope of the Arrott plot above
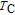 indicates a characteristic of a second-order PM–FM transition. The temperature dependences of the heat capacity in different magnetic fields are shown in Fig. 40. The peaks around 30 K, which are obvious for
indicates a characteristic of a second-order PM–FM transition. The temperature dependences of the heat capacity in different magnetic fields are shown in Fig. 40. The peaks around 30 K, which are obvious for
 T and 0.5 T, are corresponding to
T and 0.5 T, are corresponding to
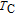 . It is found that there are obvious differences of heat capacity below T
2 for different magnetic field. The reason why the applied magnetic field affects heat capacity so greatly is that a magnetic transition from the weak AFM ground state to the FM state occurs. There is no obvious peak related to the AFM to AFM transition temperature for zero-field heat capacity curve, but an obvious peak around 13 K appears on the heat capacity curve for an applied field of 0.5 T.[18] As is illustrated in the M–T curves, the peak is corresponding to AFM to AFM transition and it has been pushed by the applied field from T
1 (
. It is found that there are obvious differences of heat capacity below T
2 for different magnetic field. The reason why the applied magnetic field affects heat capacity so greatly is that a magnetic transition from the weak AFM ground state to the FM state occurs. There is no obvious peak related to the AFM to AFM transition temperature for zero-field heat capacity curve, but an obvious peak around 13 K appears on the heat capacity curve for an applied field of 0.5 T.[18] As is illustrated in the M–T curves, the peak is corresponding to AFM to AFM transition and it has been pushed by the applied field from T
1 (
 K) to higher temperature (
K) to higher temperature (
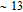 K). In the temperature range between T
2 and
K). In the temperature range between T
2 and
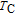 , the differences of heat capacity for different magnetic fields are not as clear as those in lower temperature range. That is because Ho12Co7 compound is FM state in this range, and the influence of applied field on heat capacity is small. The heat capacity data demonstrates that the statement of magnetic transition on the basis of M–T and M–H curves is reasonable.
, the differences of heat capacity for different magnetic fields are not as clear as those in lower temperature range. That is because Ho12Co7 compound is FM state in this range, and the influence of applied field on heat capacity is small. The heat capacity data demonstrates that the statement of magnetic transition on the basis of M–T and M–H curves is reasonable.
Tb12Co7 compound has been treated as a simple ferromagnetic material and it shows typical soft ferromagnetic features below
 =100 K. But from the isothermal magnetization (M–H) curves up to 5 T at 5 K and 80 K, which is shown in Fig. 41, it seems that Tb12Co7 compound shows weak AFM ground state at 5 K, which is very different from the FM type curve obtained at 80 K. Considering that Ho12Co7 compound with the same structure with Tb12Co7 compound undergoes complex magnetic transition as temperature increases, it is possible that Tb12Co7 compound undergoes another magnetic transition in low temperature range besides the FM to PM transition at
=100 K. But from the isothermal magnetization (M–H) curves up to 5 T at 5 K and 80 K, which is shown in Fig. 41, it seems that Tb12Co7 compound shows weak AFM ground state at 5 K, which is very different from the FM type curve obtained at 80 K. Considering that Ho12Co7 compound with the same structure with Tb12Co7 compound undergoes complex magnetic transition as temperature increases, it is possible that Tb12Co7 compound undergoes another magnetic transition in low temperature range besides the FM to PM transition at
 . The reason why the low-temperature transition was not observed according to M–T curves may be the AFM ground state in low temperature range is too weak. The temperature (T) dependences of magnetization (M) of Gd
. The reason why the low-temperature transition was not observed according to M–T curves may be the AFM ground state in low temperature range is too weak. The temperature (T) dependences of magnetization (M) of Gd
 Tb
x
Co7 (
Tb
x
Co7 (
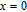 , 4, and 8) alloys under a 0.05-T applied field in zero-field cooling (ZFC) and field cooling (FC) modes are shown in Fig. 42. The Curie temperature
, 4, and 8) alloys under a 0.05-T applied field in zero-field cooling (ZFC) and field cooling (FC) modes are shown in Fig. 42. The Curie temperature
 , which is defined as the temperature at the maximum of
, which is defined as the temperature at the maximum of
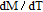 versus T plot, for Gd12Co7, Gd8Tb4Co7, and Gd4Tb8Co7 are 160.8, 140.8, and 118.9 K, respectively. All alloys undergo an FM to PM order transition. The magnetization changes rapidly with the change of temperature in the vicinity of
versus T plot, for Gd12Co7, Gd8Tb4Co7, and Gd4Tb8Co7 are 160.8, 140.8, and 118.9 K, respectively. All alloys undergo an FM to PM order transition. The magnetization changes rapidly with the change of temperature in the vicinity of
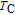 , indicating that a large entropy change may be observed near Curie temperature. Furthermore, a spin reorientation behavior with
, indicating that a large entropy change may be observed near Curie temperature. Furthermore, a spin reorientation behavior with
 =123 K is observed for the (Gd
=123 K is observed for the (Gd
 Tb
Tb
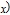 Co7 alloy with
Co7 alloy with
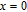 , which should result from the competition between magnetic exchange and the crystalline electric field.[98] It should be noted that no SR transition is observed on the M–T curve of Gd12Co7 compound according to Chen et al.[90] The FC and ZFC data branch out from each other in the vicinity of Tc, indicating thermomagnetic irreversibility for this alloy.
, which should result from the competition between magnetic exchange and the crystalline electric field.[98] It should be noted that no SR transition is observed on the M–T curve of Gd12Co7 compound according to Chen et al.[90] The FC and ZFC data branch out from each other in the vicinity of Tc, indicating thermomagnetic irreversibility for this alloy.
The zero-field-cooled (ZFC) and field-cooled (FC) magnetization curves for Ho
x
Er
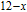 Co7 (
Co7 (
 , 4, 6, 8, 10, 12) compounds were measured[93] and the ZFC curves are shown in Fig. 43. The field dependence of magnetization (M–H) curves were also measured in detail for Ho
x
Er
, 4, 6, 8, 10, 12) compounds were measured[93] and the ZFC curves are shown in Fig. 43. The field dependence of magnetization (M–H) curves were also measured in detail for Ho
x
Er
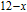 Co7 (
Co7 (
 , 4, 6, 8, 10, 12) compounds. For the Ho
x
Er
, 4, 6, 8, 10, 12) compounds. For the Ho
x
Er
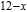 Co7 (
Co7 (
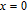 , 4, 6, 8) compounds, two drastic changes are observed at different temperature range on the M–T curves, which indicates that there are two magnetic transitions. M–H measurements show that the magnetic ground state of the three temperature zones divided by the two transition temperatures is antiferromagnetic (AFM), ferromagnetic (FM), and paramagnetic (PM), respectively. That is, Ho
x
Er
, 4, 6, 8) compounds, two drastic changes are observed at different temperature range on the M–T curves, which indicates that there are two magnetic transitions. M–H measurements show that the magnetic ground state of the three temperature zones divided by the two transition temperatures is antiferromagnetic (AFM), ferromagnetic (FM), and paramagnetic (PM), respectively. That is, Ho
x
Er
 Co7 (
Co7 (
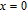 , 4, 6, 8) compounds undergo an AFM to FM transition and an FM to PM transition in sequence as temperature increases. The transition temperature (
, 4, 6, 8) compounds undergo an AFM to FM transition and an FM to PM transition in sequence as temperature increases. The transition temperature (
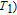 of AFM to FM transition is 6.5 K, 8.6 K, 12 K, and 11 K and the Curie temperature (
of AFM to FM transition is 6.5 K, 8.6 K, 12 K, and 11 K and the Curie temperature (
 is 13.5 K, 15 K, 18 K, and 22 K for Ho
x
Er
is 13.5 K, 15 K, 18 K, and 22 K for Ho
x
Er
 Co7 (
Co7 (
 , 4, 6, 8) compounds, respectively. All of the transition temperatures of Ho
x
Er
, 4, 6, 8) compounds, respectively. All of the transition temperatures of Ho
x
Er
 Co7 (
Co7 (
 , 4, 6, 8, 10, 12) compounds are listed in Table 5. From the results of transition temperature, it is found that as the content of Ho atoms increases, the number of transitions grows from two to three and the magnetic property becomes more and more complex. The transition at T
1, whether the AFM to FM transition for Ho
x
Er
, 4, 6, 8, 10, 12) compounds are listed in Table 5. From the results of transition temperature, it is found that as the content of Ho atoms increases, the number of transitions grows from two to three and the magnetic property becomes more and more complex. The transition at T
1, whether the AFM to FM transition for Ho
x
Er
 Co7 (
Co7 (
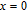 , 4, 6, 8) compounds or the AFM to AFM transition for Ho
x
Er
, 4, 6, 8) compounds or the AFM to AFM transition for Ho
x
Er
 Co7 (
Co7 (
 , 12) compounds, means the competition of two ordered magnetic states. From Table 5, the value of T
1 fluctuates not more than 5.5 K as the content of Ho atoms changes. That is because the competition is affected by crystal structure and crystal field, and the crystal structure of Ho
x
Er
, 12) compounds, means the competition of two ordered magnetic states. From Table 5, the value of T
1 fluctuates not more than 5.5 K as the content of Ho atoms changes. That is because the competition is affected by crystal structure and crystal field, and the crystal structure of Ho
x
Er
 Co7 (
Co7 (
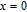 , 4, 6, 8, 10, 12) compounds is consistent with one another. The results are similar to the changing characteristics of spin reorientation transition temperature in Gd
x
Er
, 4, 6, 8, 10, 12) compounds is consistent with one another. The results are similar to the changing characteristics of spin reorientation transition temperature in Gd
x
Er
 Ga compounds.[34] However, the Curie temperature of Ho
x
Er
Ga compounds.[34] However, the Curie temperature of Ho
x
Er
 Co7 (
Co7 (
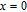 , 4, 6, 8, 10, 12) compounds shows a steadily increasing trend as the content of Ho atoms increases. That is because the Curie temperature and spin angular number (S) are positively correlated. Since the S of Ho is larger than Er, more Ho atoms means larger average S and thus the Curie temperature increases step by step as the content of Ho goes up.
, 4, 6, 8, 10, 12) compounds shows a steadily increasing trend as the content of Ho atoms increases. That is because the Curie temperature and spin angular number (S) are positively correlated. Since the S of Ho is larger than Er, more Ho atoms means larger average S and thus the Curie temperature increases step by step as the content of Ho goes up.
4.2. The magnetocaloric effect of R
12Co7 compoundsThe magnetic entropy change
 of R
12Co7 (
of R
12Co7 (
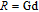 , Tb, Dy, Ho, Er) compounds can be calculated from isothermal magnetization data by using Maxwell relation
, Tb, Dy, Ho, Er) compounds can be calculated from isothermal magnetization data by using Maxwell relation
 .
.
Figure 44 displays the values of
 for R
12Co7 (
for R
12Co7 (
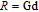 , Tb, Dy, Ho, Er) compounds as a function of temperature for a field change of 0 T–2 T. The maximum value of
, Tb, Dy, Ho, Er) compounds as a function of temperature for a field change of 0 T–2 T. The maximum value of
 for a field change of 0 T–2 T is calculated to be 4.6 J/kg
for a field change of 0 T–2 T is calculated to be 4.6 J/kg
 K, 3.1 J/kg
K, 3.1 J/kg
 K, 5J/kg
K, 5J/kg
 K, 9.2 J/kg
K, 9.2 J/kg
 K, and 10.2 J/kg
K, and 10.2 J/kg
 K for R
12Co7 (
K for R
12Co7 (
 , Tb, Dy, Ho, Er) compounds, respectively. The maximum value of
, Tb, Dy, Ho, Er) compounds, respectively. The maximum value of
 nearly shows an increase tendency as the R atom number increases. The change tendency of
nearly shows an increase tendency as the R atom number increases. The change tendency of
 is not as obvious as maximum of
is not as obvious as maximum of
 . All of the MCE parameters including MCE for 0 T–5 T are all listed in Table 5. For Dy12Co7 compound, the distinct “
. All of the MCE parameters including MCE for 0 T–5 T are all listed in Table 5. For Dy12Co7 compound, the distinct “
 ”-type of
”-type of
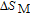 –T curves as well as the immovable position of the peak of
–T curves as well as the immovable position of the peak of
 with the increase of field change further confirms the SOPT (second-order phase transition) nature of Dy12Co7. The maximum values of
with the increase of field change further confirms the SOPT (second-order phase transition) nature of Dy12Co7. The maximum values of
 reach 5 J/kg
reach 5 J/kg
 K and 10.0 J/kg
K and 10.0 J/kg
 K for the field changes of 0 T–2 T and 0 T–5 T, respectively. These values are comparable with or larger than those of some magnetocaloric materials with a SOPT in a similar temperature range under the same field change. For example, for the field change of 0 T–5 T, the maximum values of TbCoAl (
K for the field changes of 0 T–2 T and 0 T–5 T, respectively. These values are comparable with or larger than those of some magnetocaloric materials with a SOPT in a similar temperature range under the same field change. For example, for the field change of 0 T–5 T, the maximum values of TbCoAl (
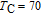 K) compound and Er(Co
K) compound and Er(Co
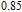 Si
Si
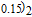 (
(
 K) compound are 10.5 J/kg
K) compound are 10.5 J/kg
 K and 8 J/kg
K and 8 J/kg
 K, respectively.[99,100] In addition, refrigerant capacity (RC), another important parameter to quantify the heat transferred between the hot and cold sinks in an ideal refrigeration cycle, is estimated by using the approach
K, respectively.[99,100] In addition, refrigerant capacity (RC), another important parameter to quantify the heat transferred between the hot and cold sinks in an ideal refrigeration cycle, is estimated by using the approach
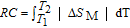 , where T
1 and T
2 are the temperatures corresponding to the both sides of half maximum value of
, where T
1 and T
2 are the temperatures corresponding to the both sides of half maximum value of
 peak.[53] Thus, the RC values of R
12Co7 compounds are obtained for the field changes of 0 T–2 T and 0 T–5 T, respectively.
peak.[53] Thus, the RC values of R
12Co7 compounds are obtained for the field changes of 0 T–2 T and 0 T–5 T, respectively.
The
 of Ho12Co7 was calculated from isothermal magnetization data by using the Maxwell relation
of Ho12Co7 was calculated from isothermal magnetization data by using the Maxwell relation
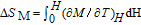 and from heat capacity data through the expression
and from heat capacity data through the expression
 as well.[50] Figure 45(a) shows the
as well.[50] Figure 45(a) shows the
 as a function of temperature for different magnetic field changes. Figure 45(b) shows the comparison of
as a function of temperature for different magnetic field changes. Figure 45(b) shows the comparison of
 curves obtained from the two different ways. The curves obtained using magnetization data are not in good agreement with the corresponding curves obtained using heat capacity. Considering that the actual combined relative error in the
curves obtained from the two different ways. The curves obtained using magnetization data are not in good agreement with the corresponding curves obtained using heat capacity. Considering that the actual combined relative error in the
 calculated from magnetization data can reach
calculated from magnetization data can reach
 % and that from heat capacity data can be
% and that from heat capacity data can be
 %,[3,50] the deviations between the value of
%,[3,50] the deviations between the value of
 calculated from the two methods are in the error range with the maximal error being
calculated from the two methods are in the error range with the maximal error being
 17% and
17% and
 11%, for the field change of 0 T–2 T and 0 T–5 T, respectively. Although there are differences between the curves obtained from the two methods, the shape of the curves matches well with each other. It is clear that there are two peaks on the
11%, for the field change of 0 T–2 T and 0 T–5 T, respectively. Although there are differences between the curves obtained from the two methods, the shape of the curves matches well with each other. It is clear that there are two peaks on the
 –T curves, which indicates that both AFM–FM and PM–FM transitions contribute to MCE. Figure 45(c) shows that when the field change is small, for example 0 T–0.2 T, the value of
–T curves, which indicates that both AFM–FM and PM–FM transitions contribute to MCE. Figure 45(c) shows that when the field change is small, for example 0 T–0.2 T, the value of
 is positive in certain temperature range, and as the field change increasing, the value of
is positive in certain temperature range, and as the field change increasing, the value of
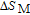 changes into negative. That results from the occurrence of metamagnetic transition, because the positive or negative value of
changes into negative. That results from the occurrence of metamagnetic transition, because the positive or negative value of
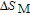 is related to AFM or FM state. Besides, with the field change increasing, the first peak corresponding to AFM–FM transition moves towards higher temperatures, while the second peak related to PM–FM transition almost centers at
is related to AFM or FM state. Besides, with the field change increasing, the first peak corresponding to AFM–FM transition moves towards higher temperatures, while the second peak related to PM–FM transition almost centers at
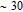 K, which is in accordance with the results of M–T and C
p
–T measurements. When the field change is high enough, for example 0 T–2 T and 0 T–5 T, the first peak is so close to the second one that they almost change into a larger peak. On the basis of magnetization measurement, the maximal value of
K, which is in accordance with the results of M–T and C
p
–T measurements. When the field change is high enough, for example 0 T–2 T and 0 T–5 T, the first peak is so close to the second one that they almost change into a larger peak. On the basis of magnetization measurement, the maximal value of
 for Ho12Co7 is found to be 19.2 J/kg
for Ho12Co7 is found to be 19.2 J/kg
 K around
K around
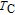 for a field change 0 T–5 T. Compared with the refrigerant materials in a similar temperature range, the value of Ho12Co7 compound is smaller than that of ErGa (21.3 J/kg
for a field change 0 T–5 T. Compared with the refrigerant materials in a similar temperature range, the value of Ho12Co7 compound is smaller than that of ErGa (21.3 J/kg
 K at 30 K),[19] DyCuAl (20.4 J/kg
K at 30 K),[19] DyCuAl (20.4 J/kg
 K at 28 K),[16] and ErCo2 (33 J/kg
K at 28 K),[16] and ErCo2 (33 J/kg
 K at 36 K)[101] compounds, but it is larger than that of DyNiAl (19 J/kg
K at 36 K)[101] compounds, but it is larger than that of DyNiAl (19 J/kg
 K at 32 K),[102] TbCoC
2 (15.3 J/kg
K at 32 K),[102] TbCoC
2 (15.3 J/kg
 K at 30 K),[103] and GdNi5 (11.5 J/kg
K at 30 K),[103] and GdNi5 (11.5 J/kg
 K at 32 K).[104]
K at 32 K).[104]
One can also see from Fig. 45(b) that both the peak value and peak width of
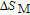 –T curves depend on the magnetic field change. When the field change is 0 T–0.5 T, the maximum value of the two peaks are small and they are far apart from each other. However, when the applied field increases to 2 T and 5 T, the two peaks become one large peak. That is to say, both of the maximal value and the width of the peak have an obvious increase. The peak shape is very helpful to improvement of the value of RC in Ho12Co7 compound. The RC value of Ho12Co7 is estimated to be 206.2 J/kg with
–T curves depend on the magnetic field change. When the field change is 0 T–0.5 T, the maximum value of the two peaks are small and they are far apart from each other. However, when the applied field increases to 2 T and 5 T, the two peaks become one large peak. That is to say, both of the maximal value and the width of the peak have an obvious increase. The peak shape is very helpful to improvement of the value of RC in Ho12Co7 compound. The RC value of Ho12Co7 is estimated to be 206.2 J/kg with
 K (temperature of the cold reservoir) and
K (temperature of the cold reservoir) and
 K (temperature of the hot reservoir) for a field changing from 0 T to 2 T. And the RC value is estimated to be 554.9 J/kg with
K (temperature of the hot reservoir) for a field changing from 0 T to 2 T. And the RC value is estimated to be 554.9 J/kg with
 K and
K and
 K for a field changing from 0 T to 5 T. As a result, Ho12Co7 compound has an outstanding refrigerant capacity among the magnetocaloric materials in a similar temperature range, such as ErGa (
K for a field changing from 0 T to 5 T. As a result, Ho12Co7 compound has an outstanding refrigerant capacity among the magnetocaloric materials in a similar temperature range, such as ErGa (
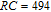 J/kg with
J/kg with
 K and
K and
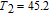 K),[19] DyCuAl (
K),[19] DyCuAl (
 J/kg with
J/kg with
 K and
K and
 K),[16] ErCo2 (
K),[16] ErCo2 (
 J/kg with
J/kg with
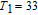 K and
K and
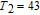 K),[101] DyNiAl (
K),[101] DyNiAl (
 J/kg with
J/kg with
 K and
K and
 K),[102] TbCoC
2 (RC= 354 J/kg with
K),[102] TbCoC
2 (RC= 354 J/kg with
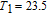 K and
K and
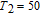 K),[103] and GdNi5 (
K),[103] and GdNi5 (
 J/kg with
J/kg with
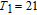 K and
K and
 K),[104] although ErGa, DyCuAl, and ErCo2 exhibit larger
K),[104] although ErGa, DyCuAl, and ErCo2 exhibit larger
 values. Here, the RC values of some compounds are estimated from the temperature dependence of
values. Here, the RC values of some compounds are estimated from the temperature dependence of
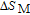 in the literatures, respectively. The large value of
in the literatures, respectively. The large value of
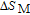 and RC suggests that Ho12Co7 can be an appropriate candidate for magnetic refrigerant in low temperature ranges.
and RC suggests that Ho12Co7 can be an appropriate candidate for magnetic refrigerant in low temperature ranges.
Figure 46 shows the temperature dependencies of
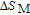 under the applied magnetic field change from 0 T to 5 T. And the inset of Fig. 46 shows the
under the applied magnetic field change from 0 T to 5 T. And the inset of Fig. 46 shows the
 curves of Tb12Co7 compound under the field change of 0 T–1 T and 0 T–2 T, respectively. The peak values of
curves of Tb12Co7 compound under the field change of 0 T–1 T and 0 T–2 T, respectively. The peak values of
 are found to be 8.8, 8.2, and 7.1 J/kg
are found to be 8.8, 8.2, and 7.1 J/kg
 K for the alloys with
K for the alloys with
 , 4, and 8, respectively.[92] The RC values are calculated to be 478.4 J/kg, 327.2 J/kg, and 160.2J/kg for Gd
, 4, and 8, respectively.[92] The RC values are calculated to be 478.4 J/kg, 327.2 J/kg, and 160.2J/kg for Gd
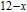 Tb
x
Co7 alloys with
Tb
x
Co7 alloys with
 , 4, and 8, respectively.[92] It should be noted that no obvious peak is found around the
, 4, and 8, respectively.[92] It should be noted that no obvious peak is found around the
 of Gd12Co7 compound. There are two possible reasons for the absence of the peak in low temperature range: firstly, the magnetic measurement was not carried out as low as possible; secondly, the SR transition is too weak.
of Gd12Co7 compound. There are two possible reasons for the absence of the peak in low temperature range: firstly, the magnetic measurement was not carried out as low as possible; secondly, the SR transition is too weak.
The
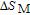 (T) curves of Ho
x
Er
(T) curves of Ho
x
Er
 Co7 (
Co7 (
 , 4, 6, 8, 10, 12) compounds for a field change of 0 T–2 T and 0 T–5 T are shown in Fig. 47, respectively.[93] The maximum value of
, 4, 6, 8, 10, 12) compounds for a field change of 0 T–2 T and 0 T–5 T are shown in Fig. 47, respectively.[93] The maximum value of
 for each Ho
x
Er
for each Ho
x
Er
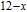 Co7 (
Co7 (
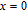 , 4, 6, 8, 10, 12) compounds is listed in Table 5. Two peaks are observed obviously on the
, 4, 6, 8, 10, 12) compounds is listed in Table 5. Two peaks are observed obviously on the
 curve of Ho12Co7 compound. Actually, this kind of multi-peaks
curve of Ho12Co7 compound. Actually, this kind of multi-peaks
 curves has been observed in many magnetocaloric materials with several transitions, such as ErGa, HoNi, TbGa, DyGa, etc.[19,32,78] For other Ho
x
Er
curves has been observed in many magnetocaloric materials with several transitions, such as ErGa, HoNi, TbGa, DyGa, etc.[19,32,78] For other Ho
x
Er
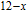 Co7 compounds, only one peak around
Co7 compounds, only one peak around
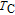 can be observed and in low temperature zone there is a small convex instead of a peak. For Er12Co7 compound the small convex can even hardly be observed.
can be observed and in low temperature zone there is a small convex instead of a peak. For Er12Co7 compound the small convex can even hardly be observed.
Another important parameter to evaluate the magnetocaloric materials is refrigerant capacity (RC) and it has been also calculated. All of the parameters,
 , RC, and
, RC, and
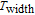 , are listed in Table 5. As the content of Ho atoms goes up, the
, are listed in Table 5. As the content of Ho atoms goes up, the
 of Ho
x
Er
of Ho
x
Er
 Co7 (
Co7 (
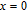 , 4, 6, 8, 10, 12) compounds increases steadily. The change characteristics of
, 4, 6, 8, 10, 12) compounds increases steadily. The change characteristics of
 are closely related to the transition temperatures. According to the analysis of transition temperatures, T
1 changes slightly and
are closely related to the transition temperatures. According to the analysis of transition temperatures, T
1 changes slightly and
 increases rapidly as the content of Ho atoms goes up. Therefore,
increases rapidly as the content of Ho atoms goes up. Therefore,
 shows an increase with the content of Ho atoms. The RC shows a similar changing trend to
shows an increase with the content of Ho atoms. The RC shows a similar changing trend to
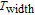 , but Ho10Er2Co7 compound is an exception. Though the maximum of
, but Ho10Er2Co7 compound is an exception. Though the maximum of
 is not so large, the
is not so large, the
 and RC show good performance for Ho
x
Er
and RC show good performance for Ho
x
Er
 Co7 (
Co7 (
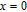 , 4, 6, 8, 10, 12) compounds.
, 4, 6, 8, 10, 12) compounds.
For the actual application of magnetocaloric materials in refrigerators, the materials with table-like
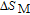 curves have more important value. That is because a much wider temperature range of cooling can be realized by this kind of magnetocaloric materials. The magnetocaloric materials with a plateau on
curves have more important value. That is because a much wider temperature range of cooling can be realized by this kind of magnetocaloric materials. The magnetocaloric materials with a plateau on
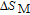 curve are so useful that people have made efforts to design them by mixing different kinks of materials, such as R
3Ni2 and RFeSi.[105,106] Considering that each Ho
x
Er
curve are so useful that people have made efforts to design them by mixing different kinks of materials, such as R
3Ni2 and RFeSi.[105,106] Considering that each Ho
x
Er
 Co7 (
Co7 (
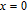 , 4, 6, 8, 10, 12) compound shows advantages on MCE at different temperature zones, the Ho
x
Er
, 4, 6, 8, 10, 12) compound shows advantages on MCE at different temperature zones, the Ho
x
Er
 Co7 compounds are mixed at different weight proportions with each two as one group. It is found that when Ho12Co7 and Ho6Er6Co7 compounds are combined at the weight ratio of 58:42, the mixed magnetocaloric material shows the best performance (Fig. 48). The maximum of
Co7 compounds are mixed at different weight proportions with each two as one group. It is found that when Ho12Co7 and Ho6Er6Co7 compounds are combined at the weight ratio of 58:42, the mixed magnetocaloric material shows the best performance (Fig. 48). The maximum of
 is 16.7 J/kg
is 16.7 J/kg
 K, and the
K, and the
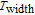 and RC are as high as 38 K and 553 J/kg, respectively. The RC of newly obtained material is comparable to Ho12Co7 compound and the
and RC are as high as 38 K and 553 J/kg, respectively. The RC of newly obtained material is comparable to Ho12Co7 compound and the
 is even better. The most important is that there is a plateau on the
is even better. The most important is that there is a plateau on the
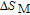 curve, which means that the mixed compounds of Ho12Co7 and Ho6Er6Co7 with a weight ratio of 58:42 has a larger using value. The excellent MCE performance indicates its potential use for cooling in the temperature range around 20 K–30 K.
curve, which means that the mixed compounds of Ho12Co7 and Ho6Er6Co7 with a weight ratio of 58:42 has a larger using value. The excellent MCE performance indicates its potential use for cooling in the temperature range around 20 K–30 K.
5. The
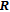 3Co compounds
3Co compoundsIn recent years, much attention has been paid to the rare-earth (R)-based intermetallic compounds with a low temperature phase transition for the purpose of magnetic refrigerant application.[18,107–109] Among the R-based compounds, the binary
 (
(
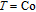 and Ni) series with the highest rare-earth content is a subject of special attention. Although these compounds have identical crystallographic structures, they exhibit complex magnetic structures and possess different magnetic-phase transitions, which will induce interesting magnetocaloric properties.[49] The magnetic properties of R
3Co (
and Ni) series with the highest rare-earth content is a subject of special attention. Although these compounds have identical crystallographic structures, they exhibit complex magnetic structures and possess different magnetic-phase transitions, which will induce interesting magnetocaloric properties.[49] The magnetic properties of R
3Co (
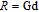 , Tb, Dy, Ho, Er) compounds were also studied by the magnetization, electrical resistivity, and NMR measurements.[110] The magnetic ordering temperatures were obtained from the electrical resistivity measurement and the complex magnetic susceptibilities were explained by the metamagnetic transition under an applied field.[110] Specific heat measurements were performed in wide temperature range T = 2 K–300 K for the R
3Co (
, Tb, Dy, Ho, Er) compounds were also studied by the magnetization, electrical resistivity, and NMR measurements.[110] The magnetic ordering temperatures were obtained from the electrical resistivity measurement and the complex magnetic susceptibilities were explained by the metamagnetic transition under an applied field.[110] Specific heat measurements were performed in wide temperature range T = 2 K–300 K for the R
3Co (
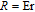 –Gd, Y) compounds. Using values of the Sommerfeld coefficient and Debye temperature estimated for Y3Co compound, the Debye temperatures, phonon and conduction electron contributions as well as magnetic contribution to the specific heat were estimated for all other R
3Co compounds.[111]
–Gd, Y) compounds. Using values of the Sommerfeld coefficient and Debye temperature estimated for Y3Co compound, the Debye temperatures, phonon and conduction electron contributions as well as magnetic contribution to the specific heat were estimated for all other R
3Co compounds.[111]
Based on magnetization, magnetoresistance, a.c. susceptibility, and specific heat, magnetic phase diagrams of the compound Dy3Co were obtained for the main crystallographic axis.[112] The different AFM structures exist below
 K: AFM1 exists at
K: AFM1 exists at
 K and AFM2 exists at the temperatures between
K and AFM2 exists at the temperatures between
 and
and
 .[112] The field-induced magnetic phase transitions observed along the three main axes are associated with a complex non-collinear AFM structure and are accompanied by a significant change in the electrical resistivity.[112] Electrical resistivity and calorimetric measurements on single crystal Dy3Co show that below
.[112] The field-induced magnetic phase transitions observed along the three main axes are associated with a complex non-collinear AFM structure and are accompanied by a significant change in the electrical resistivity.[112] Electrical resistivity and calorimetric measurements on single crystal Dy3Co show that below
 K the non-collinear antiferromagnetic structure exhibits field-induced magnetic phase transitions of a first-order type along all principal axes, accompanied by a strongly anisotropic giant magnetoresistance and by a change of the Sommerfeld coefficient of the specific heat.[113] The neutron elastic scattering experiments were used to determine the structural parameters and comparative analysis of the possible magnetic structures in the ground state of Dy3Co.[114]
K the non-collinear antiferromagnetic structure exhibits field-induced magnetic phase transitions of a first-order type along all principal axes, accompanied by a strongly anisotropic giant magnetoresistance and by a change of the Sommerfeld coefficient of the specific heat.[113] The neutron elastic scattering experiments were used to determine the structural parameters and comparative analysis of the possible magnetic structures in the ground state of Dy3Co.[114]
The single crystal Er3Co compound has been investigated by means of magnetic susceptibility, magnetization, electrical resistivity, and heat capacity measurements.[115] Complex non-collinear ferromagnetic structure was observed below 13.8 K. Using the magnetization and magnetoresistance measurements along the main crystallographic directions, a complicated and anisotropic magnetization process was revealed.[115] Magnetic and magnetocaloric properties of polycrystalline samples of Gd3Co have been studied. The Gd3Co compound is antiferromagnets with
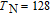 K and it undergoes metamagnetic transition in the AFM phase. The maximum values of isothermal magnetic entropy change are 11 J/kg
K and it undergoes metamagnetic transition in the AFM phase. The maximum values of isothermal magnetic entropy change are 11 J/kg
 K.[116] The magnetic and transport properties of single crystal Tb3Co compound were studied. The orthorhombic Tb3Co compound exhibits both FM and AFM behaviors below the critical temperature
K.[116] The magnetic and transport properties of single crystal Tb3Co compound were studied. The orthorhombic Tb3Co compound exhibits both FM and AFM behaviors below the critical temperature
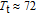 K as evidenced by metamagnetic transitions along the a and b axes and ferromagnetic magnetization process along the c axis.[117] Field-induced transitions along all three axes are observed between
K as evidenced by metamagnetic transitions along the a and b axes and ferromagnetic magnetization process along the c axis.[117] Field-induced transitions along all three axes are observed between
 and
and
 (82 K).[117] And the metamagnetic transitions are accompanied by a significant magnetoresistance effect.[117] The measurements of the magnetization in high steady and pulsed fields together with neutron diffraction measurements on a powder sample and on a single crystal have been performed to study the magnetic state of the Tb3Co compound.[118] It has been shown that the modulated antiferromagnetic structure which exists in Tb3Co below
(82 K).[117] And the metamagnetic transitions are accompanied by a significant magnetoresistance effect.[117] The measurements of the magnetization in high steady and pulsed fields together with neutron diffraction measurements on a powder sample and on a single crystal have been performed to study the magnetic state of the Tb3Co compound.[118] It has been shown that the modulated antiferromagnetic structure which exists in Tb3Co below
 K transforms to the incommensurate magnetic structure with a strong ferromagnetic component along the c axis with further cooling below the critical temperature
K transforms to the incommensurate magnetic structure with a strong ferromagnetic component along the c axis with further cooling below the critical temperature
 K. The phase transition from the high-temperature to the low-temperature magnetic state at
K. The phase transition from the high-temperature to the low-temperature magnetic state at
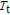 is of the first order.[118]
is of the first order.[118]
A large reversible magnetocaloric effect has been observed in Tb3Co compound. Under a magnetic field change of 5 T, the maximum value of magnetic entropy change
 is
is
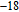 J/kg
J/kg
 K at 84 K and the relative cooling power is 738 J/kg with no hysteresis loss.[119] Magnetic properties and magnetocaloric effect of the Dy3Co compound are studied. The maximal value of magnetic entropy change
K at 84 K and the relative cooling power is 738 J/kg with no hysteresis loss.[119] Magnetic properties and magnetocaloric effect of the Dy3Co compound are studied. The maximal value of magnetic entropy change
 is
is
 J/kg
J/kg
 K with a refrigerant capacity (RC) of 498 J/kg around
K with a refrigerant capacity (RC) of 498 J/kg around
 for a field change of 0 T–5 T.[49] And the magnetic properties and magnetocaloric effects of Ho3Co, Er3Co, Tm3Co, and Er
for a field change of 0 T–5 T.[49] And the magnetic properties and magnetocaloric effects of Ho3Co, Er3Co, Tm3Co, and Er
 Gd
x
Co compounds have also been studied in detail.[120–124] In the following section, the above contents especially MCEs will be reviewed. And the data of Tb3Co compound is cited from literature.[119]
Gd
x
Co compounds have also been studied in detail.[120–124] In the following section, the above contents especially MCEs will be reviewed. And the data of Tb3Co compound is cited from literature.[119]
5.1. The magnetic properties of
 3Co compounds and
3Co compounds and
 Gd
x
Co compounds
Gd
x
Co compoundsPolycrystalline R
3Co (
 , Tb, Dy, Ho, Er, Tm) and Er
, Tb, Dy, Ho, Er, Tm) and Er
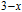 Gd
x
Co (
Gd
x
Co (
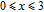 ) compounds were prepared by arc melting in a high-purity argon atmosphere. The purities of starting materials were 99.9% for R and Co. The sample was turned over and remelted several times to ensure its homogeneity. The ingot obtained by arc melting was wrapped by molybdenum foil, sealed in a quartz tube of high vacuum, annealed at 873 K for 4–8 days and then quenched to room temperature.[49,119] X-ray diffraction (XRD) measurements on powder samples were performed by using CuKα radiation to identify the crystal structure. Magnetizations were measured as functions of temperature and magnetic field by using a superconducting quantum interference device (SQUID) magnetometer and vibrate sample magnetometer (VSM). Both the ac susceptibility and the specific heat were measured by using a physical property measurement system (PPMS) from Quantum Design. The powder XRD patterns of R
3Co (
) compounds were prepared by arc melting in a high-purity argon atmosphere. The purities of starting materials were 99.9% for R and Co. The sample was turned over and remelted several times to ensure its homogeneity. The ingot obtained by arc melting was wrapped by molybdenum foil, sealed in a quartz tube of high vacuum, annealed at 873 K for 4–8 days and then quenched to room temperature.[49,119] X-ray diffraction (XRD) measurements on powder samples were performed by using CuKα radiation to identify the crystal structure. Magnetizations were measured as functions of temperature and magnetic field by using a superconducting quantum interference device (SQUID) magnetometer and vibrate sample magnetometer (VSM). Both the ac susceptibility and the specific heat were measured by using a physical property measurement system (PPMS) from Quantum Design. The powder XRD patterns of R
3Co (
 , Tb, Dy, Ho, Er, Tm) and Er
, Tb, Dy, Ho, Er, Tm) and Er
 Gd
x
Co (
Gd
x
Co (
 ) compounds obtained at room temperature were refined by the Rietveld method. The refinement shows that the prepared sample is of single phase, crystallizing in the orthorhombic Fe3C-type crystal structure (space group Pnma #62). The crystal structure of R
3Co compounds is shown in Fig. 49. R atoms occupy two nonequivalent positions [
) compounds obtained at room temperature were refined by the Rietveld method. The refinement shows that the prepared sample is of single phase, crystallizing in the orthorhombic Fe3C-type crystal structure (space group Pnma #62). The crystal structure of R
3Co compounds is shown in Fig. 49. R atoms occupy two nonequivalent positions [
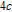 (
(
 ) and
) and
 (
(
 )] and Co atoms are located at the
)] and Co atoms are located at the
 position within the trigonal prisms formed by Tm atoms. Results of all the structural parameters are determined by the Rietveld technique using GSAS program.[123] For Er
position within the trigonal prisms formed by Tm atoms. Results of all the structural parameters are determined by the Rietveld technique using GSAS program.[123] For Er
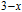 Gd
x
Co (
Gd
x
Co (
 ) compounds, with increasing Gd concentration, the diffraction peaks move to the left and the unit cell volume increases linearly. It is attributed to the contraction law of rare-earth intermetallic compound (the bigger atomic radius of the Gd atom than Er).[122]
) compounds, with increasing Gd concentration, the diffraction peaks move to the left and the unit cell volume increases linearly. It is attributed to the contraction law of rare-earth intermetallic compound (the bigger atomic radius of the Gd atom than Er).[122]
The temperature dependences of zero-field-cooling (ZFC) and field-cooling (FC) magnetization (
 for Tb3Co, Ho3Co, Er3Co, and Tm3Co compounds were measured and they are shown in Fig. 50(b), Fig. 50(d), Fig. 50(e), and Fig. 50(f), respectively. The applied field is different and the field is 0.005 T, 0.01 T, 0.05 T, and 0.01 T for Tb3Co, Ho3Co, Er3Co, and Tm3Co compounds, respectively. The ZFC curves of Gd3Co and Dy3Co compounds are shown in Fig. 50(a) and Fig. 50(c), respectively and the applied field is 0.01 T. Although the crystal structure of R
3Co (
for Tb3Co, Ho3Co, Er3Co, and Tm3Co compounds were measured and they are shown in Fig. 50(b), Fig. 50(d), Fig. 50(e), and Fig. 50(f), respectively. The applied field is different and the field is 0.005 T, 0.01 T, 0.05 T, and 0.01 T for Tb3Co, Ho3Co, Er3Co, and Tm3Co compounds, respectively. The ZFC curves of Gd3Co and Dy3Co compounds are shown in Fig. 50(a) and Fig. 50(c), respectively and the applied field is 0.01 T. Although the crystal structure of R
3Co (
 , Tb, Dy, Ho, Er, Tm) compounds is the same, the magnetic properties of them differs from each other a lot. Results show that Gd3Co is a simple AFM material and it undergoes an AFM to PM transition at
, Tb, Dy, Ho, Er, Tm) compounds is the same, the magnetic properties of them differs from each other a lot. Results show that Gd3Co is a simple AFM material and it undergoes an AFM to PM transition at
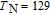 K as temperature increases.[122] According to neutron diffraction investigation by Baranov et al.,[118] Tb3Co undergoes two successive transitions at temperatures
K as temperature increases.[122] According to neutron diffraction investigation by Baranov et al.,[118] Tb3Co undergoes two successive transitions at temperatures
 =72 K (FM to AFM transition) and
=72 K (FM to AFM transition) and
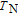 =82 K (AFM to PM transition), corresponding to the maximum slopes in the M–T curve (Fig. 50(b)). The neutron diffraction investigation suggested that the incommensurate FM state, at
=82 K (AFM to PM transition), corresponding to the maximum slopes in the M–T curve (Fig. 50(b)). The neutron diffraction investigation suggested that the incommensurate FM state, at
 for a first-order magnetic phase transition, develops to a modulated AFM state that exists below
for a first-order magnetic phase transition, develops to a modulated AFM state that exists below
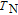 .[118] On the other hand, reversible behavior above 50 K in ZFC and FC curves at a magnetic field of 1 T (not shown here) indicates that Tb3Co experiences a second-order FM to PM phase transition at
.[118] On the other hand, reversible behavior above 50 K in ZFC and FC curves at a magnetic field of 1 T (not shown here) indicates that Tb3Co experiences a second-order FM to PM phase transition at
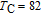 K.[119] Arrott plots also confirm its second-order nature. It can be deduced that a small magnetic field, less than 1 T, can induce a metamagnetic transition from AFM to FM state.[119] It is found that the Ho3Co compound undergoes two successive magnetic phase transitions at low temperatures (see Fig. 50(d)), corresponding to the two maximal values of M–T curve. As revealed by the neutron diffraction studies,[125] the Ho3Co compound exhibits a PM to AFM transition at
K.[119] Arrott plots also confirm its second-order nature. It can be deduced that a small magnetic field, less than 1 T, can induce a metamagnetic transition from AFM to FM state.[119] It is found that the Ho3Co compound undergoes two successive magnetic phase transitions at low temperatures (see Fig. 50(d)), corresponding to the two maximal values of M–T curve. As revealed by the neutron diffraction studies,[125] the Ho3Co compound exhibits a PM to AFM transition at
 K, followed by a spin–reorientation transition at
K, followed by a spin–reorientation transition at
 K. The anomaly at about 30 K in the M–T curve may be attributed to the existence of a minor amount of impurity phase, because no anomaly of magnetization at the same temperature was observed based on the results reported by Baranov et al.[126] and Nagai et al.
[110]
K. The anomaly at about 30 K in the M–T curve may be attributed to the existence of a minor amount of impurity phase, because no anomaly of magnetization at the same temperature was observed based on the results reported by Baranov et al.[126] and Nagai et al.
[110]
It can be seen from Fig. 50(e) that Er3Co undergoes a magnetic transition from FM to PM states, and its Curie temperature
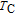 is determined to be 13 K, which is in good agreement with the value reported by Saito et al.[127] In addition, it is also observed that there is no hysteresis in ZFC and FC curves above
is determined to be 13 K, which is in good agreement with the value reported by Saito et al.[127] In addition, it is also observed that there is no hysteresis in ZFC and FC curves above
 . Below
. Below
 , the difference between the ZFC and FC curves may be related to the domain-wall pinning effect.[121] It can be seen that there is almost no thermal hysteresis between the ZFC and FC curves for Tm3Co compound when the temperature increases above 6.5 K from Fig. 50(f). The small bifurcation may be related to the domain-wall pinning effect below 6.5 K. The
, the difference between the ZFC and FC curves may be related to the domain-wall pinning effect.[121] It can be seen that there is almost no thermal hysteresis between the ZFC and FC curves for Tm3Co compound when the temperature increases above 6.5 K from Fig. 50(f). The small bifurcation may be related to the domain-wall pinning effect below 6.5 K. The
 versus T curve reveals that the Tm3Co undergoes an AFM to FM transition at
versus T curve reveals that the Tm3Co undergoes an AFM to FM transition at
 K and an FM to PM transition at
K and an FM to PM transition at
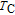 = 6.5 K, which is corresponding to the minimum value of
= 6.5 K, which is corresponding to the minimum value of
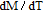 .[123] The temperature dependence of specific heat (
.[123] The temperature dependence of specific heat (
 ) in a zero-field for Dy3Co was measured and is shown in Fig. 51. The magnetic property and magnetic transition of Dy3Co compound will be discussed based on Fig. 50(c) and Fig. 51. The results show that Dy3Co has two successive magnetic transitions as reported by Baranov et al. previously.[112] The anomaly at lower temperature may be associated with the phase transition from one AFM to another AFM state.[112] The transition temperature (
) in a zero-field for Dy3Co was measured and is shown in Fig. 51. The magnetic property and magnetic transition of Dy3Co compound will be discussed based on Fig. 50(c) and Fig. 51. The results show that Dy3Co has two successive magnetic transitions as reported by Baranov et al. previously.[112] The anomaly at lower temperature may be associated with the phase transition from one AFM to another AFM state.[112] The transition temperature (
 K), determined by the position of the low-temperature peak on the specific heat curve, is fully consistent with the value derived from the
K), determined by the position of the low-temperature peak on the specific heat curve, is fully consistent with the value derived from the
 –T curve.[49] The transition at higher temperature corresponds to a change from AFM to PM state as temperature increases. The Neel temperature
–T curve.[49] The transition at higher temperature corresponds to a change from AFM to PM state as temperature increases. The Neel temperature
 is determined to be 44 K, which is in good agreement with the previous results.[112,113] The temperature dependences of the magnetization at different magnetic fields have also been measured. It is observed that the magnetic fields can drive magnetic transition temperatures
is determined to be 44 K, which is in good agreement with the previous results.[112,113] The temperature dependences of the magnetization at different magnetic fields have also been measured. It is observed that the magnetic fields can drive magnetic transition temperatures
 and
and
 to lower temperature and a field-induced metamagnetic transition from AFM to FM state is present below
to lower temperature and a field-induced metamagnetic transition from AFM to FM state is present below
 .[49] When the applied magnetic field is higher than about 1.2 T, the magnetization as a function of temperature for Dy3Co exhibits step-like behavior above
.[49] When the applied magnetic field is higher than about 1.2 T, the magnetization as a function of temperature for Dy3Co exhibits step-like behavior above
 , which corresponds to the FM to PM transition.[49]
, which corresponds to the FM to PM transition.[49]
The temperature (T) dependences of magnetization (M) data measured in an applied field of 0.01 T under zero-field-cooling (ZFC) and field-cooling (FC) conditions for Er
 Gd
x
Co (
Gd
x
Co (
 , 0.5, 1, 1.5, 2, 2.5, 3) compounds are shown in Fig. 52. The compound Er3Co shows an FM-like transition at 13 K due to the thermomagnetic irreversibility between ZFC and FC curves.[122] It is obvious that the compound with
, 0.5, 1, 1.5, 2, 2.5, 3) compounds are shown in Fig. 52. The compound Er3Co shows an FM-like transition at 13 K due to the thermomagnetic irreversibility between ZFC and FC curves.[122] It is obvious that the compound with
 shows two successive magnetic transitions at
shows two successive magnetic transitions at
 K which shows the transition from PM to AFM, and
K which shows the transition from PM to AFM, and
 K which coincides to the transition from AFM to FM-like order.[122] However, two transition behaviors seem to disappear instead of a magnetic plateau emerges below
K which coincides to the transition from AFM to FM-like order.[122] However, two transition behaviors seem to disappear instead of a magnetic plateau emerges below
 for the compounds with
for the compounds with
 . Only a sharp AFM transition is observed and
. Only a sharp AFM transition is observed and
 linearly shifts to higher temperature with x.[122] No thermal hysteresis is observed between ZFC and FC curves around
linearly shifts to higher temperature with x.[122] No thermal hysteresis is observed between ZFC and FC curves around
 for the compounds with
for the compounds with
 , which is a characteristic of the second-order magnetic transition.[122]
, which is a characteristic of the second-order magnetic transition.[122]
All of the magnetic transition temperatures of R
3Co (
 , Tb, Dy, Ho, Er, Tm) and Er
, Tb, Dy, Ho, Er, Tm) and Er
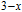 Gd
x
Co (
Gd
x
Co (
 , 0.5, 1, 1.5, 2, 2.5, 3) compounds have been listed in Table 6. From the table it can be seen that the magnetic property and magnetic transition for R
3Co compound are different from each other. It undergoes two magnetic transitions as temperature increases for Tb3Co, Dy3Co, Ho3Co, Tm3Co, and Er
, 0.5, 1, 1.5, 2, 2.5, 3) compounds have been listed in Table 6. From the table it can be seen that the magnetic property and magnetic transition for R
3Co compound are different from each other. It undergoes two magnetic transitions as temperature increases for Tb3Co, Dy3Co, Ho3Co, Tm3Co, and Er
 Gd
Gd
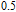 Co compounds. It undergoes only one magnetic transition as temperature increases for Gd3Co, Er3Co, Er2Gd1Co, Er
Co compounds. It undergoes only one magnetic transition as temperature increases for Gd3Co, Er3Co, Er2Gd1Co, Er
 Gd
Gd
 Co, Er1Gd2Co, and Er
Co, Er1Gd2Co, and Er
 Gd
Gd
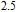 Co compounds. The Curie temperature or Neel temperature decreases as the rare earth atom number increases for R
3Co (
Co compounds. The Curie temperature or Neel temperature decreases as the rare earth atom number increases for R
3Co (
 , Tb, Dy, Ho, Er, Tm) compounds. That is because the spin angular moment quantum number (S) decreases as atom number increases for Lanthanum series and S is positively correlated with order-disorder transition temperature. With the same reason, it is found that the order–disorder transition temperature increases as the content of Gd atoms in Er
, Tb, Dy, Ho, Er, Tm) compounds. That is because the spin angular moment quantum number (S) decreases as atom number increases for Lanthanum series and S is positively correlated with order-disorder transition temperature. With the same reason, it is found that the order–disorder transition temperature increases as the content of Gd atoms in Er
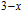 Gd
x
Co (
Gd
x
Co (
 , 0.5, 1, 1.5, 2, 2.5, 3) compounds.
, 0.5, 1, 1.5, 2, 2.5, 3) compounds.
5.2. The magnetocaloric effects of
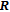 3Co compounds and
3Co compounds and
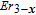 Gd
x
Co compounds
Gd
x
Co compoundsThe temperature dependences of
 for R
3Co (
for R
3Co (
 , Tb, Dy, Ho, Er, Tm) compounds calculated by using Maxwell relation formula under a magnetic field change of 0 T–2 T and 0 T–5 T are shown in Fig. 53(a) and Fig. 53(b), respectively.
, Tb, Dy, Ho, Er, Tm) compounds calculated by using Maxwell relation formula under a magnetic field change of 0 T–2 T and 0 T–5 T are shown in Fig. 53(a) and Fig. 53(b), respectively.
For Tb3Co compound, the maximum of
 is
is
 ,
,
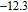 ,
,
 , and
, and
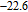 J/kg
J/kg
 K, respectively, for the magnetic field changes of 2, 3, 5, and 7 T.[119] The large
K, respectively, for the magnetic field changes of 2, 3, 5, and 7 T.[119] The large
 ,
,
 J/kg
J/kg
 K achieved for a low magnetic field change of 2 T, is beneficial to application.[119] The peaks of
K achieved for a low magnetic field change of 2 T, is beneficial to application.[119] The peaks of
 at 84 K are just corresponding to the FM to PM phase transition. It is clearly observed that the
at 84 K are just corresponding to the FM to PM phase transition. It is clearly observed that the
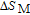 –T curves of Dy3Co exhibit two maximum values at 29 K and 44 K, respectively, which corresponds to the two transitions at
–T curves of Dy3Co exhibit two maximum values at 29 K and 44 K, respectively, which corresponds to the two transitions at
 and
and
 . The maximum value of
. The maximum value of
 for Dy3Co is found to be
for Dy3Co is found to be
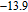 J/kg
J/kg
 K around
K around
 for a magnetic-field change from 0 T to 5 T.[49] It is apparent that the large
for a magnetic-field change from 0 T to 5 T.[49] It is apparent that the large
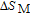 in Dy3Co is associated with the field-induced metamagnetic transition below
in Dy3Co is associated with the field-induced metamagnetic transition below
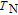 . The refrigerant capacity (RC) of Dy3Co compound has been also calculated by numerically integrating the area under the
. The refrigerant capacity (RC) of Dy3Co compound has been also calculated by numerically integrating the area under the
 –T curve, with the temperatures at half maximum of the peak used as the integration limits.[53] The RC value thus obtained for Dy3Co is 498 J/kg with
–T curve, with the temperatures at half maximum of the peak used as the integration limits.[53] The RC value thus obtained for Dy3Co is 498 J/kg with
 K (temperature of the cold reservoir) and
K (temperature of the cold reservoir) and
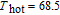 K (temperature of the hot reservoir) for a field changing from 0 T to 5 T.[49] The large RC originates from the combined contribution of two successive magnetic transitions, which enlarge the temperature span of large MCE. The full width at half maximum of the
K (temperature of the hot reservoir) for a field changing from 0 T to 5 T.[49] The large RC originates from the combined contribution of two successive magnetic transitions, which enlarge the temperature span of large MCE. The full width at half maximum of the
 peak under a magnetic field of 5 T attains
peak under a magnetic field of 5 T attains
 K
K
 for Dy3Co due to the superposition of the AFM-to-AFM and AFM-to-PM phase transitions.[49]
for Dy3Co due to the superposition of the AFM-to-AFM and AFM-to-PM phase transitions.[49]
The values of
 for Ho3Co are positive at temperatures below 7 K and at lower magnetic field, but they change to a negative value with the increase of applied field due to the field-induced magnetic transition from AFM to FM state.[120] The positive
for Ho3Co are positive at temperatures below 7 K and at lower magnetic field, but they change to a negative value with the increase of applied field due to the field-induced magnetic transition from AFM to FM state.[120] The positive
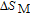 (negative MCE) is often observed in magnetocaloric materials with the first order magnetic transitions,[9] which results from the mixed exchange interaction, and the applied magnetic field leads to a further spin-disordered state near the transition temperature, thereby increasing the entropy.[10] A small positive value of
(negative MCE) is often observed in magnetocaloric materials with the first order magnetic transitions,[9] which results from the mixed exchange interaction, and the applied magnetic field leads to a further spin-disordered state near the transition temperature, thereby increasing the entropy.[10] A small positive value of
 also indicates a weak AFM coupling in Ho3Co at low temperatures. It can be seen from Fig. 53 that the peak of the
also indicates a weak AFM coupling in Ho3Co at low temperatures. It can be seen from Fig. 53 that the peak of the
 –T curve of Ho3Co shows a broad distribution due to its successive magnetic transitions. The maximal values of
–T curve of Ho3Co shows a broad distribution due to its successive magnetic transitions. The maximal values of
 for Ho3Co are found to be
for Ho3Co are found to be
 J/kg
J/kg
 K and
K and
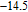 J/kg
J/kg
 K for field changes of 0 T–2 T and 0 T–5 T, respectively.[120] The large
K for field changes of 0 T–2 T and 0 T–5 T, respectively.[120] The large
 results from the high saturation magnetization and is caused by the field-induced AFM-to-FM metamagnetic transition.
results from the high saturation magnetization and is caused by the field-induced AFM-to-FM metamagnetic transition.
For Er3Co compound, both the peak and the width of
 depend on the applied magnetic field, and increase obviously with increasing field. The temperature dependences of
depend on the applied magnetic field, and increase obviously with increasing field. The temperature dependences of
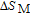 exhibit a
exhibit a
 -shape profile as shown usually in magnetic materials with a second-order magnetic transition. A giant
-shape profile as shown usually in magnetic materials with a second-order magnetic transition. A giant
 , which results from a second-order FM-to-PM transition, is observed in Er3Co around
, which results from a second-order FM-to-PM transition, is observed in Er3Co around
 . The maximal value of
. The maximal value of
 for Er3Co is found to increase monotonously with applied magnetic field and reaches a value of 24.5 J/kg
for Er3Co is found to increase monotonously with applied magnetic field and reaches a value of 24.5 J/kg
 K at 16 K for a field change of 0 T–5 T.[121] It is interesting to note that a large reversible
K at 16 K for a field change of 0 T–5 T.[121] It is interesting to note that a large reversible
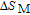 for Er3Co is as high as 12.5 J/kg
for Er3Co is as high as 12.5 J/kg
 K for a field change of 0 T–2 T, which is beneficial to the practical applications because a magnetic field of 2 T can be supplied by a permanent magnet.[121] Calculations show that the maximal value of RC for Er3Co is 476 J/kg for a magnetic field change of 0 T–5 T. In particular, a high value of RC is also obtained to be 163 J/kg for a relatively low field change of 0 T–2 T.[121] This is attributed to the appreciably large values of
K for a field change of 0 T–2 T, which is beneficial to the practical applications because a magnetic field of 2 T can be supplied by a permanent magnet.[121] Calculations show that the maximal value of RC for Er3Co is 476 J/kg for a magnetic field change of 0 T–5 T. In particular, a high value of RC is also obtained to be 163 J/kg for a relatively low field change of 0 T–2 T.[121] This is attributed to the appreciably large values of
 obtained at
obtained at
 for Er3Co.
for Er3Co.
The maximum value of
 for Tm3Co compound is found to increase monotonically with the increase of applied magnetic field and reaches a value of 19.9 J/kg
for Tm3Co compound is found to increase monotonically with the increase of applied magnetic field and reaches a value of 19.9 J/kg
 K for a magnetic field change from 0 T to 5 T. In particular, the maximum value of
K for a magnetic field change from 0 T to 5 T. In particular, the maximum value of
 is found to be 11.6 J/kg
is found to be 11.6 J/kg
 K under a low magnetic change of 2 T, which can be realized by permanent magnet and be advantageous for applications.[123] The large
K under a low magnetic change of 2 T, which can be realized by permanent magnet and be advantageous for applications.[123] The large
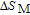 of Tm3Co compound origins from the field-induced metamagnetic transition from AFM to FM. Moreover, the
of Tm3Co compound origins from the field-induced metamagnetic transition from AFM to FM. Moreover, the
 does not die out even at temperatures well above
does not die out even at temperatures well above
 , possibly due to the presence of spin fluctuations.[124] All of the MCE parameters for R
3Co (
, possibly due to the presence of spin fluctuations.[124] All of the MCE parameters for R
3Co (
 , Tb, Dy, Ho, Er, Tm) compounds are listed in Table 6.
, Tb, Dy, Ho, Er, Tm) compounds are listed in Table 6.
The
 –T curves of Er
–T curves of Er
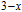 Gd
x
Co (
Gd
x
Co (
 –3) compounds under magnetic field changes of 0 T–5 T are calculated and shown in Fig. 54. And the related MCE parameters are also listed in Table 6. It can be noted from the
–3) compounds under magnetic field changes of 0 T–5 T are calculated and shown in Fig. 54. And the related MCE parameters are also listed in Table 6. It can be noted from the
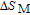 –T curves that
–T curves that
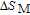 does not die out even at temperature well above the
does not die out even at temperature well above the
 . This may be attributed to the short range magnetic correlations associated with spin fluctuations.[124] Since the Er3Co compound undergoes a magnetic transition from PM to FM state, and at the same situation, the Gd3Co undergoes a magnetic transition from PM to AFM state, the interaction between the Er3Co and Gd3Co results in a spin-reorientation transition at lower temperature.[122] Therefore, two obvious peaks of Er2Gd1Co can be clearly visualized. The values of
. This may be attributed to the short range magnetic correlations associated with spin fluctuations.[124] Since the Er3Co compound undergoes a magnetic transition from PM to FM state, and at the same situation, the Gd3Co undergoes a magnetic transition from PM to AFM state, the interaction between the Er3Co and Gd3Co results in a spin-reorientation transition at lower temperature.[122] Therefore, two obvious peaks of Er2Gd1Co can be clearly visualized. The values of
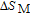 for Er
for Er
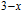 Gd
x
Co are negative at relative low temperatures and low magnetic field, and the values are changed to positive with the increase of applied field.
Gd
x
Co are negative at relative low temperatures and low magnetic field, and the values are changed to positive with the increase of applied field.
Take the Er
 Gd
Gd
 Co for instance, figure 55 shows that the
Co for instance, figure 55 shows that the
 curve of Er
curve of Er
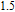 Gd
Gd
 Co is negative below the transition temperature for a low magnetic field change. When the value of
Co is negative below the transition temperature for a low magnetic field change. When the value of
 is negative, the negative value first decreases and then increases with the increase of magnetic field. It indicates the magnetic transition from AFM to FM states.[122] The
is negative, the negative value first decreases and then increases with the increase of magnetic field. It indicates the magnetic transition from AFM to FM states.[122] The
 changes to a positive value with the magnetic field increasing, which is corresponding to the magnetic transition from FM to PM states. A large magnetic entropy change results from the field-induced metamagnetic transition of FM to PM for Er
changes to a positive value with the magnetic field increasing, which is corresponding to the magnetic transition from FM to PM states. A large magnetic entropy change results from the field-induced metamagnetic transition of FM to PM for Er
 Gd
Gd
 Co.[122] This phenomenon results from the mixed exchange interaction, and the applied magnetic field leads to a further spin-disordered state near the transition temperature, thereby enlarges entropy.[10,122] It is observed that the
Co.[122] This phenomenon results from the mixed exchange interaction, and the applied magnetic field leads to a further spin-disordered state near the transition temperature, thereby enlarges entropy.[10,122] It is observed that the
 decreases from 24.6 J/kg
decreases from 24.6 J/kg
 K (
K (
 ) to 9.8 J/kg
) to 9.8 J/kg
 K (
K (
 ) with x, then increases from 9.8 J/kg
) with x, then increases from 9.8 J/kg
 K (
K (
 ) to 11.1 J/kg
) to 11.1 J/kg
 K (
K (
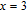 ) for a field change of 0 T–5 T.[122] However, the RC shows a contrasting behavior. The RC value increases with x showing a maximum value of 624.5 J/kg for 0 T–5 T at intermediate content of
) for a field change of 0 T–5 T.[122] However, the RC shows a contrasting behavior. The RC value increases with x showing a maximum value of 624.5 J/kg for 0 T–5 T at intermediate content of
 , which is 30% and 23.6% higher than that of Er3Co and Gd3Co, respectively.[122] Then, the RC value decreases with x above 1.5. This phenomenon is attributed to the trend of temperature span with x based on the definition of RC. It shows a widest temperature span of 83.2 K of
, which is 30% and 23.6% higher than that of Er3Co and Gd3Co, respectively.[122] Then, the RC value decreases with x above 1.5. This phenomenon is attributed to the trend of temperature span with x based on the definition of RC. It shows a widest temperature span of 83.2 K of
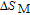 in Er
in Er
 Gd
Gd
 Co compound due to the most preferred chemical proportion with intermediate substitution of
Co compound due to the most preferred chemical proportion with intermediate substitution of
 .[122]
.[122]
6. The
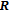 Cu2 compounds
Cu2 compoundsIn recent years, the rare-earth (R) based intermetallic compounds with a low temperature phase transition have drawn much attention for the applications in low-temperature magnetic refrigeration. RCu2 series are one of the important categories of R-based intermetallic compounds and the magnetic properties of RCu2 (
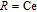 , Pr, Nd, Sm, Eu, Gd, Tb, Dy, Ho, Er, Tm, Yb, and Lu) compounds have been investigated by Sherwood et al.
[128] Especially, the magnetic properties of the single crystal samples have been studied in detail.[129–132] Much work has also been done on the specific heat, magnetoresistance, magnetostriction in RCu2 compounds.[132–137] The magnetic structures of some RCu2 compounds were determined by employing Neutron diffraction experiments.[138–140] The ground state for most RCu2 compounds is AFM state and field-induced metamagnetic transition was observed with a critical magnetic field.[128] More than one magnetic transitions were observed in HoCu2 compounds, and the transition temperatures were determined to be 7.4 K and 10.5 K, respectively.[133,136,138] The MCE of HoCu2 and DyCu2 compounds have been studied. A large effective refrigerant capacity of 194 J/kg below 44 K was obtained in DyCu2 compound, and a maximum magnetic entropy change of 19.3 J/kg
, Pr, Nd, Sm, Eu, Gd, Tb, Dy, Ho, Er, Tm, Yb, and Lu) compounds have been investigated by Sherwood et al.
[128] Especially, the magnetic properties of the single crystal samples have been studied in detail.[129–132] Much work has also been done on the specific heat, magnetoresistance, magnetostriction in RCu2 compounds.[132–137] The magnetic structures of some RCu2 compounds were determined by employing Neutron diffraction experiments.[138–140] The ground state for most RCu2 compounds is AFM state and field-induced metamagnetic transition was observed with a critical magnetic field.[128] More than one magnetic transitions were observed in HoCu2 compounds, and the transition temperatures were determined to be 7.4 K and 10.5 K, respectively.[133,136,138] The MCE of HoCu2 and DyCu2 compounds have been studied. A large effective refrigerant capacity of 194 J/kg below 44 K was obtained in DyCu2 compound, and a maximum magnetic entropy change of 19.3 J/kg
 K with a relative cooling power of 268 J/kg was found in HoCu2 compound.[97,141] In this work, the RCu2 (
K with a relative cooling power of 268 J/kg was found in HoCu2 compound.[97,141] In this work, the RCu2 (
 , Tb, Dy, Ho, Er) compounds were synthesized successfully.[142] The magnetic properties and magnetocaloric effect were investigated in detail. Though the MCEs of DyCu2 and HoCu2 compounds have been studied, the two compounds are still fabricated and investigated newly.
, Tb, Dy, Ho, Er) compounds were synthesized successfully.[142] The magnetic properties and magnetocaloric effect were investigated in detail. Though the MCEs of DyCu2 and HoCu2 compounds have been studied, the two compounds are still fabricated and investigated newly.
6.1. The crystal structure and magnetic properties of
 Cu2 compounds
Cu2 compoundsBinary polycrystalline RCu2 (
 , Tb, Dy, Ho, Er) compounds were fabricated by arc-melting method and powder x-ray diffraction (XRD) was performed to confirm their phase purity. The room temperature XRD patterns of RCu2 (
, Tb, Dy, Ho, Er) compounds were fabricated by arc-melting method and powder x-ray diffraction (XRD) was performed to confirm their phase purity. The room temperature XRD patterns of RCu2 (
 , Tb, Dy, Ho, Er) compounds are all shown in Fig. 56. Almost all of the diffraction peaks can be indexed to an orthorhombic crystal structure (space group Imma #74). The result is in accord with previous work.[140] The Bragg positions are marked at the bottom of the figure. It can also be seen that there is a small impure peak at 31.7
, Tb, Dy, Ho, Er) compounds are all shown in Fig. 56. Almost all of the diffraction peaks can be indexed to an orthorhombic crystal structure (space group Imma #74). The result is in accord with previous work.[140] The Bragg positions are marked at the bottom of the figure. It can also be seen that there is a small impure peak at 31.7
 for ErCu2 compound, which indicates that small amount of impurity may exist. However, that does not affect the discussion in the following section because the purity is negligible. That is to say, almost all the obtained RCu2 (
for ErCu2 compound, which indicates that small amount of impurity may exist. However, that does not affect the discussion in the following section because the purity is negligible. That is to say, almost all the obtained RCu2 (
 , Tb, Dy, Ho, Er) compounds are pure single phase.
, Tb, Dy, Ho, Er) compounds are pure single phase.
The lattice parameters were determined by using Rietveld refinement method. For example, the lattice constant of HoCu2 compound is calculated as follows:
 Å,
Å,
 Å, and
Å, and
 Å. As the atom number of R increases, the position of peak moves towards higher angle range. It indicates that the lattice constant becomes smaller and smaller from GdCu2 to ErCu2 compound. It has been described as follows: the RCu2 compounds crystallize in orthorhombic CeCu2-type structure, which can be considered as a stacking of alternating layers of R and Cu atoms along c axis of crystal.[140] The crystal structure of RCu2 compounds is shown in Fig. 57. There are four R atoms in form of one parallelogram along with two triangles and eight Cu atoms in form of two triangular prisms in the unit cell.
Å. As the atom number of R increases, the position of peak moves towards higher angle range. It indicates that the lattice constant becomes smaller and smaller from GdCu2 to ErCu2 compound. It has been described as follows: the RCu2 compounds crystallize in orthorhombic CeCu2-type structure, which can be considered as a stacking of alternating layers of R and Cu atoms along c axis of crystal.[140] The crystal structure of RCu2 compounds is shown in Fig. 57. There are four R atoms in form of one parallelogram along with two triangles and eight Cu atoms in form of two triangular prisms in the unit cell.
The temperature dependences of zero-field-cooled (ZFC) and field-cooled (FC) magnetization were measured under a field of 0.02 T for the RCu2 (
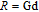 , Tb, Dy, Ho, Er) compounds.[142] And the ZFC and FC curves are shown in Fig. 58. As temperature increases, GdCu2 compound undergoes only one AFM to PM magnetic transition and the Neel temperature is determined to be 40.5 K. As temperature increases, TbCu2 compound undergoes an AFM to AFM transition and an AFM to PM transition at 23.5 K and 49.5 K, respectively. DyCu2 compound undergoes an AFM to AFM transition and an AFM to PM transition at 18.5 K and 31.5 K, respectively. HoCu2 compound undergoes an AFM to AFM transition and an AFM to PM transition at 7 K and 10 K, respectively. ErCu2 compound undergoes an AFM to AFM transition and an AFM to PM transition at 6 K and 12 K, respectively. The transition temperatures are in accord with previous reports.[128,136,138,141] All the transition temperatures for RCu2 (
, Tb, Dy, Ho, Er) compounds.[142] And the ZFC and FC curves are shown in Fig. 58. As temperature increases, GdCu2 compound undergoes only one AFM to PM magnetic transition and the Neel temperature is determined to be 40.5 K. As temperature increases, TbCu2 compound undergoes an AFM to AFM transition and an AFM to PM transition at 23.5 K and 49.5 K, respectively. DyCu2 compound undergoes an AFM to AFM transition and an AFM to PM transition at 18.5 K and 31.5 K, respectively. HoCu2 compound undergoes an AFM to AFM transition and an AFM to PM transition at 7 K and 10 K, respectively. ErCu2 compound undergoes an AFM to AFM transition and an AFM to PM transition at 6 K and 12 K, respectively. The transition temperatures are in accord with previous reports.[128,136,138,141] All the transition temperatures for RCu2 (
 , Tb, Dy, Ho, Er) compounds are shown in Table 7. It can be seen that the magnetic property and magnetic transition for RCu2 (
, Tb, Dy, Ho, Er) compounds are shown in Table 7. It can be seen that the magnetic property and magnetic transition for RCu2 (
 , Tb, Dy, Ho, Er) compounds is very complex and interesting. Firstly, the magnetic transition shows its multiformity for RCu2 (
, Tb, Dy, Ho, Er) compounds is very complex and interesting. Firstly, the magnetic transition shows its multiformity for RCu2 (
 , Tb, Dy, Ho, Er) compounds. GdCu2 compound shows only one magnetic transition, while others show two magnetic transitions. Secondly, the Neel temperatures seems not correlated to spin or atom number. From Gd to Er, the atom number increases monotonously. But the Neel temperature does not change monotonously from GdCu2 to ErCu2. Thirdly, all the ground states of RCu2 (
, Tb, Dy, Ho, Er) compounds. GdCu2 compound shows only one magnetic transition, while others show two magnetic transitions. Secondly, the Neel temperatures seems not correlated to spin or atom number. From Gd to Er, the atom number increases monotonously. But the Neel temperature does not change monotonously from GdCu2 to ErCu2. Thirdly, all the ground states of RCu2 (
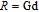 , Tb, Dy, Ho, Er) compounds are AFM ordered, and this property may bring special magnetic performances on other aspects.
, Tb, Dy, Ho, Er) compounds are AFM ordered, and this property may bring special magnetic performances on other aspects.
Table 7.
Table 7.
 | Table 7.
The transition temperatures and magnetocaloric effect parameters of RCu2 (
 , Tb, Dy, Ho, Er) compounds.
, Tb, Dy, Ho, Er) compounds.
. |
The relationship between the reciprocal magnetic susceptibility (
 and temperature was also obtained from M–T curves. All of the magnetic susceptibility of RCu2 (
and temperature was also obtained from M–T curves. All of the magnetic susceptibility of RCu2 (
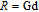 , Tb, Dy, Ho, Er) compounds can be fitted to the Curie–Weiss law. The effective magnetic moment
, Tb, Dy, Ho, Er) compounds can be fitted to the Curie–Weiss law. The effective magnetic moment
 per R atom was calculated by the linear fitting of
per R atom was calculated by the linear fitting of
 –T. The specific value of
–T. The specific value of
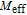 for each RCu2 compound is shown in Table 7. And for comparison, the theory value is listed as well. It can be seen that the
for each RCu2 compound is shown in Table 7. And for comparison, the theory value is listed as well. It can be seen that the
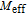 is in good accord with theory value of R ions. It can also be seen that the ZFC and FC curves overlap for most RCu2 (
is in good accord with theory value of R ions. It can also be seen that the ZFC and FC curves overlap for most RCu2 (
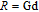 , Tb, Dy, Ho, Er) compounds except DyCu2 compound. It indicates that RCu2 series exhibit good heat reversibility. And the divarication between ZFC and FC in DyCu2 compound may result from domain wall pinning effect.[19] It has been mentioned that the ground state is AFM for the most RCu2 (
, Tb, Dy, Ho, Er) compounds except DyCu2 compound. It indicates that RCu2 series exhibit good heat reversibility. And the divarication between ZFC and FC in DyCu2 compound may result from domain wall pinning effect.[19] It has been mentioned that the ground state is AFM for the most RCu2 (
 , Tb, Dy, Ho, Er) compounds. However, the AFM coupling is weak for some compounds but strong for others. The isothermal magnetization at low temperature up to 7 T is shown in Fig. 59 for GdCu2 and TbCu2, respectively. As magnetic field increases, both GdCu2 and TbCu2 compounds undergo metamagnetic transition. And the critical field (
, Tb, Dy, Ho, Er) compounds. However, the AFM coupling is weak for some compounds but strong for others. The isothermal magnetization at low temperature up to 7 T is shown in Fig. 59 for GdCu2 and TbCu2, respectively. As magnetic field increases, both GdCu2 and TbCu2 compounds undergo metamagnetic transition. And the critical field (
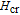 ) can be calculated by the maximum of
) can be calculated by the maximum of
 . The
. The
 of GdCu2 and TbCu2 compounds is determined to be 2 T and 5.6 T, respectively. Considering that the available magnetic field in regular lab conditions is 2 T or so, GdCu2 compound has little research value in magnetocaloric effect. Therefore, the magnetocaloric effect will be investigated on TbCu2, DyCu2, HoCu2 and ErCu2 compounds.
of GdCu2 and TbCu2 compounds is determined to be 2 T and 5.6 T, respectively. Considering that the available magnetic field in regular lab conditions is 2 T or so, GdCu2 compound has little research value in magnetocaloric effect. Therefore, the magnetocaloric effect will be investigated on TbCu2, DyCu2, HoCu2 and ErCu2 compounds.
6.2. The magnetocaloric effect of
 Cu2 compounds
Cu2 compoundsThe
 of RCu2 (
of RCu2 (
 , Dy, Ho, Er) compounds was calculated based on the isothermal magnetization data (not shown here) by using the Maxwell relation.[142] The
, Dy, Ho, Er) compounds was calculated based on the isothermal magnetization data (not shown here) by using the Maxwell relation.[142] The
 curves as a function of temperature for RCu2 (
curves as a function of temperature for RCu2 (
 , Dy, Ho, Er) compounds with a field change of 0 T–7 T are shown in Fig. 60. The inset of Fig. 60 is the low-temperature part of
, Dy, Ho, Er) compounds with a field change of 0 T–7 T are shown in Fig. 60. The inset of Fig. 60 is the low-temperature part of
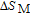 curve for TbCu2 compound. The position of
curve for TbCu2 compound. The position of
 peaks are closely correlated to the disorder–order transition temperatures of each RCu2 (
peaks are closely correlated to the disorder–order transition temperatures of each RCu2 (
 , Dy, Ho, Er) compound. Thus the
, Dy, Ho, Er) compound. Thus the
 curves for HoCu2 and ErCu2 center at lower temperature and the
curves for HoCu2 and ErCu2 center at lower temperature and the
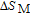 curves for TbCu2 and DyCu2 center at higher temperatures. Among all the RCu2 (
curves for TbCu2 and DyCu2 center at higher temperatures. Among all the RCu2 (
 , Dy, Ho, Er) compounds, the maximum of
, Dy, Ho, Er) compounds, the maximum of
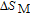 for HoCu2 compound shows the largest value and that for TbCu2 compound shows the smallest value. Though ErCu2, HoCu2, DyCu2, and TbCu2 compounds all undergo two magnetic transitions, only TbCu2 compound shows more than one peaks on the
for HoCu2 compound shows the largest value and that for TbCu2 compound shows the smallest value. Though ErCu2, HoCu2, DyCu2, and TbCu2 compounds all undergo two magnetic transitions, only TbCu2 compound shows more than one peaks on the
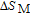 curve. It should be noted that the secondary peak for TbCu2 compound is positive
curve. It should be noted that the secondary peak for TbCu2 compound is positive
 . Positive
. Positive
 has been observed in many AFM MCE materials and the positive value in AFM ordering is considered to be due to disordered magnetic sublattices antiparallel to the applied magnetic field.[48,49] All the RCu2 (
has been observed in many AFM MCE materials and the positive value in AFM ordering is considered to be due to disordered magnetic sublattices antiparallel to the applied magnetic field.[48,49] All the RCu2 (
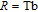 , Dy, Ho, Er) compounds are AFM ordered, but most of them does not show positive
, Dy, Ho, Er) compounds are AFM ordered, but most of them does not show positive
 because AFM ordering is so weak that a field of 7 T has destroyed it. The
because AFM ordering is so weak that a field of 7 T has destroyed it. The
 of TbCu2 compound for a field change of 0 T–7 T showing positive value means that the AFM ordering here is very strong. Furthermore, the positive refrigerant temperature width (
of TbCu2 compound for a field change of 0 T–7 T showing positive value means that the AFM ordering here is very strong. Furthermore, the positive refrigerant temperature width (
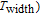 is so large that, the secondary peak is higher than the main peak. For TbCu2 compound, the maximum of negative
is so large that, the secondary peak is higher than the main peak. For TbCu2 compound, the maximum of negative
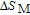 is 11.5 J/kg
is 11.5 J/kg
 K, but the maximum of positive
K, but the maximum of positive
 is 13.9 J/kg
is 13.9 J/kg
 K.
K.
Refrigeration capacity (RC) and refrigerant temperature width (
 are important parameters for MCE materials as well. The RC can be calculated on the basis of, where T
1 and T
2 are the two ends of the half-maximum width of the
are important parameters for MCE materials as well. The RC can be calculated on the basis of, where T
1 and T
2 are the two ends of the half-maximum width of the
 –T curve, and
–T curve, and
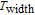 is defined as T
2–T
1. The maximum of
is defined as T
2–T
1. The maximum of
 for TbCu2, DyCu2, HoCu2, and ErCu2 compounds is calculated to be 11.5 J/kg
for TbCu2, DyCu2, HoCu2, and ErCu2 compounds is calculated to be 11.5 J/kg
 K, 14.8 J/kg
K, 14.8 J/kg
 K, 21.5 J/kg
K, 21.5 J/kg
 K, and 13.7 J/kg
K, and 13.7 J/kg
 K, respectively. The
K, respectively. The
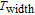 for TbCu2, DyCu2, HoCu2, and ErCu2 compounds is calculated to be 39.2 K, 33 K, 20.1 K, and 21.8 K, respectively. The RC for TbCu2, DyCu2, HoCu2, and ErCu2 compounds is calculated to be 339 J/kg, 365 J/kg, 330 J/kg, and 226.5 J/kg, respectively. All of the MCE parameters are also listed in Table 7. It should be noted that the RC for TbCu2 compound is determined only by the negative
for TbCu2, DyCu2, HoCu2, and ErCu2 compounds is calculated to be 39.2 K, 33 K, 20.1 K, and 21.8 K, respectively. The RC for TbCu2, DyCu2, HoCu2, and ErCu2 compounds is calculated to be 339 J/kg, 365 J/kg, 330 J/kg, and 226.5 J/kg, respectively. All of the MCE parameters are also listed in Table 7. It should be noted that the RC for TbCu2 compound is determined only by the negative
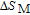 part. Since the complex magnetic properties of RCu2 compounds, (
part. Since the complex magnetic properties of RCu2 compounds, (
 ,
,
 , and RC all show no monotonous change trend as the atom number increases. As for (
, and RC all show no monotonous change trend as the atom number increases. As for (
 , HoCu2 compound shows the best performance. As for
, HoCu2 compound shows the best performance. As for
 , TbCu2 compound shows the best performance. As for RC, DyCu2 compound shows the best performance.
, TbCu2 compound shows the best performance. As for RC, DyCu2 compound shows the best performance.
The Arrott plots of RCu2 (
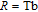 , Dy, Ho, Er) compounds at different temperatures are calculated from isothermal magnetization data and they are shown in Fig. 61. A magnetic transition is expected to be of the first order when the slope of the Arrott plot is negative, whereas it will be of the second order when the slope is positive. It can be seen that the slope around
, Dy, Ho, Er) compounds at different temperatures are calculated from isothermal magnetization data and they are shown in Fig. 61. A magnetic transition is expected to be of the first order when the slope of the Arrott plot is negative, whereas it will be of the second order when the slope is positive. It can be seen that the slope around
 for each RCu2 compound is positive, which indicates that RCu2 (
for each RCu2 compound is positive, which indicates that RCu2 (
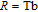 , Dy, Ho, Er) compounds are second order materials. The result is in accord with the heat reversibility observed before because heat and magnetic hysteresis are usually negligible for second order materials. And the MCE materials with small heat and magnetic hysteresis are more valuable for applications.
, Dy, Ho, Er) compounds are second order materials. The result is in accord with the heat reversibility observed before because heat and magnetic hysteresis are usually negligible for second order materials. And the MCE materials with small heat and magnetic hysteresis are more valuable for applications.
7. ConclusionsThe MCE of binary or pseudo binary R–T (
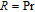 , Gd, Tb, Dy, Ho, Er, Tm;
, Gd, Tb, Dy, Ho, Er, Tm;
 , Ni, Co, Cu) intermetallic compounds (including RGa series, RNi series, R
12Co7 series, R
3Co series, and RCu2 series) have been reviewed, and it is found that these compounds exhibit various magnetic properties and different MCE in low temperature range. Generally, most of the compounds crystallize in orthorhombic structure (space group Cmcm #63, space group Pnma #62 or space group Imma #74), but the R
12Co7 series crystallize in monoclinic structure. The magnetic properties of binary R–T (
, Ni, Co, Cu) intermetallic compounds (including RGa series, RNi series, R
12Co7 series, R
3Co series, and RCu2 series) have been reviewed, and it is found that these compounds exhibit various magnetic properties and different MCE in low temperature range. Generally, most of the compounds crystallize in orthorhombic structure (space group Cmcm #63, space group Pnma #62 or space group Imma #74), but the R
12Co7 series crystallize in monoclinic structure. The magnetic properties of binary R–T (
 , Gd, Tb, Dy, Ho, Er, Tm;
, Gd, Tb, Dy, Ho, Er, Tm;
 , Ni, Co, Cu) intermetallic compounds (including RGa series, RNi series, R
12Co7 series, R
3Co series, and RCu2 series) are complex and interesting. The ground state is FM or AFM, the number of magnetic transitions is one, two or three, and the magnetocaloric effect is positive or negative. The Curie temperature or Neel temperature of R–T compounds is very low, and order–disorder temperature is negatively correlated with the maximum of
, Ni, Co, Cu) intermetallic compounds (including RGa series, RNi series, R
12Co7 series, R
3Co series, and RCu2 series) are complex and interesting. The ground state is FM or AFM, the number of magnetic transitions is one, two or three, and the magnetocaloric effect is positive or negative. The Curie temperature or Neel temperature of R–T compounds is very low, and order–disorder temperature is negatively correlated with the maximum of
 according to Oesterreicher et al.[47] Thus large value of
according to Oesterreicher et al.[47] Thus large value of
 was usually observed in the compounds with large total angular moment quantum number J, such as Ho series, Er series, and Tm series. Since several magnetic transitions exist for some compounds, multi-peaks are observed on the MCE curves. In some cases, several peaks change to one wide and high peak, which is very useful for actual application as magnetic refrigerant materials. Rare earth atoms are also substituted by other R atoms with different S (spin angular moment) or J (total angular moment), and thus several compounds with better MCE performance are obtained. Those binary and pseudo-binary R–T compounds showing good MCE performance especially under low magnetic field change, may be promising candidates for magnetic refrigeration materials in low temperature range in the future, such as the application in liquefaction of helium, hydrogen, and nitrogen.
was usually observed in the compounds with large total angular moment quantum number J, such as Ho series, Er series, and Tm series. Since several magnetic transitions exist for some compounds, multi-peaks are observed on the MCE curves. In some cases, several peaks change to one wide and high peak, which is very useful for actual application as magnetic refrigerant materials. Rare earth atoms are also substituted by other R atoms with different S (spin angular moment) or J (total angular moment), and thus several compounds with better MCE performance are obtained. Those binary and pseudo-binary R–T compounds showing good MCE performance especially under low magnetic field change, may be promising candidates for magnetic refrigeration materials in low temperature range in the future, such as the application in liquefaction of helium, hydrogen, and nitrogen.
Although the MCE of many binary R–T compounds (including RGa series, RNi series, R
12Co7 series, R
3Co series, and RCu2 series) have been investigated systematically in the past decades, a lot of important problems, such as the influence of volume transition on MCE, the mechanism of positive MCE in the AFM materials, and the reason of the enhancement of MCE for substituted compounds, have not been addressed well. In fact, some work connected with physical mechanism has been done by employing powder neutron diffraction technique. And more and further investigations are necessary to do. In conclusion, previous studies have revealed that the binary or pseudo-binary R–T (
 , Gd, Tb, Dy, Ho, Er, Tm;
, Gd, Tb, Dy, Ho, Er, Tm;
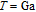 , Ni, Co, Cu) intermetallic compounds (including RGa series, RNi series, R
12Co7 series, R
3Co series, and RCu2 series) exhibit good performance on MCE in low temperature range, suggesting the promising application in low-temperature magnetic refrigeration. However, there is still a lot of work to be done.
, Ni, Co, Cu) intermetallic compounds (including RGa series, RNi series, R
12Co7 series, R
3Co series, and RCu2 series) exhibit good performance on MCE in low temperature range, suggesting the promising application in low-temperature magnetic refrigeration. However, there is still a lot of work to be done.