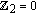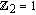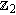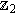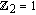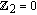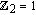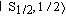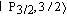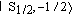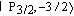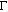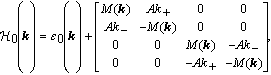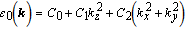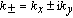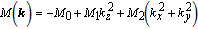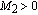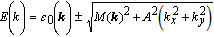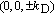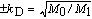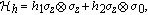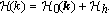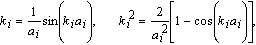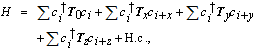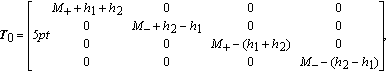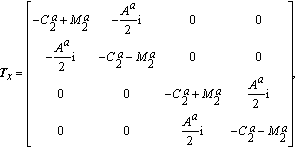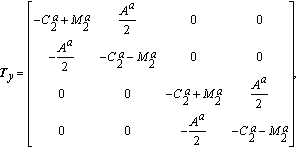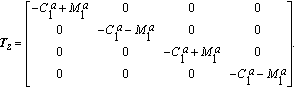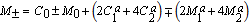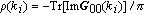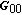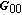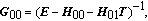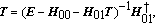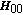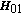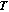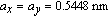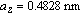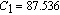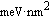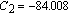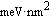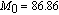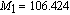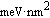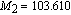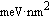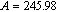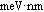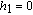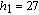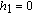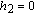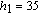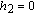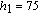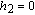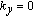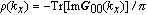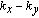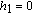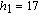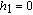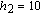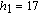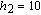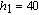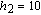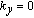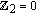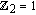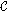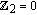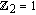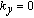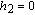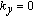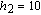Control of topological phase transitions in Dirac semimetal films by exchange fields
Department of Physics, Beihang University, Beijing 100191, China
† Corresponding author. E-mail:
hlwang@buaa.edu.cn hpan@buaa.edu.cn
1. IntroductionTopological insulators are hot topics in the study of condensed matter physics.[1,2] The topological insulators with time-reversal symmetry (TRS) can be classified by a
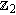 topological parameter. In these topological insulators, the gapless states in surface or edge are protected by TRS, and these topologically nontrivial states have a nonzero topological number
topological parameter. In these topological insulators, the gapless states in surface or edge are protected by TRS, and these topologically nontrivial states have a nonzero topological number
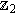 .[1–3] The nontrivial topology state, known as the quantum spin Hall (QSH) state,[4–7] is labeled by the integer
.[1–3] The nontrivial topology state, known as the quantum spin Hall (QSH) state,[4–7] is labeled by the integer
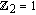 .
.
When the TRS is broken, the quantum anomalous Hall (QAH) states can be realized in a system with strong spin–orbit coupling (SOC), where no orbital magnetic field and Landau levels exist.[8–10] In the QAH states, the gapless edge modes at the boundaries have a well-defined topological order in the bulk, which is characterized by the first Chern number
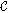 .[11–13] The QAH is expected to exist in graphene with the effects of the Rashba spin–orbit coupling and exchange field, and also in the hybrid model with the doping transition-metal atoms.[14–18] Just recently, a new theory has been proposed that the QAH can be obtained by n–p codoping in three-dimensional topological insulators at high temperatures.[19] The discovery of the QAH effect makes it possible for topological materials to realize the electronics with very low energy dissipation, and it also brings a broader prospect for the novel quantum devices’ application.[20,21] Moreover, the experiments on topology with magnetic semimetal have realized the QAH,[22–25] which greatly promotes the study of topological insulator.
.[11–13] The QAH is expected to exist in graphene with the effects of the Rashba spin–orbit coupling and exchange field, and also in the hybrid model with the doping transition-metal atoms.[14–18] Just recently, a new theory has been proposed that the QAH can be obtained by n–p codoping in three-dimensional topological insulators at high temperatures.[19] The discovery of the QAH effect makes it possible for topological materials to realize the electronics with very low energy dissipation, and it also brings a broader prospect for the novel quantum devices’ application.[20,21] Moreover, the experiments on topology with magnetic semimetal have realized the QAH,[22–25] which greatly promotes the study of topological insulator.
Besides the research and development of topological insulators, the topology of semimetal research has also entered a new stage. Dirac semimetal (DSM) has been known as a kind of topological semimetal, which has topological properties but without a bulk band gap. This kind of material is difficult to obtain, but recent experiments have found it, such as Na3Bi and Cd3As2.[26–34] The Fermi surface of this kind of material is preserved by the crystalline symmetry and has four-fold degenerate Dirac point(s).[35] Each Dirac point can be viewed as two opposite chiral Weyl points’ superposition, which is protected by the lattice symmetry.[36–39] The DSM can be viewed as a parent material to realize the topological insulators. If the DSM sample has a finite thickness in some fixed direction, the coupling in the two surface states will produce a gap at the Dirac points and realize a QSH phase.[35,40] In the DSM film system, a QSH phase can be obtained by the control of a vertical electric field too.[36]
In this work, the control of DSM’s topological phase transitions with the exchanged field are discussed in detail. Due to the quantum confinement effects, the DSM films can be in the topologically trivial state with
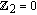 or in a topologically nontrivial state with
or in a topologically nontrivial state with
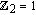 . With the help of exchange fields, the DSM films can be transited into the topological QAH state from the topology trivial state or from the topologically nontrivial state.
. With the help of exchange fields, the DSM films can be transited into the topological QAH state from the topology trivial state or from the topologically nontrivial state.
2. Physical modelThe generic low-energy effective model is the base to analyse, and this model is derived from previous works for DSM A3Bi (A = Na, K, and Rb) and Cd3As2.[36,41–43] As for these materials, their states can be expanded around Fermi energy by using a minimal four-orbital basis, which are
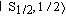 ,
,
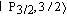 ,
,
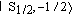 , and
, and
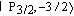 . In the Brillouin zone, the effective Hamiltonian around
. In the Brillouin zone, the effective Hamiltonian around
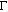 -point can be expanded up to quadratic order, and in the wave-vector k direction it can be written as
-point can be expanded up to quadratic order, and in the wave-vector k direction it can be written as
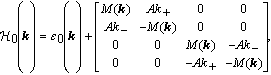 | (1) |
where
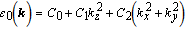
,
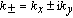
, and
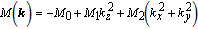
, and
M
0,
M
1,
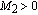
, which is in order to reproduce the band inversion feature at
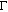
-point. By the first-principles calculation and the experimental measurement, the material-specific parameters
A,
C
i
, and
M
i
can be achieved. This model captures the essential low-energy physics compared well with experiments.
[26,30,35,44] The energy eigenvalue is
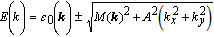
. Then the coordinates of the two Dirac points in
k
z
-axis are
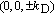
, where
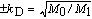
. Each Dirac point can be viewed as two opposite chiral Weyl points’ superposition and it is four-fold degenerate. In the low-energy model, when DSM is confined along different directions, one can see the different anisotropic spectrums.
The exchange field’s Hamiltonian can be written as
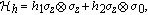 | (2) |
where
h
1,
h
2 are the exchange field strengths. Then the Hamiltonian of the system is
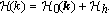 | (3) |
In order to make the calculation for DSM with finite thickness, the model is placed in the three-dimensional (3D) lattice space. The following standard substitutions are taken
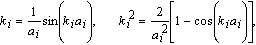 | (4) |
where
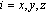
. Then the 3D lattice space’s low-energy effective Hamiltonian around
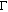
in the Brillouin zone can be written as
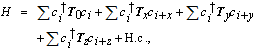 | (5) |
where
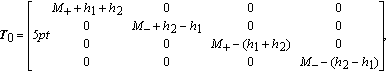 | (6) |
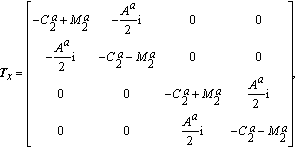 | (7) |
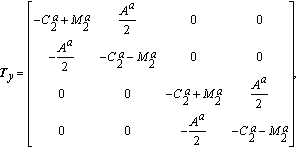 | (8) |
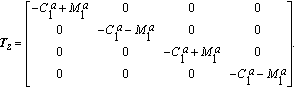 | (9) |
Here
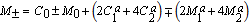
.
In order to study the topological nature further, the local density of states (LDOS) by using the iterative method of Green function
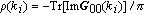 is calculated, where
is calculated, where
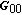 is the delayed function of the lattice surface.
is the delayed function of the lattice surface.
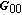 can be calculated by transfer matrix
can be calculated by transfer matrix
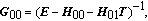 | (10) |
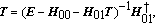 | (11) |
where
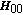
and
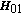
are Hamiltonian matrixes, and
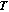
is inverse matrix. Then the surface LDOS is obtained.
3. Numerical results and discussionIn the numerical calculations, parameters of Na3Bi are taken as follows. The thickness of Na3Bi thin films l is limited along z direction and the lattice constants are taken as
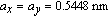 ,
,
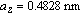 . The adopted constants are related to the separation between internal layer of DSM. The other calculation parameters for Na3Bi are
. The adopted constants are related to the separation between internal layer of DSM. The other calculation parameters for Na3Bi are
 meV,
meV,
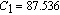
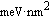 ,
,
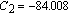
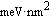 ,
,
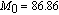 meV,
meV,
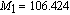
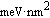 ,
,
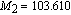
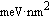 , and
, and
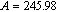
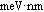 , which are determined by the first-principles calculations and experiments.[26,38]
, which are determined by the first-principles calculations and experiments.[26,38]
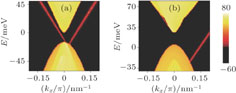
The case where the film’s layer l = 5 is discussed first. It can be seen from Fig. 1(a) that, where the exchange field h
2 is set to be 0, the energy gap is changing with the exchange field h
1. When
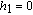 , the diagram shows that the system has a gap about 70 meV, which is marked as A point in Fig. 1(a). With the increase of h
1, energy gap decreases and closes at
, the diagram shows that the system has a gap about 70 meV, which is marked as A point in Fig. 1(a). With the increase of h
1, energy gap decreases and closes at
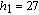 meV, which is marked as B point. Then with the increase of h
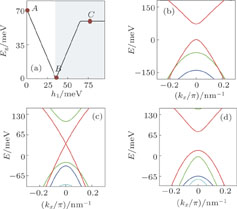
1, the gap reopens. The energy spectrums shown in Figs. 1(b)–1(d) correspond to the three states marked by A, B, and C in Fig. 1(a), respectively. The parameters are
meV, which is marked as B point. Then with the increase of h
1, the gap reopens. The energy spectrums shown in Figs. 1(b)–1(d) correspond to the three states marked by A, B, and C in Fig. 1(a), respectively. The parameters are
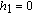 ,
,
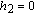 for Fig. 1(b),
for Fig. 1(b),
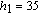 meV,
meV,
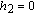 for Fig. 1(c), and
for Fig. 1(c), and
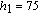 meV,
meV,
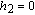 for Fig. 1(d). All the points are plotted versus k
x
(with
for Fig. 1(d). All the points are plotted versus k
x
(with
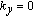 ). We can see that before the closing point, the system has a gap, which belongs to the insulating state; after the closing point the system is also in the insulation state. Thus the critical closing point divides the topological phase into a topologically trivial phase and a nontrivial phase.
). We can see that before the closing point, the system has a gap, which belongs to the insulating state; after the closing point the system is also in the insulation state. Thus the critical closing point divides the topological phase into a topologically trivial phase and a nontrivial phase.
Then, the LDOS to observe the edge states’ variation is calculated, known as
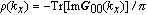 . As we all know, in the low energy model, the
. As we all know, in the low energy model, the
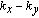 plane has the nature of the isotropic. So without loss of generality, the phase diagram along x direction is only discussed.[36] Here the system has a confinement along z direction.
plane has the nature of the isotropic. So without loss of generality, the phase diagram along x direction is only discussed.[36] Here the system has a confinement along z direction.
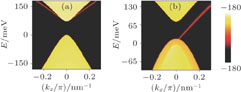
When the thin film’s layer l = 5, the surface LDOS corresponding to the point A and C in Fig. 1(a) are shown in Fig. 2. Figure 2(a) and 2(b) represent the states before and after the closing point, respectively. The energy gap can be identified clearly. Before the closing point, there are no edge states between the energy gap and it is a trivial insulator state; but after the closing point, there appears a bright line between the energy gap and it is crossing the gap. This is corresponding to the QAH phase.
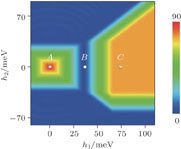
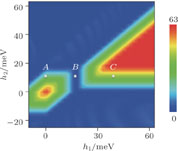
For a more comprehensive analysis of the spectrum, the energy spectrum’s phase diagrams are calculated. The phase diagram with l = 5 is shown in Fig. 3. It can be seen that the film’s energy spectrum has a gap at point A in Fig. 3, corresponding to point A in Fig. 1(a), and it is a trivial insulator. Point B in Fig. 3 is correctly the echo of point B in Fig. 1(a), where the film’s energy spectrum is gapless. Point C in Fig. 3 is also corresponding to the point C in Fig. 1(a), which is the QAH phase.
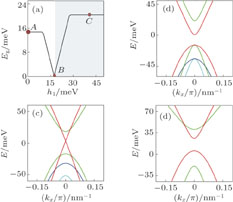
Next, the case where the film’s layer l = 7 is discussed. It can be seen from Fig. 4(a) that, when the exchange field h
2 is set to be 10 meV, the energy gap is changing with the exchange field h
1 . When
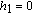 , the system has a gap about 14 meV as shown in Fig. 4(a), which is marked as A point. With the increase of h
1, the energy gap decreases and closes at
, the system has a gap about 14 meV as shown in Fig. 4(a), which is marked as A point. With the increase of h
1, the energy gap decreases and closes at
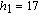 meV, which is marked as B point. Then with the increase of h
1, the gap reopens. The energy spectrums shown in Figs. 4(b)–4(d) correspond to the three states marked by A, B, and C in Fig. 4(a) in turn. The parameters are
meV, which is marked as B point. Then with the increase of h
1, the gap reopens. The energy spectrums shown in Figs. 4(b)–4(d) correspond to the three states marked by A, B, and C in Fig. 4(a) in turn. The parameters are
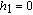 ,
,
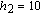 meV for Fig. 4(b),
meV for Fig. 4(b),
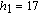 meV,
meV,
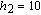 meV for Fig. 4(c), and
meV for Fig. 4(c), and
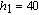 meV,
meV,
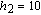 meV for Fig. 4(d). All the points are plotted versus k
x
(with
meV for Fig. 4(d). All the points are plotted versus k
x
(with
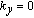 ). It can be seen that before the closing point, the system has a gap, but there are a pair of edge states in the gap, which belongs to the QSH state. After the closing point, the system is also in the insulation state. While there appears an edge state in the gap, this is a QAH state.
). It can be seen that before the closing point, the system has a gap, but there are a pair of edge states in the gap, which belongs to the QSH state. After the closing point, the system is also in the insulation state. While there appears an edge state in the gap, this is a QAH state.
The corresponding LDOS of the point A and C in Fig. 4(a) are shown in Fig. 5. Figure 5(a) and 5(b) represent the state before and after the closing point B, respectively. It can be seen that the energy gaps are identified clearly. Before the closing point, there are a pair of edge states crossing the gap and it is a QSH state, but after the closing point, there appears only a bright line between the energy gap and it is crossing the gap. This is corresponding to the QAH phase.
Finally, the phase diagram with l = 7 is calculated, shown in Fig. 6. It can be seen that the film’s energy spectrum opens a gap at point A in Fig. 6, which is corresponding to point A in Fig. 4(a), and there are a pair of edge states crossing the gap, which is a QSH phase. Point B in Fig. 6 is correctly the echo of point B in Fig. 4(a), where the film’s energy spectrum is gapless. Point C in Fig. 6 is also corresponding to the C point in Fig. 4(a), which is the QAH phase.