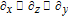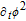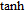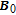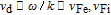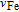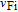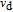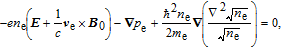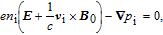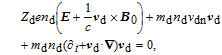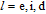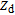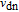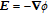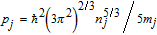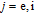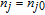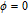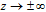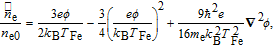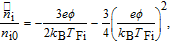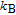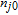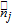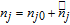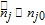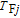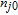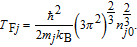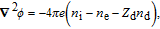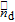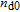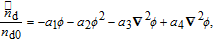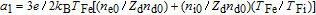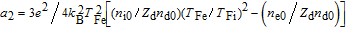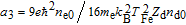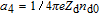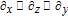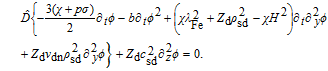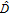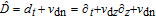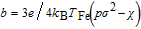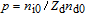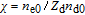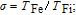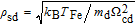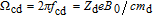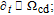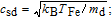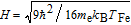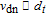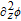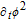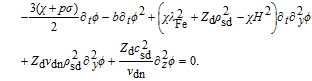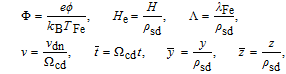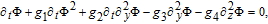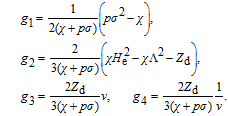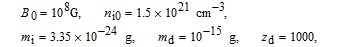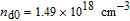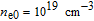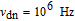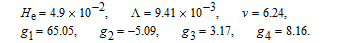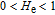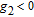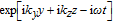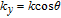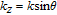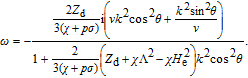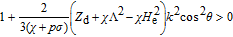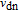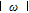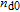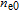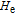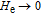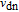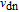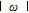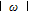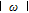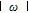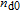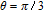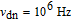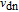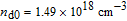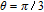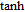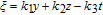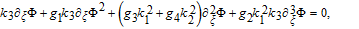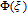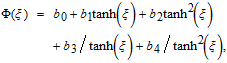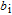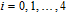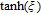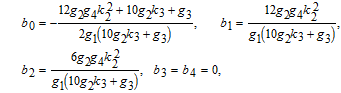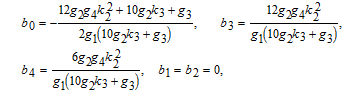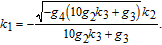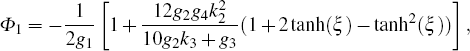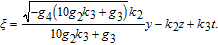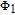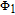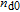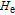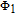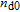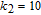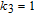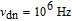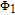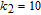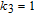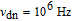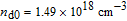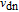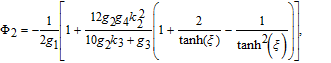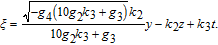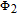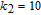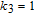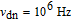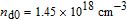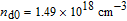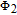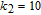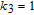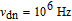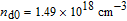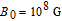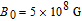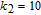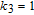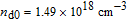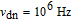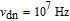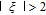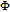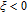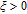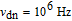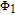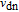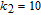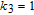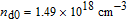Cite this Article
Yang Jian-Rong, Xu Ting, Mao Jie-Jian, Liu Ping, Liu Xi-Zhong. Dust acoustic waves in collisional uniform dense magnetoplasma. Chinese Physics B, 2017, 26(1): 015202  
Permissions
Dust acoustic waves in collisional uniform dense magnetoplasma
Yang Jian-Rong1, 2, †, Xu Ting2, Mao Jie-Jian1, Liu Ping3, Liu Xi-Zhong4
School of Physics and Electronic Information, Shangrao Normal University, Shangrao 334001, China
Department of Physics, Nanchang University, Nanchang 330031, China
College of Electron and Information Engineering, University of Electronic Science and Technology of China Zhongshan Institute, Zhongshan 528402, China
Institute of Nonlinear Science, Shaoxing University, Shaoxing 312000, China

 

† Corresponding author. E-mail: sryangjr@163.com

Abstract

In order to study the characteristics of dust acoustic waves in a uniform dense dusty magnetoplasma system, a nonlinear dynamical equation is deduced using the quantum hydrodynamic model to account for dust–neutral collisions. The linear dispersion relation indicates that the scale lengths of the system are revised by the quantum parameter, and that the wave motion decays gradually leading the system to a stable state eventually. The variations of the dispersion frequency with the dust concentration, collision frequency, and magnetic field strength are discussed. For the coherent nonlinear dust acoustic waves, new analytic solutions are obtained, and it is found that big shock waves and wide explosive waves may be easily produced in the background of high dusty density, strong magnetic field, and weak collision. The relevance of the obtained results is referred to dense dusty astrophysical circumstances.

PACS: 52.35.Mw;52.27.Lw;52.25.Xz;52.35.Fp
Keyword:dusty magnetoplasma;quantum hydrodynamic model;dust acoustic wave
1. Introduction

As it is known, dusty (complex) plasmas consist of partially or fully ionized gas and charged micron-sized particles and show the plasma state of soft matter.[1] Due to their ubiquitous nature, a world-wide studying interest is evoked in planet formation, planetary rings, solar system research, Earth’s environments, plasma technology, etc. For unmagnetized and weak coupled dusty plasmas, two types of acoustic modes are the dust acoustic (DA) waves and dust ion-acoustic (DIA) waves,[2] which were predicted in theory[3,4] and observed in some laboratory experiments.[5–8] For the DA waves, the phase velocity is much smaller than the electron and ion thermal speeds, and the frequency is much smaller than the dust plasma frequency. In the DA waves, the restoring forces originate in the pressures of the inertialess electrons and ions, and the waves are supported by the dust mass that provides the inertia.[2]

Since Haas extended the magnetohydrodynamic model[9] to the quantum hydrodynamic (QHD) model[10] in the case of nonzero magnetic field for dense plasmas with a quantum correction term generally known as the Bohm potential, the QHD model has become one of the most frequently employed models for studying dense quantum plasmas, and many research results have been gained with it.[11–17] For example, in the investigation of DA waves, the variations of the drift shock profile with the quantum Bohm potential, collision frequency, ratio of drift to shock velocity in the co-moving frame and effect of magnetic field have been investigated.[18] The quantum DA double layers show that the formation of the compressive and the rarefactive double layers relies on the quantum plasma parameters.[19] It is noted that positive and negative bell-shaped solitary pulses of a magnetized quantum dusty plasma turn into explosive pulses relying largely upon the angles of propagation and dust polarity.[20]

In this paper, the linear and nonlinear propagation characteristics of the DA waves are investigated in a dense dusty plasma system comprising inertia dust particles, and inertialess Boltzmann distributed electrons and ions. Using the QHD model, assuming 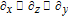, considering the case that the collision between dust particles and neutrals is dominant, and keeping the nonlinear term 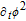, a simplified two-dimensional nonlinear equation is derived in the next section. In Section 3, a dispersion relation expression is derived by linearizing the equation. The variations of the dispersion frequency with the typical parameters are discussed by graph. In Section 4, investigating the coherent nonlinear DA waves, new shock solution and explosive solution of the nonlinear equation are obtained by 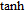function transform methods. The effects of the typical parameters on the shock wave and explosive wave are investigated in detail. The final section is devoted to the conclusion and discussion.

2. Derivation of nonlinear equation

We investigate a multi-component homogeneous quantum dusty magnetoplasma containing inertialess electrons, inertialess ions, and negatively charged dusts (e–i–d) with neutrals in the background, and consider the collision effect between dust particles and neutrals. Assume that the equilibrium magnetic field 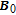is in the z direction, and the final derived nonlinear equation will be in the y–z plane. The phase velocity of the wave is 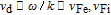( 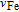, , 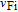and 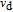are the electron, ion, and dust Fermi velocities, respectively), so the quantum statistical and Bohm potential contributions of dust can be ignored. Employing the QHD model, the following governing equations for individual species can be written.

Three dynamic equations for inertialess electrons, inertialess ions, and negatively charged dust particles are governed by

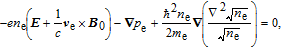(1)
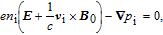(2)
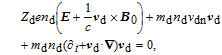(3)
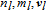
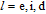
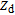
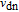
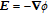
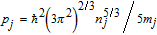
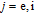
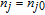
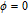
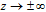
where ( ), e and are density, mass, velocity, electron charge, and dust charge number, respectively; is the collision frequency between dust particles and neutrals; the electrostatic field (ϕ is the electrostatic potential); the electron and ion Fermi pressure ( ). Applying the boundary conditions and at , and the Talor expansion in the parallel component of Eqs. (1) and (2), the following results can be obtained:
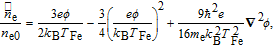(4)
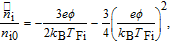(5)
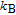
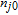
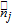
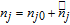
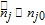
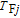
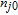
where is the Boltzmann constant, is the equilibrium density, is the perturbed density, , and , the electron and ion Fermi temperature and the equilibrium density are related by

On the basis of the Poisson equation

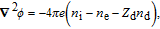(6)
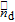
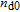
the rate of the dust density perturbation to density equilibrium is
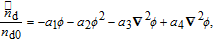(7)
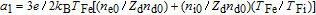
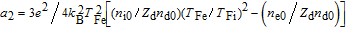
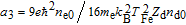
where , , , and .

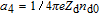
The parallel component and perpendicular component of dust velocity can be gained from Eq. (3). Substituting them and Eq. (7) into the following dust continuity equation:

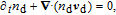(8)
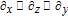
and making the supposition , a nonlinear dynamic equation is obtained as
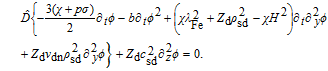(9)
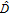
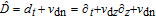
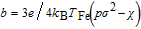
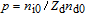
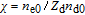
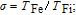
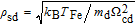
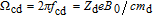
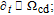
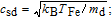
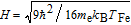
Here, the operator is defined as , , , , the electron Fermi wavelength the dust Larmor radius at the electron temperature , the dust cyclotron frequency , and the low frequency waves satisfy the dust acoustic speed is and the quantum parameter is . It is noticed that the inhomogeneous drift equation with shock wave is studied using KdVB and KPB equations.[18]

In this paper, when the collision effect is taken into account between neutrals and dust particles in the dense plasma system, the nonlinear dust acoustic waves such as the shock wave and explosive wave will be studied in detail. When 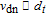in the operate, and the terms 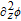and 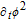are kept, equation (9) becomes a two-dimensional nonlinear dynamic equation

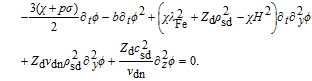(10)
When these rescalings are introduced,
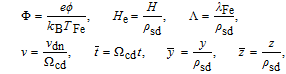(11)
equation (10) is normalized as
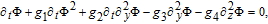(12)
where the bars have been dropped. In Eq. (12), the dimensionless coefficients are
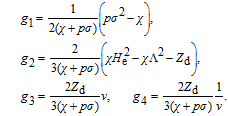(13)

According to the plasma characteristic of the dense astrophysical atmosphere,[21–23] the physical quantities can be selected as

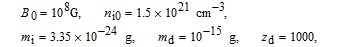(14)
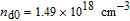
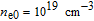
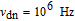
then taking , , and , the normalized quantities can be calculated as
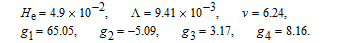(15)
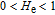
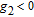
Thereinto, the normalized quantities , and when and .

3. Dispersion relation

The dispersion relations of plasmas are important research problems that attract a great deal of attention. Recently, for numerical calculation of kinetic plasma dispersion relations, a general and effective approach is developed according to Pade approximation and matrix transformation.[24] Here, in order to discuss the linear propagation character of the dust acoustic waves, we make Eq. (12) linearized and suppose that the perturbation is proportional to 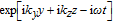. Here, k is the wave number, 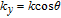, , 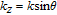θ is the obliqueness angle, and ω is the wave frequency.

The dispersion relation can then be obtained as

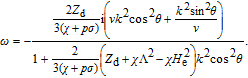(16)
For a finite solution,
must hold. It is obvious that our parameters in Eq. (15) certainly satisfy this demand.

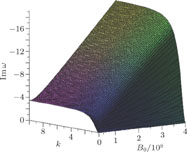
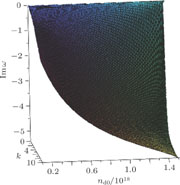
Equation (16) reveals that the collision frequency 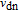between neutrals and dust particles effects directly on the dispersion frequency, and the quantum parameter revises the scale lengths of this dusty plasma system. The ω is a pure imaginary number, so the wave motion decays gradually and this system tends to a stable state eventually. Besides, ω is also related to the density, magnetic field, wave number, obliqueness angle, etc. Under the parameters given by Eqs. (14) and (15), the variations of ω with the parameters are displayed in Figs. 1–3. Figure 1 shows that the absolute frequency 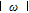increases purely with the dust density 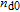increasing. It is noticed that increasing the negatively charged dust density may result in the decrease of the electron density 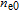in this system, which is equivalent to the increase of the normalized quantum correction 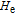, so ω gradually tends to a small stable value as 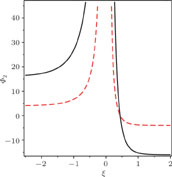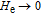. Figure 2 shows how ω changes with 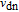. With the increase of 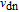, 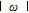decreases rapidly at first, then changes slowly and tends to a small value. Accordingly, increasing dissipation and damping leads to small 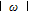. Figure 3 shows th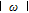at increases with the increase of magnetic field B 0. From Figs. 1–3, it is clear that 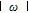always increases when increasing the wave number k.

Fig. 1. (color online) Change of the dispersion frequency with the dust density and the wave number k determined by Eq. (16) for , , and other parameters given in Eq. (14).
Fig. 2. (color online) Change of the dispersion frequency with the collision frequency and the wave number k determined by Eq. (16) for , , and other parameters given in Eq. (14).
Fig. 3. (color online) Change of the dispersion frequency with the magnetic field B 0 and the wave number k determined by Eq. (16) for , , and other parameters given in Eq. (14).
4. Shock wave

As it is known, nonlinearities should be considered when the amplitudes of waves become large enough. The nonlinearity in the plasma system devotes to the localization of waves, which results in different types of meaningful coherent structures such as shock waves, solitary waves, voids, vortices, etc.[2,25,26] In this section and the next section, investigating the coherent nonlinear dust acoustic waves, we look for the shock and explosive solutions of equation (12) employing nonlinear methods.

We utilize the general traveling wave method and 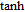function method to achieve the shock solution and explosive solution for the nonlinear dynamic equation (12). Using this coordinate transformation 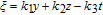, equation (12) becomes

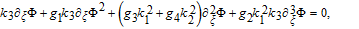(17)
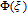
where k 1 and k 2 are the nonlinear wave numbers, and k 3 is the wave frequency. Furthermore, is expanded as the form
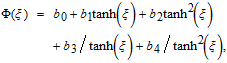(18)
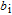
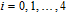
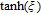
where ( ) are undetermined constants. After equation (18) is substituted into Eq. (17), and all the coefficients of the terms and their derivatives are set to zero, we get an over-determined set of equations for the unknowns. Two groups of significative solutions can then be obtained by solving the determinant equations as follows:
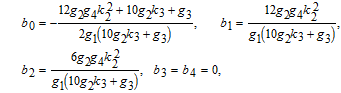(19)
and
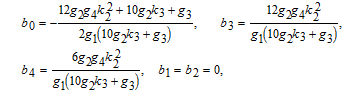(20)
where k 2 and k 3 are the positive constants, and k 1 satisfies the constraint
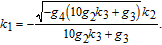(21)

Substituting the first group of solution (19) into Eq. (18) results in this shock solution

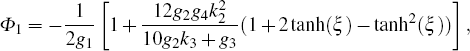(22)
where

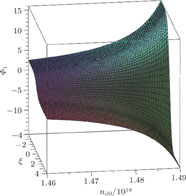
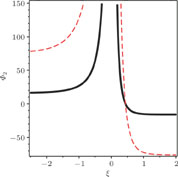
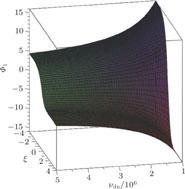
For studying the effects of the parameters on the dust-acoustic shock wave, we use the numerical parameters in Eqs. (14) and (15), and give the graphic representations. It is noticed that the expressions of quantum effects of electrons and ions include density, so the variation of the electrostatic potential 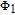with density reflects indirectly the change of 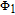with quantum parameter. Figure 4 displays that the intensity of the shock wave is strengthened with the increase of the dust density 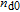(that is increasing the quantum parameter 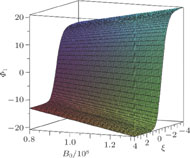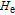). Figure 5 shows that increasing the magnetic field strength B 0 leads to the enhancement of shock wave, which means that the physical environment of a strong magnetic field can easily generate a large shock wave.

Fig. 4. (color online) The shock wave with the dust density determined by Eq. (22) for , , , and other parameters given in Eq. (14).
Fig. 5. (color online) The shock wave with the magnetic field B 0 determined by Eq. (22) for , , , , and other parameters given in Eq. (14).

Besides, the shock waves can be excited in nonlinear dissipative medium, and the dissipation is related to collision, kinematic viscosity, etc. Figure 6 presents that the strength of the shock wave increases with the decrease of the collision frequency 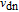between neutrals and dust particles, which presents the decrease of dust dissipation in the system.

Fig. 6. (color online) The shock wave with the collision frequency determined by Eq. (22) for , , , and other parameters given in Eq. (14).
5. Explosive wave

Substituting the second group of solution (20) into Eq. (18) results in this new explosive solution[27]

(23)
with

It is noticed from Figs. 7–9 that the width of the explosive wave gets bigger when increasing the dust density and magnetic field strength, respectively, but gets narrower when increasing the collision between dust particles and neutrals, so a wide explosive wave can be generated under the conditions of high dust density, strong magnetic field, and weak collision in this dusty plasma system.

Fig. 7. (color online) The explosive wave given by Eq. (23) with , , , (solid line), and (dash line). Other parameters are given in Eq. (14).
Fig. 8. (color online) The explosive wave given by Eq. (23) with , , , , (solid line), and (dash line). Other parameters are given in Eq. (14).
Fig. 9. (color online) The explosive wave given by Eq. (23) with , , , (solid line), and (dash line). Other parameters are given in Eq. (14).

It is found that the shock and explosive solutions have an inversely-proportional relationship with the nonlinear coefficient g 1, which reflects that the nonlinear effect in this system plays a very important role in the shock and explosive waves. Besides, the variations of the shocks and explosions with the phase ξ of space–time have similar characters as displayed in Figs. 4–9. The electrostatic potential varies quickly when is small. While is big enough ( ), approaches separately to positive or negative steady values as or .

6. Conclusion and discussion

In order to study dust acoustic waves in the dense uniform dusty plasma system, the two-dimensional nonlinear governing Eq. (10) is deduced by the QHD model when the collision effect is considered between neutrals and dust particles. The linear dispersion relation presents that the quantum parameter revises the scale lengths of this dusty plasma system; in addition, the wave motion decays gradually resulting in the system leading to a stable state eventually. The plots show that the absolute dispersion frequency 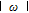raises purely with the increase of the dust density, magnetic field strength, and wave number, respectively. With the increase of the collision frequency, 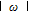decreases rapidly at first, then changes slowly and tends to a small value eventually.

For the coherent nonlinear dust acoustic waves, the shock solution shows that the strength of the shock wave raises with enhancing the dust density and the magnetic field, respectively, but decreases with the increase of the collision effect between dust particles and neutrals (i.e., with the increase of the dissipation). Besides, the explosive solution displays that the width of the explosive wave gets bigger when increasing the dust density and magnetic field strength, respectively; but gets smaller when increasing the collision between dust particles and neutrals. Therefore, regarding this dusty plasma system, a big shock wave and wide explosive wave may be easily produced in the background of high dusty density, strong magnetic field, and weak collision that may cause small dissipation and damping.

Under the astrophysical circumstances of dense atmosphere where collision and quantum effect are prominent, the above outcomes may support useful references to the dust acoustic waves having coherent nonlinear structures.

Reference
[1] Morfill G E Ivlev A V 2009 Rev. Mod. Phys. 81 1353
[2] Shukla P K Mamun A A 2002 Introduction to Dusty Plasma Physics Bristol IOP
[3] Shukla P K Silin V P 1992 Phys. Scr. 45 508
[4] Rao N N Shukla P K Yu M Y 1990 Planet. Space Sci. 38 543
[5] Prabhakara H R Thana V L 1996 Phys. Plasmas 3 3176
[6] Barkan A D’Angelo N Merlino R L 1995 Phys. Plasmas 2 3563
[7] Pieper J B Goree J 1996 Phys. Rev. Lett. 77 3137
[8] Nakamura Y Bailung H Shukla P K 1999 Phys. Rev. Lett. 83 1602
[9] Shukla P K Rahman H U 1996 Phys. Plasmas 3 430
[10] Haas F 2005 Phys. Plasmas 12 062117
[11] Masood W 2009 Phys. Lett. A 373 1455
[12] Yang J R Tang X Y Lou S Y 2011 Phys. Plasmas 18 022303
[13] Haque Q Mahmood S 2008 Phys. Plasmas 15 034501
[14] Haque Q Saleem H 2008 Phys. Plasmas 15 064504
[15] Yang J R Tang X Y Gao X N Cheng X P Lou S Y 2011 Europhys. Lett. 94 45001
[16] Mao J J Yang J R 2012 Acta Phys. Sin. 61 020206 (in Chinese)
[17] Yang J R Wu B Mao J J Liu P Wang J Y 2014 Commun. Theor. Phys. 62 871
[18] Masood W Karim S Shah H A 2010 Phys. Scr. 82 045503
[19] Moslem W M Shukla P K Ali S Schlickeiser R 2007 Phys. Plasmas 14 042107
[20] Sadiq M Ali S Sabry R 2009 Phys. Plasmas 16 013706
[21] van Horn H M 1979 Phys. Today 32 23
[22] Padmanabhan T 2001 Theoretical Astrophysics II Stars and Stellar Systems Cambridge Cambridge University Press
[23] Dev A N Deka M K Sarma J Adhikary N C 2015 J. Korean Phys. Soc. 67 339
[24] Xie H S Xiao Y 2016 Plasma Sci. Technol. 18 97
[25] Shukla P K Eliasson B 2009 Rev. Mod. Phys. 81 25
[26] Ferdousi M Yasmin S Ashraf S Mamun A A 2015 Chin. Phys. Lett. 32 015201
[27] Moslem W M Ali S Shukla P K Tang X Y Rowlands G 2007 Phys. Plasmas 14 082308