† Corresponding author. E-mail:
Project supported by the National Natural Science Foundation of China (Grant Nos. 11305037, 11347114, and 11374054) and the Natural Science Foundation of Fujian Province, China (Grant No. 2013J01012).
We investigate the effect of the dipole–dipole interaction (DDI) on the photon statistics with two atoms trapped in an optical cavity driven by a laser field and subjected to cooperative emission. By means of the quantum trajectory analysis and the second-order correlation functions, we show that the photon statistics of the cavity transmission can be flexibly modulated by the DDI while the incoming coherent laser selectively excites the atom–cavity system’s nonlinear Jaynes–Cummings ladder of excited states. Finally, we find that the effect of the cooperatively atomic emission can also be revealed by the numerical simulations and can be explained with a simplified picture. The DDI induced nonlinearity gives rise to highly nonclassical photon emission from the cavity that is significant for quantum information processing and quantum communication.
The standard Jaynes–Cummings (JC) model in cavity quantum electrodynamics describes the interaction of a two-level atom with a quantized cavity mode.[1] The atom–cavity strong coupling results in the anharmonicity of the JC ladder of eigenstates,[2] which gives rise to photon blockade[3] and thereby nonclassical output photon statistics. The JC model along with the quantum light sources appears as one of the key ingredients for applications in quantum information processing and secure quantum communication.[4]. Generalizing the standard JC model to the case with two atoms in a cavity, the photon emitted by an excited atom can be absorbed by the other in the ground state and the dipole–dipole interaction (DDI) between the two atoms can be induced through exchange of virtual photons.[5,6] The DDI with long-range and anisotropic nature can also be modulated by the electromagnetic environment[7] and significantly affects the dynamical properties[8–10] and the transmission spectrum in the driven atom–cavity systems.[11,12]
Cooperative emission is a fundamental effect in quantum optics arising in systems that are symmetric under the interchange of any pair of particles.[13,14] For few-particle systems where each particle’s state and position can be precisely controlled, the collective emission effects can be tailored.[15–17] In particular, by means of cavity quantum electrodynamics, when the emitters are equally coupled to the cavity mode, the spontaneous emission from the particles is indistinguishable even if they are spatially separated. The preferential emission into a single cavity mode has been recently observed by coupling two atoms[18] or two ions[19] to the normal mode of an optical cavity, where the cooperative emission from the ion–cavity or atom–cavity system can be flexibly tuned and can be used for preparation of the two-particle entangled state. The efficient atom/ion–cavity coupling is significant for the realization of photonic quantum memories[20] and long distance quantum networks.[21,22]
Recently, there has been increased interest in engineering nonclassical features of light since its importance for quantum information devices.[20,23,24] In a cavity QED system, the nonclassical photon statistics of light can be controlled via the photon blockade and electromagnetically induced transparency with emitters strongly coupled to an optical resonator.[25–33] Here, we will discuss the similar physics in a different way that involves the interatomic interaction. We consider two dipole-coupled atoms trapped in an optical cavity driven by an external laser field and take the cooperative emission into account. The effect of the dipole–dipole interaction between the two atoms on the photon statistics and correlations of the cavity transmission is numerically studied via the quantum jump method. If an incoming photon resonantly excites the atom–cavity system from its ground state to the n-excitation dressed state consisting of the two-atom collective state and the cavity field, then a second photon at the same frequency will be detuned from or resonant with the next steps up the ladders depending on the DDI strength. The photon statistics can therefore be modulated by the DDI, which is revealed by the sudden changes of cavity intensity in a random quantum jump trajectory, and by the second order correlation functions. The method can also reveal the effect of the cooperative emission on the photon statistics. The results will be of great interest for the two-atom cavity QED experiment considering the recent progress in observation of collective emission effects.[18,19]
The paper is organized as follows. In Section 2, we derive the effective model of the system with two identical dipole-coupled two-level atoms trapped in a single-mode optical cavity. In Section 3, we briefly review the theory of the quantum-trajectory approach and introduce the method for calculating the second order correlation function. In Section 4, we study the influence of the cavity decay on the cavity intensity and thus the photon statistics involving the interatomic DDI, via the method presented in Section 3. In Section 5, we investigate the effect of the cooperative emission on the cavity intensity. Finally, we summarize our results in Section 6.
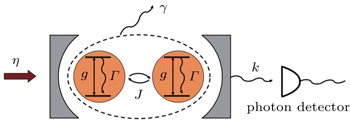
Consider a system composed of two identical two-level atoms that are trapped in a single-mode high-finesse optical cavity, and are localized at positions r1 and r2 along the cavity axis, as shown in Fig.
The cavity field is driven by a coherent laser field with the strength η and the detuning from cavity resonance Δcl. The two atoms interact with each other via a dipole–dipole potential with the interaction strength defined by[8]


For the atom–cavity interaction part, it is convenient to rewrite the Hamiltonian Eq. (
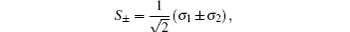

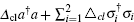
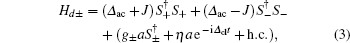
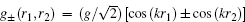
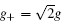

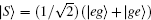


The dissipative dynamics of the system including the atomic spontaneous emission and cavity photonic leakage can be described by the master equation of the Lindblad form in the Born–Markov approximation[37]
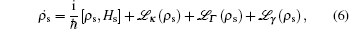

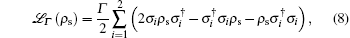

The dissipation in the system can be alternatively treated by the quantum-trajectory method,[38–41] which presents stochastic traces of photon detection events followed by a transient evolution until the next quantum jump is detected. Averaging over many independent realizations of these random trajectories, one recovers the predictions of the corresponding master equation. In addition, the individual random simulations are also available, offering representative records of the randomly selected detection events that directly correspond to what would be observed in a veritable transmission experiment. Thus, by measuring the times for photons leaving the cavity through the output mirror,[38] we are able to analyze the photon statistics and photon correlations.
In this paper, we will focus on the statistical properties of photons under the effect of the interatomic dipolar interaction, where the photon correlation for the cavity transmission in the steady state is described by the second-order correlation function

Numerically, we first calculate the density matrix 


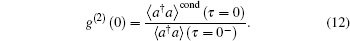
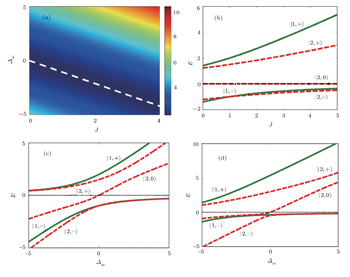
We first consider the resonant interaction of the atoms with the cavity field, i.e., Δac = 0, and simply modulate the interatomic interaction J. By sweeping the driving laser frequency, we can find a sudden increase of the cavity intensity resulting from the single-photon or two-photon resonant transition from the ground state to the corresponding dressed energy levels. The effect of the nonlinearity induced by J on the photon statistics can be revealed by the equal-time correlation function g(2) (0), as shown in Fig.
 | Fig. 3. The equal-time correlation function g(2) (0) as a function of the dipolar coupling strength J for Δac = 0. Further parameters in units of g are (κ,Γ,γ,η) = (0.2,0.1,0.1,0.1). |
For J = 1, the driving laser with detuning Δcl = 1 meets both the one-photon resonance and two-photon resonance conditions, as can be seen in Figs.
The laser driving with Δcl = 0.37 and Δcl = 0.5 correspond to the one-photon resonance and two-photon resonance for J = 5, respectively. Due to the dipolar interaction induced nonlinearity, the former exhibits photon anti-bunching with g(2) (0) < 1 and the latter behaves the other way around. As J decreases from 5 to 1, both the DELs |1,−〉 and |2,−〉 decline, but finally the single-photon resonance condition dominates for J becoming less than 4, since the DEL |1,−〉 is closer to the laser driving frequency. The situation changes while J < 1, where the two-photon resonance in reverse plays the main role since the DEL |2,−〉 appears above the DEL |1,−〉, and g(2) (0) becomes larger than 1.
The photon counting statistics can be directly modelled by the quantum-trajectory approach, as shown in Fig.
As mentioned before, the dipolar interaction plays a role similar to atom-cavity detuning. So it is worthwhile investigating the system dynamics with non-resonant interaction between atom and cavity field, and we will focus on the condition Δac + J = 0 in particular. In Fig.
The photon statistics in the non-resonant case is shown in Fig. 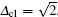
For Δac = −5, the atoms interact with the cavity field dispersively. The eigenenergy for |2,−〉 is only weakly perturbed by the dipolar interaction J. The laser frequency tuned to the red-detuning Δcl = 5.1 from the cavity resonance is however far off-resonant with the intermediate DELs |1,±〉, therefore, the photons can hardly enter the cavity. While J decreases towards the intersection of |1,−〉 and the rescaled |2,−〉, the photon statistics become obviously anti-bunching.
In addition to the photon jumps, the cavity intensity is significantly influenced by the collective atom jumps. The events corresponding to the atomic cooperative emission are marked by the red cross in Fig.


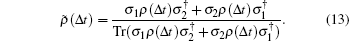
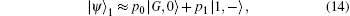
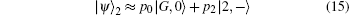
In summary, we have studied the effect of the dipole-dipole interaction on the photon statistics in the laser-driven cavity QED system by including the cooperatively atomic emission. We have found that by modulating the interatomic dipolar coupling, the photon statistics described by the equal-time second order correlation function can vary from bunching to anti-bunching for both the resonant and non-resonant atom–field interaction. The behavior is revealed by the random quantum trajectory as well, which shows sudden increase or decrease of the cavity intensity soon after quantum jumps. This can be further used to explain the effect of the cooperatively atomic emission on the photon statistics and will be of great interest for the two-atom cavity QED experiment.
| 1 | |
| 2 | |
| 3 | |
| 4 | |
| 5 | |
| 6 | |
| 7 | |
| 8 | |
| 9 | |
| 10 | |
| 11 | |
| 12 | |
| 13 | |
| 14 | |
| 15 | |
| 16 | |
| 17 | |
| 18 | |
| 19 | |
| 20 | |
| 21 | |
| 22 | |
| 23 | |
| 24 | |
| 25 | |
| 26 | |
| 27 | |
| 28 | |
| 29 | |
| 30 | |
| 31 | |
| 32 | |
| 33 | |
| 34 | |
| 35 | |
| 36 | |
| 37 | |
| 38 | |
| 39 | |
| 40 | |
| 41 | |
| 42 | |
| 43 |