† Corresponding author. E-mail:
‡ Corresponding author. E-mail:
Project supported by the Doctoral Funds of Guizhou Normal College, China (Grant No. 2015BS006) and the National Natural Science Foundation of China (Grant Nos. 11271158 and 11174108).
The interference between two condensates with repulsive interaction is investigated numerically by solving the one-dimensional time-dependent Gross–Pitaevskii equation. The periodic interference pattern forms in two condensates, which are prepared in a double-well potential consisting of two truncated harmonic wells centered at different positions. Dark solitons are observed when two condensates overlap. Due to the existence of atom–atom interactions, atoms are transferred among the ground state and the excited states, which coincides with the condensate energy change.
Since the realization of Bose–Einstein condensates (BECs) in dilute and ultracold gases, lots of theoretical and experimental studies on BECs have been reported, such as the creation of solitons,[1–5] vortices,[6,7] and symmetry breaking.[8,9] Since BECs have long coherence time and high controllability, as ideal coherent sources they are widely used to study matter–wave interference.[10–12] In the mean-field approximation, the condensates can be described by the macroscopic wavefunction. This theory paves a way toward studying the interference phenomena of condensates theoretically.
Interference between two condensates was first observed experimentally in 1997.[13] Wallis et al.[14] calculated theoretically the macroscopic interference of two independent condensates released from a double-well potential trap. The double-well potential used to form two condensates was created by adding a Gaussian barrier into the center of a harmonic potential.[15] The interference pattern was aperiodic, while dark solitons were generated when two condensates overlapped.[16]
A recent experiment[17] reports a novel pattern of interference between two condensates, which is different from the previous works.[13–15] Motivated by this experiment, the periodic interference pattern of two condensates is demonstrated by numerical simulation in this paper. We also give a theoretical explanation on the generation mechanism of the periodic interference pattern, which has been mentioned by little research up to now.
In the zero-temperature limit, single-component BECs trapped in a harmonic potential are described by the nonlinear Schrödinger equation, known as Gross–Pitaevskii (GP) equation[18]


We consider the condensates in a quasi-one-dimensional system, where the harmonic potential Vh(x,y,z) is a cigar-shaped potential with ωz = ωy ≫ ωx. The three-dimensional (3D) GP equation (

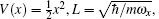
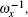

As is known to all, two condensates without interaction can form periodic interference.[16] However, there is little research on the periodic interference between two condensates with interaction. Here we first demonstrate the periodic interference between two condensates with repulsive interaction and give a theoretical explanation of this periodic interference pattern. The condensates are initially trapped in a double-well potential, which consists of truncated harmonic wells centered at positions xi with i = 1,2 and 

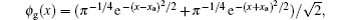
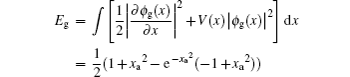
| Table 1. The relations between the physical quantities |ϕg(x = 0)|2, Eg, Ng and xa. . |
From Table 
When gs ≠ 0, the ground state wavefunction of condensates ψ(x;t = 0), chosen as the initial state wavefunction for the following interference, is obtained by propagating in imaginary time starting with the test wavefunction ϕ0(x) = π−1/4e−x2/2. With xa = 5, the ground state density |ψ (x;t = 0)|2 varying with gs is shown in Fig.
As is known to all, when atom interaction is too strong, the condensates are trapped in one well (i.e., self-trapping), so we choose gs = 20 and xa = 5 to demonstrate the interference between two condensates with repulsive interaction. We aim to analyze the interference between these two condensates. After preparing the initial state, the trap V(x) is suddenly changed into 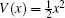
From Fig.
 | Fig. 2. (a) Time evolution of density distributions of two condensates with gs = 20. (b) The density (solid line) and phase (dashed line) distributions of the condensates at t = 1.59. |
Comparing Fig.
In order to explain this periodic behavior, we assume that the two condensates execute harmonic motions with their periods Tg, and their momentums satisfy
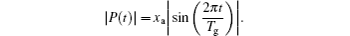
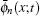

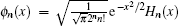

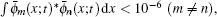
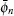
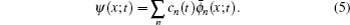

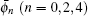
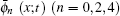

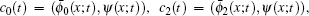
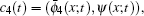
The period Tg in Eq. (
Then we focus on analyzing the impact of interaction on the density distribution in ψ(x;t) for 0 ≤ t ≤ Tg/4. The density distributions in ψ(x;t) and Φ(x;t) at t = 0,1.0,1.2, and 1.4 are shown in Fig.
 | Fig. 3. The time evolution of |ψ(x = 0;t)|2 (solid line) and |Φ(x = 0;t)|2 (dashed line) described by Eqs. ( |
 | Fig. 4. The density distribution in ψ(x;t) (solid line) and Φ(x;t) (dashed line) described by Eqs. ( |
The density distribution in ψ(x;t) and Φ(x;t) at t = 1.59 is shown in Fig.



At t = 1.59, the momentum of Φ(x;t = 1.59) is |P(1.59)| = 5.00. There is obvious difference between the density in Φ(x;t = 1.59) and ψ(x;t = 1.59). However, when the momentum is changed into P0 = 4.41, and d0 = 0.9223, d2 = 0.3681, d4 = 0.1178, the |Φt0|2 can coincide well with |ψ(x;t = 1.59)|2. This implies that even when two condensates are maximally overlapped, the condensates wavefunction ψ(x;t) can also be expanded with 
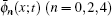
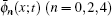
In this paper, we demonstrate that two condensates with repulsive interaction execute approximately harmonic motions. They form a periodic interference pattern, which can be expressed in terms of one-dimensional harmonic oscillator wavefunctions with optimal parameters. It is also noticeable that during time evolution of condensates, a small amount of atoms are transferred among three states ϕn (n = 0,2,4), which is due to the existence of interaction leading to the internal excitations of atoms. This atom transfer coincides with the energy change, for which the repulsive interaction tends to hold off atoms. Besides, dark solitons are generated when two condensates overlap. Our method provides a way to study the interaction-leading internal excitations of atoms.
| 1 | |
| 2 | |
| 3 | |
| 4 | |
| 5 | |
| 6 | |
| 7 | |
| 8 | |
| 9 | |
| 10 | |
| 11 | |
| 12 | |
| 13 | |
| 14 | |
| 15 | |
| 16 | |
| 17 | |
| 18 | |
| 19 | |
| 20 | |
| 21 | |
| 22 | |
| 23 | |
| 24 | |
| 25 | |
| 26 | |
| 27 |





