Cite this Article
Medjber Salim, Bekkar Hacene, Menouar Salah, Ryeol Choi Jeong. Testing the validity of the Ehrenfest theorem beyond simple static systems: Caldirola–Kanai oscillator driven by a time-dependent force. Chinese Physics B, 2016, 25(8): 080301  
Permissions
Testing the validity of the Ehrenfest theorem beyond simple static systems: Caldirola–Kanai oscillator driven by a time-dependent force
Medjber Salim1, Bekkar Hacene2, Menouar Salah3, Ryeol Choi Jeong4, †,
Department of Material Science, Faculty of Science, University of M’sila, M’sila, 28000, Algeria
Faculty of Technology, University of Ferhat Abbas Setif 1, Setif 19000, Algeria
Laboratory of Optoelectronics and Compounds (LOC), Department of Physics, Faculty of Science, University of Ferhat Abbas Setif 1, Setif 19000, Algeria
Department of Radiologic Technology, Daegu Health College, Buk-gu, Daegu 41453, Republic of Korea

 

† Corresponding author. E-mail: choiardor@hanmail.net

Project supported by Fund from the Algerian Ministry of Higher Education and Scientific Research (Grant No. CNEPRU/ D01220120010) and the Basic Science Research Program of the year 2015 through the National Research Foundation of Korea (NRF) funded by the Ministry of Education (Grant No. NRF-2013R1A1A2062907).

Abstract
Abstract

The relationship between quantum mechanics and classical mechanics is investigated by taking a Gaussian-type wave packet as a solution of the Schrödinger equation for the Caldirola–Kanai oscillator driven by a sinusoidal force. For this time-dependent system, quantum properties are studied by using the invariant theory of Lewis and Riesenfeld. In particular, we analyze time behaviors of quantum expectation values of position and momentum variables and compare them to those of the counterpart classical ones. Based on this, we check whether the Ehrenfest theorem which was originally developed in static quantum systems can be extended to such time-varying systems without problems.

PACS: 03.65.–w;03.65.Db;03.65.Sq;03.65.Ta
Keyword:Ehrenfest theorem;time-dependent Hamiltonian system;invariant theory
1. Introduction

As is well known, Planck’s novel theory for blackbody radiation found in 1900 led to the birth of the so-called quantum mechanics, which works on the basis of new principles that are very different from classical mechanics. Wave mechanics for quantum phenomena has been developed to date on the basis of manipulating probability interpretation with the Schrödinger wave equation for actual and particular systems. This led us to succeed in interpreting underlying mechanisms for various systems in the fields ranging from condensed matter physics to elementary particle physics with the support of rigorous experiments. Now, without quantum mechanics, we cannot understand the structure of solids, the color of lasers, the action of DNA, and the formation of the cosmos. The most basic and important task in this context is to find exact quantum solutions for a well-established physical model describing the behavior of a certain mechanical system. Much effort has been expended to develop quantum mechanics regarding this direction so far. A relationship between the quantum geometric phase and the classical Hannay angle has been found by Maamache and Bekkar.[1] Kim et al. showed that the dispersions of quantum states do not depend on external forces.[2] A solution of the Schrödinger equation for a time-dependent Hamiltonian system (TDHS) was obtained by Abdalla and Choi[3] and de Lima et al.[4] using linear invariants and quadratic invariants.

In spite of this remarkable progress for deriving quantum solutions, the correspondence of the quantum solutions with their counterpart classical ones until now has not been extensively checked for complicated Hamiltonian systems that have time-dependent parameters, while lots of efforts have been made to find a relationship between quantum and classical mechanics for simple static systems such as ordinary harmonic oscillator.[5–8] Historically, the efforts for finding such correspondence led to the Ehrenfest theorem.[9] The Ehrenfest theorem is that the time behavior of expectation values of canonical variables such as position and momentum for a quantized system follows the classical equations of motion. Actually, in case of any macroscopic systems, the quantum effects are less prominent and, consequently, it is expected in a particular limit that the quantum results reduce to those of the classical mechanics. The Ehrenfest theorem plays a crucial heuristic role in understanding the validity of quantum mechanics in describing certain physical systems.

The purpose of this work is to examine whether the Ehrenfest theorem is also valid for time-dependent quantum systems beyond simple static systems. A dissipative harmonic oscillator (Caldirola–Kanai oscillator) driven by a time-dependent force will be chosen for this purpose. Actual models of the most dynamical systems may suffer dissipation due to their various possible interactions with the environment. The details of the processes leading the dissipation mechanism are in general described by friction, viscosity, resistance, etc. On the other hand, the force term in the system makes the quantum motion become complicated. The time-dependent forced harmonic oscillator has been studied in detail by several research groups through different methods such as those using trial functions,[10] path integral, and propagator formulations,[11] Heisenberg picture approach,[12,13] coherent and squeezed state approach,[14] and so on. Nevertheless, it is a delicate problem to verify whether they are physically acceptable quantum descriptions for such time-dependent oscillators.

In order to investigate the Ehrenfest theorem for the system, we will derive one of interesting solutions of the Schrödinger equation, which is the one that corresponds to a semiclassical Gaussian wave packet. Gaussian wave packets are indeed ubiquitous in various physical systems and govern the time evolution of a large class of quantum wave motions. A useful technique for deriving this type of quantum solution for a dynamical system is developed by Ge and Child.[15] The expectation values of canonical variables will be derived and the validity of the Enrenfest theorem will be tested by making use of such a Gaussian wave packet in this work.

2. Fundamentals of the Ehrenfest theorem

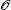
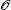
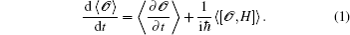
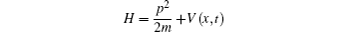
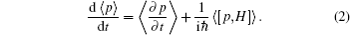
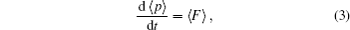
To understand the Ehrenfest theorem, let us consider an arbitrary quantum operator . If we denote the Hamiltonian of a system as H, the time derivative of the expectation value can be represented as

For the case that the system is subjected by a time-dependent potential V(x, t), the Hamiltonian has the form

in many cases. If is the momentum operator p, the above equation becomes

Now, from a minor evaluation, we can easily get the formula

where the external force F is of the form F = − dV(x, t)/dx. This is the Newton’s second law that governs the classical equations of motion for a particle.

In a similar way, we can also obtain the time derivative of the expectation value of the position operator such that

Indeed, equations (3) and (4) imply that the expectation values of quantum canonical variables yield the classical equations of motion. This concept is the core of the Ehrenfest theorem. Ehrenfest’s affirmation for the correspondence of quantum mechanics with Newton’s second law had a great appeal to the community of physics because it guarantees quantum mechanics as being a sound theory and thus acceptable. In the subsequent sections, we will investigate whether the expectation values of canonical variables for a general quantized TDHS obey the Ehrenfest theorem.

3. Classical treatment

Let us consider an arbitrary time-dependent harmonic oscillator driven by an external force f(t). In this case, the Hamiltonian can be written as

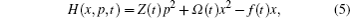
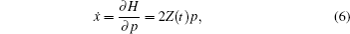
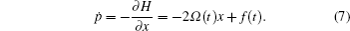
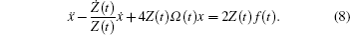
where Z(t) and Ω(t) are some time-dependent functions. If the time-dependence of Z(t) disappears, the first term in the right-hand side of Eq. (5) reduces to that of the system described in the previous section. The equations of motion for the system are

By combining these two equations, we can find the classical equation of motion for x as

Now, as an application to a particular system, let us choose the time functions to be

where Z0, Ω0, and F0 are constants and ω is a driving frequency. After the substitution of Z(t), Ω(t), and f(t) in Eq. (8), we obtain

This is the equation of motion for the driven Caldirola–Kanai oscillator. To investigate the solution of this equation, let us write the cosine term in the above equation as an exponential form, i.e.,

Let us consider a particular solution of the form xc = E eiωt for this equation, where E is a constant that will be determined afterwards. If we substitute this in Eq. (13), we find

Hence, the amplitude E in this case becomes

yielding a particular solution in the form

Here, the real part of the solution, that has the form

is physically meaningful. Similarly, the particular solution for the momentum can be obtained to be

Thus, the exact classical motion of the system is identified.

4. Quantum analysis

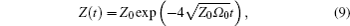
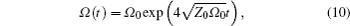
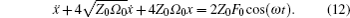
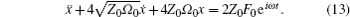
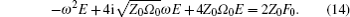
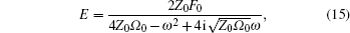
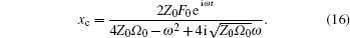
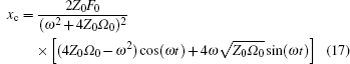
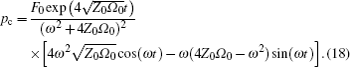
In the previous section, classical features of the system are described. Now we analyze the system in view of quantum mechanics. Ehrenfest derived an equation for the time behavior of expectation values of quantum observables, that can be regarded as a generalized Newton’s second law in quantum mechanics, through the consideration of the motion of the center of gravity of the wave packet for a particle.[9] His proof was relevant to only a one-particle-system that had a degree of freedom, but it was immediately extended to the case of a conservative system involving an arbitrary number of particles.[16]

Let us see now whether the Ehrenfest theory is equally extended to the driven Caldirola–Kanai oscillator which is a more generalized system characterized by a time-dependent driving force. Then, the problem is to find the solution of the Schrödinger equation:

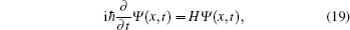
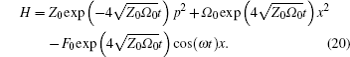
with the quantum Hamiltonian of the form

We can see from Eq. (20) that the system is a kind of TDHS. Even in the case where the driving force disappears (F0 = 0), the time-dependence of the Hamiltonian does not disappear. This means that a standard dissipative system with a constant damping factor is also a kind of TDHS.[13]

Because H is a function of time, it is not an easy task to solve Eq. (19) using a conventional separation of variables method. Hence, we will use an alternate method for solving the Schrödinger equation, which is the invariant operator method. Lewis and Riesenfeld[17] found that solutions of the Schrödinger equation with a time-dependent Hamiltonian can be solved by introducing a Hermitian invariant operator I(t). In general, I(t) is derived from the Liouville–von Neumann equation of the form

If we denote the eigenstates and eigenvalues of I(t) as φλ (x, t) and λ, the solution of the Schrödinger equation is represented in the form

where αλ (t) is a phase that satisfies an eigenequation, which is

Usually, the phase αλ (t) consists of a dynamical phase and a geometrical phase. Among them, the geometrical phase for a certain quantum system can be applied to implementing geometric phase gates in quantum computation protocols.[18,19]

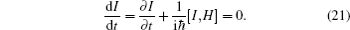
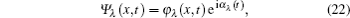
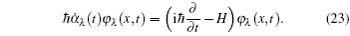
In this task, we take a linear time-dependent type of the invariant operator, such that

By inserting this formula into Eq. (21),[20,21] we can easily obtain the differential equation for A(t) as

The solution of this equation is easily identified to be

Then, from the fundamental relations for the invariant operator, we also get other time variables of I(t) as

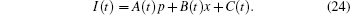
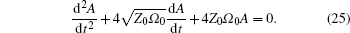
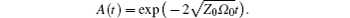
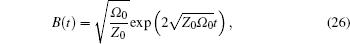
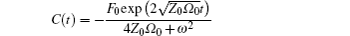
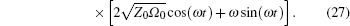
Thus, the explicit formula of I(t) for our system is identified. The eigenfunction φλ (x, t) of I(t) can be derived from

Once the formula of φλ (x, t) is obtained by solving this, the form of the phase αλ (t), under the initial condition αλ (0) = 0, is also derivable by an evaluation of the Schrödinger equation after inserting Eq. (22) with the complete expression of φλ (x, t) into Eq. (19). When the wave function Ψλ (x, t) is derived, we use the formula

which gives a Gaussian wave function,[20] where g(λ) is a weight function. In this procedure, we choose g(λ) = bexp(−λ2)[21] for simplicity. Then, finally, the complete wave function is obtained and it is given in the form

where

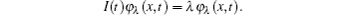
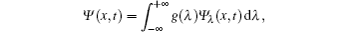
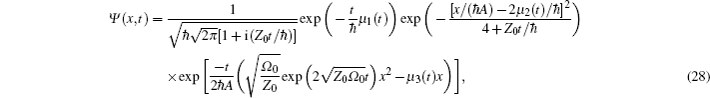
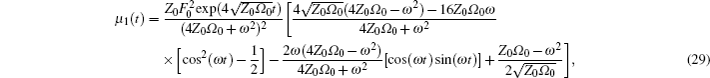
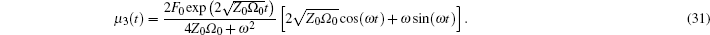
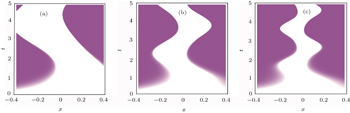
This wave function enables us to study various quantum features of the system. The absolute square of Eq. (28), |Ψ(x, t)|2, is the probability density, where its meaning is the probability for finding the oscillator in position x at time t. We have depicted it in Fig. 1.

Fig. 1. The time evolution of the probability density |Ψ(x, t)|2 with ω = 1 for panel (a), ω = 2 for panel (b), and ω = 3 for panel (c). We used Z0 = 0.1, Ω0 = 1, F0 = 1, and ħ = 1. This figure is a density plot produced using the Mathematica program (Wolfram Research).

We see from this figure that the centroid of the wave packet oscillates back and forth as time goes by like a classical state. In fact, it follows classical trajectory of the oscillator. We can also see from this figure that the oscillating amplitude of the wave packet decreases over time. This means that there is a dissipation of energy of the oscillator due to the damping factor, 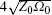, given in Eq. (12). The method for producing Fig. 1 is represented in Appendix A.

5. Testing the Ehrenfest theorem

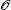
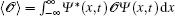
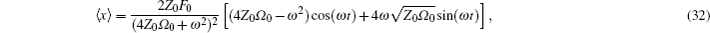
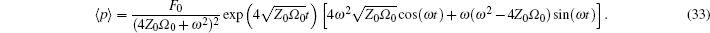
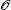
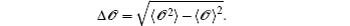
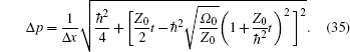
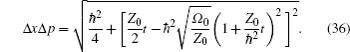
The study of time behavior of expectation values of canonical variables is necessary in order to investigate the validity of the Ehrenfest theorem. As is well known, for an arbitrary observable , the expectation value in the quantum state is evaluated from . For the case of x and p, we obtain (see Appendix B)

Further, the uncertainty for an operator can be derived from

For the case of x and p, we have

The product of these two quantities leads to the uncertainty relation for the system such that

Notice that this product is always larger than the minimally accepted value, ħ/2, in quantum mechanics as expected.

Now we test the validity of the Ehrenfest theorem for the system using the results obtained from the analysis of quantum theory developed so far. The time derivative of Eqs. (32) and (33) yields

We easily confirm that these results are exactly the formulas that are predicted by the Ehrenfest theorem for the TDHS. Hence, the Ehrenfest theorem is satisfied. This implies that there is a fundamental coincidence between the expectation values of position and momentum operators in quantum mechanics and their corresponding classical equations of motion.

6. Conclusion

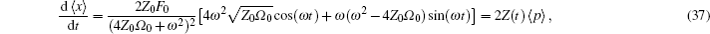
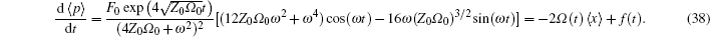
From the explicit evaluation of the expectation values of canonical variables, we have confirmed that the Ehrenfest theorem, that states the universal correspondence between the theory of quantum mechanics and classical mechanics, can be extended to the driven Caldirola–Kanai oscillator which is a more general time-dependent quantum system. By comparing quantum results for the time behaviors of averaged position and momentum, given in Eqs. (32) and (33), with those of classical position and momentum, Eqs. (17) and (18), we conclude that 〈x〉 = xc and 〈p〉 = pc. The extendibility of the Ehrenfest theorem to more general quantum systems that have time-varying parameters is crucial as a supporting theory to the universal validity of quantum mechanics.

In the mean time, the results of our research do not imply that the Ehrenfest theorem is always applicable in any quantum system. It is known that the Ehrenfest theorem is violated in some cases, such as the system described by the Galilean-invariant Schrödinger equations that have nonlinear terms which couple the phase and the amplitude in wave functions.[22] This may imply that there is a difficulty for logical generalizations of quantum mechanics in a consistent way, especially via possible nonlinear corrections in quantum mechanics. A theoretical test of quantum mechanics along this line is performed by Weinberg.[23]

The understanding of quantum mechanics can be deepened by studying the Ehrenfest theorem extended to more generalized systems. The mechanism of time-dependent phenomena for a complicated system can be understood by this extension within quantum mechanics. From a conceptual point of view, quantum mechanics cannot be reduced to classical mechanics (Newtonian mechanics) by the Ehrenfest theorem, but it merely makes quantum results analogous to classical ones.[24] Recall that quantum mechanics is constructed on the basis of intrinsically different foundations from the classical mechanics and its manipulations used for unfolding the theory are totally different from the classical ones. In modern physics, it is known that the classical realm of the behavior of a mechanical system is emergent from the quantum world, ruled by the Schrödinger equation, via a particular process which is called “decoherence”. However, the exact mechanism for the transition from quantum mechanics to classical mechanics is not completely known yet.

Reference
1Maamache MBekkar H 2003 J. Phys. A: Math. Gen. 36 L359
2Kim H CLee M HJi J YKim J K 1996 Phys. Rev. 53 3767
3Abdalla M SChoi J R 2007 Ann. Phys. 322 2795
4de Lima A LRosas APedrosa I A 2008 Ann. Phys. 323 2253
5Planck M1967“The Genesis and Present State of Developement of the Quantum Theory”Nobel Lectures: Physics 1901–1921AmsterdamThe Nobel Foundation
6Heilbron J L2000The Dilemmas of an Upright Man: Max Planck and the Fortunes of German ScienceCambridgeHarvard University Press0674004396
7Pickover C2008Archimedes to Hawking: Laws of Sciences and Great Minds Behind ThemOxfordOxford University Press9780195336115
8Gamow G1985Thirty Years that Shook Physics: The Story of Quantum TheoryDoverDover Publications
9Ehrenfest P 1927 Zeits. f. Physik 45 455
10Gao X CXu J BQian T Z 1990 Ann. Phys. 204 235
11Yüce C 2003 Ann. Phys. 308 599
12Colegrave R KAbdalla M S 1981 Opt. Act. 28 495
13Choi J R 2004 Int. J. Theor. Phys. 43 2113
14Nassar A B 1984 Phys. Lett. 106 43
15Ge Y GChild M S 1997 Phys. Rev. Lett. 78 2507
16Ruark A E 1928 Phys. Rev. 31 533
17Lewis H RJrRiesenfeld W B 1969 J. Math. Phys. 10 1458
18Das RKumar S K KKumar A2006J. Magn. Reson.177318
19Wu L ABishop C AByrd M S 2011 Phys. Rev. 84 022341
20Bekkar HBenamira FMaamache M 2003 Phys. Rev. 68 016101
21Maamache MSaadi SChoi J RYeon K H 2010 J. Korean Phys. Soc. 56 1063
22Kälbermann G 2004 J. Phys. A: Math. Gen. 37 2999
23Weinberg S 1989 Ann. Phys. 194 336
24Jammer M1966The Conceptual Development of Quantum MechanicsNew YorkTomash Publishers