† Corresponding author. E-mail:
Project supported by the Research Foundation of Jinling Institute of Technology, China (Grant No. JIT-B-201426), the Jiangsu Modern Education and Technology Key Project, China (Grant No. 2014-R-31984), the Jiangsu 333 Project Funded Research Project, China (Grant No. BRA2010004), and the University Science Research Project of Jiangsu Province, China (Grant No. 15KJB520010).
Reduction of electromagnetic scattering from a conducting cylinder could be achieved by covering it with optimized multilayers of normal dielectric and plasmonic material. The plasmonic material with intrinsic losses could degrade the cloaking effect. Using a genetic algorithm, we present the optimized design of loss and gain multilayers for reduction of the scattering from a perfect conducting cylinder. This multilayered structure is theoretically and numerically analyzed when the plasmonic material with low loss and high loss respectively is considered. We demonstrate by full-wave simulation that the optimized nonmagnetic gain-loss design can greatly compensate the decreased cloaking effect caused by loss material, which facilitates the realization of practical electromagnetic cloaking, especially in the optical range.
In 2006, transformation optics (TO), first proposed by Pendry et al., was introduced as a technique to manipulate the electromagnetic (EM) wave propagation.[1] TO can be used to design different kinds of novel EM devices, and the most significant device designed by TO is the invisibility cloak which can conceal an object from detection by EM illumination.[2,3] Apart from various theories on the cloak that have been analyzed extensively, some experimental verifications have also been reported from microwave to optical frequencies with assistance of artificial EM materials.[4,5] As the magnetic responses of natural materials, even artificial EM materials, are weak in the optical regime, a reduced set of medium parameters has been proposed to solve the problem of optical cloaking, which is still difficult to fabricate practically.[5]
Another approach to cloaking is to use layers of isotropic plasmonic shells or metamaterials to cover the cloaked object.[6–8] This approach based on scattering cancellation mechanism can drastically reduce the total scattering cross section of an object, which is only limited to the dimension of the object much smaller than the EM wavelength. Moreover, the optimization procedure used to design layers of non-magnetic moderate-size cloaking shells can achieve satisfactory cloaking performance.[9,10] However, these optimized shells with layers of anisotropic metamaterials for hiding a perfect electric conductor (PEC) cylinder are difficult to fabricate in practice. Afterwards, Yu proposed optimized cylindrical multilayers composed of isotropic dielectric and plasmonic material.[11] Besides, another optimized design made of normal dielectrics was proposed, which further simplifies the fabrication but induces a noticeable scattering when the radius of the cloaked target is compared with the working wavelength.[12]
To achieve good cloaking performance, we continue the optimized studies on designing non-magnetic multi-layers made of gain and loss materials through a genetic algorithm (GA). In our previous work, lossless plasmonic materials have been used with permittivities far less than unity which are always accompanied with losses.[11] As reported in Han’s paper, the gain-assisted method can be used to overcome the loss problem of the invisibility cloak.[13] Therefore, considering introduction gain in the dielectric material to compensate the loss in the plasmonic material, we demonstrate that the optimized gain-loss shells can yield much better performance than cloaking shells designed with only lossy materials.
For simplicity, we consider a two-dimensional cylindrical multilayer constructed alternately by two kinds of non-magnetic materials: one is a lossy plasmonic material, the other is a dielectric gain material. Let us assume that a TM uniform plane wave is traveling in the +x direction upon the multi-layer cylindrical structure with inner radius a and outer radius b, as depicted in Fig.
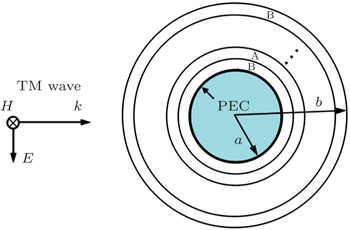 | Fig. 1. TM plane wave incident on a PEC cylinder surrounded by multi-layers of alternating material A and material B. The inner and outer radii of the coating are a and b, respectively. |
The form of time-harmonic variation is represented by ejωt throughout this paper. The normalized magnetic field polarized along the cylinder axis can be written as 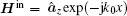
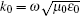
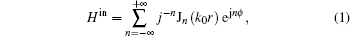
The scattered magnetic field from the multilayers can be represented by the sum of Hankel functions of the second kind as
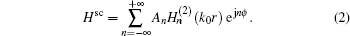
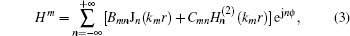
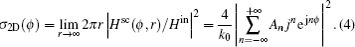
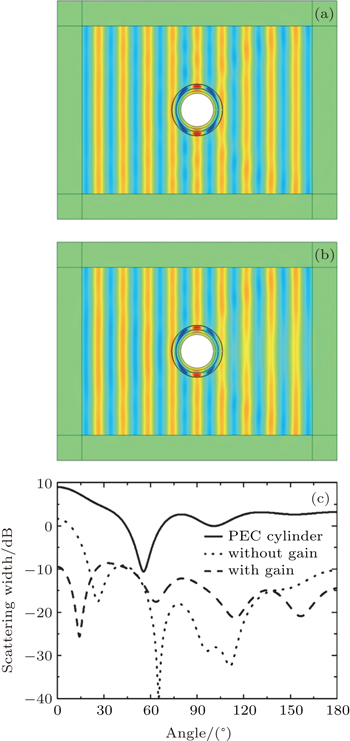
To simplify the multilayered structure, we consider a five-layer structure composed of two layers of dielectric gain material A sandwiched by three layers of lossy plasmonic material B. The complex permittivities of the gain and loss materials can be expressed as 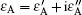
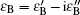
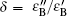
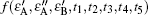
| Table 1. The permittivity and thickness for the optimized low-loss five-layer structure with gain material. . |
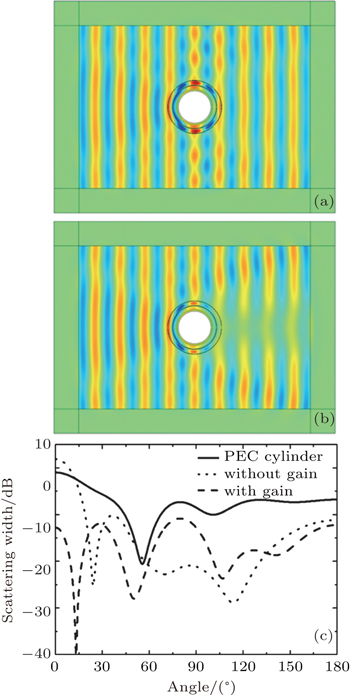
As to the second example, we study the case of high loss with loss tangent 0.5. Through optimization, the complex permittivities of the gain material and the loss plasmonic material are calculated with resulting values ɛA = 5.158 + i1.131 and ɛB = 0.0355(1 − 0.5i), respectively. The field profiles and the far-field SW for the multilayered cover using the optimized parameters from Table
| Table 2. The relative permittivity and thickness for the optimized high-loss five-layer structure with gain material. . |
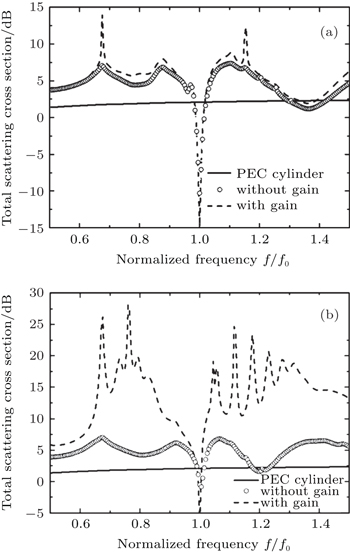
The plasmonic material always disperses heavily as frequency changes. Here, the plasmonic material is supposed to follow the Drude model with 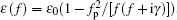
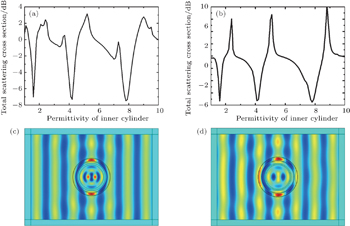
What happens if we exchange the inner PEC cylinder for a dielectric one? We plot the dependency of normalized total scattering cross section on the permittivity of the inner dielectric cylinder. Although the original purpose of the optimized multilayered cover is to conceal the inner PEC cylinder, it is amazing to find that the multilayers are also effective to reduce the scattering of the inner dielectric core with some specific permittivities, shown in Fig.
The isotropic plasmonic material with relative permittivity close to zero in this design is available at infrared and visible ranges, for example, the noble metals, polar dielectrics, some semiconductors, and even composite materials realized by embedding metallic nano-particles and nano-wires into dielectrics.[16–19] These plasmonic materials, in general, are unavoidably endured with losses at infrared or visible wavelengths, which will affect the cloaking performance of the optimized multilayers. This troublesome problem could be conquered by introducing proper gain in the dielectric material. The gain can be achieved by quantum dots and III–V semiconductors at long wavelengths (about 1500 nm) and by dye at short wavelengths (for example, PMMA with Rhodamine 6G dye at wavelength of 594 nm).[20] Moreover, giant gain coefficients of 6755 cm−1 and 6.8× 104 cm−1 are reported for a CdSe nano-belt and a layer of InAs/GaAs quantum dot, respectively.[21,22] These gain values indicate that the gain materials are expected to achieve the isotropic gain-assisted cloaking device.
In conclusion, we have studied the optimization design of alternating layers of isotropic gain and loss materials, which can overcome the loss problem in the invisibility of PEC core. We have demonstrated by full-wave EM simulation that the performance of the optimized isotropic gain-loss cloak is much better than that of the one without adding gain materials, especially in the reduction of the forward EM scattering. Moreover, when in the inner domain we replace the PEC cylinder with a dielectric material of some specific permittivities, the optimized multilayers are also effective to reduce the EM scattering. This optimized design approach by introducing the gain material properly can compensate the plasmonic material’s loss, which can be extended to design other transformation optics devices.
| 1 | |
| 2 | |
| 3 | |
| 4 | |
| 5 | |
| 6 | |
| 7 | |
| 8 | |
| 9 | |
| 10 | |
| 11 | |
| 12 | |
| 13 | |
| 14 | |
| 15 | |
| 16 | |
| 17 | |
| 18 | |
| 19 | |
| 20 | |
| 21 | |
| 22 |