Cite this Article
Parida Priyadarshini, Ganguli Biplab. Electronic and magnetic properties at rough alloyed interfaces of Fe/Co on Au substrates: An augmented space study. Chinese Physics B, 2016, 25(5): 057302  
Permissions
Electronic and magnetic properties at rough alloyed interfaces of Fe/Co on Au substrates: An augmented space study
Parida Priyadarshini, Ganguli Biplab†,
Department of Physics & Astronomy, National Institute of Technology Rourkela, Rourkela 769008, India

 

† Corresponding author. E-mail: biplabg@nitrkl.ac.in

Project supported by the INSPIRE Program Division, Department of Science and Technology, India.

Abstract
Abstract

We studied the interface electronic and magnetic properties of Fe/Co deposited on Au substrate and researched the effects of roughness at the interfaces within augmented space formalism (ASF). The full calculation is carried out by recursion and tight-binding linear muffin tin orbital (TB-LMTO) methods. The amount of roughness is different at different atomic layers. The formalism is also applied to sharp interface, when interdiffusion of atoms is negligible. Our results of one monolayer transition metal agree with other reported results. A realistic rough interface is also modeled with three and four monolayers of transition metals, deposited on Au substrates.

PACS: 73.20.At;75.20.En;
Keyword:augmented space formalism;tight-binding linear muffin tin orbital;rough interface;magnetic properties;
1. Introduction

The interface properties of transition metals and metals significantly contribute to technological applications such as magnetic recording devices and sensors.[1–4] Therefore, the study of layerwise properties at the interface is important.

When a transition metal is deposited on a metal substrate, there is interdiffusion of atoms at the interface due to the difference between their surface energies.[5] When Fe is deposited on Au substrate, Fe atoms are found to segregate[5] and the intermixing of Fe and Au atoms is observed experimentally.[5,6] This intermixing of atoms at the interface leads to rough interface, which is also observed experimentally in this system.[7] Sharp interfaces of Fe/Au with only one Fe monolayer have been considered in various theoretical studies.[8–10] While, in this paper, we studied both sharp and rough layerwise interface properties to research the effect of roughness. The main objective of this paper is to study rough interface properties with three and four ML transition metal depositions on semi-infinite Au substrate. Augmented space formalism (ASF) had also been applied earlier[11] to study the Fe/Au interface with one ML of Fe. Therefore, we have also considered one monolayer (ML) with both sharp and rough interfaces to compare our result with the results in earlier literature.[8–11] Three ML case or more monolayer cases are realistic from the experimental point of view. In our recent work[12] on three MLs of Fe on Ag substrate with rough interface, the result obtained on magnetic properties agrees well with the experimental observations. Therefore we have modeled a realistic rough interface with three and four ML depositions. In this four ML case, the interface is defined as four alloyed layers, two from the transition metal side, and two from the Au side. The interdiffusion of atoms is considered into these four interface layers.

2. Computational methods

Augmented space formalism[13–16] is a suitable technique to deal with binary disordered alloys, and this formalism has been applied successfully to rough surface and alloyed interfaces.[12,17–19] To deal with the first principle calculation, ASF is combined with the TB-LMTO method. The Hamiltonian in TB-LMTO basis[20] is

Here, R is the lattice sites and L = (lm) are the orbital indices. For transition metal, l < 2. The and are the TB-LMTO potential parameters of the constituents A and B of the alloy. The potential parameters are generated from TB-LMTO within local spin density approximation (LSDA) using Barth and Hedin exchange correlation potential.[21]

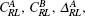
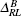
The augmented Hamiltonian[16] is

where

and Ĩ is the identity operator in the augmented space, M̃R is the operator in the configuration space, and M̃R in the second quantized notation as

where and are the creation and annihilation operators in the augmented space.[22]

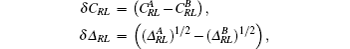
When the recursion method[23] is applied to the augmented Hamiltonian, the averaged Green function can be written in the form of a continued fraction. Infinite continued fraction is to be terminated after a finite terms using a suitable terminator

and the density of states (DOS) is given by

where α and β are recursive parameters and T(z) is the terminator. In our calculations, we used Luchini and Nex terminator.[24]

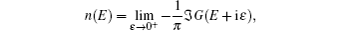
ASF has been successfully extended to study the properties of almost smooth surfaces and sharp interfaces in the limiting case when roughness is negligible,[12,17–19] by using Viswanath and Müller[25,26] terminator. In our calculations, we used eight-shell augmented space calculation and nine steps of recursion.

3. Results and discussion

Fe is deposited on Au in its bcc phases and there is a large lattice mismatch (42%) between bcc Fe and fcc Au. When Fe is deposited on Au, the layers of bcc Fe are rotated by 45˚ with respect to the Au (001) plane,[5,27–30] which reduces the lattice mismatch to only 0.6%. However, Co is deposited on Au in its fcc phase and there is also 15% lattice mismatch between Co and Au. Therefore, the lattice parameter of the Co overlayer relaxes and it is obtained by energy minimum principle. The relaxed parameters are within 2% lattice mismatch. In our calculations, we considered these relaxed parameters.

3.1. One monolayer of transition metals

Figure 1 shows the layerwise density of states (DOS). The spin-polarized DOS shows the amount of splitting in spin-up and spin-down electronic states, the nature of hybridization and its contribution towards the magnetic moments for the transition metal overlayer. The splitting of spin-up and down states is more for Fe overlayers. The peaks of transition metal atoms do not coincide with those of Au substrate atoms at the S − 1 layer. This means that the sp–d hybridization between Fe/Co and Au is weak and narrows down the Fe/Co bands. Therefore, the magnetic moment of transition metal on Au increases (Table 1). The spin-down band is wider than the spin-up band in both cases. The spin-up states contribute more to the magnetic moment in these systems. The spin-resolved DOS for the top Fe layer in the Fe/Au(001) system matches well with the earlier results calculated with the FPLAPW method.[10] The interface spin DOS for Fe also matches with the earlier study results carried out using DFT.[30] The width of DOS increases from the top most layer towards inner atomic layers. At the fourth substrate layer (S − 4), the DOS approaches bulk Au DOS.

Fig. 1. Layerwise variation of DOSs for one ML of transition metal (Fe and Co) on Au metal substrates from top most (S) layer to inner atomic layers. Fermi level is reset at zero. (a) Fe/Au(001), (b) Co/Au(001).

Table 1 shows layerwise magnetic moments for one ML of transition metals. The magnetic moments of the top most transition metal overlayers increase compared to bulk Fe and Co values. The obtained magnetic moment of the top most (S) layer as well as the induced moment at the first substrate (S − 1) layer agrees with the earlier results obtained by the FPLAPW method.[8–10] This shows that ASF is useful to study sharp interfaces. The induced magnetic moment gradually decreases and ceases to zero at the fourth substrate layer. The magnetic moment of the top most layer in this case is more compared to the magnetic moment of smooth Fe and Co surfaces.[18] This is because the sp–d hybridization between transition metal and metal substrate is weak compared to the 3d–3d hybridization between transition metal layers.

Table 1.

Layerwise magnetic moments (μ) per atom for one layer of transition metals deposited on Au substrate. Here, S represents the top most layer of the interface. x = 0.0 (sharp interface). Comparison is done with the FPLAPW method.

.

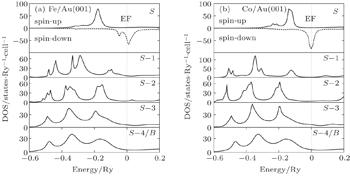
The roughness in this one ML case is considered with 5% and 10% interdiffusion of atoms. Figure 2 shows the effect of roughness on the DOS at the interface layer(sS and S − 1). The amount of both spin-up and spin-down electronic states of the top most layer decreases with the increase of the Au atoms in the case of Fe/Au. Whereas in the case of Co/Au, the spin-up DOS increases when 5% Au is introduced. With further increasing Au concentration to 10%, the spin-up states in the lower energy region increase but those close to Fermi energy decrease in the case of Co/Au. In Au rich layers (S − 1), the amount of DOS decreases with the increase in transition metal atoms.

Fig. 2. Layerwise spin-polarized DOS for one layer of Fe/Co deposited on Au substrate. Here, S and S − 1 represent interface layers. (a) and (d) x = 0 (sharp interface), (b) and (e) x = 0.05, and (c) and (f) x = 0.10 (rough interface). Solid line: spin-up DOS. Dotted line: spin-down DOS. Fermi level is reset at zero.

Tables 2 and 3 show the effect of roughness at the interface with 5% and 10% interdiffusion of atoms respectively. With the increase of Au concentration in Fe and Co top most layers (S), the average magnetic moment decreases, whereas, the average induced magnetic moment at the first substrate layer (S − 1) increases. This is due to the increase in transition metal atoms in the S − 1 layer. Our results match with the earlier results.[11] In these cases, the induced magnetic moment also ceases to zero at the fourth substrate layers (S − 4), as in the case with a sharp interface.

Table 2.

Layerwise magnetic moments (μ) per atom for one layer (S) of transition metals on Au metal substrate. Here, S and S − 1 are T1−xMx and M1−xTx respectively with T and M standing for transition metal and metal atoms respectively, M = Au and x = 0.05 (rough interface). Comparison is done with ASF without surface relaxation.

.
Table 3.

Layerwise magnetic moments (μ) per atom for one layer (S) of transition metals on Au metal substrate. Here, S and S − 1 are T1−xMx and M1−xTx respectively with T and M standing for transition metal and metal atoms respectively, M = Au and x = 0.10 (rough interface). Comparison is done with ASF without surface relaxation.

.
3.2. Three monolayers of transition metals

In our earlier work,[12] the obtained rough interface magnetic properties with 5% interdiffusion of atoms for three MLs of Fe on Ag substrates agree with the results in the experimental work.[31,32] Therefore, the study of three ML depositions on Au substrates is also important. There are no experimental and theoretical studies reported for Fe and Co on Au substrate in this for comparison. Table 4 shows the layerwise variation of magnetic moment with sharp interface when interdiffusion is negligible. The magnetic moment of the top most Fe layer is the same as that of one ML case.

Table 4.

Layerwise magnetic moments (μ) per atom for three MLs of Fe/Co on Au. S stands for the top most layer, S − 2 and S − 3 are sharp interface layers (x = 0.0).

.

Table 5 shows the average and interface magnetic moments, both for sharp and rough interfaces. The average magnetic moments as well as the interface transition metal magnetic moment reduce with the increase in roughness.

Table 5.

Calculated average and interface magnetic moments (μ) per atom for three layers of transition metals on Au substrates.

.
3.3. Four layered rough interface

Next, we modeled a more realistic case where we considered four ML depositions of transition metals on Au substrates. The rough interface is considered as four alloyed layers, two from transition metal layers and the other two from metal substrate layers. In these four layers, the transition metal atoms and metal atoms interdiffuse into each other. Therefore, the interface is defined by four layers I + 2, I + 1, I − 1, and I − 2. The host atom at layers I + 2 and I + 1 is transition metal atom, whereas the host at layers I − 1 and I − 2 is substrate metal atoms. The composition of the alloyed interface at different layers from top to bottom are T95M5, T90M10, M90T10, and M95T5, respectively. Here, T and M stand for the transition metal atom (Fe/Co) and metal substrate atom (Au), respectively.

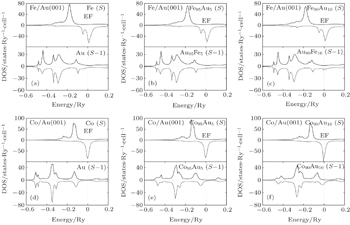
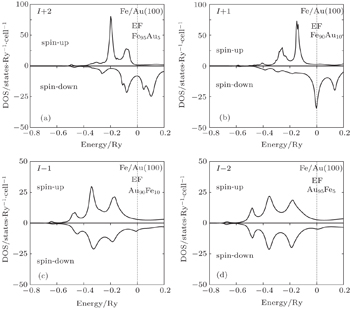Figures 3 and 4 show the variation DOS at the four-layered rough interface for Fe and Co overlayers respectively. With the increase in Au concentration from 5% to 10% at Fe/Co rich layers at the interface, both spin-up and spin-down electronic states show significant change. However, at Au rich layers (I − 1 and I − 2), the splitting of spin-up and spin-down electronic states decreases with the decrease in Fe concentration.

Fig. 3. Layerwise variation of spin DOS at Fe/Au(001) with rough alloyed interface. Fermi energy is reset at zero. (a) Fe95Au5, (b) Fe90Au10, (c) Au90Fe10, and (d) Au95Fe5.
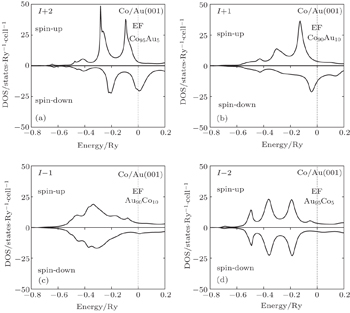Fig. 4. Layerwise variation of spin DOS at Co/Au(001) with rough alloyed interface. Fermi energy is reset at zero. (a) Co95Au5, (b) Co90Au10, (c) Au90Co10, and (d) Au95Co5.

In the case of Fe/Au, the spin-up electronic states at the lower energy region become significant in the I + 2 layer (Fig. 3). This is because of the presence of Au impurities. As the Au impurities in Fe layers increase from 5% to 10%, there is significant change in both spin-up and spin-down DOS. With the increase in the Au amount in the Fe layer, the spin-up electronic states near the Fermi level increase and spin-down electronic states are maximum at the Fermi level. In Au layers with Fe impurities, the peaks are shifted towards the lower energy region as for the bulk Au. The splitting of spin-up and spin-down electronic states decreases in these regions (I − 1 and I − 2 layers). Bulk Au DOS is obtained at the fifth substrate layer (I − 5) from the interface. The DOS of Fe rich I + 1 and Au rich I − 1 layers match well with the results in the earlier work.[30]

The spin-up and spin-down electronic states are more at the Co top layer in the case of the Co/Au system (Fig. 4). The states decrease at the next layer which reduces the Co magnetic moment. When Au impurities increase from 5% to 10%, the spin-up states contribute more to the magnetic moment than the spin-down states. In the region away from Fermi level, electronic states are more affected with the increase of Au atoms at Co atomic layers. DOS attains bulk Au DOS at the fifth substrate layer (I − 5) from the interface.

The effect of roughness is shown in Table 6. The transition metal layers with 5% Au have more magnetic moments compared to that with 10% Au. However, at Au rich layers, the magnetic moment of the I − 1 layer is more compared to that of the I − 2 layer. That is because there are more transition metal atoms in the I − 1 layer, the induced magnetic moments due to Fe/Co are more. The magnetic moment of the Co atom as well as the average magnetic moment of the I + 1 layer is less than its bulk value. This is due to the increase in hybridization between Co and Au atoms. The layerwise magnetic moments at transition metal layers (I + 4 to I + 1) show oscillatory behavior but that in Au substrate layers (I − 1 to I − 5) gradually ceases to zero. The magnetic moment is zero at the fifth substrate (I − 5) layer. Therefore, Au attains bulk properties at the fifth substrate layer for both the systems.

Table 6.

Layerwise magnetic moment (μ) per atom at the four-layer rough interface of two layers of transition metal plus two layers Au substrates. I: boundary of transition metal and Au substrate. Interdiffusion, x = 0.1 at I ± 1 layer and x = 0.05 at I ± 2 layers, and x = 0 at other layers.

.
4. Conclusion

In this paper, augmented space formalism coupled with the recursion method and the TB-LMTO method has been applied to carry out the layer-based density of states and magnetic moments of rough and sharp interfaces of Fe/Co on Au(001) metal substrates. The calculated DOS and magnetic moment for a sharp interface with one ML deposition agrees well with the results by other first principle calculations. The effects of roughness on DOS and magnetic moments are discussed for one, three, and four ML deposition cases. The obtained rough interface properties with one ML Fe/Co agree with other reported results. The average and interface transition metal magnetic moments get reduced with the increase in roughness in three ML cases. The layerwise magnetic moments cease to zero at the fourth substrate layer in the case of one and three ML depositions, whereas, they reduce to zero at the fifth substrate layer in the case of the four layered interface with four ML depositions.

Reference
1Sellmyer D JZheng MSkomski R 2001 J. Phys.: Condens. Metter 13 R433
2Csontos MBalogh JKaptas DKiss L FKovacs AMihaly G 2006 Phys. Rev. 73 184412
3Granroth SKnut RMarcellini MAndersson GSvensson SKaris OGorgoi MSchafers FBraun WEberhardt WOlovsson WHolmstrom EMartensson N 2009 Phys. Rev. 80 094104
4Falicov L MPierce D TBader S DGronsky RHathaway K BHopster H JLambeth D NParkin S S PPrinz GSalamon MSchuller I KVictora R H 1990 J. Mater. Res. 5 1299
5Begley A MKim S KQuinn JJona FOver HMarcus P M 1993 Phys. Rev. 48 1779
6Blum VRath ChMuller SHammer LHeinz KGarcia J MOrtega J EPrieto J EHernan O SGallego J Mde Parga A L VMiranda R 1999 Phys. Rev. 59 15966
7Bischoff M M JYamada TQuinn A Jvan der Krann R G Pvan Kempen H 2001 Phys. Rev. Lett. 87 246102
8Li CFreeman A JJansen H J FFu C L 1990 Phys. Rev. 42 5433
9Li CFreeman A JFu C L 1990 J. Magn. Magn. Mater. 83 51
10Li CFreeman A JFu C L 1988 J. Magn. Magn. Mater. 75 201
11Sanyal B 2001 Computational Mater. Sci. 20 429
12Parida PGanguli BMookerjee A 2015 J. Magn. Magn. Mater. 381 422
13Mookerjee A 1973 J.Phys. C: Solid state Phys. 6 L205
14Mookerjee A 1973 J.Phys. C: Solid state Phys. 6 1340
15Banerjee RMookerjee A 2010 Int. J. Mod. Phys. 21 205
16Mookerjee ASarma D D2003Electronic Structure of Alloys, Surfaces and ClustersLondon and New YorkTaylor-Francis71
17Parida PGanguli B2014Int. J. Sci. Eng. Res.581
18Parida PGanguli BMookerjee A 2015 Superlattices and Microstructures 86 173
19Parida PGanguli B 2015 Thin Solid Films 594 24
20Andersen O K 1975 Phys. Rev. 12 3060
21von Barth UHedin L 1972 J.Phys.C: Solid State Phys. 5 1629
22Saha TDasgupta IMookerjee A 1996 J. Phys.: Condens. Matter 8 1979
23Haydock RHeine VKelly M J 1972 J. Phys. C: Solid State Phys. 5 2845
24Luchini M UNex C M M 1987 J. Phys. C: Solid State Phys. 20 3125
25Viswanath V SMuller G 1990 J. Appl.Phys. 67 5486
26Viswanath V SMuller G1994The Recursion Method: Application to Many-body DynamicsSpringer-Verlag109
27Freeman A JWu R 1991 J. Magn. Magn. Mater. 100 497
28Freeman A JFu C LOguchi T 1985 Mat. Res. Soc. Symp. Proc. 63 1
29Freeman A JFu C L 1987 J. Appl. Phys. 61 3356
30Benoit MLanglois CCombe NTang HCasanove M J 2012 Phys. Rev. 86 075460
31Wooten C LChen JMulhollan G AErskine J LMarkert J T 1994 Phys. Rev. 49 10023
32Hahlin AAndersson CHunter D JSanyal BKaris OArvanitis D 2006 Phys. Rev. 73 134423