Many interesting phenomena can be produced by using the pressure, such as semiconductor to metal,^[1] conventional insulator to topological insulator,^[2–4] and ferromagnetic semiconductor to spin gapless semiconductor^[5] transitions. Exploring the magnetic coupling mechanism in magnetic materials has always been a fundamental topic.^[6–10] The exchange coupling in transition-metal oxides can be understood by the superexchange interaction,^[6] and the double-exchange mechanism^[7] which can explain the metallicity and ferromagnetic coupling in the mixed-valence Mn compounds.

The inverse-trirutile compounds Cr₂XO₆ (X = W, Te, Mo) with space group P4₂/mnm are antiferromagnetic insulators, in which the antiferromagnetic superexchange interaction cannot give an outright explanation about the magnetic coupling of Cr atoms.^[11–13] Their magnetic structures, namely the Cr–O–Cr magnetic coupling, depend on the X atom. In Cr₂XO₆ (X = W, Mo), the inter-layer Cr atoms are antiferromagnetically coupled, while the neighboring intra-bilayer Cr atoms are ferromagnetically aligned. However, the neighboring intra-bilayer Cr atoms are antiferromagnetically aligned in Cr₂TeO₆. The novel intra-bilayer ferromagnetic coupling can be explained by assuming a virtual transfer between O and W (Mo) atoms which tunes the orbital hybridization between Cr-d and O-p orbitals.^[12,13]

Here, we investigate magnetic ordering change of inter-bilayer and intra-bilayer Cr atoms of Cr₂MoO₆ under hydrostatic pressure. The electronic structures are calculated by using Tran and Blaha’s modified Becke and Johnson (mBJ) exchange potential, which describes properly the gaps and positions of d electrons of Cr₂MoO₆. The calculated results show that the inter-bilayer magnetic coupling changes from antiferromagnetic coupling to ferromagnetic, and the intra-bilayer coupling changes from ferromagnetic ordering to antiferromagnetic. When the magnetic phase transition happens, a semiconductor–metal phase transition also occurs. The magnetic phase transition can be understood by the intra-bilayer ferromagnetic coupling mechanism proposed by Zhu et al. in Refs. [12] and [13].

The rest of the paper is organized as follows. In the next section, we shall give our computational details. In Section 3, we shall present our main calculated results and analysis. Finally, we shall give our conclusion in Section 4.

We use the full-potential linearized augmented-plane-waves method within the density functional theory (DFT)^[14,15] as implemented in the package WIEN2k.^[16] We use the mBJ exchange potential plus local density approximation (LDA) correlation potential for the exchange–correlation potential^[17] to do our main electronic structure calculations, and use the popular generalized gradient approximation of Perdew, Burke, and Ernzerhof (GGA-PBE)^[18] to optimize the internal position parameters with a force standard of 2 mRy/a.u. The full relativistic effects are calculated with the Dirac equations for the core states, and the scalar relativistic approximation is used for the valence states.^[19–21] The atomic sphere radii of Cr, Mo, and O are chosen to be 1.82 a.u., 1.78 a.u., and 1.61 a.u., respectively. We use a 11 × 11 × 6 Monkhorst–Pack k-points grid for the self-consistent calculation and make harmonic expansion up to l_max = 10 in each of the atomic spheres, and set R_mtk_max = 7. The self-consistent calculations are considered to be converged when the integration of the absolute charge-density difference between the input and the output electron densities is less than 0.0001|e| per formula unit, where e is the electron charge.

The Cr₂MoO₆ has an inverse-trirutile structure with the lattice parameters a = b = 4.58717 Å and c = 8.81138 Å at T = 4 K, which undergoes a paramagnetic–antiferromagnetic transition at T = 93 K with inter-bilayer antiferromagnetic coupling and intra-bilayer ferromagnetic coupling.^[13] The sketch map of Cr₂MoO₆ crystal structure is shown in Fig. 1. The experimental crystal structure is used as a reference. The hydrostatic pressure is simulated by using V/V_exp, where V_exp is the volume of the experimental crystal structure. The calculations are performed at six different V/V_exp, and the six calculated points are labeled as A (1.0), B (0.97), C (0.94), D (0.91), E (0.88), and F (0.85). Four different magnetic configurations denoted as A–B (AF–AF, F–AF, AF–F, and F–F) are considered, where A represents the inter-bilayer magnetic ordering and B represents the intra-bilayer magnetic ordering. In all calculations, the ionic positions are relaxed by using GGA-PBE. The magnetic energy differences as a function of V/V_exp are plotted in Fig. 2. The results show that the AF–F magnetic configuration with the experimental crystal structure is the ground state, which agrees well with the experiment and other GGA or GGA+U calculations.^[13] As the volume decreases, the magnetic energy difference between F–AF and AF–F magnetic configurations becomes negative, which implies a magnetic phase transition. The V/V_exp corresponding to the magnetic phase transition is about 0.882, and the ground state at A, B, C, and D points has the AF–F magnetic configuration, while the ground state at E and F points has the F–AF magnetic configuration. The magnetic energy differences also show that the F–F magnetic configuration is most unstable under the considered pressure.

Fig. 1.

	Figure Option ViewDownloadNew Window
	Fig. 1. The sketch map of Cr2MoO6 crystal structure. The black balls, blue balls, and red balls represent Cr atoms, Mo atoms, and O atoms, respectively. The Cr bilayers are separated by a Mo layer. The O atoms connecting inter-bilayer Cr atoms are marked as O1, and the O atoms connecting intra-bilayer Cr atoms are marked as O2. The labeled Mo1 and Mo2 atoms are equivalent.

Fig. 2.

	Figure Option ViewDownloadNew Window
	Fig. 2. The magnetic energy differences as a function of V/Vexp (AF–F magnetic configuration is used as a reference). The points represent calculated values, and the lines are obtained by linear or curve fitting. The inset shows the energy band gap as a function of V/Vexp.

As is well known, GGA generally does not accurately describe the position of transition-metal d electrons, and underestimates the gaps of magnetic insulators. The improved mBJ exchange potential has been proved to be very effective to describe electronic structures and gaps of magnetic insulators.^[5,22–25] The projected spin-dependent densities of states of Cr₂MoO₆ calculated by using mBJ and GGA are shown in Fig. 3. An obvious gap near the Fermi level is produced by using mBJ with respect to the GGA results, and another gap between Mo-d and Cr-d states in the conduction bands is opened. The calculated energy band gap is about 1.04 eV by using mBJ. It is clearly seen that the widths of the d character bands with mBJ become narrower than the ones with GGA. These results are in accordance with the related experiment and the GGA+U calculations in Ref. [13]. The calculated Cr magnetic moment is 2.26 μ_B by using GGA, and 2.50 μ_B with mBJ (the magnetic moment depends on the chosen atomic sphere radius of Cr). The mBJ result is closer to the experiment value 2.47 μ_B with respect to the GGA one. So, we use mBJ to study the electronic structures of Cr₂MoO₆ under hydrostatic pressure. The ground-state total densities of states of Cr₂MoO₆ are plotted in Fig. 4. Figures 4(a)–4(d) correspond to points A–D with AF–F magnetic ordering, and figures 4(e) and 4(f) correspond to points E and F with F–AF magnetic ordering. Because of the long-range ordered antiferromagnetic state of Cr₂MoO₆, the majority and minority channels are symmetric, and only the majority channel is shown. As the pressure increases, the energy band gap gradually decreases. When V/V_exp equals 0.88, the energy band gap disappears, which means a semiconductor–metal phase transition. The energy band gap as a function of V/V_exp is plotted in Fig. 2. With the increasing pressure, the gap shows a linear decrease with AF–F magnetic ordering. When the AF–F magnetic ordering changes into the F–AF one, the gap disappears, leading to a semiconductor–metal phase transition.

Fig. 3.

	Figure Option ViewDownloadNew Window
	Fig. 3. The partial spin-dependent densities of states of Cr2MoO6 projected in Cr, Mo, O1, and O2 atom spheres by using (a) mBJ and (b) GGA, respectively.

Fig. 4.

	Figure Option ViewDownloadNew Window
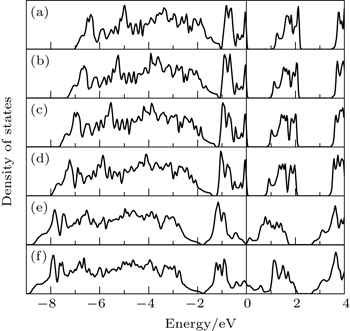	Fig. 4. The total densities of states of Cr2MoO6 with (a)–(d) AF–F and (e)–(f) F–AF magnetic configurations by using mBJ. Panels (a)–(f) correspond to points A–F, respectively.

In Refs. [12] and [13], the mechanism of the intra-bilayer ferromagnetic coupling was explained as follows. The virtual hopping of an O2-p electron to the empty Mo2-d states makes the O2-p state partially occupied, which creates a virtual hole in the O-p band and leads to ferromagnetic coupling between intra-bilayer Cr atoms. The hybridization strength between O1 (O2) and Mo1 (Mo2) is a key factor that determines the inter-bilayer (intra-bilayer) magnetic coupling. Since the distance between O1 and Mo1 and that between O2 and Mo2 control the hybridization strength, we plot the bond lengths of O1–Mo1 and O2–Mo2 (and their average values) as a function of V/V_exp with AF–F and F–AF magnetic configurations in Fig. 5. With the increasing of pressure, d₁ decreases more quickly than d₂ with both AF–F and F–AF magnetic configurations. To consider the combined effect of the two kinds of magnetic configurations, the average value of d₁ and d₂ is used to decide the hybridization strength of O and Mo. In Fig. 5(c), it is clearly seen that d₁ becomes smaller than d₂ with the pressure increasing, and then magnetic phase transition occurs. So, the hybridization strength between O and Mo controls the magnetic configuration of Cr₂MoO₆, and if the hybridization strength between O2 (O1) and Mo2 (MO1) is stronger than the one between O1 (O2) and Mo1 (Mo2), Cr₂MoO₆ will possess the AF–F (F–AF) magnetic configuration.

Fig. 5.

	Figure Option ViewDownloadNew Window
	Fig. 5. The bond lengths of O1–Mo1 (d1) and O2–Mo2 (d2) as a function of V/Vexp with (a) AF–F and (b) F–AF magnetic configurations, and (c) their average values.

To see clearly the magnetic ordering, we show the magnetization density distributions of Cr₂MoO₆ at points A (AF–F) and F (F–AF) with energy ranging from −8 eV to the Fermi level in Fig. 6, within the magnetization density −0.01–0.01 μ_B/Bohr³. Here, the plane is defined by a Cr1–Cr2–Cr3 triangle. The magnetization densities of the nearest O1 related with Cr1 and Cr4 (inter-bilayer coupling) with AF–F are very similar to the ones of the nearest O2 related with Cr1 and Cr2 (intra-bilayer coupling) with F–AF, and the related Cr couplings are all antiferromagnetic. It is clearly seen that the hybridizations among Cr1, Cr4, O1, and Mo1 at point F (F–AF) are very obvious, and the Cr and Mo1 spin densities are the same and opposite to the O1 spin densities, which agrees well with the mechanism of the ferromagnetic coupling in Refs. [12] and [13].

Fig. 6.

	Figure Option ViewDownloadNew Window
	Fig. 6. The magnetization density distributions of Cr2MoO6 at (a) A (AF–F) and (b) F (F–AF) points. The plane is defined by a Cr1–Cr2–Cr3 triangle. The actual magnetization density is −0.01–0.01 |e|/Bohr3 and the density increment is 0.00067 |e|/Bohr3. The blue, black, and red lines stand for positive, negative, and zero values, respectively.

The improved mBJ exchange potential is used to investigate the electronic structures of Cr₂MoO₆ under pressure. The x-ray emission spectra of delafossite type oxide CuFeO₂ (magnetic insulator) calculated by using mBJ are quite compatible with the experimental data.^[22] The mBJ gives an appropriate description for HgCr₂Se₄, CdCr₂S₄, and CdCr₂Se₄ (magnetic insulator), and the mBJ gaps agree well with the experimental values.^[5] So, the mBJ may be suitable to study the electronic structures of Cr₂MoO₆ under pressure.

We investigate the change of magnetic ordering and gaps of Cr₂MoO₆ under pressure. The calculated results show that magnetic and semiconductor–metal phase transitions in Cr₂MoO₆ are caused by the pressure. With the increasing pressure, the AF–F magnetic configuration transforms into the F–AF one, followed by a semiconductor–metal phase transition. The proposed mechanism of intra-bilayer ferromagnetic coupling in Cr₂MoO₆^[12,13] can be used to understand the magnetic phase transition, and the Mo–O hybridization strength plays a key role in determining the inter-bilayer and intra-bilayer magnetic ordering. We use the trend of bond lengths of Mo1–O1 and Mo2–O2 under pressure to explain the magnetic phase transition. The idea that empty Mo-d states by perturbing the O-p states can give rise to ferromagnetic coupling between two Cr atoms is further confirmed.