† Corresponding author. E-mail:
Project supported by the National Natural Science Foundation of China (Grant Nos. 61575077, 11271158, and 11574117).
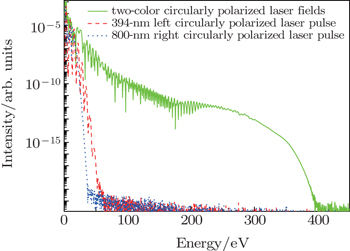
The generation of high-order harmonics and the attosecond pulse of the N2 molecule in two-color circularly polarized laser fields are investigated by the strong-field Lewenstein model. We show that the plateau of spectra is dramatically extended and a continuous harmonic spectrum with the bandwidth of 113 eV is obtained. When a static field is added to the x direction, the quantum path control is realized and a supercontinuum spectrum can be obtained, which is beneficial to obtain a shorter attosecond pulse. The underlying physical mechanism is well explained by the time–frequency analysis and the semi-classical three-step model with a finite initial transverse velocity. By superposing several orders of harmonics in the combination of two-color circularly polarized laser fields and a static field, an isolated attosecond pulse with a duration of 30 as can be generated.
In the past two decades, high-order harmonic generation (HHG) has been extensively studied as a powerful tool for generating isolated attosecond pulses (IAP)[1–5] and a coherent soft x-rays source.[6] The physical mechanism of the HHG is well established by the three step model:[7–9] the electron is freed by tunneling through the barrier of the atomic potential, then the ionized electron is accelerated by the laser field and captures energy, finally it may be driven back to the parent ion, emitting high energy photons. Compared with HHG from atoms, the process of harmonic emission from the molecules is much more complicated because of the interferences of different cores and complex molecular structures.
The potential applications of HHG from molecules such as real-time observation, molecular orbital tomographic imaging and reconstruction have attracted a lot of attention.[10–15] The effect of intramolecular interference was investigated theoretically in
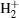
In this paper, we theoretically study the high-order harmonic generation of the N2 molecule in the two-color circularly polarized laser fields by the strong-field Lewenstein model. We show that a continuous harmonic spectrum is obtained by the two-color circularly polarized laser fields alone, and in the addition of a static field to the x direction, the quantum path control is realized. We perform the time–frequency analysis and the semi-classical three-step model to show the underlying physical mechanism. As a result, an isolated attosecond pulse with a duration of 30 as can be generated directly by the superposition of the supercontinuum harmonics.
The strong-field Lewenstein model has been widely used to investigate the HHG of atoms and molecules.[7,28,31,32] The transition amplitude for HHG can be calculated by the integral
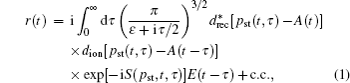
We investigate the HHG of the N2 molecule in the two-color circularly polarized laser fields, in which the HOMO constructed by the GAMESS-UK package[33] is taken as the initial state. The transition amplitudes along the x and y direction can be expressed respectively as
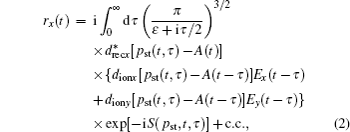
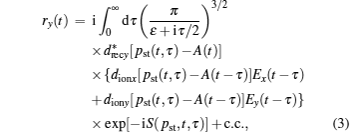
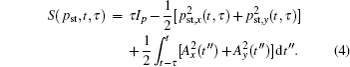
The harmonic spectrum intensity is proportional to the Fourier transformation of the time dependent dipole acceleration
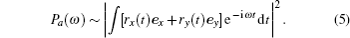
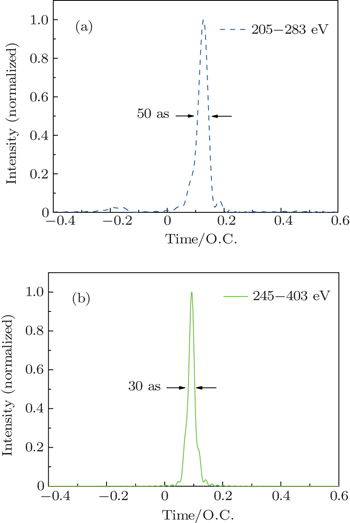
The temporal profile of an attosecond pulse can be obtained by superposing several harmonics in the x and y directions as follows:
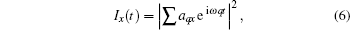
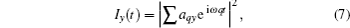
As we know, the electron ionized without an initial transverse velocity in the elliptical polarized laser field will miss the parent ion. A semi-classical three-step model with a proper initial transverse velocity was introduced, and the transverse displacement caused by the external field is compensated by an initial transverse velocity.[34–36] Thus the semi-classical electron trajectories can be obtained as
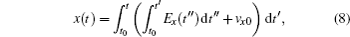
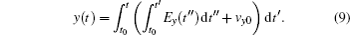
The initial velocities can be calculated as vx0 = v|| cos(α0) + v⊥ sin(α0) and vy0 = − v|| sin(α0) + v⊥ cos(α0), where v|| and v⊥ are the initial parallel velocity and transverse velocity, respectively. Since the electron leaves the molecule by tunneling, the initial velocity parallel to the ionizing field can be set as v|| = 0. α0 is the angle between the ionizing field vector and the coordinate axis at the ionization time t0. The recombination time with a proper finite initial velocity will be obtained by calculating x(t) = 0, y(t) = 0.
We investigate the HHG process of the N2 molecule in two-color circularly polarized laser fields and the HOMO is taken as the initial state as shown in Fig.
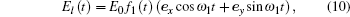
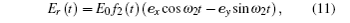
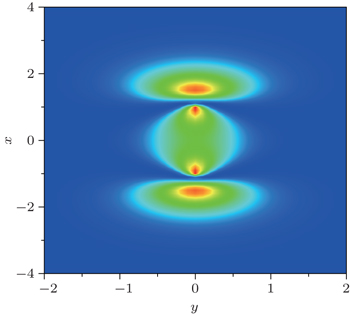 | Fig. 1. The highest occupied molecular orbital (HOMO) of the N2 molecule using the GAMESS-UK package. |
Figure
Figure
Fig.
Figure
To explain the underlying mechanism of the extension of the HHG plateau, we demonstrate the emission time of the harmonics along the x and y directions in terms of the time–frequency analysis as shown in Figs.
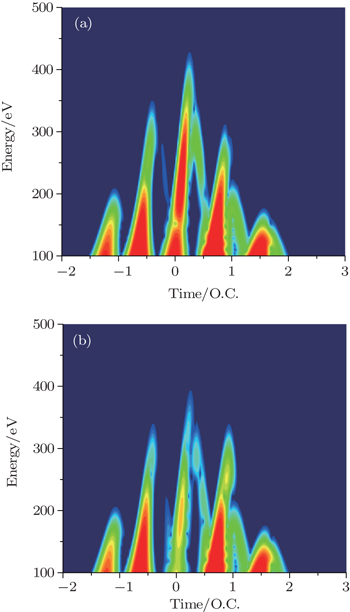 | Fig. 4. The time–frequency analysis of the HHG spectrum along (a) x direction and (b) y direction in the two-color circularly polarized laser fields. |
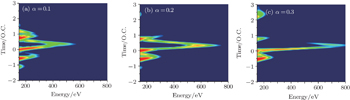
In order to extend the cut-off energy, we add a static electric field to the x direction and the expression is Estatic = α E0, where α is the static parameter. We investigate the HHG by the two-color circularly polarized laser fields combined with the static electric field for α = 0.1, α = 0.2, and α = 0.3. Figure
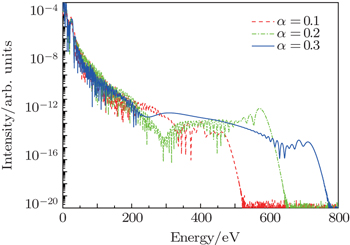 | Fig. 5. Harmonic spectrums generated by the two-color circularly polarized laser fields combined with a static electric field for the static parameters α = 0.1, α = 0.2, and α = 0.3, respectively. |
As the intensity of the harmonics for the x component is stronger by adding a static field in the x direction, we focus on the HHG process along the x direction. We demonstrate the corresponding time–frequency analysis of the spectrums along the x direction for three cases in Fig.
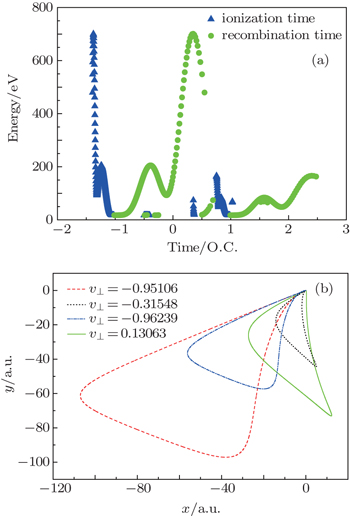
In order to further explain the mechanism of HHG in two-color circularly polarized laser fields combined with a static field for α = 0.3, we demonstrate the semi-classical three-step model with a finite initial transverse velocity, which is shown in Fig.
The electron trajectories around −1.35 O.C. with proper initial transverse velocities are shown in Fig.
Figure
We investigated the HHG and the attosecond pulse generation of the N2 molecule in two-color circularly polarized laser fields by the strong-field Lewenstein model. We show that a continuous spectrum of 113 eV can be generated by the two-color circularly polarized laser fields. By adding a static electric field to the x direction, the plateau of harmonic spectra is extended and a supercontinuum spectrum is generated. Both the time–frequency analysis and the semi-classical three-step model show that a dominant short quantum path is selected to contribute the harmonics in the higher energy region. By superposing several orders of harmonics in the combination of two-color circularly polarized laser fields and a static electric field, an isolated attosecond pulse with a bandwidth of 30 as is obtained directly.
| 1 | |
| 2 | |
| 3 | |
| 4 | |
| 5 | |
| 6 | |
| 7 | |
| 8 | |
| 9 | |
| 10 | |
| 11 | |
| 12 | |
| 13 | |
| 14 | |
| 15 | |
| 16 | |
| 17 | |
| 18 | |
| 19 | |
| 20 | |
| 21 | |
| 22 | |
| 23 | |
| 24 | |
| 25 | |
| 26 | |
| 27 | |
| 28 | |
| 29 | |
| 30 | |
| 31 | |
| 32 | |
| 33 | |
| 34 | |
| 35 | |
| 36 |