† Corresponding author. E-mail:
Project supported by the National Natural Science Foundation of China (Grant No. 11205162).
A three-dimensional model of the double-slot coupled cavity slow-wave structure (CCSWS) with a solid round electron beam for the beam–wave interaction is presented. Based on the “cold” dispersion, the “hot” dispersion equation is derived with the Maxwell equations by using the variable separation method and the field-matching method. Through numerical calculations, the effects of the electron beam parameters and the staggered angle between adjacent walls on the linear gain are analyzed.
The traveling wave tube with the coupled cavity slow wave structure (CCSWS) is well known for its ability to generate high peak and average powers as well as the moderate bandwidth.[1,2] As one of the most essential members of the CCSWS family, the double-slot CCSWS is more likely to be used at a higher voltage than the Hughes structure, which means a larger microwave output power.
The equivalent circuit model is the commonly used beam wave interaction method of the CCSWS. However, the parameters of this circuit are obtained from the experiment or the simulation software, as this model cannot directly provide a precise dispersion relation in the whole band from the geometrical dimensions.[3–5] In Ref. [6], the authors analyzed the high frequency characteristics of the double-slot CCSWS by building up a theoretical model using the boundary field matching method and the Green’s function, and the numerical results showed that the dispersion and interaction impedance calculated by this model are in good agreement with those calculated by the Ansoft HFSS code and the experimental results. Based on the above works, the “hot” dispersion equation, which describes the beam–wave interaction, was derived by using the self-consistent field theory and the boundary field matching method.[7,8] Then the influence of the beam parameters and the structure parameters on the small signal gain were investigated and discussed based on the numerical calculation.
For the double-slot CCSWS, the two slots on one wall are usually staggered by an angle of 180° from one to the other. The “cold” dispersion equation of the structure is[6]
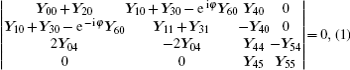
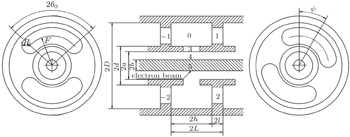
The configuration of the double-slot CCSWS, in which the guided waves interact with a solid round electron beam, is shown in Fig.
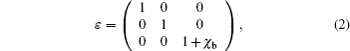
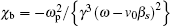
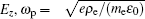
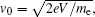
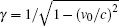
It can be seen from Eq. (
Based on the above analysis, the axial electric field component Ez of the TM mode in the drift tunnel can be obtained from the Helmholtz equation by employing the method of variable separation, while the transverse magnetic field component Hφ can then be expressed in terms of the corresponding Ez.
In region 5 (0 < r < b),
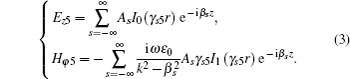
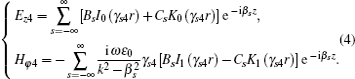
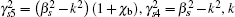
The boundary conditions of the tangent field components at r = b are
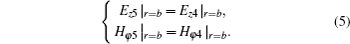
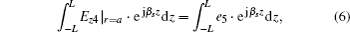
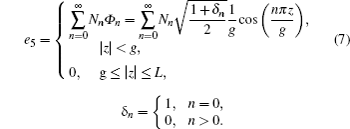
It is assumed that the electromagnetic field in each region is excited by the surface electric fields, so the magnetic field in region 4 can also be written as follows:[6]
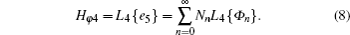
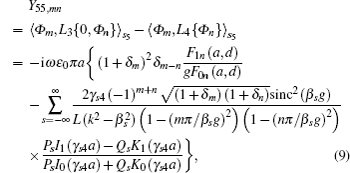
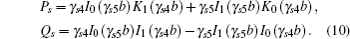
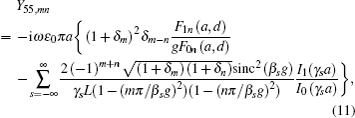
Thus, by substituting Eq. (
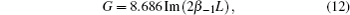
In order to illustrate the above theory, a special case of the Ka-band CCSWS with double staggered slots (Ψ = 90°) is employed. Table
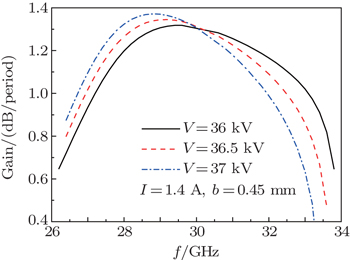
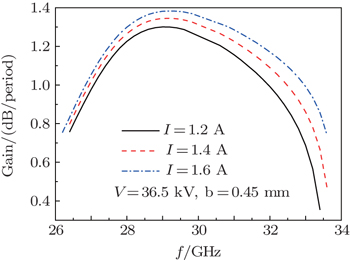
Figure
| Table 1. Dimensions of the Ka-band double-slot CCSWS used for simulation and analysis. . |
Figure
Then, the gain characteristics of two common double-slot CCSWS, the ones with in-line slots (ψ = 0°) and staggered slots (ψ = 90°), are investigated. To illustrate this issue more clearly, the high frequency characteristics of these two structures are shown in Fig.
Comparison of the high frequency characteristics of the Ka-band CCSWS with in-line (ψ = 0°) and overlap staggered (ψ = 90°) double slots.
Comparison of the small signal gain of the Ka-band CCSWS with in-line (ψ = 0°) and overlap staggered (ψ = 90°) double slots.
The hot dispersion equation for the double-slot coupled cavity slow-wave structure (CCSWS) with arbitrary staggered angle between two adjacent walls was developed by means of the self-consistent field theory. The influence of the beam parameters and the staggered angle between adjacent walls on the small signal gain was illustrated by numerical calculations. The results of the calculations show that, as the beam voltage and current increase, the maximum gain will augment. Meanwhile, the former factor will lead to a lower frequency range and a narrower bandwidth, whereas the latter factor has a great influence on the bandwidth. The analysis also indicates that, for the CCSWS with double in-line slots (ψ = 0°) and staggered slots (ψ = 90°) with the same dimensions, the former one is suitable to the requirement of high power, while the latter one keeps a better balance between the bandwidth and the gain, and has potential for application in medium-bandwidth high-power TWTs. This paper introduces a fast method to obtain the gain from the geometrical and electrical dimensions directly, which is helpful for the engineering design.
| 1 | |
| 2 | |
| 3 | |
| 4 | |
| 5 | |
| 6 | |
| 7 | |
| 8 | |
| 9 | |
| 10 |