The study of ion–solid collisions has great significance not only for fundamental research but also for technical application, like ion-beam modification and analysis of surfaces. In addition, the inner-shell electron excitation, ionization and transfer from one collision partner to the other, during ion–atom collisions, have been studied extensively both theoretically and experimentally for many years.^[1–8] Particularly, K-shell ionization has been most widely studied for a large range of projectile number and incident energy. However, even today, a complete theory that can predict K-shell vacancy production for all collision systems in different velocities is still lacking, especially at an intermediate energy. In this range, the inner-shell processes mentioned above may all play an important part in inducing vacancies. For example, in near-symmetric systems, vacancy transfer via 2pσ–1sσ radial coupling can result in both the projectiles and target atoms K-holes production, based on the Fano–Lichten model.^[9–11] Unfortunately, as for vacant 2pσ molecular orbitals (MOs) production, Morenzoni et al., Knudson et al., and Feldman et al. argued that they originate from 2pσ–2pπ electron promotion, whether or not there are vacancies in higher-Z partner L-shell, i.e., 2pπ vacant MOs, before interactions with target atoms.^[9,12–16] In contrast, Winters et al. and Mizogawa et al. argued that the light-Z partner K-shell electrons can be directly removed to a level higher than 2p or continuum states, and then these vacancies evolve to 2pσ and share between two partners. Only if the higher-Z partners bear the L-shell vacancies, will the 2pσ–2pπ electron promotion predominate the 2pσ generation.^[17,18] So far, it is not clear where the electron promotion picture breaks down and where the direct production processes become the dominant role when the higher-Z partners do not carry L-shell holes before entering the target. On the other hand, the detailed research on the vacancy sharing ratio between 2pσ and 1sσ as a function of incident energy is scarcely reported, since previous studies were mainly focused on searching for the origin of 2pσ production by different experimental designs. However, in order to confirm the individual inner-shell process, both the experimental sharing ratio and ionization cross sections should be investigated when no initial 2p-vacancies are brought into the collisions.

Toward this end, in the present paper, we extend the investigation to the detailed comparisons of both the sharing ratio and ionization cross sections with the theoretical predictions by 60–100 MeV Cu⁹⁺ ions incident on the thin Zn target. Specifically, we have measured the target and projectile K-shell ionization cross section ratio as a function of the incident energy. It shows very weak dependence on the incoming energies and agrees well with the prediction of K-vacancy sharing via the 2pσ–1sσ rotational coupling. Experimentally, it gives clear evidence for vacancy transfer between 2pσ–1sσ MOs. Besides, both the projectile and target ionization cross sections are measured, and physical mechanisms responsible for the initial Cu⁹⁺ ions K-vacancy production before 2pσ and 1sσ coupling are discussed. The total ionization cross sections give a good agreement with the Binary–Encounter approximation (BEA) calculations, while they deviate from the modified BEA model. Hence, in the present collision system and energy range, as supported by the experimental results, we are of the view that the initial K-holes direct production plays a predominant role in collisions.

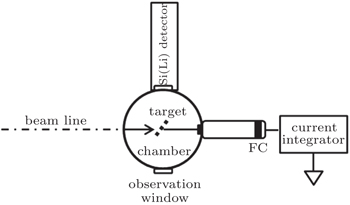
The experimental set-up is schematically shown in Fig. 1. The experiment was performed using HI-13 tandem accelerator, which usually provides a voltage from 2 MV to 13 MV for the terminal, at China Institute of Atomic Energy (CIAE). In our experiment, Cu⁹⁺ ions with kinetic energies 60, 65, 70, 80, 85, 90, and 100 MeV were used. The beams were collimated highly by a 2-mm aperture and strike on a thin Zn target at 45°. Then the ions were collected in a Faraday about 19.5 cm from the target. Beam currents were slightly lower than 10 nA before the target and just above 10 nA after passing through the target. In order to reduce the statistical errors, 1500 s was taken for each measurement. The x-rays produced by beam–target interactions were probed by a Si(Li) detector with a 10-mm diameter placed at 90° relative to the incident direction of the beams. The Si(Li) detector had a resolution of 185 eV at 5.9 keV and a solid angle of 6.22 msr. The energy calibration was made by using Am²⁴¹ both before and after the experiment. The dead time was less than 5% during the measurement. In addition, the target chamber was sealed up with a Be window about 50 μm, installed between the detector and the target. The vacuum in the target chamber was kept at 4×10⁻⁴ Pa in our experiment. Besides, the targets used in this work were prepared by evaporating Zn of purity 99.99% on carbon foils of 15 μg/cm² in a vacuum system about 10⁻⁵ mbar, and had a thickness of 15 μg/cm² with uncertainties about 17.3%.

Fig. 1.

	Figure Option ViewDownloadNew Window
	Fig. 1. Schematic diagram of the experimental set-up.

Typical x-ray spectra taken with the Si(Li) detector are shown in Fig. 2 for Cu⁹⁺ ion interactions with the Zn target at 65 MeV. On the basis of energetics, the peak (A) arises from the projectile Cu⁹⁺ 2p→1s transitions, including Kα₁ and Kα₂ x-rays. The interesting thing is the appearance of the peak (B), which has contribution from 2p→1s transitions for the target Zn atoms. The simultaneous observation of target and projectile Kα (Kα₁ + Kα₂) x-rays give direct evidence that K-shell vacancies have been created both in Zn atoms and Cu⁹⁺ ions.

Fig. 2.

	Figure Option ViewDownloadNew Window
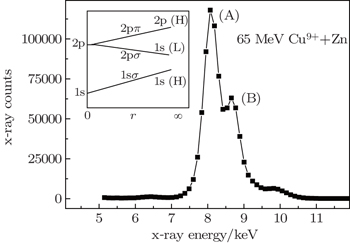	Fig. 2. The x-ray spectra emitted in collisions Cu9+ + Zn at 65 MeV. The inset in the upper left corner is a simple molecular orbital correlation diagram for Cu+Zn system.[10,11]

To explain production of both projectile and target K-holes, we first present a simple molecular orbital correlation diagram (the insert in Fig. 2) for the Cu + Zn system according to the Fano–Lichten model.^[10,11] In their model, the radial coupling between 2pσ and 1sσ MOs can take place after 2pσ–2pπ electron promotion at a certain inter-nuclear distance r.^[12] The 2pσ, 1sσ, and 2pπ MOs correspond to 1s shell of the lower-Z partner (1s (L)), 1s and 2p shell of the higher-Z partner (1s (H) and 2p (H)).

Specifically, as partners retreat, the 1sσ electrons transfer to the unoccupied 2pσ MOs via the radial coupling 2pσ–1sσ with a certain probability W of less than 1/2. Here, 1sσ and 2pσ MOs evolve from Zn 1s and Cu⁹⁺ 1s atomic orbitals, respectively. After they completely separate, part of the vacancies exist in the 1s-shell of the Zn atomic orbitals. Hence, both the projectile ions and the target atoms are in 1s-ionized state after the collisions. Moreover, obviously, the ratio of K-shell ionization cross sections for the target to the projectile ions strongly reflects the vacancy ratio R in 1sσ to 2pσ, which equals to W/(1−W). The experimental ratio σ(Zn)/σ(Cu) as a function of the incoming energy is shown in Fig. 3. The errors of the ratio are about 26%, including statistical and systematic errors. As can be seen in the figure, the ionization cross section ratio almost remains stable during the energy range studied.

Fig. 3.

	Figure Option ViewDownloadNew Window
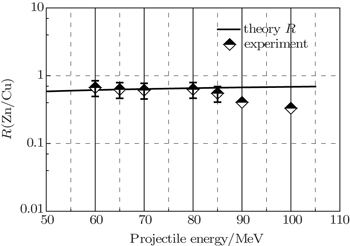	Fig. 3. Ratio of the K-shell ionization cross sections between Zn and Cu9+ ions, σ(Zn/Cu), as a function of the incident energy. The solid line represents the ratio value proposed by Meyerhof.[9]

Based on the above physical process and the procedure presented by Meyerhof, the transfer probability W between 2pσ and 1sσ MOs can be expressed as^[9]

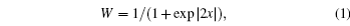
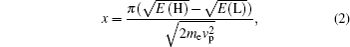
Hence, the K-vacancy sharing ratio between the target and the projectile, R, is expressed by

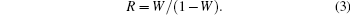
However, it should turn our attention to K-vacancies production in projectile Cu⁹⁺ ions since the collision systems bring no initial K- or L-shell holes into the interactions. The relative velocity between two partners in the present experiment is about 1/5 to 1/3 of the Cu⁹⁺ 1s electron velocity, therefore, one explanation is that molecular-orbital (MO) effect might reasonably be expected for the production of the 2pσ vacancies, i.e., Cu⁹⁺ K-vacancies. To be specific, the Zn 2p vacancies are first formed in an initial collision and the vacancies are transferred along 2pπ to 2pσ orbitals resulting in Cu⁹⁺ ions 1s-holes in a second violent collision. Meanwhile, another plausible interpretation to account for our experimental data is the direct ionization (and/or excitation) bringing the Cu⁹⁺ ions 1s-holes as the initial 2p vacancies are not present in target Zn for this work. The above two processes for Cu⁹⁺ ions K-vacancies production cross sections before 2pσ–1sσ sharing are described by the binding energy modified BEA model and BEA model, respectively.

In searching for a more accurate origin of the Cu⁹⁺ ions 1s-vacancies, first, the experimental ionization cross sections, σ(cm²/atom), are given, which are deduced from the relation^[20,21]

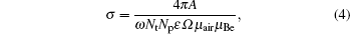
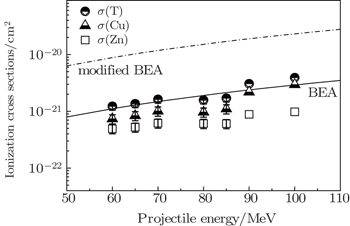
The experimental projectile K-shell ionization cross sections σ(Cu), the target K-shell ionization cross sections σ(Zn), and the total ionization cross sections σ(T) versus the indent energies are shown in Fig. 4. As a note, the sum of σ(Cu) and σ(Zn) is assumed to represent the total experimental Cu⁹⁺ ion K-shell ionizations σ(T) before 2pσ–1sσ radial coupling, and it is independent of the subsequent vacancy sharing process. Uncertainties of the ionization cross sections σ(Cu) and σ(Zn) are estimated to be ±18.4%, including statistical and systematic errors. In addition, uncertainties of σ(T) are estimated to be ±14.5%. Specifically, the contributions to these errors involve the number of incident ions ±3%, the solid angle and air absorption ±0.1%, the efficiency of the detector ±2% and the x-ray counts ±5%, respectively. Clearly, the experimental results illustrate that σ(Cu) and σ(Zn) increase with the impact energy increasing, and the cross sections for the projectile are observed to be very similar to the target atom in magnitude.

Fig. 4.

	Figure Option ViewDownloadNew Window
	Fig. 4. Dependence of Cu9+ K-shell and Zn K-shell ionization cross sections on the incident energy. The solid curve represents the theoretical results calculated by the BEA model and the top dashed line represents modified BEA calculations.

Next, theoretical ionization cross sections calculated by the BEA model and the binding energy modified BEA are established. In the analysis below, Cu⁹⁺ beams and Zn atoms play a role of “target” and “projectile”, respectively, as K-vacancies produce in Cu⁹⁺ ions first. The detail of the models will be discussed in the following.

According to the BEA model, the K-shell ionization cross sections are given by^[24]

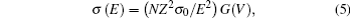
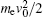
Nevertheless, it is worth noticing that, in Figs. 3 and 4, small disagreements between the theoretical predictions and our experimental data are observed at higher energies 90 and 100 MeV, where the target has been used for 11.25 h. Hence, one possible reason for these disagreements is that long hours of bombardments make the target charred as dark dots (C) and folds in the target are observed. Obviously, in this case, beam interactions with the carbon atoms before with the target will increase initial Cu⁹⁺ ions K-shell ionization cross sections, i.e., σ(T). Moreover, only K-vacancies induced by Zn atoms will take part in the subsequent sharing between 2pσ and 1sσ MOs, since the transfer occurs during the separation of the united-partner.^[9] Therefore, a smaller ratio value occurs at these last two points in our experiment, which is in agreement with that in Fig. 3. Of course, the beam instability in the experiment for two energies could also lead to some additional uncertainties, and the above analysis is estimating the lack of uncertainty for the ionization cross sections. Therefore, no error bars for (Zn), σ(Cu), σ(T) and σ(Zn)/σ(Cu) are given in 90 and 100 MeV. In spite of some differences involved in the higher incident energies, it is evident that both experimental σ(T) and σ(Zn)/σ(Cu) exhibit the same order of magnitude as the theories predicted. In addition, we conclude that these slight disagreements have no effect on the previous discussion on the birth of Cu⁹⁺ ions 1s-holes and their sharing between 2pσ–1sσ MOs in our paper.

Both the target and projectile K-shell ionization cross sections have been measured in 60–100 MeV near-symmetric collisions of Cu⁹⁺ ions with the thin target Zn. The data show that even if the projectile does not carry initial L-shell vacancies, the inner-shell ionization can occur in the projectile and the target and both ionization cross sections are of the same order of magnitude. The ratio of target to projectile K-shell ionization exhibits a very weak dependence on projectile energy and agrees well with the K-vacancy sharing predictions. Besides, the total ionization cross sections are found to be in fair agreement with the BEA model, while the modified BEA calculations do not reproduce our result. Hence, we conclude that 2pπ–2pσ rotational coupling fails to explain the initial K-holes production, while the Cu⁹⁺ ions direct ionization (and/or excitation) is the dominant process in the present work. In summary, a violent collision directly induces a K-vacancy in Cu⁹⁺ ion, which shares between two partners, subsequently, and results in both the Zn atom and Cu⁹⁺ ion being in K-ionization state, finally. However, at what Z and incident energy the direct process will play a dominant role when there is no L-shell vacancy in higher-Z partner is still not clear, so further experimental and more thorough theoretical studies on K and higher n-shell vacancy production in ion–atom collisions are required to clarify the situation.