New variable separation solutions for the generalized nonlinear diffusion equations

Cite this Article

Ji Fei-Yu, Zhang Shun-Li. New variable separation solutions for the generalized nonlinear diffusion equations. Chinese Physics B, 2016, 25(3): 030202 Copy to clipboard

Permissions

New variable separation solutions for the generalized nonlinear diffusion equations

Ji Fei-Yu^{1, †,}, Zhang Shun-Li²

1School of Science, Xi’an University of Architecture and Technology, Xi’an 710055, China

2Center for Nonlinear Studies, School of Mathematics, Northwest University, Xi’an 710069, China

† Corresponding author. E-mail: feiyuji@xauat.edu.cn

Project supported by the National Natural Science Foundation of China (Grant Nos. 11371293, 11401458, and 11501438), the National Natural Science Foundation of China, Tian Yuan Special Foundation (Grant No. 11426169), and the Natural Science Basic Research Plan in Shaanxi Province of China (Grant No. 2015JQ1014).

Abstract

The functionally generalized variable separation of the generalized nonlinear diffusion equations u_t = A(u,u_x)u_xx + B(u,u_x) is studied by using the conditional Lie–Bäcklund symmetry method. The variant forms of the considered equations, which admit the corresponding conditional Lie–Bäcklund symmetries, are characterized. To construct functionally generalized separable solutions, several concrete examples defined on the exponential and trigonometric invariant subspaces are provided.

PACS: 02.30.Jr;02.20.Sv;02.30.Ik;

Keyword:conditional Lie–Bäcklund symmetry;functionally generalized separable solution;generalized nonlinear diffusion equation;invariant subspace;

Show Figures

1. Introduction

It is well known that symmetry-related methods play important roles in studying symmetry reductions and constructing exact solutions to nonlinear partial differential equations (NPDEs). Up to now, a number of effective approaches have been presented. For instance, the Lie point symmetry method,^[1–4] also known as the classical symmetry method due to Sophus Lie in 1881, is regarded as the most popular technique. The nonclassical symmetry method,^[5–12] as an extension of Lie’s classical method, which was introduced by Bluman and Cole, can be applied to find more types of exact solutions to some NPDEs. In comparison with the symmetry method, the direct method^[13–17] proposed by Clarkson and Kruskal can be used to realize reductions of NPDEs without any reference to the Lie group theory. Here we especially emphasize the conditional Lie–Bäcklund symmetry (CLBS) method introduced by Fokas and Liu^[18] and Zhdanov,^[19] which is a natural extension of the nonclassical symmetry method. For that matter, the algorithm of the CLBS is similar to that of the nonclassical symmetry. From the point of view of the ansatz form of the solution, we now focus on the following two kinds of ansatzs. One is the functional separable solution (FSS)^[20,21]

The other is the derivative-dependent functional separable solution^[22,23]

which is an extension of the former. These two variable separation approaches based on their corresponding CLBSs have been widely applied to classify and construct exact solutions of some nonlinear evolution equations.

In this paper, we develop the CLBS method to study the functionally generalized separable solutions (FGSSs)

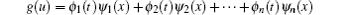
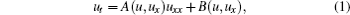
to the generalized nonlinear diffusion equations

where A(u,u_x) and B(u,u_x) are smooth functions of the indicated variables to be settled. Obviously, the FGSS is an extension of the FSS. The FSSs to Eq. (1) were solved by using CLBS.^[24] Furthermore, seeking for the approximate FSSs of Eq. (1) with perturbation was taken by way of approximate CLBS.^[25]

The layout of this paper is as follows. In Section 2, we review some basic definitions and fundamental theorems on the CLBS method and the invariant subspace (IS) method. In Section 3, the classification of Eq. (1) admitting certain CLBSs and the corresponding ISs are presented. In Section 4, some FGSSs to the resulting equations are constructed by way of examples. The last section gives the conclusion and discussion.

2. Preliminaries

For convenience, we review some basic facts on the CLBS and the IS methods for the nonlinear evolution equations.^[18–23]

Consider the k-th order nonlinear evolution equation

let

be an evolutionary vector field and η = η(t,x,u,u₁,u₂,…,u_n) be its characteristic, where the total derivative operators are defined as

Definition 1 The evolutionary vector field (3) is said to be a Lie–Bäcklund symmetry of Eq. (2) if and only if

where [L] is the set of all the differential consequences of Eq. (2), that is

Definition 2 The evolutionary vector field (3) is said to be a CLBS of Eq. (2) if and only if

where [M] denotes the set of all the differential consequences of equation η = 0 with respect to x, that is

j = 0,1,2,….

Proposition 1^[18,19] Equation (2) admits the CLBS (3) if there exists a function S(t,x,u,η) so that

where [E,η] = E′η − η′E, the prime denotes the Fréchet derivative, and S is an analytic function of t, x, u, u_x,…and η, D_xη,

….

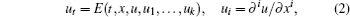
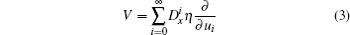
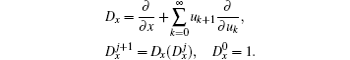
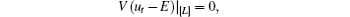
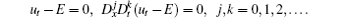
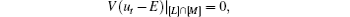
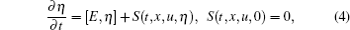
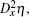
Following this proposition, equation (2) admits the CLBS (3) with the characteristic η if

Let

be a characteristic of Eq. (1), where [g(u)]_ix = ∂ⁱ(g(u))/∂xⁱ.

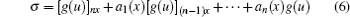
If equation (1) admits CLBS (6), then equation

admits CLBS

In fact, equation (1) is related to Eq. (7) via

where the primes denote the derivatives with respect to u, and u = f(v) denotes the inverse function of v = g(u). To proceed, the following proposition is necessary.

Proposition 2 Equation (7) admits the CLBS with the characteristic (8) if and only if

where the prime denotes the Fréchet derivative, and

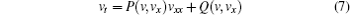
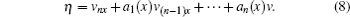
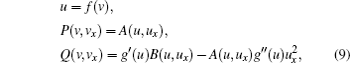
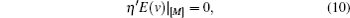
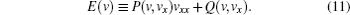
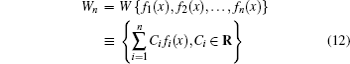
By Proposition 2, we conclude that equation (10) is right, the invariance condition of the subspace

with respect to the operator E, where W_n is the solution space of η = v_nx + a₁(x)v_(n−1)x + … + a_n(x)v = 0.

Thus it is easy to know that E[W_n] ⊂ W_n, that is

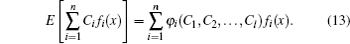
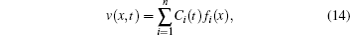
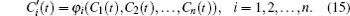
If equation (7) admits CLBS (8), then equation (7) has the generalized separable solution (GSS)

where the coefficients {C_i(t)} are determined by the first order dynamical system

To estimate the dimension of the IS admitted by the operator E, the following theorem is helpful.

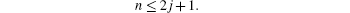
Theorem 1^[26] If linear space W_n is invariant with respect to the nonlinear operator E of order j, then

In terms of this theorem, we investigate CLBSs (8) with 2 ≤ n ≤ 5 for Eq. (7).

3. Equation (7) admitting GSSs (14)

We first consider the case of n = 2.

By proposition 2, equation (7) admits CLBS (8) if

where P = P(v,v_x), Q = Q(v,v_x), and a_i = a_i(x) (i = 1,2). The primes denote the derivatives with respect to x, and the subscripts denote the partial derivatives with respect to the indicated variables.

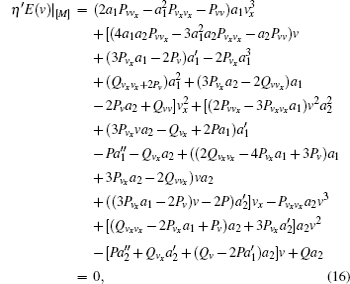
Because not all solutions of Eq. (16) can be obtained, we just present some special solutions listed in Table 1. It is worth noting that the following cases:

are two special cases in Eq. (7).

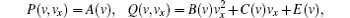
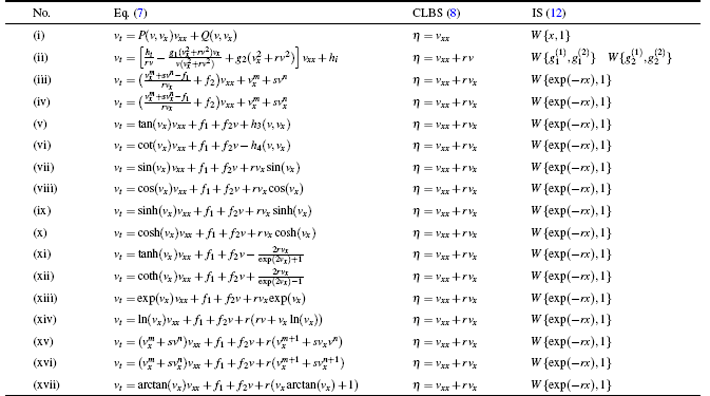
Table 1.

CLBSs (8) and ISs with n = 2 of Eq. (7).

In Table 1,

and r, s, m, and n are arbitrary constants in their sets of definition when not specified.

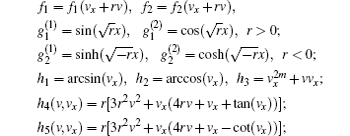
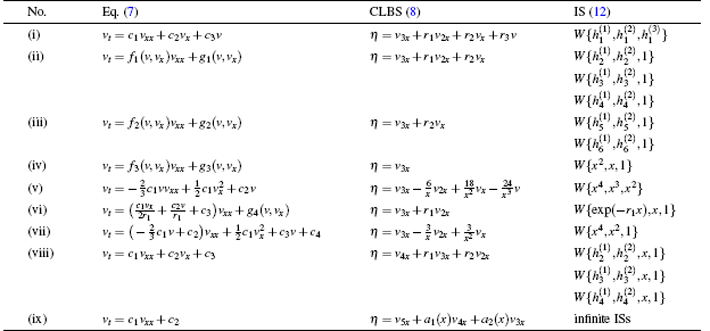
In the same way, we can obtain the determining systems for n = 3,4,5. Since the determining systems are quite complicated, they are omitted here. Finally, a complete classification to CLBSs (8) and ISs (12) with n = 3,4,5 of Eq. (7) is presented in Table 2.

In Table 2,

and r_i, c_i (i ∈ N) are arbitrary constants in their sets of definition when not specified.

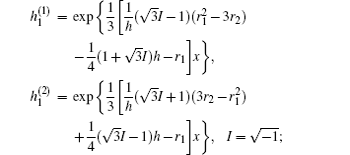
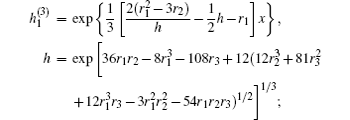
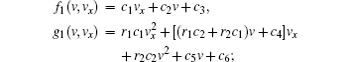
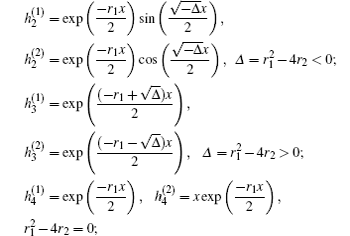
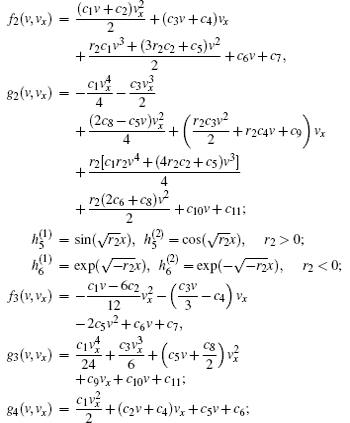
Table 2.

CLBSs (8) and ISs with n = 3,4,5 of Eq. (7).

4. Some GSSs of Eqs. (1) and (7)

In this section, we construct GSSs to the equations obtained in the last section. These solutions are determined explicitly with some finite-dimensional dynamical systems. We take several examples to illustrate the calculation procedure.

Example 1 The equation

admits the CLBS

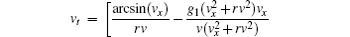
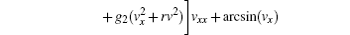
By the transformation v = g(u), we can obtain the corresponding FGSS of the equation

where

Example 2 The equation

admits the CLBS

The corresponding GSS is

where ϕ₁(t) and ϕ₂(t) satisfy the following dynamical system:

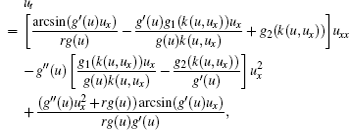
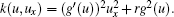
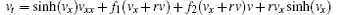
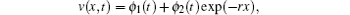
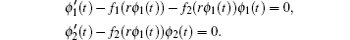
By the appropriate transformation v = g(u), we can obtain the FGSS of the equation

where l(u,u_x) = g′(u)u_x + rg(u).

Example 3 The equation

admits the CLBS

The corresponding GSSs are given as follows.

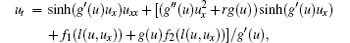
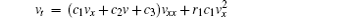
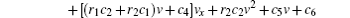
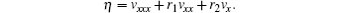
By the appropriate transformation v = g(u), we can obtain the FGSSs of the equation

from the above GSSs of Eqs. (17)–(19) respectively.

Example 4 The equation

admits the CLBS

The corresponding GSSs are given below.

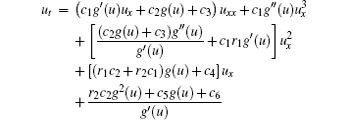
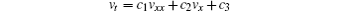
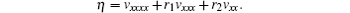
By the appropriate transformation v = g(u), we can respectively obtain the FGSSs of the equation

from the above GSSs of Eqs. (20)–(22).

5. Discussion and conclusion

By using the CLBS and IS methods, we have studied the problem of FGSSs for the generalized nonlinear diffusion equations. Those equations admitting the corresponding CLBSs with ISs have been obtained and displayed in tables. Finally, we have derived the FGSSs of some resulting equations. In general, these solutions cannot be obtained by other relevant approaches.

There are still two interesting questions to be answered in the future: (i) How to develop the CLBS method with IS to investigate the FGSSs of other types of evolution equations, such as higher-dimensional equations? (ii) How to solve the approximate FGSS of NPDEs with perturbation terms? We expect that certain other types of NPDEs could be investigated by means of the CLBS and the IS approaches, and some new results would be obtained sooner or later.

Reference

1	Bluman G WKumei S1989Symmetries and Differential EquationsNew YorkSpringer-Verlag
2	Olver P J1993Applications of Lie Groups to Differential EquationsNew YorkSpringer-Verlag
3	Ibragimov N H1985Transformation Groups Applied to Mathematical PhysicsBostonReidel
4	Ovsiannikov L V1982Group Analysis of Differential EquationsNew YorkAcademic Press
5	Bluman G WCole J D1969J. Math. Mech.181025
6	Ames W F1972Nonlinear Partial Differential Equations in EngineeringNew YorkAcademic Press
7	Levi DWinternitz P 1989 J. Phys. A: Math. Gen. 22 2915
8	Wang J YTang X YLiang Z FLou S Y 2015 Chin. Phys. B 24 050202
9	Hu X RChen Y 2015 Chin. Phys. B 24 090203
10	Liu X ZYu JR B 2015 Chin. Phys. B 24 030202
11	Wang XMa T BNing J G 2014 Chin. Phys. Lett. 31 030201
12	Yi L NTaogetusang 2015 Acta Phys. Sin. 64 020201 (in Chinese)
13	CLarkson P AKruskal M D 1989 J. Math. Phys. 30 2201
14	Lou S Y 1990 Phys. Lett. A 151 133
15	Fuschch W IZhdanov R Z 1994 J. Nonlinear Math. Phys. 1 60
16	King J R 1993 Physica D 64 35
17	Hill J MAvagliano A JEdwards M P 1992 IMA J. Appl. Math. 48 283
18	Fokas A SLiu Q M 1994 Phys. Rev. Lett. 72 3293
19	Zhdanov R Z 1995 J. Phys. A: Math. Gen. 128 3841
20	Qu C ZZhang S LLiu R C 2000 Physica D 144 97
21	Feng WJi L N 2013 Physica A 392 618
22	Zhang S LLou S YQu C Z 2003 J. Phys. A: Math. Gen. 36 12223
23	Di Y MZhang D DShen S FZhang J 2014 Appl. Math. Model. 38 4409
24	Zhang S LLou S YQu C Z 2002 Chin. Phys. Lett. 19 1741
25	Ji F YYang C X 2013 Chin. Phys. B 22 100202
26	Galaktionov V ASvirshchevskii S R2007Exact Solutions and Invariant Subspaces of Nonlinear Partial Differential Equations in Mechanics and PhysicsLondonChapman and Hall/CRC